CBSE Sample Papers Class 11 Mathematics 2025

CBSE Sample Paper for Class 11 Mathematics

Download Free Class 11 Mathematics Sample Papers 2024-25 from myCBSEguide As per the newly released CBSE marking scheme and blueprint for Class 11, myCBSEguide provides updated Class 11 Mathematics sample papers 2024-25. These sample papers are designed to match the latest exam pattern and are available for free download in PDF format on the myCBSEguide app and myCBSEguide website.To excel in the upcoming exams, practicing CBSE Sample Papers Class 11 Mathematics is essential for students. These sample papers offer a detailed overview of the exam format and the types of questions students can expect. By regularly working through CBSE Sample Papers Class 11 Mathematics, students can enhance their problem-solving abilities and improve time management skills. Moreover, CBSE Sample Papers Class 11 Mathematics help students identify key areas of improvement by providing solutions to difficult problems. The latest CBSE Sample Papers Class 11 Mathematics are designed according to the current syllabus and exam pattern, ensuring that students are fully prepared for the board exams. Make sure to download and practice these sample papers to boost your confidence and achieve high marks in your Class 11 exams. Practicing CBSE Sample Papers Class 11 Mathematics is one of the most effective ways to prepare for your exams. The Class 11 Mathematics sample papers with solutions will help students thoroughly understand the exam format, improve problem-solving skills, and enhance exam preparation. By practicing these sample papers, students can gain confidence and boost their chances of scoring high marks in the Class 11 exams. Download your CBSE Class 11 Mathematics Sample Papers 2024-25 today from myCBSEguide and start preparing effectively for your exams.

Download Mathematics Sample Papers as PDF

CBSE Sample Papers Class 11 Mathematics 2024-25– in PDF

myCBSEguide offers CBSE Class 11 Mathematics sample papers for the 2024-25 session with solutions in PDF format for free download. These new pattern sample papers are designed to align with the latest syllabus, marking scheme, and blueprint for the current academic year. We recommend that students download these Class 11 Mathematics model papers and practice them regularly to familiarize themselves with the updated exam format. The Class 11 Mathematics sample papers on myCBSEguide are designed to boost students’ problem-solving skills and exam readiness. These sample papers, available on both the myCBSEguide app and website, are crafted based on the latest exam pattern and blueprint issued by CBSE for the 2024-25 session. Download the Class 11 Mathematics sample papers today from myCBSEguide and start practicing to excel in your exams. These papers provide a real-time exam experience, helping students get accustomed to the question format and time constraints.

Marking Scheme for the Class 11 exam 2025

CBSE has made significant updates to the marking scheme for the 2024-25 exams, as reflected in the latest model papers. This year, the exam will primarily feature subjective questions worth 2, 3, and 5 marks. Additionally, there is a fair weightage of objective-type questions, including MCQs, ARQs (Assertion & Reason), and CBQs (Case Study Questions). Given this balanced approach, it’s crucial for students not to ignore any section, as each contributes to the overall score. By solving CBSE Sample Papers Class 11 Mathematics, students can identify their strengths and weaknesses, which is crucial for focused revision.

We strongly advise against the selective study method. Instead, aim to cover the entire syllabus on time and revise consistently for better retention. By practicing a variety of question types and focusing on both subjective and objective sections, students can improve their performance in the upcoming CBSE board exams. The CBSE Sample Papers Class 11 Mathematics are aligned with the current syllabus, ensuring that the practice is relevant and up-to-date with the latest exam pattern.

Class 11 – Mathematics Sample Paper (2024-25)

---

Class 11 – Mathematics
Sample Paper – 01 (2024-25)

---

Maximum Marks: 80
Time Allowed: : 3 hours

---

General Instructions:

1. This Question paper contains 38 questions. All questions are compulsory.
2. This Question paper is divided into five Sections – A, B, C, D and E.
3. In Section A, Questions no. 1 to 18 are multiple choice questions (MCQs) and Questions no. 19 and 20 are Assertion-Reason based questions of 1 mark each.
4. In Section B, Questions no. 21 to 25 are Very Short Answer (VSA)-type questions, carrying 2 marks each.
5. In Section C, Questions no. 26 to 31 are Short Answer (SA)-type questions, carrying 3 marks each.
6. In Section D, Questions no. 32 to 35 are Long Answer (LA)-type questions, carrying 5 marks each.
7. In Section E, Questions no. 36 to 38 are Case study-based questions, carrying 4 marks each.
8. There is no overall choice. However, an internal choice has been provided in 2 questions in Section B, 3 questions in Section C, 2 questions in Section D and one subpart each in 2 questions of Section E.
9. Use of calculators is not allowed.

---

1. Section A
2. If x is an acute angle and tan x = {tex}\frac{1}{\sqrt{7}}{/tex}, then the value of {tex}\frac{\operatorname{cosec}^{2} x-\sec ^{2} x}{\operatorname{cosec}^{2} x+\sec ^{2} x}{/tex} is

   a){tex}\frac {1} {2}{/tex}

   b){tex}\frac {5} {4}{/tex}

   c)2

   d){tex}\frac {3} {4}{/tex}

3. Let f (x) = cos^-1 2x  then, dom f(x) = ?

   a)[-1,1]

   b){tex}\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]{/tex}

   c){tex}\left[\frac{-1}{2}, \frac{1}{2}\right]{/tex}

   d){tex}\left[\frac{-\pi}{4}, \frac{\pi}{4}\right]{/tex}

4. A fair coin is tossed repeatedly. The probability of getting a result in the fifth toss different from those obtained in the first four tosses is

   a){tex}\frac{31}{32}{/tex}

   b){tex}\frac{1}{2}{/tex}

   c){tex}\frac{1}{32}{/tex}

   d){tex}\frac{1}{16}{/tex}

5. The derivative of {tex}\sec ^{-1}\left(\frac{1}{2 x^2-1}\right){/tex} with respect to {tex}\sqrt {1 – {x^2}} {/tex} at x = {tex}\frac{1}{2}{/tex} is

   a)2

   b)4

   c)1

   d)-2

6. Slope of a line is not defined if the line is

   a)parallel to the line x – y

   b)parallel to the line x + y

   c)parallel to Y axis

   d)parallel to X axis

7. If A = {2, 3, 4, 8, 10}, B = {3, 4, 5, 10, 12} and C = {4, 5, 6, 12, 14}, then (A {tex}\cup{/tex} B) {tex}\cap{/tex} (A {tex}\cup{/tex} C) is equal to

   a){4, 5, 8, 10, 12}

   b){2, 4, 5, 10, 12}

   c){3, 8, 10, 12}

   d){2, 3, 4, 5, 8, 10, 12}

8. The value of {tex}\frac {\left(i^{5}+i^{6}+i^{7}+i^{8}+i^{9}\right)} {(1 + i)}{/tex} is

   a)1

   b){tex}\frac{1}{2}{/tex} (1 + i)

   c){tex}\frac{1}{2}{/tex}

   d){tex}\frac{1}{2}{/tex} (1 – i)

9. Consider the non – empty set consisting of children in a family and a relation R defined as aRb if a is brother of b. Then R is

   a)both symmetric and transitive

   b)transitive but not symmetric

   c)neither symmetric nor transitive

   d)symmetric but not transitive

10. If x and a are real numbers such that a > 0 and |x| > a, then

    a){tex}x \in(-a, \infty){/tex}

    b){tex}x \in(-\infty,-a) \cup(a, \infty){/tex}

    c)x {tex}\in{/tex} (-a, a)

    d){tex}x \in[-\infty, a]{/tex}

11. The value of cos (36°-A ) cos(36° + A) + cos (54° + A) cos(54° – A) is

    a)sin 3A

    b)cos 2A

    c)sin 2A

    d)cos 3A

12. For any two sets A and B, {tex}A \cap (A \cup B) = ….{/tex}

    a)A

    b){tex}\phi{/tex}

    c){tex}\ne{/tex}{tex}\phi{/tex}

    d)B

13. If {tex}\frac{1}{3}{/tex}, x₁, x₂, 9 are in GP then x₂ = ?

    a)3

    b)Cannot be determined

    c)6

    d)1

14. {tex}\sqrt 5 \left\{ {{{(\sqrt 5 + 1)}^{4}} – {{\left( {\sqrt 5 – 1} \right)}^{4}}} \right\}{/tex}is

    a)0

    b)212

    c)240

    d)224

15. If a, b, c are real numbers such that a {tex}\leq{/tex} b, c < 0, then

    a)ac {tex}\leq{/tex} bc

    b)ac > bc

    c)ac {tex} \geq{/tex} bc

    d)ac < bc

16. Which of the following is a null set?

    a)C = {tex}\phi {/tex}

    b)B = {x : x + 3 = 3}

    c)D = {0}

    d)A = {x : x > 1 and x < 3}

17. If cos {tex}\theta=\frac{4}{5}{/tex} and cos {tex}\phi=\frac{12}{13}{/tex}, where {tex}\theta{/tex} and {tex}\phi{/tex} both lie in quadrant IV, then sin {tex}(\theta+\phi){/tex} = ?

    a){tex}\frac{16}{65}{/tex}

    b){tex}\frac{-16}{65}{/tex}

    c){tex}\frac{-33}{65}{/tex}

    d){tex}\frac{-56}{65}{/tex}

    Boost Your Exam Preparation with myCBSEguide App and Examin8 App

    For comprehensive exam preparation, download the myCBSEguide app. It offers complete study material for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams. With practice questions, sample papers, and NCERT solutions, it’s the perfect tool to help you score well and excel in your exams. Regular practice with these sample papers can boost confidence and improve speed in solving problems. CBSE Sample Papers Class 11 Mathematics also help students understand the mark distribution and the types of questions likely to appear in the final exams.

    Teachers can also use the Examin8 app to easily create customized exam papers featuring their own name and logo, offering a personalized experience for their students. Whether you’re a student looking to prepare effectively or a teacher seeking to design tailored assessments, visit myCBSEguide and Examin8 are your go-to resources for academic success.

    |z₁ + z₂| = |z₁| + |z₂| is possible if

    a)z₂ = {tex}\overline{z_{1}}{/tex}

    b)arg (z₁) = arg (z₂)

    c)|z₁| = |z₂|

    d)z₂ = {tex}\frac{1}{z_{1}} {/tex}

18. A convex polygon of n sides has n diagonals. Then value of n is

    a)6

    b)8

    c)7

    d)5

19. Assertion (A): The expansion of (1 + x)ⁿ = {tex}n_{c_0}+n_{c_1}x + n_{c_2} x^2 \ldots+n_{c_n} x^n{/tex}.
    Reason (R): If x = -1, then the above expansion is zero.

    a)Both A and R are true and R is the correct explanation of A.

    b)Both A and R are true but R is not the correct explanation of A.

    c)A is true but R is false.

    d)A is false but R is true.

20. Assertion (A): The mean deviation about the mean for the data 4, 7, 8, 9, 10, 12, 13, 17 is 3.
    Reason (R): The mean deviation about the mean for the data 38, 70, 48, 40, 42, 55, 63, 46, 54, 44 is 8.5.

    a)Both A and R are true and R is the correct explanation of A.

    b)Both A and R are true but R is not the correct explanation of A.

    c)A is true but R is false.

    d)A is false but R is true.

21. Section B
22. Draw the graphs of the logarithmic functions log_a x, when 0 < a < 1.

    OR

    Let A = {1, 2}, B = {2, 3, 4}, C = {4, 5}. Find A {tex}\times{/tex} (B {tex}\cup{/tex} C)

23. Find the derivative of f (x) = tan x at x = 0
24. Find the equation of each of the following parabolas directrix x = 0, focus at (6, 0)

    OR

    lf y₁, y₂, y₃ be the ordinates of a vertices of the triangle inscribed in a parabola y² = 4ax, then show that the area of the triangle is {tex}\frac{1}{8 a}{/tex} | (y₁ – y₂) (y₂ – y₃) (y₃ – y₁) |.

25. Let A = {x : x {tex}\in{/tex} N, x is a multiple of 3} and B = {x : x {tex}\in{/tex} N and x is a multiple of 5}. Write A {tex}\cap{/tex} B.
26. If a, b, c are in G.P. write the area of the triangle formed by the line ax + by + c = 0 with the coordinates axes.
27. Section C
28. Given the relation R = {(1, 2), (2, 3)} on the set A = {1, 2, 3}, add a minimum number of ordered pairs so that the enlarged relation is symmetric, transitive and reflexive.
29. Solve the inequality {tex}\frac{(2 x-1)}{3} \geq \frac{(3 x-2)}{4}-\frac{(2-x)}{5}{/tex} for real x.
30. If the origin is the centroid of the triangle PQR with vertices P(2a, 2, 6), Q(-4, 3b, -10) and R(8, 14, 2c), then find the values of a, b and c.

    OR

    Find the equation of the curve formed by the set of all points which are equidistant from the points A (-1, 2, 3) and B(3, 2, 1).

31. Using g binomial theorem, expand {tex}\left\{\left(x+y\right)^5\;+\left(x-y\right)^5\right\}{/tex} and hence find the value of {tex}\left\{(\sqrt{2}+1)^{5}+(\sqrt{2}-1)^{5}\right\}{/tex}

    OR

    Simplify (x + 2y)⁸ + (x – 2y)⁸

32. Find the real values of x and y for which {tex}\left(\frac{x-1}{3+i}+\frac{y-1}{3-i}\right)=i{/tex}

    OR

    Solve the equation Izl + z = (2 + i) for complex value of z.

33. If A = {3, 6, 9, 12, 15, 18, 21}, B = {4, 8, 12,16, 20}, C = {2, 4, 6, 8, 10, 12, 14, 16}, D = {5, 10, 15, 20}, find: A – B?
34. Section D
35. A sample space consists of 9 elementary outcomes e₁, e₂, …, e₉ whose probabilities are
    P(e₁) = P(e₂) = .08, P(e₃) = P(e₄) = P(e₅) = 0.1
    P(e₆) = P(e₇) = .2, P(e₈) = P(e₉) = .07
    Suppose A = {e₁, e₅, e₈}, B = {e₂, e₅, e₈, e₉}
    1. Calculate P (A), P (B), and P (A {tex}\cap{/tex} B)
    2. Using the addition law of probability, calculate P (A {tex}\cup{/tex} B)
    3. List the composition of the event A ∪ B, and calculate P (A {tex}\cup{/tex} B) by adding the probabilities of the elementary outcomes.
    4. Calculate P ({tex}\bar B{/tex}) from P ({tex}\bar B{/tex}), also calculate P ({tex}\bar B{/tex}) directly from the elementary outcomes of {tex}\bar B{/tex}
36. The differentiation of sec x with respect to x is sec x tan x.

    OR

    Evaluate : {tex}\mathop {\lim }\limits_{x \to \sqrt {10} } \frac{{\sqrt {7 – 2x} – (\sqrt 5 – \sqrt 2 )}}{{{x^2} – 10}}{/tex}

37. The sum of first three terms of a G.P. is {tex}\frac { 13 } { 12 }{/tex} and their product is -1. Find the common ratio and the terms.
38. If 2 tan{tex}\alpha{/tex} = 3 tan {tex}\beta{/tex}, prove that tan ({tex}\alpha{/tex} – {tex}\beta{/tex}) = {tex}\frac{\sin 2 \beta}{5-\cos 2 \beta}{/tex}.

    OR

    Prove that {tex}\cos 2 x \cdot \cos \frac { x } { 2 } – \cos 3 x \cdot \cos \frac { 9 x } { 2 } = \sin 5 x \cdot \sin \frac { 5 x } { 2 }{/tex}

39. Section E
40. Read the following text carefully and answer the questions that follow:
    Indian track and field athlete Neeraj Chopra, who competes in the Javelin throw, won a gold medal at Tokyo Olympics. He is the first track and field athlete to win a gold medal for India at the Olympics.
    
    
    1. Name the shape of path followed by a javelin. If equation of such a curve is given by x² = -16y, then find the coordinates of foci. (1)
    2. Find the equation of directrix and length of latus rectum of parabola x² = -16y. (1)
    3. Find the equation of parabola with Vertex (0,0), passing through (5,2) and symmetric with respect to y-axis and also find equation of directrix. (2)
       OR
       Find the equation of the parabola with focus (2, 0) and directrix x = -2 and also length of latus rectum. (2)
41. Read the following text carefully and answer the questions that follow:
    Consider the data

    xi	4	8	11	17	20	24	32
    fi	3	5	9	5	4	3	1

    1. Find the standard deviation. (1)
    2. Find the variance. (1)
    3. Find the mean. (2)
       OR
       Write the formula of variance? (2)
42. Read the following text carefully and answer the questions that follow:
    Ashish is writing examination. He is reading question paper during reading time. He reads instructions carefully. While reading instructions, he observed that the question paper consists of 15 questions divided in to two parts – part I containing 8 questions and part II containing 7 questions.
    
    1. If Ashish is required to attempt 8 questions in all selecting at least 3 from each part, then in how many ways can he select these questions (1)
    2. If Ashish is required to attempt 8 questions in all selecting 3 from I part, then in how many ways can he select these questions (1)
    3. If Ashish is required to attempt 8 questions in all selecting 4 from part I and 4 from part II, then in how many ways can he select these questions (2)
       OR
       If Ashish is required to attempt 8 questions in all selecting 6 from one section and remaining from another section, then in how many ways can he select these questions (2)
       Download myCBSEguide App for Comprehensive Exam Preparation & PracticeTo boost your exam preparation and practice more questions, download the myCBSEguide app. It offers complete study material for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams, including sample papers, NCERT solutions, and chapter-wise tests. With these resources, students can easily prepare and excel in their exams, gaining confidence and mastering key concepts.

       In addition, teachers can leverage the Examin8 app to create customized exam papers with their own branding, including name and logo. This makes it easier to assess student performance and offer personalized learning experiences. Whether you are a student aiming for top scores or a teacher creating unique assessments, visit myCBSEguide Website and Examin8 Website are the perfect tools for success.

Class 11 – Mathematics
Sample Paper – 01 (2024-25)

---

Solution

1. Section A
2. (d)

   {tex}\frac {3} {4}{/tex}

   Explanation: We have:
   tan x {tex}=\frac{1}{\sqrt{7}}{/tex}
   {tex}\therefore \tan ^{2} x=\frac{1}{7}{/tex}
   Now, dividing the numerator and the denominator of {tex}\frac{\operatorname{cosec}^{2} x-\sec ^{2} x}{\operatorname{cosec}^{2} x+\sec ^{2} x}{/tex} by cosec² x:
   {tex}\frac{1-\tan ^{2} x}{1+\tan ^{2} x}{/tex}
   {tex}=\frac{1-\frac{1}{7}}{1+\frac{1}{7}}{/tex}
   {tex}=\frac{6}{8}=\frac{3}{4}{/tex}

3. (c)

   {tex}\left[\frac{-1}{2}, \frac{1}{2}\right]{/tex}

   Explanation: f(x) = cos^-1 2x
   The domain for function cos^-1 x is [-1,1] and range is [0, {tex}\pi{/tex}] When a function is multiplied by an integer, the domain of the function is decreased by the same number.
   So, domain of cos^-1 x is [-1, 1] Multiply function by 2
   {tex}\Rightarrow{/tex} domain of cos^-1 2x is {tex}\left[\frac{-1}{2}, \frac{1}{2}\right]{/tex}

4. (d)

   {tex}\frac{1}{16}{/tex}

   Explanation: Probability of getting head in a single toss, P(H) = {tex}\frac{1}{2}{/tex}
   Probability of getting tail in a single toss, P(T) = {tex}\frac{1}{2}{/tex}
   {tex}\therefore{/tex} Required probability
   = P(HHHHT or TTTTH)
   = P(HHHHT) + P(TTTTH)
   = P(H) {tex}\cdot{/tex} P(H) {tex}\cdot{/tex} P(H) {tex}\cdot{/tex} P(H) {tex}\cdot{/tex} P(T) + P(T) {tex}\cdot{/tex} P(T) {tex}\cdot{/tex} P(T) {tex}\cdot{/tex} P(T) {tex}\cdot{/tex} P(H)
   = {tex}\left(\frac{1}{2}\right)\left(\frac{1}{2}\right)\left(\frac{1}{2}\right)\left(\frac{1}{2}\right)\left(\frac{1}{2}\right)+\left(\frac{1}{2}\right)\left(\frac{1}{2}\right)\left(\frac{1}{2}\right)\left(\frac{1}{2}\right)\left(\frac{1}{2}\right){/tex}
   = 2 {tex}\times \frac{1}{32}=\frac{1}{16}{/tex}

5. (b)

   4

   Explanation: Substitute x = cos {tex}\theta{/tex}
   {tex}\Rightarrow{/tex} {tex}\frac{d(2 \theta)}{d(\sin \theta)}=\frac{\frac{d(2 \theta)}{d t}}{\frac{d(\sin \theta)}{d t}}{/tex}
   {tex}\Rightarrow{/tex} 2 sec {tex}\theta{/tex}
   {tex}\Rightarrow{/tex} 2(2) = 4

6. (c)

   parallel to Y axis

   Explanation: Vertical lines have undefined slopes. Hence a line which is parallel to Y-axis has undefined slopes.

7. (d)

   {2, 3, 4, 5, 8, 10, 12}

   Explanation: Given A = {2, 3, 4, 8, 10}, B = {3, 4, 5, 10, 12} and C = {4, 5, 6, 12, 14}
   Here A {tex}\cup{/tex} B = {2, 3, 4, 5, 8, 10, 12} and A {tex}\cup{/tex} C = {2, 3, 4, 5, 6, 8, 10, 12, 14}
   Now (A {tex}\cup{/tex} B) {tex}\cap{/tex} (A {tex}\cup{/tex} C) = {2, 3, 4, 5, 8, 10, 12}

8. (b)

   {tex}\frac{1}{2}{/tex} (1 + i)

   Explanation: {tex}\frac{1}{2}{/tex} (1 + i)
   {tex}\frac {i^{5}+i^{6}+i^{2}+i^{8}+i^{9}} {1 + i}{/tex}
   {tex}=\frac{i-1-i+1+i}{1+i}{/tex} [As, i⁵ = i, i⁶ = -1, i⁷ = -i, i⁸ = 1, i⁹ = i
   {tex}=\frac {i} {i + 1}{/tex}
   {tex}=\frac{i}{i+1} \times \frac{i-1}{i-1}{/tex}
   {tex}=\frac{i(i-1)}{i^{2}-1}{/tex}
   {tex}=\frac{i^{2}-i}{-2}{/tex}
   {tex}=\frac{1}{2}{/tex} (1 + i)

9. (b)

   transitive but not symmetric

   Explanation: Consider the non – empty set consisting of children in a family and a relation R defined as aRb if a is brother of b. Then R is not symmetric, because aRb means a is brother of b, then, it is not necessary that b is also brother of a , it can be the sister of a. Therefore, bRa is not true. Therefore, the relation is not symmetric . Again, if aRb and bRc is true, then aRc is also true. Therefore, R is transitive only.

10. (b)

    {tex}x \in(-\infty,-a) \cup(a, \infty){/tex}

    Explanation: |x| > a
    {tex}\Rightarrow{/tex} x < -a or x > a
    {tex}\Rightarrow{/tex}x {tex}\in{/tex} ({tex}-\infty{/tex}, -a)  {tex}\cup{/tex} (a, {tex}\infty{/tex})

11. (b)

    cos 2A

    Explanation: cos(36^o – A) cos(36^o + A) + cos(54^o + A) cos(54^o – A)
    = cos(36^o – A) cos(36^o + A) + sin[90^o – (54^o + A)] sin [90^o – (54^o – A)] [Since {tex}\sin \left(90^{\circ}-\theta\right)=\cos \theta{/tex}] = cos(36^o – A) cos(36^o + A) + sin(36^o – A) sin(36^o + A)
    = cos(36^o + A – 36^o + A) [Using cos(A – B) formula] = cos 2A

12. (a)

    A

    Explanation: Since, {tex}A \subseteq A \cup B{/tex}, therefore, {tex}A \cap(A \cup B){/tex} = A

13. (a)

    3

    Explanation: Here, we have a = {tex}1 \over 3{/tex} and let r be the common ratio.
    Then, {tex}x_{1}=a r=\frac{1}{3} r{/tex}, {tex}, x_{2}=a r^{2}=\frac{1}{3} r^{2} {/tex} and {tex}9=a r^{3}=\frac{1}{3} r^{3}{/tex}
    {tex}\therefore \quad r^{3}=27=3^{3} \Rightarrow r=3{/tex}
    Therefore, {tex}x_{2}=\frac{1}{3} \times 3^{2}=\frac{1}{3} \times 9=3{/tex}.

14. (c)

    240

    Explanation: {tex}\sqrt{5}\left\{(\sqrt{5}+1)^{4}-(\sqrt{5}-1)^{4}\right\}{/tex}
    Let a = {tex}\sqrt{5}{/tex} and b = 1
    Then {tex}(\sqrt{5}+1)^{4}-(\sqrt{5}-1)^{4}{/tex} = (a + 4)⁴ – (a – b)⁴ 
    = {tex}\left[\begin{array}{llll} ^{4} C_{0} a^{4}+^{4} C_{1} a^{3} b+^{4} C_{2} a^{2} b^{2} \end{array}^{2}\right.{/tex} + {tex}\left.^{4} C_{3} \quad a^{1} b^{3}+^{4} C_{4} \quad b^{4}\right]{/tex} – {tex}\left[\begin{array}{llll} ^{4} C_{0} a^{4}-^{4} C_{1} a^{3} b+^{4} C_{2} a^{2} b^{2} \end{array}^{2}\right.{/tex} – {tex}\left.^{-4} C_{3} a^{1} b^{3}+^{4} C_{4} b^{4}\right]{/tex}
    = {tex}2\left[^{4} C_{1}a^{3} b+^{4} C_{3} a^{1} b^{3}\right]{/tex}
    = 2[4a³b + 4a¹b³] = 8ab[a² + b²] {tex}\therefore{/tex} {tex}(\sqrt{5}+1)^{4}-(\sqrt{5}-1)^{4}{/tex} = {tex}8 \cdot \sqrt{5} \cdot 1\left[(\sqrt{5})^{2}+1^{2}\right]{/tex} = {tex}48 \sqrt{5}{/tex}
    Hence {tex}\sqrt{5}\left\{(\sqrt{5}+1)^{4}-(\sqrt{5}-1)^{4}\right\}{/tex} = {tex}\sqrt{5} .48 \sqrt{5}{/tex} = 240

15. (c)

    ac {tex} \geq{/tex} bc

    Explanation: The sign of the inequality is to be reversed ({tex}\le{/tex} to {tex}\ge{/tex} or {tex}\ge{/tex} to {tex}\le{/tex}) if both sides of an inequality are multiplied by the same negative real number.

16. (a)

    C = {tex}\phi {/tex}

    Explanation: {tex}\phi {/tex} is denoted as null set.

17. (d)

    {tex}\frac{-56}{65}{/tex}

    Explanation: In quadrant IV, {tex}\cos \theta>0, \sin \theta<0{/tex}, {tex}\cos \phi>0{/tex} and {tex}\sin \phi<0{/tex}
    Now, {tex}\cos \theta=\frac{4}{5} \Rightarrow \sin ^{2} \theta{/tex}{tex}=\left(1-\cos ^{2} \theta\right){/tex}{tex}=\left(1-\frac{16}{25}\right)=\frac{9}{25}{/tex}
    {tex}\Rightarrow \sin \theta=-\sqrt{\frac{9}{25}}=\frac{-3}{5}{/tex}
    {tex}\cos \phi=\frac{12}{13} \Rightarrow \sin ^{2} \theta{/tex}{tex}=\left(1-\cos ^{2} \phi\right){/tex}{tex}=\left(1-\frac{144}{169}\right)=\frac{25}{169}{/tex}
    {tex}\Rightarrow \sin \phi=-\sqrt{\frac{25}{169}}=\frac{-5}{13}{/tex}
    {tex}\therefore \sin (\theta+\phi)=\sin \theta{/tex}{tex} \cos \phi+\cos\theta \sin \phi{/tex}
    {tex}=\left(\frac{-3}{5} \times \frac{12}{13}\right)+\left(\frac{-5}{13} \times \frac{4}{5}\right){/tex}{tex}=\left(\frac{-36}{65}-\frac{20}{65}\right)=\frac{-56}{65}{/tex}

    Enhance Your Exam Preparation with myCBSEguide and Examin8 Apps

    To boost your exam preparation, download the myCBSEguide.com app, your one-stop solution for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams. The app offers complete study material, including practice questions, sample papers, NCERT solutions, and chapter-wise test papers, all designed to help you achieve top scores. By regularly practicing CBSE Sample Papers Class 11 Mathematics, students can build confidence and improve their time-management skills.

    For teachers, the Examin8.com app provides a powerful tool to create customized exam papers with their own name and logo, making it easy to assess and track students’ progress. Whether you’re a student aiming for high marks or a teacher designing unique assessments, both myCBSEguide Website and Examin8 Website offer the resources you need for success. The CBSE Sample Papers Class 11 Mathematics align with the latest syllabus and marking scheme, making them the perfect tool for exam preparation.

    (b)

18. arg (z₁) = arg (z₂)

    Explanation: Let z₁ = r₁ (cos {tex}\theta{/tex}₁ + i sin {tex}\theta{/tex}₁) and z₂ = r₂ (cos {tex}\theta{/tex}₂ + i sin {tex}\theta{/tex}₂)
    Since |z₁ + z₂| = |z₁| + |z₂|
    {tex}\Rightarrow{/tex} z₁ + z₂ = r₁ cos {tex}\theta{/tex}₁ + ir₁ sin {tex}\theta{/tex}₁+ r₂ cos {tex}\theta{/tex}₂ + ir₂ sin {tex}\theta{/tex}₂
    {tex}\Rightarrow{/tex} |z₁ + z₂|
    = {tex}\sqrt{\mathrm{r}_{1}^{2} \cos ^{2} \theta_{1}+\mathrm{r}_{2}^{2} \cos ^{2} \theta_{2}+2 \mathrm{r}_{1} \mathrm{r}_{2} \cos \theta_{1} \cos \theta_{2}+\mathrm{r}_{1}^{2} \sin ^{2} \theta_{1}+\mathrm{r}_{2}^{2} \sin ^{2} \theta_{2}+2 \mathrm{r}_{1} \mathrm{r}_{2} \sin \theta_{1} \sin \theta_{2}}{/tex}
    = {tex}\sqrt{\mathrm{r}_{1}^{2}+\mathrm{r}_{2}^{2}+2 \mathrm{r}_{1} \mathrm{r}_{2} \cos \left(\theta_{1}-\theta_{2}\right)}{/tex}
    But |z₁ + z₂| = |z₁| + |z₂|
    {tex}\Rightarrow{/tex} {tex}\sqrt{\mathrm{r}_{1}^{2}+\mathrm{r}_{2}^{2}+2 \mathrm{r}_{1} \mathrm{r}_{2} \cos \left(\theta_{1}-\theta_{2}\right)}=\mathrm{r}_{1}+\mathrm{r}_{2}{/tex}
    Squaring both sides,
    {tex}\Rightarrow{/tex} {tex}r_{1}^{2}+r_{2}^{2}+2 r_{1} r_{2} \cos \left(\theta_{1}-\theta_{2}\right)=r_{1}^{2}+r_{2}^{2}+2 r_{1} r_{2}{/tex}
    {tex}\Rightarrow{/tex} 2r₁r₂ – 2r₁r₂ cos ({tex}\theta{/tex}₁ – {tex}\theta{/tex}₂) = 0
    {tex}\Rightarrow{/tex} 1 – cos ({tex}\theta{/tex}₁ – {tex}\theta{/tex}₂) = 0
    {tex}\Rightarrow{/tex} cos ({tex}\theta{/tex}₁ – {tex}\theta{/tex}₂) = 1
    {tex}\Rightarrow{/tex} ({tex}\theta{/tex}₁ – {tex}\theta{/tex}₂) = 0
    {tex}\Rightarrow{/tex} {tex}\theta{/tex}₁ = {tex}\theta{/tex}₂
    {tex}\therefore{/tex} arg (z₁) = arg (z₂)

19. (d)

    5

    Explanation: If you join every distinct pair of vertices you will get {tex}^{ n }{ C_{ 2\quad } }{/tex} lines.
    These {tex}^{ n }{ C_{ 2\quad } }{/tex} lines account for the n sides of the polygon as well as for the diagonals.

    So the number of diagonals is given by {tex}^{n} C_{2}-n=\frac{n(n-1)}{2}-n=\frac{n(n-3)}{2}{/tex}

    But given number of  sides = number of diagonals =n
    {tex}\Rightarrow n=\frac{n(n-3)}{2}{/tex}
    {tex}\Rightarrow 2 n=n(n-3){/tex}
    {tex}\Rightarrow n-3=2{/tex}
    {tex}\Rightarrow n=5{/tex}

20. (b)

    Both A and R are true but R is not the correct explanation of A.

    Explanation: Assertion:
    (1 + x)ⁿ = {tex}n_{c_0}+n_{c_1}x + n_{c_2} x^2 \ldots+n_{c_n} x^n{/tex}
    Reason:
    (1 + (-1))ⁿ = {tex}n_{c_0}{ 1}^n+n_{c_1}(1)^{n-1}(-1)^1+n_{c_2}(1)^{n-2}(-1)^2{/tex} +… + {tex}{ }^n c_n(1)^{n-n}(-1)^n{/tex}
    = {tex}n_{c_8}-n_{c_1}+n_{c_2}-n_{c_3}{/tex} + … (-1)ⁿ{tex}n_{c_n}{/tex}
    Each term will cancel each other
    {tex}\therefore{/tex} (1 + (-1))ⁿ = 0
    Reason is also the but not the correct explanation of Assertion.

21. (c)

    A is true but R is false.

    Explanation: Assertion Mean of the given series
    {tex}\bar{x} =\frac{\text { Sum of terms }}{\text { Number of terms }}=\frac{\sum x_i}{n}{/tex}
    {tex}=\frac{4+7+8+9+10+12+13+17}{8}{/tex} = 10

    xi	|xi – {tex}\bar x{/tex}|
    4	|4 – 10| = 6
    7	|7 – 10| = 3
    8	|8 – 10| = 2
    9	|9 – 10| = 1
    10	|10 – 10| = 0
    12	|12 – 10| = 2
    13	|13 – 10| = 3
    17	|17 – 10| = 7
    {tex}\sum x_i{/tex} = 80	{tex}\sum \left|x_i-\bar{x}\right|{/tex} = 24

    {tex}\therefore{/tex} Mean deviation about mean
    {tex}=\frac{\Sigma\left|x_i-\bar{x}\right|}{n}=\frac{24}{8}{/tex} = 3
    Reason Mean of the given series
    {tex}\bar{x}= \frac{\text { Sum of terms }}{\text { Number of terms }}=\frac{\sum x_i}{n}{/tex}
    {tex}= \frac{38+70+48+40+42+55}{+63+46+54+44}{/tex} = 50
    {tex}\therefore{/tex} Mean deviation about mean
    {tex}= \frac{\Sigma\left|x_i-\bar{x}\right|}{n}{/tex}
    {tex}=\frac{84}{10}{/tex} = 8.4
    Hence, Assertion is true and Reason is false.

22. Section B
23. 
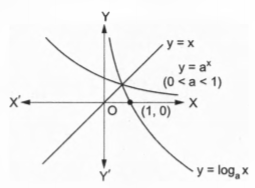
    We know that when 0 < a < 1, the function a^x is strictly decreasing, i.e., different values of x give different values of a^x. So, the function is one-one. Also, its range is R. So, it is onto.
    Thus, the function a^x is invertible. Its inverse is the log function reflected by the line y = x, as shown. The graph clearly passes through (1, 0).
    Required graph of the function shown in the above fig.

    OR

    Given, A = {1, 2}, B = {2, 3, 4} and C = {4, 5}
    B {tex}\cup{/tex} C = {2, 3, 4} {tex}\cup{/tex} {4, 5}
    = {2, 3, 4, 5}
    {tex}\therefore{/tex} A {tex}\times{/tex} (B {tex}\cup{/tex} C) = {1, 2} {tex}\times{/tex} {2, 3, 4, 5}
    = {(1, 2), (1, 3), (1, 4), (1, 5), (2, 2), (2, 3), (2, 4), (2, 5)}

24. Derivative of a function f(x) at any real number a is given by f'(a)= {tex}\lim \limits_{h \rightarrow 0}{/tex}{tex}\frac{f(a+h)-f(a)}{h}{/tex}  {where h is a very small positive number}
    {tex}\therefore{/tex}derivative of cos x at x = 0 is given as
    f'(0) = {tex}\lim \limits_{h \rightarrow 0}{/tex}{tex}\frac{\mathrm{f}(0+\mathrm{h})-\mathrm{f}(0)}{\mathrm{h}}{/tex}
    {tex}\Rightarrow{/tex} f’ (0) = {tex}\lim \limits_{h \rightarrow 0}{/tex}{tex}\frac{\tan (\mathrm{h})-\tan 0}{\mathrm{h}}{/tex}
    {tex}\Rightarrow{/tex} f’ (0) = {tex}\lim \limits_{h \rightarrow 0}{/tex}{tex}\frac{\tanh }{\mathrm{h}}{/tex}
    {tex}\therefore{/tex} we can’t find the limit by direct substitution as it gives {tex}\frac 00{/tex} (indeterminate form)
    Use the formula: {tex}\lim \limits_{x \rightarrow 0}{/tex}{tex}\frac{\tan x }{\mathrm{x}}{/tex} = 1 {sandwich theorem}
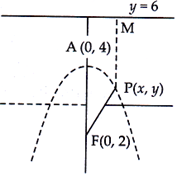
    {tex}\therefore{/tex} f’(0) = 1
25. Given that directrix = 0 and focus (6, 0)
    {tex}\therefore{/tex} by definition,
    The equation of the parabola is
    MP² = PF²
    (x – 6)² + y² = x²
    {tex}\Rightarrow{/tex} x² + 36 – 12x + y² = x²
    {tex}\Rightarrow{/tex}y² – 12x + 36 = 0
    Hence, the required equations is y² – 12x + 36 = 0
    

    OR

    Let A (x₁, y₁), B (x₂, y₂) and C (x₃, y₃) vertices of triangle ABC.
    Since (x₁, y₁), (x₂, y₂) and (x₃, y₃) lie on the parabola.
    Hence, {tex}y_{1}^{2}=4 a x_{1}, y_{2}^{2}=4 a x_{2}{/tex}  and {tex}y_{3}^{2}=4 a x_{3}{/tex}
    {tex}\Rightarrow{/tex} {tex}x_{1}=\frac{y_{1}^{2}}{4 a}{/tex}, {tex}x_{2}=\frac{y_{2}^{2}}{4 a}{/tex} and {tex}x_{3}=\frac{y_{3}^{2}}{4 a}{/tex}
    {tex}\therefore{/tex} Area of {tex}\Delta{/tex}ABC = {tex}\frac{1}{2}{/tex} [x₁ (y₂ – y₃) + x₂ (y₃ – y₁) + x₃ (y₁ – y₂)] = {tex}\frac{1}{2}{/tex} {tex}\left[\frac{y_{1}^{2}}{4 a}\left(y_{2}-y_{3}\right)\right.{/tex} + {tex}\frac{y_{2}^{2}}{4 a}\left(y_{3}-y_{1}\right){/tex} + {tex}\left.\frac{y_{3}^{2}}{4 a}\left(y_{1}-y_{2}\right)\right]{/tex}
    = {tex}\frac{1}{8 a}{/tex} {tex}\left[y_{1}^{2}\left(y_{2}-y_{3}\right)\right.{/tex} + {tex}\left(y_{2}^{2} y_{3}-y_{2} y_{3}^{2}\right){/tex} – {tex}\left.y_{1}\left(y_{2}^{2}-y_{3}^{2}\right)\right]{/tex}
    = {tex}\frac{1}{8 a}{/tex} {tex}\left[y_{1}^{2}\left(y_{2}-y_{3}\right)+y_{2} y_{3}\right.{/tex} {tex}\left.\left(y_{2}-y_{3}\right)-y_{1}\left(y_{2}^{2}-y_{3}^{2}\right)\right]{/tex}
    = {tex}\frac{1}{8 a}{/tex} (y₂ – y₃){tex}\left[y_{1}^{2}+y_{2} y_{3}-y_{1}\left(y_{2}+y_{3}\right)\right]{/tex}
    = {tex}\frac{1}{8 a}{/tex} (y₂ – y₃) {tex}\left[\left(y_{1}^{2}-y_{1} y_{2}\right)+\left(y_{2} y_{3}-y_{1} y_{3}\right)\right]{/tex}
    = {tex}\frac{1}{8 a}{/tex} (y₂ – y₃) [y₁ (y₁ – y₂) – y₃ (y₁ – y₂)] = {tex}\frac{1}{8 a}{/tex} (y₂ – y₃) (y₁ – y₂) (y₁ – y₃)
    = – {tex}\frac{1}{8 a}{/tex} (y₁ – y₂) (y₂ – y₃) (y₃ – y₁)
    Therefore, Area of {tex}\Delta{/tex}ABC = {tex}\frac{1}{8 a}{/tex} | (y₁ – y₂) (y₂ – y₃) (y₃ – y₁) |

26. According to the question, we can write,
    A = {x : x {tex}\in{/tex} N, x is a multiple of 3}
    = {3, 6, 9, 12, 15, 18, 21, 24, 27, 30, 33, 36, 39, 42, 45, …}
    B = {x : x {tex}\in{/tex} N and x is a multiple of 5}
    = {5, 10, 15, 20, 25, 30, 35, 40, 45,…}
    Thus, we have
    A {tex}\cap{/tex} B = {15, 30, 45, …}
    = {x : x {tex}\in{/tex} N, where x is a multiple of 15}
27. The point of intersection of the line ax + by + c = 0 with the coordinate axis are {tex}\left(-\frac{c}{a}, 0\right){/tex} and {tex}\left(0,-\frac{c}{b}\right){/tex}.
    Therefore, the vertices of the triangle are  {tex}\left(-\frac{c}{a}, 0\right){/tex} and {tex}\left(0,-\frac{c}{b}\right){/tex}.
    Suppose A be the area of the required triangle.
    {tex}A=\frac{1}{2}\left|\begin{array}{ccc} 0 & 0 & 1 \\ \frac{-c}{a} & 0 & 1 \\ 0 & \frac{-c}{b} & 1 \end{array}\right|{/tex}
    {tex}A=\frac{1}{2}\left|-\frac{c}{a} \times \frac{-c}{b}\right|{/tex} {tex}=\frac{1}{2}\left|\frac{c^{2}}{a b}\right|{/tex}
    It is given that a, b and c are in GP.
    {tex}\therefore {/tex} b² = ac
    {tex}\Rightarrow A=\frac{1}{2}\left|\frac{b^{4}}{a^{2} \times a b}\right|{/tex} {tex}=\frac{1}{2}\left|\frac{b}{a}\right|^{3}{/tex} square units.
28. Section C
29. We have,
    A = {1, 2, 3} and R {(1, 2) (2, 3)}
    Now,
    To make R reflexive, we will add (1, 1) (2, 2) and (3, 3) to get
    {tex}\therefore{/tex} R1 = {(1, 2), (2, 3), (1, 1), (2, 2), (3, 3)} is reflexive
    Again to make R’ symmetric we shall add (3, 2) and (2, 1)
    {tex}\therefore{/tex} R” = {(1, 2), (2, 3), (1, 1), (2, 2), (3, 3), (3, 2), (2, 1)} is reflexive and symmetric
    Now,
    To R” transitive we shall add (1, 3) and (3, 1)
    {tex}\therefore{/tex} R”‘ = {(1, 2), (2, 3), (1, 1), (2, 2), (3, 3), (3, 2), (2, 1)} is reflexive and symmetric
    Now,
    To make R” transitive we shall add (1, 3) and (3, 1)
    {tex}\therefore{/tex} R”‘ {(1, 2), (2, 3), (1, 1) ,(2, 2), (3, 3), (3, 2), (2, 1), (1, 3) ,(3, 1)}
    {tex}\therefore{/tex} R”‘ is reflexive, symetric and transitive.
30. Here {tex}\frac{{(2x – 1)}}{3} \geqslant \frac{{(3x – 2)}}{4} – \frac{{(2 – x)}}{5}{/tex}
    {tex} \Rightarrow \frac{{2x}}{3} – \frac{1}{3} \geqslant \frac{{3x}}{4} – \frac{2}{4} – \frac{2}{5} + \frac{x}{5}{/tex}
    {tex} \Rightarrow \frac{{2x}}{3} – \frac{3x}{4}-\frac{x}{5}\geqslant \ \frac{-2}{4} – \frac{2}{5} + \frac{1}{3}{/tex}
    {tex} \Rightarrow \frac{{40x – 45x – 12x}}{{60}} \geqslant \frac{{ – 30 – 24 + 20}}{{60}}{/tex}
    {tex} \Rightarrow \frac{{ – 17x}}{{60}} \geqslant \frac{{ – 34}}{{60}}{/tex}
    Multiplying both sides by 60, we have
    {tex} – 17x \geqslant – 34{/tex}
    Dividing both sides by -17, we have
    {tex}\frac{{ – 17x}}{{ – 17}} \leqslant \frac{{ – 34}}{{ – 17}}{/tex}
    {tex} \Rightarrow x \leqslant 2{/tex}
    Thus the solution set is {tex}\left( { – \infty ,2} \right]{/tex}
31. Here P(2a, 2, 6), {tex}Q( – 4,3b, – 10){/tex} and R(8, 14, 2c) are vertices of triangle PQR.
    {tex}\therefore{/tex} Coordinates of centriod of {tex}\Delta PQR{/tex} is {tex}\left( {\frac{{2a – 4 + 8}}{3},\frac{{2 + 3b + 14}}{3},\frac{{6 – 10 + 2c}}{3}} \right){/tex}
    {tex} = \left( {\frac{{2a +4}}{3},\frac{{3b + 16}}{3},\frac{{2c – 4}}{3}} \right){/tex}
    But is it given that coordinates of centroid is (0, 0, 0)
    {tex}\frac{{2a + 4}}{3} = 0 \Rightarrow{/tex} 2a + 4 = 0 {tex}\therefore{/tex} a = -2
    {tex}\frac{{3b + 16}}{3} = 0 \Rightarrow{/tex} 3b + 16 = 0 {tex}\Rightarrow b = \frac{{ – 16}}{3}{/tex}
    {tex}\frac{{2c – 4}}{3} = 0 \Rightarrow{/tex} 2c – 4 = 0 {tex}\Rightarrow{/tex} c = 2

    OR

    Consider, C(x, y, z) point equidistant from points A(-1, 2, 3) and B(3, 2, 1).
    {tex}\therefore{/tex} AC = BC
    {tex}\sqrt{(x+1)^{2}+(y-2)^{2}+(z-3)^{2}}=\sqrt{(x-3)^{2}+(y-2)^{2}+(z-1)^{2}}{/tex}
    Squaring both sides,
    {tex}\Rightarrow{/tex} (x + 1)² + (y – 2)² + (z – 3)²  = (x – 3)² + (y – 2)² + (z – 1)²
    {tex}\Rightarrow{/tex} x² +2x + 1 + y² – 4y + 4 + z² – 6z + 9 = x² – 6x + 9 + y² – 4y + 4 + z² -2z +1
    {tex}\Rightarrow{/tex} 8x – 4z = 0
    {tex}\Rightarrow{/tex} 2x – z = 0
    {tex}\Rightarrow{/tex} z = 2x
    
    Equation of curve is z = 2x

32. {tex}\style{font-family:Tahoma}{\style{font-size:8px}{\begin{array}{l}We\;have\\\left(x+y\right)^5\;+\left(x-y\right)^5\;=\;2\;\left[{}^5C_0\;x^5\;+^5C_2\;x^3y^2\;+^5C_4\;x^1y^4\right]\;\\\;\;\;\;\;\;\;\;=\;2\;(x^5+10x^3y^2\;+5xy^4\\Putting\;\;x\;=\;\sqrt2\;and\;y\;=\;1,\;we\;get\\(\sqrt2+1)^5+{(\sqrt2-1{)^5\;=\;2\left[\left(\sqrt2\right)^5\;+10\left(\sqrt2\right)^3\;+5\sqrt2\right]}}\\\;\;\;\;\;=\;2\left[4\;\sqrt2\;+20\;\sqrt2+\;5\sqrt2\right]\\\;\;\;=\;58\sqrt2\\\\\\\\\end{array}}}{/tex}

    OR

    (x + 2y)⁸ + (x – 2y)⁸ {tex} = 2{[^8}{C_0}{x^8}{ + ^8}{C_2}{x^5}{(2y)^2}{ + ^8}{C_4}{x^4}{(2y)^4}{/tex}{tex}{ + ^8}{C_6}{x^2}{(2y)^6}{ + ^8}{C_8}{(2y)^8}]{/tex}
    {tex}\style{font-family:Tahoma}{\style{font-size:8px}{\begin{array}{l}\because{(x+a)^n}+{(x-a)^n}=2\lbrack^nC_0x^n+^nC_2x^{n-2}a^2+^nC_4x^{n-4}a^4+……….\rbrack\\\\\\\\\end{array}}}{/tex}
    {tex} \Rightarrow {(x + 2y)^8} + {(x – 2y)^8}{/tex}{tex} = 2[{x^8} + 28{x^6} \times 4{y^2} + 70{x^4} \times 16{y^4}{/tex}{tex} + 28{x^2} \times 64{y^6} + 256{y^8}]{/tex}
    {tex} = 2[{x^8} + 112{x^6}{y^2} + 1120{x^4}{y^4}{/tex}{tex} + 1792{x^2}{y^6} + 256{y^8}]{/tex}

33. We have
    {tex}\frac{x-1}{3+i}+\frac{y-1}{3-i}=i{/tex}
    {tex}\Rightarrow \frac{(x-1)}{(3+i)} \times \frac{(3-i)}{(3-i)}+\frac{(y-1)}{(3-i)} \times \frac{(3+i)}{(3+i)}=i{/tex}
    {tex}\Rightarrow \frac{(x-1)(3-i)}{\left(9-i^{2}\right)}+\frac{(y-1)(3+i)}{\left(9-i^{2}\right)}=i{/tex}
    {tex}\Rightarrow \frac{3(x-1)-i(x-1)}{10}+\frac{3(y-1)+i(y-1)}{10}=i{/tex}
    {tex}\Rightarrow{/tex} 3(x – 1) – i(x – 1) + 3(y – 1) + i(y – 1) = 10i
    {tex}\Rightarrow{/tex} (3x – 3 + 3y – 3) + i(y – 1 – x + 1) = 10i
    {tex}\Rightarrow{/tex} (3x + 3y – 6) + i(y – x) = 10i
    {tex}\Rightarrow{/tex} 3(x + y – 2 ) = 0 and y – x = 10 [equating real parts and the imaginary parts separately] {tex}\Rightarrow{/tex} x + y – 2 = 0 and y – x = 10
    {tex}\Rightarrow{/tex} x + y = 2 an d -x + y = 10
    {tex}\Rightarrow{/tex} x = – 4 and y = 6 [by solving x + y = 2 and – x + y = 10].
    Hence, the Real value of x and y  is -4 and 6

    OR

    Let z = (x + iy). Then,
    lzl + z = 2 + i
    {tex}\Rightarrow{/tex} lx + iy| + (x + iy) = 2 + i
    {tex}\Rightarrow \{\sqrt{x^{2}+y^{2}}{/tex} + x} + iy = (2 + i)
    {tex}\Rightarrow \sqrt{x^{2}+y^{2}}+x = 2{/tex} and y = 1 [equating real parts and imaginary parts separately] {tex}\Rightarrow{/tex} y = 1 and {tex}\sqrt{x^{2}+1^{2}}+x=2 \Rightarrow y=1{/tex} and {tex}\sqrt{x^{2}+1}=(2-x){/tex}
    {tex}\Rightarrow{/tex} y = and x² + 1 = (2 – x)² {tex}\Rightarrow{/tex} y = 1 and x² + 1 = x² – 4x + 4
    {tex}\Rightarrow{/tex} 4x = 3 and y = 1 {tex}\Rightarrow x=\frac{3}{4}{/tex} and y = 1.
    Hence, {tex}z=\left(\frac{3}{4}+i\right){/tex} is the desired solution.

34. Here A = {3, 6,9, 12, 15, 18, 21}, B = {4, 8, 12, 16, 20}, C = {2, 4, 6, 8, 10, 12, 14, 16}, D = {5, 10, 15, 20}A – B = {3,6,9,12,15,18,21} – {4,8,12,16,20}
    = { 3, 6, 9, 15, 18, 21}
35. Section D
36. Given that:Sample Space S = {e₁, e₂, e₃, e₄, e_5, e₆, e₇, e₈, e₉}
    A = {e₁, e₅, e₈} and B = {e₂, e₅, e₈, e₉}
    P(e₁) = P(e₂) = .08, P(e₃) = P(e₄) = P(e₅) = .1
    P(e₆) = P(e₇) = .2, P(e₈) = P(e₉) = .07
    1. To find: P(A), P(B) and P(A {tex}\cap{/tex} B)
       1. A = {e₁, e₅, e₈}
          On adding the probabilities of elements of A, we get
          P(A) = P(e₁) + P(e₅) + P(e₈)
          {tex}\Rightarrow{/tex} P(A) = 0.08 + 0.1 + 0.07 [given] {tex}\Rightarrow{/tex} P(A) = 0.25
       2. B = {e₂, e₅, e₈, e₉}
          Similarly, on adding the probabilities of elements of B, we get
          P(B) = P(e₂) + P(e₅) + P(e₈) + P(e₉)
          {tex}\Rightarrow{/tex} P(B) = 0.08 + 0.1 + 0.07 + 0.07 [given] {tex}\Rightarrow{/tex} P(B) = 0.32
       3. Now, we have to find P(A {tex}\cap{/tex} B)
          A = {e₁, e₅, e₈} and B = {e₂, e₅, e₈, e₉}
          {tex}\therefore{/tex} A ⋂ B = {e₅, e₈}
          On adding the probabilities of elements of A ⋂ B, we get
          P(A ⋂ B) = P(e₅) + P(e₈)
          = 0.1 + 0.07 = 0.17
    2. To find: P(A {tex}\cup{/tex} B)
       1. By General Addition Rule: P(A {tex}\cup{/tex} B) = P(A) + P(B) – P(A {tex}\cap{/tex} B)
          from part (a), we have
          P(A) = 0.25, P(B) = 0.32 and P(A {tex}\cap{/tex} B) = 0.17
          Putting the values, we get
          P(A {tex}\cup{/tex} B) = 0.25 + 0.32 – 0.17 = 0.40
    3. A = {e₁, e₅, e₈} and B = {e₂, e₅, e₈, e₉}
       {tex}\therefore{/tex} A ⋃ B = {e₁, e₂, e₅, e₈, e₉}
       Again, on adding the probabilities of elements of A ⋃ B, we get
       P(A ⋃ B) = P(e₁) + P(e₂) + P(e₅) + P(e₈) + P(e₉)
       = 0.08 +0.08 + 0.1 + 0.07 + 0.07 = 0.40
    4. To find: P({tex}\bar B{/tex})
       1. By Complement Rule, we have
          P ({tex}\bar B{/tex}) = 1 – P({tex}\ B{/tex})
          {tex}\Rightarrow{/tex} P ({tex}\bar B{/tex}) = 1 – 0.32 = 0.68
       2. Given: B = {e₂, e₅, e₈, e₉}
          {tex}\therefore{/tex} {tex}\bar B{/tex} = {e₁, e₃, e₄, e₆, e₇}
          P({tex}\bar B{/tex}) = P(e₁) + P(e₃) + P(e₄) + P(e₄) + P(e₆) + P(e₁₇)
          = 0.08 + 0.1 + 0.1 + 0.2 + 0.2 [given] = 0.68

     

37. Let f(x) = sec x. Then, f(x + h) = sec (x + h)
    {tex}\therefore{/tex} {tex}\frac{d}{dx}{/tex} (f (x)) = {tex}\mathop {\lim }\limits_{h \to 0} \frac{{f(x + h) – f(x)}}{h}{/tex}
    {tex}\Rightarrow{/tex} {tex}\frac{d}{dx}{/tex} (f (x)) = {tex}\mathop {\lim }\limits_{h \to 0} \frac{{\sec (x + h) – \sec x}}{h}{/tex}
    {tex}\Rightarrow{/tex} {tex}\frac{d}{dx}{/tex} (f (x)) = {tex}\mathop {\lim }\limits_{h \to 0} \frac{{\frac{1}{{\cos (x + h)}} – \frac{1}{{\cos x}}}}{h}{/tex}
    {tex}\Rightarrow{/tex} {tex}\frac{d}{dx}{/tex} (f (x)) = {tex}\mathop {\lim }\limits_{h \to 0} \frac{{\cos x – \cos (x + h)}}{{h\cos x\cos (x + h)}}{/tex}
    {tex}\Rightarrow{/tex} {tex}\frac{d}{dx}{/tex} (f (x)) = {tex}\mathop {\lim }\limits_{h \to 0} \frac{{2\sin \left( {\frac{{x + x + h}}{2}} \right)\sin \left( {\frac{{x + h – x}}{2}} \right)}}{{h\cos x\cos (x + h)}}{/tex} {tex}\left[ {\because \cos C – \cos D = 2\sin \left( {\frac{{C + D}}{2}} \right)\sin \left( {\frac{{D – C}}{2}} \right)} \right]{/tex}
    {tex}\Rightarrow{/tex} {tex}\frac{d}{dx}{/tex} (f (x)) = {tex}\mathop {\lim }\limits_{h \to 0} \frac{{2\sin \left( {\frac{{2x + h}}{2}} \right)\sin \left( {\frac{h}{2}} \right)}}{{h\cos x\cos (x + h)}}{/tex}
    {tex}\Rightarrow{/tex} {tex}\frac{d}{dx}{/tex} (f (x)) = {tex}\mathop {\lim }\limits_{h \to 0} \frac{{\sin \left( {\frac{{2x + h}}{2}} \right)}}{{\cos x\cos (x + h)}} \times \mathop {\lim }\limits_{h \to 0} \frac{{\sin (h/2)}}{{(h/2)}}{/tex}
    {tex}\Rightarrow{/tex} {tex}\frac{d}{dx}{/tex} (f (x)) = {tex}\frac{\sin x}{\cos x \cos x} \times{/tex} 1 = tan x sec x. {tex}\left[ {\because \mathop {\lim }\limits_{h \to 0} \frac{{\sin (h/2)}}{{(h/2)}} = 1} \right]{/tex}
    Hence, {tex}\frac{d}{dx}{/tex} (sec x) = sec x tan x.

    OR

    We have,
    {tex}\mathop {\lim }\limits_{x \to \sqrt {10} } \frac{{\sqrt {7 – 2x} – (\sqrt 5 – \sqrt 2 )}}{{{x^2} – 10}}{/tex}
    = {tex}\mathop {\lim }\limits_{x \to \sqrt {10} } \frac{{\sqrt {7 – 2x} – \sqrt {{{(\sqrt 5 – \sqrt 2 )}^2}} }}{{{x^2} – 10}}{/tex} {tex}\left(\text { form } \frac{0}{0}\right){/tex}
    = {tex}\mathop {\lim }\limits_{x \to \sqrt {10} } \frac{{\sqrt {7 – 2x} – \sqrt {7 – 2\sqrt {10} } }}{{{x^2} – 10}}{/tex} {tex}\left(\text { form } \frac{0}{0}\right){/tex}
    = {tex}\mathop {\lim }\limits_{x \to \sqrt {10} } \frac{{\sqrt {7 – 2x} – \sqrt {7 – 2\sqrt {10} } }}{{{x^2} – 10}}{/tex} {tex}\times{/tex} {tex}\frac{{\sqrt {7 – 2x} + \sqrt {7 – 2\sqrt {10} } }}{{\sqrt {7 – 2x} + \sqrt {7 – 2\sqrt {10} } }}{/tex}
    = {tex}\mathop {\lim }\limits_{x \to \sqrt {10} }{/tex} {tex}\frac{{(7 – 2x) – (7 – 2\sqrt {10} )}}{{(x – \sqrt {10} )(x + \sqrt {10} )\left\{ {\sqrt {7 – 2x} + \sqrt {7 – 2} \sqrt {10} } \right\}}}{/tex}
    = {tex}\mathop {\lim }\limits_{x \to \sqrt {10} }{/tex} {tex}\frac{{ – 2x + 2\sqrt {10} }}{{(x – \sqrt {10} )(x + \sqrt {10} )\left\{ {\sqrt {7 – 2x} + \sqrt {7 – 2} \sqrt {10} } \right\}}}{/tex}
    = {tex}\mathop {\lim }\limits_{x \to \sqrt {10} }{/tex} {tex}\frac{{ – 2(x – \sqrt {10} )}}{{(x – \sqrt {10} )(x + \sqrt {10} )\{ \sqrt {7 – 2x} + \sqrt {7 – 2\sqrt {10} } \} }}{/tex}
    = {tex}\mathop {\lim }\limits_{x \to \sqrt {10} }{/tex} {tex}\frac{{ – 2}}{{(x + \sqrt {10} )\left\{ {\sqrt {7 – 2x} + \sqrt {7 – 2\sqrt {10} } } \right\}}}{/tex}
    = {tex}\mathop {\lim }\limits_{x \to \sqrt {10} }{/tex} {tex}\frac{{ – 2}}{{2\sqrt {10} \left\{ {\sqrt {7 – 2\sqrt {10} } + \sqrt {7 – 2\sqrt {10} } } \right\}}}{/tex}
    = {tex}\frac{{ – 1}}{{\sqrt {10} \times 2 \times \sqrt {7 – 2\sqrt {10} } }}{/tex} = {tex}\frac{{ – 1}}{{2\sqrt {10} (\sqrt 5 – \sqrt 2 )}}{/tex} {tex}\left[\because(\sqrt{5}-\sqrt{2})^{2}=7-2 \sqrt{10}\right]{/tex}
    = {tex}\frac{{ – 1}}{{2\sqrt {10} }} \times \frac{{(\sqrt 5 + \sqrt 2 )}}{3}{/tex} = –{tex}\frac{(\sqrt{5}+\sqrt{2})}{6 \sqrt{10}}{/tex}

38. Let the three numbers be {tex}\frac { a } { r }{/tex}, a and ar.
    Their sum = {tex}\frac { a } { r }{/tex} + a + ar = {tex}\frac { 13 } { 12 }{/tex}
    {tex}\Rightarrow{/tex} {tex}a \left[ \frac { 1 } { r } + 1 + r \right] = \frac { 13 } { 12 }{/tex}
    {tex}\Rightarrow{/tex} {tex}a \left[ \frac { 1 + r + r ^ { 2 } } { r } \right] = \frac { 13 } { 12 }{/tex}
    {tex}\Rightarrow{/tex} a (1 + r + r²) = {tex}\frac { 13 } { 12 } r{/tex} …(i)
    Their product = {tex}\frac { a } { r }{/tex} {tex}\times{/tex} a{tex}\times{/tex} ar = -1 {tex}\Rightarrow{/tex} a³ = -1
    {tex}\Rightarrow{/tex} a = -1 [taking cube root on both sides] …(ii)
    On putting the value of a in Eq. (i), we get
    (- 1) [1 + r + r²] = {tex}\frac { 13 } { 12 } r{/tex}
    {tex}\Rightarrow{/tex} – 1 – r – r² = {tex}\frac { 13 } { 12 } r{/tex}
    {tex}\Rightarrow{/tex} – 12 – 12r – 12r² = 13r
    {tex}\Rightarrow{/tex} 12r² + 25r + 12 = 0
    {tex}\Rightarrow{/tex} 12r² + 16r + 9r + 12 = 0
    {tex}\Rightarrow{/tex} 4r(3r + 4) + 3(3r + 4) = 0
    {tex}\Rightarrow{/tex} (4r + 3) (3r + 4) = 0
    {tex}\Rightarrow{/tex} Either 3r + 4 = 0 or 4r + 3 = 0
    {tex}\Rightarrow{/tex} r = {tex}-\frac { 4 } { 3 }{/tex} or r = {tex}\frac { – 3 } { 4 }{/tex}
    When a  = – 1 and r = – {tex}\frac { 4 } { 3 }{/tex}, then the numbers are
    {tex}\frac { – 1 } { – 4 / 3 } , – 1 , – 1 \times \frac { – 4 } { 3 } i . e . , \frac { 3 } { 4 } , – 1 , \frac { 4 } { 3 }{/tex}
    And when a = – 1 and r = – {tex}\frac { 3 } { 4 }{/tex}, then the numbers are
    {tex}\frac { – 1 } { – 3 / 4 } , – 1 , – 1 \times \frac { – 3 } { 4 } \text { i.e., } \frac { 4 } { 3 } , – 1 , \frac { 3 } { 4 }{/tex}
39. LHS = tan ({tex}\alpha{/tex} – {tex}\beta{/tex}) = {tex}\frac{\sin 2 \beta}{5-\cos 2 \beta}{/tex}
    = {tex}\frac{\tan \alpha-\tan \beta}{1+\tan \alpha \tan \beta}{/tex}
    = {tex}\frac{\frac{3}{2} \tan \beta-\tan \beta}{1+\frac{3}{2} \tan \beta \tan \beta}{/tex} … [{tex}\because{/tex} 2 tan{tex}\alpha{/tex} = 3 tan{tex}\beta{/tex} {tex}\Rightarrow{/tex} tan{tex}\alpha{/tex} = {tex}\frac{3}{2}{/tex}tan{tex}\alpha{/tex}] = {tex}\frac{\tan \beta\left(\frac{3}{2}-1\right)}{1+\frac{3}{2} \tan ^{2} \beta}{/tex}
    = {tex}\frac{\frac{1}{2} \tan \beta}{1+\frac{3}{2} \tan ^{2} \beta}{/tex}
    = {tex}\frac{\frac{1}{2} \frac{\sin \beta}{\cos \beta}}{1+\frac{3}{2} \cdot\left(\frac{\sin \beta}{\cos \beta}\right)^{2}}{/tex} … [{tex}\because{/tex} tan{tex}\beta{/tex} = {tex}\frac{\sin \beta}{\cos \beta}{/tex}] = {tex}\frac{\frac{\sin \beta}{2 \cos \beta}}{1+\frac{3 \sin ^{2} \beta}{2 \cos ^{2} \beta}}{/tex}
    = {tex}\frac{\frac{\sin \beta}{2 \cos \beta}}{\frac{2 \cos ^{2} \beta+3 \sin ^{2} \beta}{2 \cos ^{2} \beta}}{/tex}
    = {tex}\frac{2 \cos ^{2} \beta \sin \beta}{2 \cos \beta\left(2 \cos ^{2} \beta+3 \sin ^{2} \beta\right)}{/tex}
    = {tex}\frac{2 \cos \beta \sin \beta}{2\left(2 \cos ^{2} \beta+3 \sin ^{2} \beta\right)}{/tex}
    = {tex}\frac{\sin 2 \beta}{2\left(2 \cos ^{2} \beta\right)+3\left(2 \sin ^{2} \beta\right)}{/tex} .. {{tex}\because{/tex} sin 2x = 2(sin x)(cos x)}
    = {tex}\frac{\sin 2 \beta}{2(1+\cos 2 \beta)+3(1-\cos 2 \beta)}{/tex} … {{tex}\because{/tex} 2 cos² x = 1 + cos 2x and 2 sin² x = 1 – cos 2x}
    = {tex}\frac{\sin 2 \beta}{2+2 \cos 2 \beta+3-3 \cos 2 \beta}{/tex}
    = {tex}\frac{\sin 2 \beta}{5-\cos 2 \beta}{/tex}
    LHS = RHS
    Hence Proved

    OR

    LHS = {tex}cos 2x\times  cos{/tex} {tex}\frac { x } { 2 }{/tex} {tex}- cos 3x \times cos{/tex} {tex}\frac { 9 x } { 2 }{/tex}
    = {tex}\frac { 1 } { 2 }{/tex} [2 cos 2x {tex}\times{/tex} cos {tex}\frac { x } { 2 }{/tex}  – 2 cos {tex}\frac { 9 x } { 2 }{/tex} {tex}\times{/tex} cos 3x]  [multiplying numerator and denominator by 2] = {tex}\frac { 1 } { 2 }{/tex} [cos {tex}\left( 2 x + \frac { x } { 2 } \right){/tex} + cos {tex}\left( 2 x – \frac { x } { 2 } \right){/tex} – cos {tex}\left( \frac { 9 x } { 2 } + 3 x \right){/tex} – cos {tex}\left( \frac { 9 x } { 2 } – 3 x \right){/tex}]  [{tex}\because{/tex} {tex}2 cos\ x \times cos\ y = cos (x + y) + cos (x – y){/tex}] = {tex}\frac { 1 } { 2 }{/tex} [ cos {tex}\frac { 5 x } { 2 }{/tex} + cos {tex}\frac { 3 x } { 2 }{/tex} – cos {tex}\frac { 15 x } { 2 }{/tex} – cos {tex}\frac { 3 x } { 2 }{/tex}] = {tex}\frac { 1 } { 2 }{/tex} [cos {tex}\frac { 5 x } { 2 }{/tex} – cos {tex}\frac { 15 x } { 2 }{/tex}] = {tex}\frac { 1 } { 2 }{/tex} [- 2 sin {tex}\left( \frac { \frac { 5 x } { 2 } + \frac { 15 x } { 2 } } { 2 } \right) \sin \left( \frac { \frac { 5 x } { 2 } – \frac { 15 x } { 2 } } { 2 } \right) ]{/tex}  [{tex}\because{/tex}{tex} cos\ x – cos\ y = – 2sin{/tex} {tex}\left( \frac { x + y } { 2 } \right) \cdot \sin \left( \frac { x – y } { 2 } \right) ]{/tex}
    = – sin 5x sin {tex}\left( \frac { – 5 x } { 2 } \right){/tex} {tex}= sin 5x \times  sin{/tex} {tex}\frac { 5 x } { 2 }{/tex} [{tex}\because{/tex} {tex}sin (-\theta ) = – sin{/tex}{tex}\theta{/tex}] = RHS
    {tex}\therefore{/tex} LHS = RHS
    Hence proved.

40. Section E
    1. The path traced by Javelin is parabola. A parabola is the set of all points in a plane that are equidistant from a fixed line and a fixed point (not on the line) in the plane.
       compare x² = -16y with x² = -4ay
       {tex}\Rightarrow{/tex} – 4a = -16
       {tex}\Rightarrow{/tex} a = 4
       coordinates of focus for parabola x² = -4ay is (0, -a)
       {tex}\Rightarrow{/tex} coordinates of focus for given parabola is (0, -4)
    2. compare x² = -16y with x² = -4ay
       {tex}\Rightarrow{/tex} -4a = -16
       {tex}\Rightarrow{/tex} a = 4
       Equation of directrix for parabola x² = -4ay is y = a
       {tex}\Rightarrow{/tex} Equation of directrix for parabola x² = -16y is y = 4
       Length of latus rectum is 4a = 4 × 4 = 16
    3. Equation of parabola with axis along y – axis
       x² = 4ay
       which passes through (5, 2)
       {tex}\Rightarrow{/tex} 25 = 4a {tex}\times{/tex} 2
       {tex}\Rightarrow{/tex} 4a = {tex}\frac{25}{2}{/tex}
       hence required equation of parabola is
       {tex}x^2=\frac{25}{2} y{/tex}
       {tex}\Rightarrow{/tex} 2x² = 25y
       Equation of directrix is y= -a
       Hence required equation of directrix is 8y + 25 = 0.
       OR
       Since the focus (2,0) lies on the x-axis, the x-axis itself is the axis of the parabola.
       Hence the equation of the parabola is of the form either y² = 4ax or y² = -4ax.
       Since the directrix is x = -2 and the focus is (2,0), the parabola is to be of the form y² = 4ax with a = 2.
       Hence the required equation is y² = 4(2)x = 8x
       length of latus rectum = 4a = 8
    1. By using formula,
       {tex}{\sigma ^2} = \frac{1}{N}\left[ {\sum\limits_{i = 1}^n {{f_i}} {{\left( {{x_i} – \bar x} \right)}^2}} \right]{/tex}

       xi	fi	fixi	xi – {tex}\overline x{/tex}	(xi – {tex}\overline x{/tex})2	fi(xi – {tex}\overline x{/tex})2
       4	3	12	-10	100	{tex}300{/tex}
       8	5	40	-6	36	{tex}180{/tex}
       11	9	99	-3	9	{tex}81{/tex}
       17	5	85	3	9	{tex}45{/tex}
       20	4	80	6	36	{tex}144{/tex}
       24	3	72	10	100	{tex}300{/tex}
       32	1	32	18	324	{tex}324{/tex}
       Total	30	420			{tex}1374{/tex}

       Given, N = {tex}\sum f_i{/tex} = 30, {tex}\sum f_ix_i{/tex} = 420 and {tex}\sum f_{i}\left(x_{i}-\overline{x}\right)^{2}{/tex} = 1374
       {tex}\therefore{/tex} {tex}\bar x = \frac{{\sum\limits_{i = 1}^7 {{f_i}} {x_i}}}{N}{/tex} = {tex}\frac{420}{30}{/tex} = 14
       Variance ({tex}\sigma^2{/tex}) = {tex}\frac{1}{N}\sum\limits_{i = 1}^7 {{f_i}} {\left( {{x_i} – \bar x} \right)^2}{/tex} = {tex}\frac{1}{30}{/tex} {tex}\times{/tex} {tex}1374 = 45.8{/tex}
       Standard deviation, {tex}\sigma{/tex} = {tex}\sqrt{\sigma^2}{/tex} = {tex}\sqrt{45.8}{/tex} = 6.77

    2. Variance ({tex}\sigma^2{/tex}) = {tex}\frac{1}{N}\sum\limits_{i = 1}^7 {{f_i}} {\left( {{x_i} – \bar x} \right)^2}{/tex} = {tex}\frac{1}{30}{/tex} {tex}\times{/tex} {tex}1374 = 45.8{/tex}
    3. Given, N = {tex}\sum f_i{/tex} = 30, {tex}\sum f_ix_i{/tex} = 420 and {tex}\sum f_{i}\left(x_{i}-\overline{x}\right)^{2}{/tex} = 1374
       {tex}\therefore{/tex} {tex}\bar x = \frac{{\sum\limits_{i = 1}^7 {{f_i}} {x_i}}}{N}{/tex} = {tex}\frac{420}{30}{/tex} = 14
       OR
       {tex}\sigma^{2}=\frac{1}{N} \Sigma\left(x_{i}-\bar{x}\right){/tex}
    1. Since, at least 3 questions from each part have to be selected
       

       Part I	Part II
       3	5
       4	4
       3	5

       So number of ways are
       3 questions from part I and 5 questions from part II can be selected in {tex}n^8 C_3 \times{ }^7 C_5{/tex} ways
       4 questions from part I and 4 questions from part II can be selected in {tex}{ }^8 C_4 \times{ }^7 C_4{/tex} ways
       5 questions from part I and 3 questions from part II can be selected in {tex}{ }^8 C_5 \times{ }^7 C_3{/tex} ways
       So required number of ways are
       {tex}{ }^8 C_3 \times{ }^7 C_5+{ }^8 C_4 \times{ }^7 C_4+{ }^8 C_5 \times{ }^7 C_3{/tex}
       {tex}\Rightarrow \frac{8 !}{5 ! \times 3 !} \times \frac{7 !}{5 ! \times 2 !}+\frac{8 !}{4 ! \times 4 !} \times \frac{7 !}{4 ! \times 3 !}+\frac{8 !}{5 ! \times 3 !} \times \frac{7 !}{4 ! \times 3 !}{/tex}
       {tex}\Rightarrow \frac{8 \times 7 \times 6}{3 \times 2 \times 1} \times \frac{7 \times 6}{2 \times 1}+\frac{8 \times 7 \times 6 \times 5}{4 \times 3 \times 2 \times 1} \times \frac{7 \times 6 \times 5}{3 \times 2 \times 1}+\frac{8 \times 7 \times 6}{3 \times 2 \times 1} \times \frac{7 \times 6 \times 5 \times 4}{4 \times 3 \times 2 \times 1}{/tex}
       {tex}\Rightarrow{/tex} 56 {tex}\times{/tex} 21 + 70 {tex}\times{/tex} 35 + 56 {tex}\times{/tex} 35
       {tex}\Rightarrow{/tex} 1176 + 2450 + 1960
       {tex}\Rightarrow{/tex} 5586

    2. Ashish is selecting 3 questions from part I so he has to select remaining 5 questions from part II
       The number of ways of selection is
       3 questions from part I and 5 questions from part II can be selected in {tex}{ }^8 C_3 \times{ }^7 C_5{/tex} ways
       {tex}\Rightarrow{ }^8 C_3 \times{ }^7 C_5{/tex}
       {tex}\Rightarrow \frac{8 !}{5 ! \times 3 !} \times \frac{7 !}{5 ! \times 2 !}{/tex}
       {tex}\Rightarrow \frac{8 \times 7 \times 6}{3 \times 2 \times 1} \times \frac{7 \times 6}{2 \times 1}{/tex}
       {tex}\Rightarrow{/tex} 56 {tex}\times{/tex} 21
       {tex}\Rightarrow{/tex} 1176
    3. 4 questions from part I and 4 questions from part II can be selected
       {tex}{ }^8 C_4 \times{ }^7 C_4{/tex}
       {tex}\Rightarrow \frac{8 !}{4 ! \times 4 !} \times \frac{7 !}{4 ! \times 3 !}{/tex}
       {tex}\Rightarrow \frac{8 \times 7 \times 6 \times 5}{4 \times 3 \times 2 \times 1} \times \frac{7 \times 6 \times 5}{3 \times 2 \times 1}{/tex}
       {tex}\Rightarrow{/tex} 70 {tex}\times{/tex} 35
       {tex}\Rightarrow{/tex} 2450
       OR
       6 questions from part I and 2 questions from part II can be selected or
       2 questions from part I and 6 questions from part II can be selected
       {tex}\Rightarrow{ }^8 C_6 \times{ }^7 C_2+{ }^8 C_2 \times{ }^7 C_6{/tex}
       {tex}\Rightarrow \frac{8 !}{6 ! \times 2 !} \times \frac{7 !}{2 ! \times 5 !}+\frac{8 !}{6 ! \times 2 !} \times \frac{7 !}{1 ! \times 6 !}{/tex}
       {tex}\Rightarrow \frac{8 \times 7}{2 \times 1} \times \frac{7 \times 6}{2 \times 1}+\frac{8 \times 7}{2 \times 1} \times 7{/tex}
       {tex}\Rightarrow{/tex} 28 {tex}\times{/tex} 21 + 28 {tex}\times{/tex} 7
       {tex}\Rightarrow{/tex} 588 + 196 = 784
       Download myCBSEguide App for Effective Exam Preparation and PracticeFor comprehensive exam preparation, download the myCBSEguide app. It provides complete study material for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams. With sample papers, NCERT solutions, practice tests, and revision notes, the app ensures that students are well-equipped to excel in their exams. Whether you are struggling with specific topics or looking to reinforce your understanding, these sample papers offer a range of problems to solve.

       Additionally, teachers can use the Examin8 app to create customized exam papers with their own name and logo, allowing for personalized assessments. Whether you’re a student looking to improve your exam readiness or a teacher crafting custom exams, both myCBSEguide Website and Examin8 Website offer the perfect tools for success. CBSE Sample Papers Class 11 Mathematics help students assess their preparation level and identify areas that need more focus.

CBSE Sample Papers for Class 11 2025

• Physics
• Chemistry
• Mathematics
• Biology
• Accountancy
• Economics
• Business Studies
• Computer Science
• Informatics Practices
• English Core
• Hindi Core
• Hindi Elective
• History
• Political Science
• Geography
• Sociology
• Physical Education
• Other Subjects

Download Class 11 Sample Papers for All Subjects from myCBSEguide

For comprehensive exam preparation, download Class 11 sample papers for Physics, Chemistry, Biology, History, Political Science, Economics, Geography, Computer Science, Home Science, Accountancy, and Business Studies from the myCBSEguide app or website. myCBSEguide provides sample papers with solutions, chapter-wise test papers, NCERT solutions, NCERT Exemplar solutions, and quick revision notes to help students prepare effectively for their exams.

Additionally, CBSE guess papers and important question papers are available for free download to assist students in their revision. All these study resources are accessible through the myCBSEguide app, the best app for CBSE students, and our user-friendly website. Start your preparation today with myCBSEguide for the latest and most accurate study materials. These CBSE Sample Papers Class 11 Mathematics are designed to mirror the actual exam format, helping students become familiar with the types of questions they will face.