CBSE Class 11 Applied Maths Sample Papers 2025

CBSE Class 11 Applied Maths Sample Papers 2025

Download free CBSE Class 11 Applied Maths sample papers for 2025 on the myCBSEguide app! These model question papers are based on the latest CBSE marking scheme and blueprint of Applied Maths for the academic session 2024-25, ensuring they follow the most up-to-date exam pattern. Download the latest CBSE Class 11 Applied Maths model question papers for 2025 from myCBSEguide. These papers follow the updated CBSE blueprint, making them perfect for practicing essential concepts. Model question papers provide insights into the expected exam format and difficulty level. Use these test papers to refine your exam strategy and boost confidence. The content is regularly updated to reflect any changes in the CBSE curriculum. Get started now and boost your preparation with myCBSEguide for free, reliable, and comprehensive study material! Download free practice papers for CBSE Class 11 Applied Maths from the Examin8 and  myCBSEguide app.

Class 11 Applied Maths Model Papers 2024-25

Applied Mathematics is a newly introduced subject in senior secondary classes, specifically designed to provide a more relevant option for commerce students. Previously, students in the commerce stream had to study core mathematics, which was not directly applicable to their field of study. The Applied Maths curriculum focuses on topics that are more aligned with the practical needs of commerce students, making it a valuable addition to their academic path. These papers are designed to help you understand the exam pattern and improve problem-solving skills. With detailed solutions, these practice papers provide comprehensive coverage of key topics. Access a wide range of CBSE Class 11 Applied Maths Sample Papers 2025.

To help students excel in this subject, we are offering CBSE Class 11 Applied Maths Sample Papers 2025. These sample papers have been carefully crafted by our team of expert teachers, in accordance with the latest CBSE blueprint and guidelines issued by CBSE New Delhi. Download these sample question papers today to practice and improve your understanding of key concepts in Applied Maths.

Class 11 – Applied Mathematics Sample Paper (2024-25)

---

Class 11 – Applied Maths
Sample Paper – 01 (2024-25)

---

Maximum Marks: 80
Time Allowed: : 3 hours

---

General Instructions:

Read the following instructions very carefully and strictly follow them:

1. This Question paper contains 38 questions. All questions are compulsory.
2. This Question paper is divided into five Sections – A, B, C, D and E.
3. In Section A, Questions no. 1 to 18 are multiple choice questions (MCQs) and Questions no. 19 and 20 are Assertion-Reason based questions of 1 mark each.
4. In Section B, Questions no. 21 to 25 are Very Short Answer (VSA)-type questions, carrying 2 marks each.
5. In Section C, Questions no. 26 to 31 are Short Answer (SA)-type questions, carrying 3 marks each.
6. In Section D, Questions no. 32 to 35 are Long Answer (LA)-type questions, carrying 5 marks each.
7. In Section E, Questions no. 36 to 38 are case study-based questions carrying 4 marks each.
8. There is no overall choice. However, an internal choice has been provided in 2 questions in Section B, 2 questions in Section C, 2 questions in Section D and one sub-part each in 2 questions of Section E.
9. Use of calculators is not allowed.

---

1. Section A
2. Three persons A, B and C fire a target in turn. Their probabilities of hitting the target are 0.4, 0.3 and 0.2 respectively. The probability of two hits is:

   a)0.336

   b)0.188

   c)0.024

   d)0.452

3. If two distributions A and B have same mean and their variance are 81 and 100 respectively, then which distribution has more variability

   a)A

   b)can’t say

   c)B

   d)both are equal

4. Deduction of principal of home loan is allowed under section

   a)80D

   b)80E

   c)24

   d)80C

5. The value of {tex}\left(5 \frac{1}{16}\right)^{-\frac{3}{4}}{/tex} is

   a){tex}\frac{9}{4}{/tex}

   b){tex}\frac{4}{9}{/tex}

   c){tex}\frac{27}{8}{/tex}

   d){tex}\frac{8}{27}{/tex}

6. If f(x) ={tex}\sqrt { – x} {/tex}, then domain of fof is

   a){tex}({\rm{ }}0,\infty ){/tex}

   b)none of these

   c){tex}( – \infty ,\infty ){/tex}

   d){tex}( – \infty ,{\rm{ }}0){/tex}

7. If {tex}\frac{\log x}{a-b}=\frac{\log y}{b-c}=\frac{\log z}{c-a}{/tex}, then xyz is equal to:

   a)2

   b)0

   c)1

   d)-1

8. A card is picked at random from a pack of 52 playing cards. Given that the picked card is a queen, the probability of this card to be a card of hearts is:

   a){tex}\frac 4{14}{/tex}

   b){tex}\frac 14{/tex}

   c){tex}\frac 13{/tex}

   d){tex}\frac 12{/tex}

9. The equation of a circle concentric with the circle x² + y² – 6x + 12y + 15 = 0 and double its area is:

   a)x² + y² – 6x + 12y + 30 = 0

   b)x² + y² – 6x + 12y – 30 = 0

   c)x² + y² – 6x + 12y + 45 = 0

   d)x² + y² – 6x + 12y – 15 = 0

10. If ignorance is to education, then disease is to ________

    a)medicine

    b)nurse

    c)doctor

    d)hospital

11. Which of the following is not a measure of central tendency:

    a)range

    b)mode

    c)median

    d)mean

12. Characteristic of logarithm of a number 14768 is

    a)3

    b)2

    c)4

    d)5

13. The difference between simple interest and compound interest on ₹ 15000 for one yea 8% per annum calculated half-yearly is:

    a)₹ 24

    b)₹ 20

    c)₹ 22

    d)₹ 26

14. A retailer purchases a fan for ₹ 1500 from a wholesaler and sells it to a consumer at 10% profit. If the sales are intra-state and the rate of GST is 12%, the tax (under GST) received by the Central Government is:

    a)₹ 90

    b)₹ 18

    c)₹ 198

    d)₹ 99

15. A problem in mathematics is given to three students A, B, C and their chances of solving the problem {tex}\frac{1}{2}, \frac{1}{3}{/tex} are {tex}\frac 14{/tex} and respectively. The probability that the problem is solved is:

    a){tex}\frac 34{/tex}

    b){tex}\frac 23{/tex}

    c){tex}\frac 58{/tex}

    d){tex}\frac 12{/tex}

16. A bag A contains 3 white, 2 red balls and a bag B contains 4 white and 5 red balls. One ball is drawn at random from one of the bags and is found to be red, then the probability that it was drawn from bag B is

    a){tex}\frac{20}{43}{/tex}

    b){tex}\frac{25}{43}{/tex}

    c){tex}\frac{27}{43}{/tex}

    d){tex}\frac{21}{43}{/tex}

17. The compound interest on ₹ 30,000 at 7% per annum is ₹ 4347. This period (in years) is:

    a)2

    b)4

    c)3

    d){tex}2\frac{1}{2}{/tex}

18. The number of 4 digit numbers that can be formed with the digits 2, 3, 4, 7 and using each digit only once is

    a)24

    b)96

    c)120

    d)100

19. A relation R is defined from {2, 3, 4, 5} to {3, 6, 7, 10} by: x R y {tex}\Leftrightarrow{/tex} x is relatively prime to y. Then domain of R is

    a){2, 3, 4, 5}

    b){3, 5}

    c){2, 3, 5}

    d){2, 3, 4}

20. Assertion (A): The difference between maximum and minimum values of variate is called Range.
    Reason (R): Coeff. of Range = {tex}\frac{L-S}{L+S}{/tex}
    Where, L is the largest value
    S is the smallest value

    a)Both A and R are true and R is the correct explanation of A.

    b)Both A and R are true but R is not the correct explanation of A.

    c)A is true but R is false.

    d)A is false but R is true.

21. Assertion (A): If nth term of an A.P. is a_n = pn + q, then common difference of A.P. is 2p.
    Reason (R): Common difference of an A.P. is d = a_n – a_{n – 1}.

    a)Both A and R are true and R is the correct explanation of A.

    b)Both A and R are true but R is not the correct explanation of A.

    c)A is true but R is false.

    d)A is false but R is true.

    Boost Your Exam Preparation with the myCBSEguide App – Download Now! For more visit our Website myCBSEGuide
    Get ready to ace your exams by downloading the myCBSEguide app! Get ready for your exams with CBSE Class 11 Applied Maths Sample Papers 2025. It provides complete study resources for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams, helping you stay ahead in your studies. With access to a wide range of practice questions, sample papers, and mock tests, the myCBSEguide app is your go-to tool for effective exam preparation.

    Examin8 is the ideal app for teachers, allowing them to create customized question papers with their own branding, logo, and name. Download the myCBSEguide and Examin8 apps today to enhance your learning experience and achieve exam success.

22. Section B
23. The average of five consecutive numbers is x. If next two numbers are also included, then show that the average will increase by 1.
24. In a certain code language 327 means Truth is eternal, 7983 means ‘Enmity is not eternal’ and 9426 means Truth does not perish. What does Enmity mean in this language?

    OR

    In a certain language TABLE is coded as NBDXJ, how CHAIR is coded?

25. A pipe can fill a tank in 12 hours. By mistake, a waste pipe at the bottom is left opened and the tank is filled in 16 hours. If the tank is full, how much time will the waste pipe take to empty it?
26. Differentiate the following function w.r.t. x: {tex}\frac{\sqrt{x^{2}+1}-x}{\sqrt{x^{2}+1}+x}{/tex}

    OR

    Find {tex}\frac {dy}{dx}{/tex}: (x + y)² = 2axy

27. Represent the number (11001.0101)₂ in decimal system.
28. Section C
29. If a, b, c are pth, qth and rth terms respectively of an A.P, prove that: a(q – r) + b(r – p) + c(p – q) = 0

    OR

    If a, b, c, d are in G.P., prove that aⁿ + bⁿ, bⁿ + cⁿ, cⁿ + dⁿ are in G.P.

30. Rohit is the husband of Vanshika. Sumita is the sister of Rohit. Anushka is the sister of Vanshika. How Anushka is related to Rohit?
31. Find the domain and the range of the given function: f(x) = {tex}\frac{1}{1-x^{2}}{/tex}
32. A man deposits ₹ 5000 in the State Bank saving account allowing simple interest at 4{tex}\frac 12{/tex} percent per year. At the end of the first year, he transfers the entire amount to a fixed deposit account for 2 years at 7% per annum compounded annually. Find the total amount of money in the name of the depositor at the end of the third year, nearest to a rupee.
33. A family in Delhi consumed 48 kL of water in a month. Calculate the water bill for the month. The tariff plan for Delhi is as given below:
    

    Monthly Consumption (in kL)	Service Charge	Water Consumption Charge per kL
    Up to 20	₹ 146.41	₹5.27
    20 – 30	₹219.62	₹26.36
    >30	₹292.82	₹43.93

    Also, sewerage charges are applicable at 60% of the water consumption charges.

34. Using properties of sets and their complements prove that:
    1. (A {tex}\cup{/tex} B) {tex}\cap{/tex} (A {tex}\cap{/tex} B’) = A
    2. A – (A {tex}\cap{/tex} B) = A – B
35. Section D
36. How many three digit odd numbers can be formed by using the digits 1, 2, 3, 4, 5, 6 when
    1. the repetition of digits is not allowed?
    2. the repetition of digits is allowed?

    OR

    From 7 consonants and 4 vowels, how many different words can be formed consisting of 3 consonants and 2 vowels?

37. Evaluate : {tex}\mathop {Lim}\limits_{x \to 0} \frac{{{e^{{x^2}}} – \cos x}}{{{x^2}}}{/tex}
38. Ten competitors in a musical test were ranked by the three judges x, y and z in the following order:
    

    Ranks by X:	1	6	5	10	3	2	4	9	7	8
    Ranks by Y:	3	5	8	4	7	10	2	1	6	9
    Ranks by Z:	6	4	9	8	1	2	3	10	5	7

    Using rank correlation method, discuss which pair of judges has the nearest approach to common likings in music.

    OR

    Calculate the mean and standard deviation for the following distribution:

    Marks:	20-30	30-40	40-50	50-60	60-70	70-80	80-90
    No. of students:	3	6	13	15	14	5	4

39. A person has the following informations for his tax return for the financial year 2019-20
    Salary – ₹ 10 Lacs
    Income from other sources – ₹ 3 Lacs
    Savings – ₹ 2.5 Lacs Advance tax paid – ₹ 60,000
    The rates of income tax for assessment year 2020-21 are as follows:
    Standard deduction – ₹ 50,000

    Income (₹)	Old rate	New rate	Remark
    Upto ₹ 2.5 lakhs	0%	0%
    ₹ 2.5L – ₹ 5.0L	0%	0%	If net taxable income is < ₹ 5 lakh
    ₹ 2.5L – ₹ 5.0L	5%	5%	If net taxable income is > ₹ 5 lakh
    ₹ 5.0L – ₹ 7.5L	20%	10%
    ₹ 7.5L – 10.0 L	20%	15%
    ₹ 10.0L – 12.5L	30%	20%
    ₹ 12.5L – 15.0L	30%	25%
    > 15L	30%	30%
    Exemptions and Deductions	Yes	No

    Education cess – 4%
    Calculate the income tax according to

    1. old rates
    2. new rates
40. Section E
41. Read the following text carefully and answer the questions that follow:
    During sports day of a school different patterns are made for students to perform. One such pattern is made by putting flags at B(3, 2) and C(4, -1), other flags and lines are to be put based on some calculations. Let us try the different positions.
    1. Find the Equation of BC. (1)
    2. Find the Equation of AC. (1)
    3. Find the Equation of AB. (2)
       OR
       Coordinates of a point which divides medians of a triangle ABC in the ratio 2 : 1 (2)
42. Read the following text carefully and answer the questions that follow:
    An analysis of monthly wages paid to workers in two firms A and B, belonging to the same industry, gives the following results:

    Particulars	Firm A	Firm B
    No. of wage earners	586	648
    Mean of monthly wages	₹ 5253	₹ 5253
    Variance of the distribution of wages	100	121

    

    1. Which firm A or B shows greater variability in individual wages? (1)
    2. Find the standard deviation of the distribution of wages for frim B. (1)
    3. Find the coefficient of variation of the distribution of wages for firm A. (2)
       OR
       Find the amount paid by firm A. (2)
43. Read the following text carefully and answer the questions that follow:
    Mr. Kalra manufactures ball point pens. He sells pens in packets of 100 pieces. It is known that 6% of his products are defective. A packet of pens is selected at random and checked for number of defective pens.
    1. Find the probability that packet contains no defective pen. (1)
    2. Find the probability that packet contains atmost 4 defective pens. (1)
    3. Find the probability that packet contains atleast 2 defective pens. (2)
       OR
       Find the probability that the packet contains exactly 3 defective pens. (2)
       For effective exam preparation and extensive practice, download the myCBSEguide App today! It provides complete study material for CBSE, NCERT, JEE Main, NEET UG, and NDA exams, helping students master key concepts and improve their performance. Access practice questions, sample papers, NCERT solutions, and detailed chapter notes, all in one app.Teachers can also take advantage of the Examin8 App to easily create customized question papers and online exams, featuring their own branding, logo, and name.

       Key Features:

       • Comprehensive study resources for CBSE, JEE Main, NEET UG, and NDA
       • Interactive mock tests and practice papers for exam preparation
       • Customizable exam creation with Examin8 for teachers
       • Affordable plans with both free and premium content

       Visit myCBSEguide Website for a complete study.

Class 11 – Applied Maths
Sample Paper – 01 (2024-25)

---

Solutions of Applied Maths Class 11

1. Section A
2. (b)

   0.188

   Explanation: Here, P(A) = 0.4,P({tex}\bar{A}{/tex}) = 0.6, P(B) = 0.3, P({tex}\bar{B}{/tex}) = 0.7,
   P(C) = 0.2 and P({tex}\bar{C}{/tex}) = 0.8
   {tex}\therefore{/tex} Probability of two hits {tex}=P_{A} \cdot P_{B} \cdot P_{\bar{C}}+P_{A} \cdot P_{\bar{B}} \cdot P_{C}+P_{\bar{A}} \cdot P_{B} \cdot P_{C}{/tex}
   = 0.4 {tex}\times{/tex} 0.3 {tex}\times{/tex} 0.8 + 0.4 {tex}\times{/tex} 0.7 {tex}\times{/tex} 0.2 + 0.6 {tex}\times{/tex} 0.3 {tex}\times{/tex} 0.2
   {tex}={/tex} 0.096 + 0.056 + 0.036 = 0.188

3. (c)

   B

   Explanation: B has more variability, because variance is greater.

4. (d)

   80C

   Explanation: 80C

5. (d)

   {tex}\frac{8}{27}{/tex}

   Explanation: {tex}\left(5 \frac{1}{16}\right)^{-\frac{3}{4}} =\left(\frac{81}{16}\right)^{-\frac{3}{4}}{/tex}{tex}=\left(\frac{16}{81}\right)^{\frac{3}{4}}=\left(\frac{2^4}{3^4}\right)^{\frac{3}{4}}{/tex}
   {tex}=\left(\frac{2}{3}\right)^3=\frac{8}{27} {/tex}

6. (b)

   none of these

   Explanation: {tex}\sqrt {f(x)} {/tex}  is defined only if
   f(x) will be non negative.
   Hence {tex} \sqrt { – f(x)} {\rm{ }}{/tex}is defined only for 0.

7. (c)

   1

   Explanation: 1

8. (b)

   {tex}\frac 14{/tex}

   Explanation: No. of spade cards =13
   No. of queen in spade set =1
   {tex}\therefore{/tex} n(spade queen) = ¹³C₁
   {tex}\therefore{/tex} n (Total no. of cases) = ⁵²C₁
   {tex}\therefore{/tex} P (spade queen) = {tex}\frac{13_{\mathrm{c}_{1}}}{52_{\mathrm{c}_{1}}}{/tex} = {tex}\frac{13}{52}=\frac{1}{4}{/tex}

9. (d)

   x² + y² – 6x + 12y – 15 = 0

   Explanation:

   Given equation of the circle is
   x² + y² – 6x + 12y + 15 = 0 ….. (1)
   or (x – 3)² + (y + 6)² {tex}=(\sqrt{30})^{2}{/tex}
   Hence, centre is (3, -6) and radius is {tex}(\sqrt{30}){/tex}
   Since, the required circle is concentric with above circle.
   Therefore, the center of the required circle is (3, -6)
   Now, it given that
   Area of the required circle
   = 2 {tex}\times{/tex} Area of the given circle
   {tex}\pi r^{2}=2 \times \pi(\sqrt{30})^{2}{/tex}
   {tex}\Rightarrow r^{2} {/tex} = 60
   {tex}\Rightarrow r =\sqrt {60}{/tex}
   So, The required equation of the circle is (x – 3)² + (y + 6)² = 60
   {tex}\Rightarrow{/tex} x² + y² – 6x + 12y – 15 = 0

10. (a)

    medicine

    Explanation: medicine

11. (a)

    range

    Explanation: Meausre of central tendencies give the middle most or average value whereas range gives the difference between highest and lowest value.

12. (c)

    4

    Explanation: As 14768 = 1.4768 {tex}\times{/tex} 10⁴

13. (a)

    ₹ 24

    Explanation: For C.I.:
    C.I. = 15000 {tex}\left(1+\frac{4}{100}\right)^2{/tex} – 15000
    = 15000 {tex}\left[\frac{26}{25} \times \frac{26}{25}-1\right]=\frac{15000 \times 51}{25 \times 25}{/tex} = ₹ 1224.
    S.I. = {tex}\frac{15000 \times 51}{25 \times 25}{/tex} = ₹ 1200
    {tex}\therefore{/tex} Difference between C.I. and S.I. =  ₹ 1224 – ₹ 1200 = ₹24

14. (d)

    ₹ 99

    Explanation: ₹ 99

15. (a)

    {tex}\frac 34{/tex}

    Explanation: as P(problem solved)
    = 1 – P(none solves)
    = 1 – {tex}\frac{1}{2} \times \frac{2}{3} \times \frac{3}{4}{/tex} = 1 – {tex}\frac{1}{4}{/tex} = {tex}\frac{3}{4}{/tex}

16. (b)

    {tex}\frac{25}{43}{/tex}

    Explanation:

    
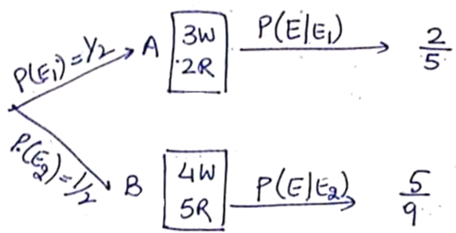
    E₁ : Ball drawn from bag A.
    E₂ : Ball drawn from bag B.
    E : Drawn ball is Red:
    By Bayes’s tho.,
    {tex}P\left(E_2 \mid E\right){/tex}   = {tex}\frac{P\left(E_2\right) P\left(E \mid E_2\right)}{P\left(E_1\right) P\left(E \mid E_1\right)+P\left(E_2\right) P\left(E \mid E_2\right)}{/tex}
    = {tex}\frac{\frac{1}{2} \cdot \frac{5}{9}}{\frac{1}{2} \cdot \frac{2}{5}+\frac{1}{2} \cdot \frac{5}{9}}{/tex}
    = {tex}\frac{\frac{5}{18}}{\frac{2}{10}+\frac{5}{18}}{/tex} {tex}=\frac{5}{18} \times \frac{90}{43} {/tex}
    = {tex}\frac{25}{43}{/tex}
    P(E₂ | E) = {tex}\frac{25}{43}{/tex}

17. (a)

    2

    Explanation: Amount = ₹(30,000 + 4347) = ₹ 34347
    Let the time be n years.
    Then, using formula of compound interest A_n = P(1 + i)ⁿ
    Here, A_n = 34347, i = {tex}\frac{7}{100}{/tex} = 0.04 and P = 30,000
    {tex}\therefore{/tex} 34347 = 30,000 (1 + 0.07)ⁿ
    {tex}\therefore{/tex} (1.07)ⁿ = {tex}\frac{34347}{30,000}{/tex}
    {tex}\Rightarrow{/tex} {tex}\left(\frac{107}{100}\right)^n{/tex} = {tex}\frac{11449}{10000}{/tex}
    {tex}\Rightarrow{/tex} {tex}\left(\frac{107}{100}\right)^n{/tex} = {tex}\left(\frac{107}{100}\right)^2{/tex}
    = n = 2 years.

18. (a)

    24

    Explanation: Given digits 2, 3, 4 and 7.
    We have to find 4-digit numbers using these digits.
    {tex}\therefore{/tex} Required number of ways
    = ⁴P₄ = 4! = 4 {tex}\times{/tex} 3 {tex}\times{/tex} 2 {tex}\times{/tex} 1 = 24

19. (a)

    {2, 3, 4, 5}

    Explanation: Relatively prime numbers are those numbers that have only 1 as the common factor.
    So, according to this definition we get to know that (2, 3), (2, 7), (3, 7), (3, 10), (4, 3), (4, 7), (5, 3), (5, 6), (5, 7) are relatively prime.
    So, R = {(2, 3), (2, 7), (3, 7), (3, 10), (4, 3), (4, 7), (5, 3), (5, 6) ,(5, 7)}.
    Therefore, the Domain of R is the values of x or the first element of the ordered pair.
    So, Domain = {2, 3, 4, 5}

20. (b)

    Both A and R are true but R is not the correct explanation of A.

    Explanation: Assertion: True
    Range = maximum value -minimum value
    R = L – S
    Reason: True
    But not the correct explanation of Assertion.

21. (d)

    A is false but R is true.

    Explanation: In an A.P., d = a_n – a_n-1
    {tex}\therefore{/tex} Reason is true.
    Now, given a_n = pn + q
    {tex}\Rightarrow{/tex} d = a_n – a_n-1
    {tex}\Rightarrow{/tex} d = pn + q – {p (n – 1) + q}
    {tex}\Rightarrow{/tex} d = pn + q – pn + p – q
    {tex}\Rightarrow{/tex} d = p
    Assertion is false.

    Visit our website myCBSEGuide. Boost your exam preparation by downloading the myCBSEguide App! It offers a complete range of study materials for CBSE, NCERT, JEE Main, NEET UG, and NDA exams. From practice questions and NCERT solutions to sample papers and detailed chapter notes, this app is your go-to resource for comprehensive learning.

    For educators, the Examin8 Website provides a powerful platform to craft customized online tests and question papers with personalized branding, including logos and names. Teachers can easily create tailored assessments to enhance student learning.

    Key Benefits of myCBSEguide App:

    • Extensive resources for CBSE, JEE, NEET, and NDA preparation
    • Timely practice through interactive tests and mock exams
    • Convenient exam creation and customization for educators via Examin8
    • Affordable plans offering both free and premium content

    Get access to question papers for Class 11 Applied Maths, designed to reflect the current CBSE exam pattern. These question papers are an excellent resource for practicing a variety of questions from previous years. By solving these question papers, you can familiarize yourself with the kind of questions asked in the exam. The question papers available on myCBSEguide App are updated regularly to match the most recent changes in the curriculum. Make sure to download and solve these question papers to build confidence and improve your exam performance.

    Maximize Your Exam Success with the myCBSEguide and Examin8 Apps – Download Now!

    Boost Your Preparation with CBSE Class 11 Applied Maths Sample Papers for 2025
    Prepare for your CBSE Class 11 Applied Maths exams with expertly designed sample papers for 2025.

    Download Class 11 Mock Paper as PDF
    Boost your exam preparation with the myCBSEguide and Examin8 apps! Download these powerful apps today to access a wide range of study materials, practice questions, sample papers, and more. Whether you’re preparing for CBSE Class 10, Class 12, or other exams, these apps provide the tools you need to excel. Stay ahead in your studies with expert-curated content, personalized learning paths, and comprehensive resources. Get started now with myCBSEguide and Examin8 to elevate your exam preparation and increase your chances of success

22. Section B
23. We know that the average of 5 consecutive natural numbers beginning with y i.e. y, y + 1, y + 2, y + 3, y + 4 is y + {tex}\frac {5-1}2{/tex} = y + 2
    {tex}\therefore{/tex} x = y + 2 [The average of n consecutive natural numbers x, x + 1, x + 2, x + 3, …, x + (n – 1) is x +{tex}(\frac {n-1}2){/tex}] If next two numbers i.e. y + 5 and y + 6 are also included, then their average z is given by z = y + {tex}\frac {7-1}2{/tex} = y + 3 [The average of n consecutive natural numbers x, x + 1, x + 2, x + 3, …, x + (n – 1) is x +{tex}(\frac {n-1}2){/tex}] … (ii)
    From (i) and (ii), we obtain z = x + 1 i.e. the mean is increased by 1.
24. In the first and second statements, common codes are 3 and 7, and common words are ‘is’ and ‘eternal’.
    In the first and third statements, the common code is 2 and the common word is ‘truth’.
    So code 2 means ‘truth’
    In the second and third statements, the common code is 9 and the common word is ‘not’.
    So code 8 stands for the word ‘Enmity’.

    OR

    Here, the new positions are obtained by multiplying the original position by 2.
    So,
    
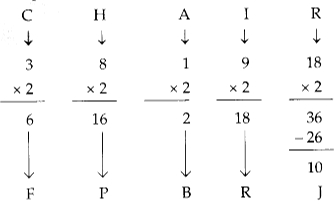
    So, ‘CHAIR’ is coded as ‘FPBRJ’.

25. In 1 hour pipe can fill {tex}\frac{1}{12}{/tex}  of the tank.
    When both the pipes were working together it took 16 hours to fill, so, in 1 hour working together they can fill {tex}\frac{1}{16}{/tex}  of the tank.
    Work done in 1 hour by the pipe which empties the tank = {tex}\frac{1}{12}-\frac{1}{16}=\frac{1}{48}{/tex} of the tank.
    Hence, the waste pipe working alone can empty the tank in 48 hours.
26. Let y = {tex}\frac{\sqrt{x^{2}+1}-x}{\sqrt{x^{2}+1}+x}=\frac{\sqrt{x^{2}+1}-x}{\sqrt{x^{2}+1}+x} \times \frac{\sqrt{x^{2}+1}-x}{\sqrt{x^{2}+1}-x}{/tex}
    {tex}=\frac{\left(x^{2}+1\right)+x^{2}-2 x \sqrt{x^{2}+1}}{\left(x^{2}+1\right)-x^{2}}=\frac{2 x^{2}+1-2 x \sqrt{x^{2}+1}}{1}{/tex}
    = 2x² + 1 – 2x {tex}\sqrt{x^{2}+1}{/tex}, differentiating w.r.t. x, we get
    {tex}\frac{d y}{d x}{/tex} = 2{tex}\cdot{/tex}2x + 0 – 2 [x{tex}\cdot{/tex}{tex}\frac{1}{2}{/tex} {tex}(x^2 + 1)^{-1 \over2}{/tex} {tex}\cdot{/tex} 2x + {tex}\sqrt{x^{2}+1}{/tex} {tex}\cdot{/tex} 1] = 4x – 2 {tex}\left[\frac{x^{2}}{\sqrt{x^{2}+1}}+\sqrt{x^{2}+1}\right]{/tex} = 4x – 2 {tex}\cdot\frac{x^{2}+\left(x^{2}+1\right)}{\sqrt{x^{2}+1}}=2\left[2 x-\frac{2 x^{2}+1}{\sqrt{x^{2}+1}}\right]{/tex}

    OR

    Given (x + y)² = 2axy
    Differentiating the equation on both sides with respect to x,
    {tex}\Rightarrow \frac{d}{d x}{/tex}(x + y)² = {tex}\frac{d}{d x}{/tex}(2axy)
    {tex}\Rightarrow{/tex} 2(x + y){tex}\frac{d}{d x}{/tex}(x + y) = 2a[x {tex}\frac{d y}{d x}{/tex} + y{tex}\frac{d}{d x}{/tex}(x)] {tex}\Rightarrow{/tex} 2(x + y)[1 + {tex}\frac{d y}{d x}{/tex}] = 2a[x{tex}\frac{d y}{d x}{/tex} + y(1)] {tex}\Rightarrow{/tex} 2(x + y)+2(x + y) {tex}\frac{d y}{d x}{/tex} = 2ax{tex}\frac{d y}{d x}{/tex} + 2ay
    {tex}\Rightarrow \frac{d y}{d x}{/tex}[2(x + y) – 2ax] = 2ay – 2(x + y)
    {tex}\Rightarrow \frac{d y}{d x}=\frac{2[a y-x-y]}{2[x+y-a x]}{/tex}
    {tex}\Rightarrow \frac{d y}{d x}=\left(\frac{a y-x-y}{x+y-a x}\right){/tex}

27. (11001.0101)₂ = 1 {tex}\times{/tex} 2⁴ + 1 {tex}\times{/tex} 2³ + 0 {tex}\times{/tex} 2² + 0 {tex}\times{/tex} 2¹ + 1 {tex}\times{/tex} 2⁰ + 0
    {tex}\times{/tex} {tex}\frac {1}{2}{/tex} + 1 {tex}\times{/tex} {tex}\frac {1}{4}{/tex} + 0 {tex}\times{/tex} {tex}\frac {1}{8}{/tex} + 1 {tex}\times{/tex} {tex}\frac {1}{16}{/tex}
    = 16 + 8 + 0 + 0 + 1 + 0 + 0.25 + 0 + 0.0625
    = 25.3125
28. Section C
29. Let A be the first erm and D be the common difference of the given A.P.
    Then, a = pth term
    {tex}\Rightarrow{/tex} a = A + (p – 1) D
    b = qth term
    {tex}\Rightarrow{/tex} b = A + (q – 1) D
    c = rth term
    {tex}\Rightarrow{/tex} c = A + (r – 1)D
    We have.
    a(q – r) + b(r – p) + c(p – q)
    = [A + (p – 1) D](q – r)+[A + (q – 1) D](r – p) + [A + (r – 1) D](p – q)
    = A[(q – r) + (r – p) + (p – q)] + D[(p – 1)(q – r) + (q – 1)(r – p)+(r – 1)(p – q)] = A {tex}\times{/tex} 0 + D[p(q – r) + q(r – p) + r(p – q) – (q – r) – (r – p) -(p – q)] = 0 + D {tex}\times{/tex} 0
    = 0

    OR

    Given a, b, c, d are in G.P., let r be the common ratio.
    {tex}\therefore{/tex} b = ar, c = ar² and, d = ar³
    (bⁿ + cⁿ)² = ((ar)ⁿ + (ar²)ⁿ)² = (anrⁿ + aⁿr²ⁿ)²+ bⁿ) (cⁿ + dⁿ) = (aⁿ + (ar)ⁿ) ((ar²)ⁿ + (ar³)ⁿ)
    = (aⁿ + aⁿrⁿ) (aⁿr²ⁿ + aⁿr³ⁿ)
    = aⁿ (1 + rⁿ) aⁿr²(1 + rⁿ)
    = a²ⁿr²ⁿ (1 + rⁿ)²
    Hence, (bⁿ + cⁿ)2 = (aⁿ + bⁿ) (cⁿ + dⁿ)
    {tex}\Rightarrow{/tex} aⁿ + bⁿ
    = (aⁿrⁿ)² (1 + rⁿ)² = a²ⁿr²ⁿ (1 + rⁿ)2
    and aⁿ , bⁿ + cⁿ, cⁿ + dⁿ are in G.P.

30. Rohit is the husband of Vanshika
    
    Sumita is the sister of Rohit
    
    Anushka is the sister of Vanshika
    
    So Anushka is Rohit’s wife’s sister
    Anushka is the sister-in-law of Rohit.
31. Given f(x) = {tex}\frac{1}{1-x^{2}}{/tex}
    For D_f, f(x) must be a real number {tex}\Rightarrow \frac{1}{1-x^{2}}{/tex} must be a real number
    {tex}\Rightarrow{/tex} 1 – x² {tex}\neq{/tex} 0 {tex}\Rightarrow{/tex} x {tex}\neq{/tex} -1, 1
    {tex}\Rightarrow{/tex} D_f = set of all real numbers except -1, i.e. D_f = R – {-1, 1}
    For R_f, let y = {tex}\frac{1}{1-x^{2}}{/tex}
    {tex}\Rightarrow{/tex} 1 – x² = {tex}\frac{1}{y}{/tex}, y {tex}\neq{/tex} 0 {tex}\Rightarrow{/tex} x² = 1 – {tex}\frac{1}{y}{/tex}, y {tex}\neq{/tex} 0
    But x² {tex}\geq{/tex} 0 for all x {tex}\in{/tex} D_f {tex}\Rightarrow{/tex} 1 – {tex}\frac{1}{y}{/tex} {tex}\geq{/tex} 0 but y² > 0, y {tex}\neq{/tex} 0 (Multiply both sides by y², a positive real number)
    {tex}\Rightarrow{/tex} y² (1 – {tex}\frac{1}{y}{/tex}) {tex}\geq{/tex} 0 {tex}\Rightarrow{/tex} y (y – 1) {tex}\geq{/tex} 0 {tex}\Rightarrow{/tex} (y – 0) (y – 1) {tex}\geq{/tex} 0
    {tex}\Rightarrow{/tex} either y {tex}\leq{/tex} 0 or y {tex}\geq{/tex} 1 but y {tex}\neq{/tex} 0
    {tex}\Rightarrow{/tex} R_f = {tex}(-\infty, 0) \cup[1, \infty){/tex}
32. Here
    Principal =  ₹5000
    Rate {tex}=4 \frac{1}{2}{/tex}% p.a. {tex}=\frac{9}{2}{/tex}% p.a.
    {tex}I=\frac{5000-9 \times 1}{2 \times 100}{/tex}
    I = 25 {tex}\times{/tex} 9
    = ₹ 5225
    Now he transfers the whole amount to fixed deposits
    i.e. P = ₹ 5225
    R = 7%
    T = 7% p.a. compounded annually
    Amount at the third year.
    {tex}A =P\left(1+\frac{R}{100}\right)^n{/tex}
    {tex}=5225\left(1+\frac{7}{100}\right)^{2}{/tex}
    {tex}=5225\left(\frac{107}{100}\right)^{2}{/tex}
    {tex}=5225 \times \frac{107}{100} \times \frac{107}{100}{/tex}
    {tex}=\frac{5225 \times 11449}{10000}{/tex}
    {tex}=\frac{598210{25}}{10000}{/tex} =  ₹ 5982.1025 {tex}\approx{/tex} 5982
33. Here, the volume of water consumed is 48 kL which is above 30 kL.
    Thus, the rate for first 20 kL will be ₹ 5.27/kL, for the next 10 kilolitres thr rate will be ₹ 26.36/kL and for the balance 18 kiloliters, the rate will be ₹ 43.93 / kL.
    {tex}\therefore{/tex} Water consumption charges = ₹( 5.27 {tex}\times{/tex} 20) + ₹ ( 26.36 {tex}\times{/tex}10) + ₹(18 {tex}\times{/tex}43.93)
    = ₹(105.40+263.60+790.74)
    = ₹ 1159.74
    Also, the Sewerage charge is 60% of consumption charges.
    {tex}\therefore{/tex} Sewerage charge = 60% of ₹ 1159.74 = ₹695.84
    According to the given tariff plan, the service charge for the consumption above 30 kL is ₹ 292.82
    {tex}\therefore{/tex} Service charge = ₹ 292.82
    {tex}\therefore{/tex} Total water bill = Water consumption charge + Sewerage charge + Service charge
    = ₹ 1159.74 + ₹695.84 + ₹292.82 = ₹2148.40
    1. (A {tex}\cup{/tex} B) {tex}\cap{/tex} (A {tex}\cap{/tex} B’) = A
       L.H.S. = (A {tex}\cup{/tex} B) {tex}\cap{/tex} (A {tex}\cap{/tex} B’)
       = A {tex}\cup{/tex} (B {tex}\cap{/tex} B’)
       (By distributive law)
       = A {tex}\cup{/tex} {tex}\phi{/tex} (B {tex}\cap{/tex} B’ = {tex}\phi{/tex})
       = A
       = R.H.S. Hence proved.
    2. A – (A {tex}\cap{/tex} B) = A – B
       L.H.S. = A – (A {tex}\cap{/tex} B)
       = A {tex}\cap{/tex} (A {tex}\cap{/tex} B)’
       [{tex}\therefore{/tex} A – B = A {tex}\cap{/tex} B’] = {tex}A \cap\left(A^{\prime} \cup B^{\prime}\right){/tex} (By Demorgan’s law)
       = {tex}\left(A \cap A^{\prime}\right) \cup\left(A \cap B^{\prime}\right){/tex} (By distributive law)
       = {tex}\phi \cup A \cap B^{\prime}\left(\therefore A \cap A^{\prime}=\phi\right){/tex}
       = A {tex}\cap{/tex} B’
       = A – B
       = R.H.S. Hence proved
34.                                                                                                                                                            Section D
    1. Number of ways for units place (1, 3, 5) = 3.
       Ten’s place = 5 (one is occupied in units place),
       Hundred’s place = 4 ways
       Required number of ways = 3 {tex}\times{/tex} 5 {tex}\times{/tex} 4 = 60.
    2. Number of ways for units place = (1, 3, 5) = 3
       Ten’s place = 6, Hundred’s place = 6
       Required number of numbers = 3 {tex}\times{/tex} 6 {tex}\times{/tex} 6 = 108

35. OR

    Number of ways of choosing 3 consonants and 2 vowels = ⁷C₃ {tex}\cdot{/tex}⁴C₂
    Number of ways of arranging 5 letters = 5!.
    Required number of words = ⁷C₃ . ⁴C₂ . 5! = 25200

36. {tex}\mathop {Lim}\limits_{x \to 0} \frac{{{e^{{x^2}}} – \cos x}}{{{x^2}}}{/tex} {tex}= \mathop {Lim}\limits_{x \to 0} \frac{{{e^{{x^2}}} – 1 – \cos x + 1}}{{{x^2}}}{/tex}
    {tex}= \mathop {Lim}\limits_{x \to 0} \frac{{{e^{{x^2}}} – 1}}{{{x^2}}} + \frac{{1 – \cos x}}{{{x^2}}}{/tex}
    {tex}= \mathop {Lim}\limits_{{x^2} \to 0} \left[ {\frac{{{e^{{x^2}}} – 1}}{{{x^2}}}} \right] + \mathop {Lim}\limits_{x \to 0} \left[ {\frac{{2{{\sin }^2}x/2}}{{\frac{{{x^2}}}{4} \times 4}}} \right]{/tex}
    {tex} = 1 + \frac{1}{2}\mathop {Lim}\limits_{\frac{x}{2} \to 0} {\left[ {\frac{{\sin \frac{x}{2}}}{{\frac{x}{2}}}} \right]^2}{/tex}
    {tex} = 1 + \frac{1}{2}(1) = \frac{3}{2}{/tex}

37. Calculation of rank correlation

    Ranks by X xi	Ranks by Y yi	Range of Z zi	di = xi – yi	di‘ = yi – zi	di” = xi – zi	{tex}d_i^2{/tex}	{tex}d_i’^2{/tex}	{tex}d_i”^2{/tex}
    1	3	6	-2	-3	-5	4	9	25
    6	5	4	1	1	2	1	1	4
    5	8	9	-3	-1	-4	9	1	16
    10	4	8	6	-4	2	36	16	4
    3	7	1	-4	6	2	16	36	4
    2	10	2	-8	8	0	64	64	0
    4	2	3	2	-1	1	4	1	1
    9	1	10	8	-9	-1	64	81	1
    7	6	5	1	1	2	1	1	4
    8	9	7	-1	2	1	1	4	1
    Total						{tex}\Sigma d_i^2{/tex} = 200	{tex}\Sigma d_i’^2{/tex} = 214	{tex}\Sigma d_i”^2{/tex} = 60

    Thus, we have n = 10, {tex}\Sigma d_i^2{/tex} = 200, {tex}\Sigma d_i’^2{/tex} = 214 and, {tex}\Sigma d_i”^2{/tex} = 60
    {tex}\therefore{/tex} r(X, Y) = 1 – {tex}\frac{6 \Sigma d_{i}^{2}}{n\left(n^{2}-1\right)}{/tex} = 1 – {tex}\frac{6 \times 200}{10(100-1)}{/tex} = 1 – {tex}\frac{40}{33}=\frac{-7}{33}=\frac{-35}{165}{/tex}
    r(Y, Z) = 1 – {tex}\frac{6 \Sigma d_{i}’^{2}}{n\left(n^{2}-1\right)}{/tex} = 1 – {tex}\frac{6 \times 214}{10(100-1)} = \frac {-49}{165}{/tex}
    and, r(X, Z) = 1 – {tex}\frac{6 \Sigma d_{i}”^{2}}{n\left(n^{2}-1\right)}{/tex} = 1 – {tex}\frac{6 \times 60}{10(100-1)}{/tex} = 1 – {tex}\frac{4}{11}=\frac{7}{11}=\frac{105}{165}{/tex}
    Since r (X, Z) is maximum. Therefore, the pair of judges X and Z has the nearest approach to common likings in music.

    OR

    For the Calculation of Standard Deviation we prepare the following table.

    Class-interval	Frequency (fi)	Mid-values (xi)	ui = {tex}\frac{x_{i}-55}{10}{/tex}	fi ui	{tex}u_{i}^{2}{/tex}	fi {tex}u_{i}^{2}{/tex}
    20-30	3	25	-3	-9	9	27
    30-40	6	35	-2	-12	4	24
    40-50	13	45	-1	-13	1	13
    50-60	15	55	0	0	0	0
    60-70	14	65	1	14	1	14
    70-80	5	75	2	10	4	20
    80-90	4	85	3	12	9	36
    	N = {tex}\sum{/tex} fi = 60			{tex}\sum{/tex} fi ui = 2		{tex}\Sigma f_{i} u_{i}^{2}{/tex} = 134

    Here, N = 60, {tex}\sum{/tex} f_i u_i = 2, {tex}\Sigma f_{i} u_{i}^{2}{/tex} = 134 and h = 10
    {tex}\therefore{/tex} Mean = {tex}\bar{X}{/tex} = A + h {tex}\left(\frac{1}{N} \Sigma f_{i} u_{i}\right){/tex} = 55 + 10 {tex}\left(\frac{2}{60}\right){/tex} = 55.333
    and, Var(X) = h² {tex}\left\{\left(\frac{1}{N} \Sigma f_{i} u_{i}^{2}\right)-\left(\frac{1}{N} \Sigma f_{i} u_{i}\right)^{2}\right\}{/tex} = 100 {tex}\left[\frac{134}{60}-\left(\frac{2}{60}\right)^{2}\right]{/tex} = 222.9
    {tex}\therefore{/tex} S.D. = {tex}\sqrt{\operatorname{Var}(X)}=\sqrt{222.9}{/tex} = 14.94

    1. Income tax according to old rate:
       

       Income	Amount (₹)
       Income from salary	10,00,000
       Form other sources	3,00,000
       Gross income	13,00,000
       Standard deduction	50,000
       Taxable income	12,50,000
       Savings	2,50,000
       Net taxable income	10,00,000
       Income tax:
       2.5 Lakh – 5 Lakh (5%)	12,500
       5.0 Lakh – 10 Lakh (20%)	1,00,000
       Tax Payable	1,12,500
       Education cess: (4%)	4,500
       Total payable tax	1,17,000
       Advance tax paid	60,000
       Net tax Payable	57,000

    2. Income tax according to new rate:
       

       Income	Amount (₹)
       Income from salary	10,00,000
       Form other sources	3,00,000
       Gross income	13,00,000
       Net taxable income	13,00,000
       Income tax:
       2.5 Lakh – 5 Lakh (5%)	12,500
       5.0 Lakh – 7.5 Lakh (10%)	25,000
       7.5 Lakh – 10.0 Lakh (15%)	37,500
       10.0 Lakh – 12.5 Lakh (20%)	50,000
       12.5 Lakh – 13.0 Lakh (25%)	12,500
       Tax Payable	1,37,500
       Education Cess: (4%)	5,500
       Total tax payable	1,43,000
       Advance tax paid	60,000
       Net tax payable	83,000

38.                                                                                                                                                         Section E
    1. Slope of BC = {tex}\frac{-1-2}{4-3}{/tex} = -3
       Equation of BC is y – 2 = -3(x – 3)
       {tex}\Rightarrow{/tex} y – 2 = -3x + 9
       {tex}\Rightarrow{/tex} 3x + y – 11 = 0
    2. A(1, 2), C(4, -1)
       Slope of AC = {tex}\frac{-1-2}{4-1}{/tex} = -1
       Equation of AC is y – 2 = -1(x – 1)
       {tex}\Rightarrow{/tex} y – 2 = -x + 1
       {tex}\Rightarrow{/tex} x + y -3 = 0
    3. A(1, 2), B(3, 2)
       Slope of AB = {tex}\frac{2-2}{3-1}{/tex} = 0
       Equation of AB is y – 2 = 0(x – 1) {tex}\Rightarrow{/tex} y = 2
       OR
       Centroid of triangle = {tex}\left(\frac{1+3+4}{3}, \frac{2+2-1}{3}\right){/tex} = {tex}\left(\frac{8}{3}, 1\right){/tex}
    1. coefficient of variation of wages, of firm A = 0.19
       coefficient of variation of wages, of firm B = {tex}\frac{121}{5253} \times 100{/tex} = 0.21
       {tex}\therefore{/tex} Firm B shows greater variability in individual wages.
    2. Standard deviation, {tex}\sigma=\sqrt{\sigma^{2}}=\sqrt{121}{/tex} = 11
    3. Variance of distribution of wages, {tex}\sigma^2{/tex} = 100
       Standard deviation, {tex}\sigma=\sqrt{\sigma^{2}}{/tex} = {tex}\sqrt{100}{/tex} = 10
       coefficient of Variation = {tex}\frac{\sigma}{\bar{x}} \times 100{/tex}
       = {tex}\frac{10}{5,253} \times 100{/tex}
       = 0.19
       OR
       No. of wage earners = 586
       Mean of monthly wages, {tex}\bar x{/tex} = ₹5253
       Amount paid by firm A = ₹(586 {tex}\times{/tex} 5253) = ₹3078258
    4. Given n = 100, p = 6% = {tex}\frac{6}{100} \Rightarrow \lambda{/tex} = np = 6
       P(X = 0) {tex}=e^{-\lambda}=e^{-6}{/tex}
    5. P(X {tex}\leq{/tex} 4) = P(X = 0) + P(X = 1) + P(X = 2) + P(X = 3) + P(X = 4)
       {tex}=e^{-\lambda}+\frac{\lambda e^{-\lambda}}{1}+\frac{\lambda^2 e^{-\lambda}}{2}+\frac{\lambda^3 e^{-\lambda}}{6}+\frac{\lambda^4 e^{-\lambda}}{24}{/tex}
       {tex}=e^{-\lambda}\left(1+\lambda+\frac{\lambda^2}{2}+\frac{\lambda^3}{6}+\frac{\lambda^4}{24}\right){/tex}
       {tex}=e^{-6}\left(1+6+\frac{36}{2}+\frac{216}{6}+\frac{1296}{24}\right)=115 e^{-6}{/tex}
    6. P(X {tex}\geq{/tex} 2) = 1 – {P(X = 0) + P(X = 1)}
       = 1 {tex}-\left(e^{-\lambda}+\lambda e^{-\lambda}\right)=1-e^{-6}{/tex} (1 + 6)
       = 1 – 7e^-6
       OR
       {tex}P ( X =3)=\frac{6^3 \cdot e^{-6}}{3!}=36 e^{-6}{/tex}                                                         
       Download the myCBSEguide App to Practice More Questions and Excel in Your Exams
       Boost your exam preparation by downloading the myCBSEguide app today! Access a wide range of practice questions, sample papers, and study resources for CBSE Class 10 and Class 12 exams. The CBSE Class 11 Applied Maths sample papers for 2025 are created by expert educators to align with the latest CBSE syllabus and marking scheme With the myCBSEguide app, you can practice more questions, follow the latest CBSE syllabus, and get personalized study material to enhance your learning. Whether it’s solving model papers or reviewing important topics, the myCBSEguide app is the ultimate tool to help you prepare thoroughly and score high in your exams.

MyCBSEguide offers model question papers for Class 11 Applied Maths, tailored to match the CBSE syllabus for 2025. These model question papers are perfect for understanding the structure of your exams and how questions are typically framed. Download CBSE Class 11 Applied Maths sample papers for 2025 to enhance your exam preparation. These sample papers are designed according to the latest CBSE syllabus and marking scheme. Practice regularly to improve problem-solving skills, boost confidence, and excel in your upcoming CBSE exams. Start preparing today! Start practicing with these updated model question papers on myCBSEguide App today.

CBSE Sample Papers for Class 11 2025

• Physics
• Chemistry
• Mathematics
• Biology
• Accountancy
• Economics
• Business Studies
• Computer Science
• Informatics Practices
• English Core
• Hindi Core
• Hindi Elective
• History
• Political Science
• Geography
• Sociology
• Physical Education
• Other Subjects

Why to choose myCBSEGuide?

• Comprehensive Resources: This site offers NCERT solutions, sample papers, chapter notes, and mock tests to cover all CBSE subjects thoroughly.
• Interactive & Convenient Learning: This site features interactive quizzes, video lessons, and a mobile app for studying anytime, anywhere.
• Affordable & Effective: This provides free and premium plans with real-time updates, expert guidance, and proven success in helping students improve their exam performance.