Continuity and Differentiability class 12 Notes Mathematics

Table of Contents

myCBSEguide App

Download the app to get CBSE Sample Papers 2023-24, NCERT Solutions (Revised), Most Important Questions, Previous Year Question Bank, Mock Tests, and Detailed Notes.

Install Now

Continuity and Differentiability Class 12 Notes Mathematics in PDF are available for free download in myCBSEguide mobile app. The best app for CBSE students now provides Continuity and Differentiability class 12 Notes latest chapter wise notes for quick preparation of CBSE board exams and school-based annual examinations. Class 12 Mathematics notes on chapter 5 Continuity and Differentiability are also available for download in CBSE Guide website.

Continuity and Differentiability Class 12 Notes Mathematics

Download CBSE class 12th revision notes for chapter 5 Continuity and Differentiability in PDF format for free. Download revision notes for Continuity and Differentiability class 12 Notes and score high in exams. These are the Continuity and Differentiability class 12 Notes prepared by team of expert teachers. The revision notes help you revise the whole chapter 5 in minutes. Revision notes in exam days is one of the best tips recommended by teachers during exam days.

Download Revision Notes as PDF

CBSE Class 12 Mathematics Chapter 5
Continuity and Differentiability

Continuity of function at a point: Geometrically we say that a function $Continuity and Differentiability class 12 Notes Mathematics$ is continuous at $Continuity and Differentiability class 12 Notes Mathematics$ if the graph of the function $Continuity and Differentiability class 12 Notes Mathematics$ is continuous (without any break) at $Continuity and Differentiability class 12 Notes Mathematics$ .
A funciton $Continuity and Differentiability class 12 Notes Mathematics$ is said to be continuous at a point $x = a$ if:

(i) $Continuity and Differentiability class 12 Notes Mathematics$ exists i.e., $Continuity and Differentiability class 12 Notes Mathematics$ is finite, definite and real.

(ii) $\mathop {\lim }\limits_{x \to a} f\left( x \right)$ exists.

(iii) $\mathop {\lim }\limits_{x \to a} f\left( x \right) = f\left( a \right)$

A function $f\left( x \right)$ is continuous at $x = a$ if $Continuity and Differentiability class 12 Notes Mathematics$ where $h \to 0$ through positive values.
Continuity of a function in a closed interval: A function $f\left( x \right)$ is said to be continuous in the closed interval if it is continuous for every value of $x$ lying between a and b continuous to the right of a and to the left of $x = b$ i.e., $Continuity and Differentiability class 12 Notes Mathematics$ and $\mathop {\lim }\limits_{x \to b - 0} f\left( x \right) = f\left( b \right)$
Continuity of a function in a open interval: A function $f\left( x \right)$ is said to be continuous in an open interval $\left( {a,b} \right)$ if it is continuous at every point in $\left( {a,b} \right)$ .
Discontinuity (Discontinuous function): A function $f\left( x \right)$ is said to be discontinuous in an interval if it is discontinuous even at a single point of the interval.
Suppose $f$ is a real function and $c$ is a point in its domain. The derivative of $f$ at $c$ is defined by $Continuity and Differentiability class 12 Notes Mathematics$ provided this limit exists.
A real valued function is continuous at a point in its domain if the limit of the function at that point equals the value of the function at that point. A function is continuous if it is continuous on the whole of its domain.
${{dy} \over {dx}}$ is derivative of first order and is also denoted by $y'$ or $y_1$ .
Sum, difference, product and quotient of continuous functions are continuous. i.e., if f and g are continuous functions, then is continuous. (f . g) (x) = f (x) . g(x) is continuous.

(wherever g (x) ≠ 0) is continuous.

Every differentiable function is continuous, but the converse is not true.
Chain rule is rule to differentiate composites of functions. If f = v o u, t = u (x) and if both 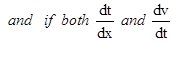exist then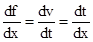
Following are some of the standard derivatives (in appropriate domains):
$\left( {u \pm v} \right)' = u' \pm v'$
$\left( {uv} \right)' = u'v + uv'$ [Product Rule]
$\left( {{u \over v}} \right)' = {{u'v - uv'} \over {{v^2}}}$ , wherever $v \ne 0$ [Quotient Rule]
If $y = f\left( u \right);u = g\left( x \right),$ then $Continuity and Differentiability class 12 Notes Mathematics$ [Chain Rule]
If $x = f\left( t \right);y = g\left( t \right)$ , then ${{dy} \over {dx}} = {{dy} \over {dt}} \div {{dx} \over {dt}}$ [Parametric Form]
${d \over {dx}}\left( {{x^n}} \right) = n{x^{n - 1}}$
${d \over {dx}}\left( {\sin x} \right) = \cos x$
${d \over {dx}}\left( {\cos x} \right) = - \sin x$
$Continuity and Differentiability class 12 Notes Mathematics$
${d \over {dx}}\left( {\cot x} \right) = - \cos e{c^2}x$
${d \over {dx}}\left( {\sec x} \right) = \sec x.\tan x$
${d \over {dx}}\left( {\cos ec x} \right) = - \cos ec x.\cot x.$
${d \over {dx}}\left( {{a^x}} \right) = {a^x}.{\log _e}a$
${d \over {dx}}\left( {{{\log }_e}x} \right) = {1 \over x}$
${d \over {dx}}\left( {{{\cos }^{ - 1}}x} \right) = {{ - 1} \over {1 + {x^2}}}$
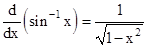
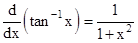
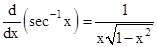
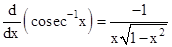
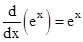
Logarithmic differentiation is a powerful technique to differentiate functions of the form 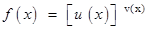Here both f (x) and u (x) need to be positive for this technique to make sense.
If we have to differentiate logarithmic funcitons, other than base $e$ , then we use the result ${\log _b}a = {{{{\log }_e}a} \over {{{\log }_e}b}}$ and then differentiate R.H.S.
While differentiating inverse trigonometric functions, first represent it in simplest form by using suitable substitution and then differentiate simplified form.
If we are given implicit functions then differentiate directly w.r.t. suitable variable involved and get the derivative by readusting the terms.
${{{d^2}y} \over {d{x^2}}} = {d \over {dx}}\left( {{{dy} \over {dx}}} \right)$ is derivative of second order and is denoted by $y''$ or $y_2$ .
Rolle’s Theorem: If f: [a, b] → R is continuous on [a, b] and differentiable on (a, b) such that f (a) = f (b), then there exists some c in (a, b) such that f ′(c) = 0.
Lagrange’s Mean Value Theorem: If f: [a, b] → R is continuous on [a, b] and differentiable on (a, b). Then there exists some c in (a, b) such that

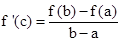
CBSE Class 12 Revision Notes and Key Points

Continuity and Differentiability class 12 Notes Mathematics. CBSE quick revision note for class-12 Chemistry Physics Math’s, Biology and other subject are very helpful to revise the whole syllabus during exam days. The revision notes covers all important formulas and concepts given in the chapter. Even if you wish to have an overview of a chapter, quick revision notes are here to do if for you. These notes will certainly save your time during stressful exam days.

To download Continuity and Differentiability class 12 Notes, sample paper for class 12 Physics, Chemistry, Biology, History, Political Science, Economics, Geography, Computer Science, Home Science, Accountancy, Business Studies and Home Science; do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise pract, ce, NCERT Continuity and Differentiability, NCERT Exemplar Continuity and Differentiability, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.

Test Generator

Create question paper PDF and online tests with your own name & logo in minutes.

Create Now

myCBSEguide

Question Bank, Mock Tests, Exam Papers, NCERT Solutions, Sample Papers, Notes

Install Now

Continuity and Differentiability class 12 Notes Mathematics

myCBSEguide App

Continuity and Differentiability Class 12 Notes Mathematics

CBSE Class 12 Mathematics Chapter 5
Continuity and Differentiability

CBSE Class 12 Revision Notes and Key Points

Related Posts

1 thought on “Continuity and Differentiability class 12 Notes Mathematics”

Leave a Comment

myCBSEguide App

Continuity and Differentiability Class 12 Notes Mathematics

CBSE Class 12 Mathematics Chapter 5 Continuity and Differentiability

CBSE Class 12 Revision Notes and Key Points

Related Posts

1 thought on “Continuity and Differentiability class 12 Notes Mathematics”

Leave a Comment

CBSE Class 12 Mathematics Chapter 5
Continuity and Differentiability