
myCBSEguide App
Download the app to get CBSE Sample Papers 2025-26, NCERT Solutions (Revised), Most Important Questions, Previous Year Question Bank, Mock Tests, and Detailed Notes.
Install NowIntegrals Class 12 Notes Mathematics in PDF are available for free download in myCBSEguide mobile app. The best app for CBSE students now provides Integrals class 12 Notes latest chapter wise notes for quick preparation of CBSE board exams and school-based annual examinations. Class 12 Mathematics notes on chapter 7 Integrals are also available for download in CBSE Guide website.
Integrals Class 12 Notes Mathematics
Download CBSE class 12th revision notes for chapter 7 Integrals in PDF format for free. Download revision notes for Integrals class 12 Notes and score high in exams. These are the Integrals class 12 Notes prepared by team of expert teachers. The revision notes help you revise the whole chapter 7 in minutes. Revision notes in exam days is one of the best tips recommended by teachers during exam days.
Download Revision Notes as PDF
CBSE Class 12 Mathematics Revision Notes Chapter 7 Integrals
- Integration is the inverse process of differentiation. In the differential calculus, we are given a function and we have to find the derivative or differential of this function, but in the integral calculus, we are to find a function whose differential is given. Thus, integration is a process which is the inverse of differentiation. Let.
 Then we write.
Then we write. These integrals are called indefinite integrals or general integrals, C is called constant of integration. All these integrals differ by a constant.
These integrals are called indefinite integrals or general integrals, C is called constant of integration. All these integrals differ by a constant. - From the geometric point of view, an indefinite integral is collection of family of curves, each of which is obtained by translating one of the curves parallel to itself upwards or downwards along the y-axis.
- Some properties of indefinite integrals are as follows:
1.
2. For any real number k, 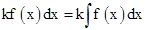
More generally, if 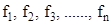 are functions and
are functions and 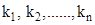 are real numbers. Then
are real numbers. Then
![]()
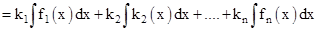
- Some standard integrals:
(i)  Particularly,
Particularly, 
(ii) 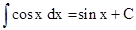
(iii) 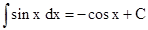
(iv) 
(v) 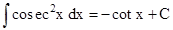
(vi) 
(vii) 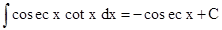
(viii) 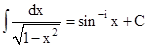
(ix) 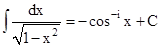
(x)
(xi)
(xii) 
(xiii) 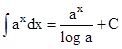
(xiv) 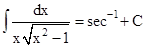
(xv) 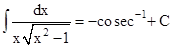
(xvi) 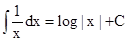
- Integration by Partial Fraction: A rational fraction is the ratio of the form where P(x) and Q(x) are polynomials in x and Q(x) 0. If the degree of the polynomial P(x) is greater than the degree of the polynomial Q(x), then we may divide P(x) by Q(x) so that where T(x) is a polynomial in x and degree of P1(x) is less than Q(x). T(x) is a polynomial can be easily integrated.
can be integrated by expressing as the sum of partial fractions of the following types:
(a)
(b)
(c)
(d)
(e) where cannot be factorize further into linear fraction.
- Integration by Substitution: In this method we change the variable to some other variable. When the integrand involves some trigonometric functions, we shall be using some well-known identities to find the integrals. Using substitution technique, we obtain the following standard integrals:
(a)
(b)
(c)
(d)
- Integrals of Some Special Functions:
(a) (b)
(c) (d)
(e) (f)
- Integration by Parts: For the given function f(x) and g(x),
We must take proper care to choose the first function and second function clearly. We must choose that function as the second function whose integral is well-known to us.
- Some Special Type of Integrals:
(a)
(b)
(c)
- Integrals of the types or
(a) These type of integrals are transformed into standard form by expressing
(b) Integrals of the types or are transformed into standard form by expressing
where A abd B are determined by comparing coefficients on both sides.
- We have already defined as the area of the region bounded by the curve , the x-axis and the ordinates x = a and x = b. Let x be a given point in [a, b], then represents the area function A(x).
- First Fundamental Theorem of Integral Calculus: Let the area function be defined by for all where the function f is assumed to be continuous on [a, b], then for all .
- Secind Fundamental Theorem of Integral Calculus: Let f be a continuous function of x defined on the closed interval ]a, b] and let F be another function such that for all x in the domain of f, then
This is called the definite integral of f over the range [a, b] were a and b are called the limits of integration, being the lower limit and the upper limit.
CBSE Class CBSE Revision notes (PDF Download) Free
CBSE Revision notes for Class 12 Mathematics PDF
CBSE Revision notes Class 12 Mathematics – CBSE
CBSE Revisions notes and Key Points Class 12 Mathematics
Summary of the NCERT books all chapters in Mathematics class 12
Short notes for CBSE class 12th Mathematics
Key notes and chapter summary of Mathematics class 12
Quick revision notes for CBSE board exams
12 Revision Notes and Key Points
Integrals class 12 Notes Mathematics. CBSE quick revision note for class-12 Chemistry Physics Math’s, Biology and other subject are very helpful to revise the whole syllabus during exam days. The revision notes covers all important formulas and concepts given in the chapter. Even if you wish to have an overview of a chapter, quick revision notes are here to do if for you. These notes will certainly save your time during stressful exam days.
- Revision Notes for class-12 Physics
- Revision Notes for class-12 Chemistry
- Revision Notes for class-12 Mathematics
- Revision Notes for class-12 Biology
- Revision Notes for class-12 Accountancy
- Revision Notes for class-12 Economics
- Revision Notes for class-12 Business Studies
- Revision Notes for class-12 Computer Science
- Revision Notes for class-12 Informatics Practices
- Revision Notes for class-12 English Core
- Revision Notes for class-12 History
- Revision Notes for class-12 Physical Education
To download Integrals class 12 Notes Mathematics, sample paper for class 12 Physics, Chemistry, Biology, History, Political Science, Economics, Geography, Computer Science, Home Science, Accountancy, Business Studies and Home Science; do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT Integrals, NCERT Exemplar Integrals, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.
- Relations and Functions class 12 Notes Mathematics
- Inverse Trigonometric Functions class 12 Notes Mathematics
- Matrices class 12 Notes Mathematics
- Determinants class 12 Notes Mathematics
- Continuity and Differentiability class 12 Notes Mathematics
- Application of Derivatives class 12 Notes Mathematics
- Application of Integrals class 12 Notes Mathematics
- Differential Equations class 12 Notes Mathematics
- Vector Algebra class 12 Notes Mathematics
- Three Dimensional Geometry class 12 Notes Mathematics
- Linear Programming class 12 Notes Mathematics
- Probability class 12 Notes Mathematics

Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
Learn8 App
Practice unlimited questions for Entrance tests & government job exams at ₹99 only
Install Now