Probability Class 12 Notes Mathematics

Table of Contents

myCBSEguide App

Download the app to get CBSE Sample Papers 2023-24, NCERT Solutions (Revised), Most Important Questions, Previous Year Question Bank, Mock Tests, and Detailed Notes.

Install Now

Probability Class 12 Notes Mathematics in PDF are available for free download in myCBSEguide mobile app. The best app for CBSE students now provides Probability class 12 Notes latest chapter wise notes for quick preparation of CBSE board exams and school-based annual examinations. Class 12 Mathematics notes on chapter 13 Probability are also available for download in CBSE Guide website.

CBSE Guide Probability class 12 Notes Mathematics

CBSE guide notes are the comprehensive notes which covers the latest syllabus of CBSE and NCERT. It includes all the topics given in NCERT class 12 Mathematics textbook. Users can download CBSE guide quick revision notes from myCBSEguide mobile app and my CBSE guide website.

Class 12 Mathematics notes Chapter 13 Probability

Download CBSE class 12th revision notes for chapter 13 Probability in PDF format for free. Download revision notes for Probability class 12 Notes and score high in exams. These are the Probability class 12 Notes prepared by team of expert teachers. The revision notes help you revise the whole chapter 13 in minutes. Revision notes in exam days is one of the best tips recommended by teachers during exam days.

Download Revision Notes as PDF

CBSE Class 12 Mathematics Chapter 13 Probability

Sample Space: The set of all possible outcomes of a random experiment. It is denoted by the symbol S.
Sample points: Elements of the sample space.
Event: A subset of the sample space.
Impossible Event: The empty set.
Sure Event: The whole sample space.
Complementary event or “not event”: The set “S” or S – A.
The event A or B: The set A $\cup$ B.
The event A and B: The set A $\cap$ B.
The event A but not B: A – B.
Mutually exclusive events: A and B are mutually exclusive if A $\cap$ B = $\phi$ .
Exhaustive and Mutually exclusive events: Events E₁, E₂,…….., E_n are mutually exclusive and exhaustive if E₁ $\cup$ E₂ $\cup$ ……. $\cup$ E_n = S and E_i $\cap$ E_j = $\phi$ for all $i \ne j$ .
Exiomatic approach to probability: To assign probabilities to various events, some axioms or rules have been described.

Let S be the sample space of a random experiment. The probability P is a real values function whose domain is the power set of S and range is the interval [0, 1] satisfying the following axioms:

(a) For any event E, P(E) $\ge$ 0

(b) P(S) = 1

If E₁, E₂, E₃………… are n mutually exclusive events, then ${\rm{P}}\left( {\mathop \cup \limits_{i = 1}^n {{\rm{E}}_i}} \right) = \sum\limits_{i = 1}^n {{\rm{P}}\left( {{{\rm{E}}_i}} \right)}$

Probability of an event in terms of the probabilities of the same points (outcomes): Let S be the sample space containing n exhaustive outcomes ${{\rm{W}}_1},{{\rm{W}}_2},{{\rm{W}}_3},......{{\rm{W}}_n}$ i.e., S = $\left( {{{\rm{W}}_1},{{\rm{W}}_2},{{\rm{W}}_3},......{{\rm{W}}_n}} \right)$

Now from the axiomatic definition of the probability:

(a) 0 $\le$ P(W_i) $\le$ 1, for each ${{\rm{W}}_i} \in {\rm{S}}$ .

(b) P(W₁) + P(W₂) + …….+ P(W₃) = P(S) = 1

Equally likely outcomes: All outcomes with equal probability.
Classical definition of the probability of an event: For a finite sample space with equally likely outcome, probability of an event A.

P(A) = ${{n\left( {\rm{A}} \right)} \over {n\left( {\rm{S}} \right)}}$

where $n\left( {\rm{A}} \right)$ = Number of elements in the set A. and $n\left( {\rm{S}} \right)$ = Number of elements in set S.

If A is any event, then P(not A) = 1 – P(A) $\Rightarrow$ ${\rm{P}}\left( {\overline {\rm{A}} } \right) = 1 - {\rm{P}}\left( {\rm{A}} \right)$ $\Rightarrow$ ${\rm{P}}\left( {{\rm{A'}}} \right) = 1 - {\rm{P}}\left( {\rm{A}} \right)$
The conditional probability of an event E, given the occurrence of the event F is given by
·
·
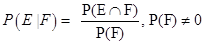
Theorem of total probability:

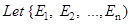be a partition of a sample space and suppose that each of 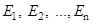has non zero probability. Let A be any event associated with S, then

Bayes’ theorem: If 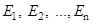are events which constitute a partition of sample space S, i.e. 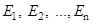are pairwise disjoint and 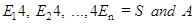be any event with non-zero probability, then,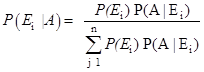
Random variable: A random variable is a real valued function whose domain is the sample space of a random experiment.
Probability distribution: The probability distribution of a random variable X is the system of numbers

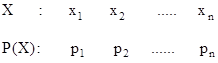
Where,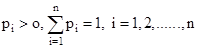

Mean of a probability distribution: Let X be a random variable whose possible values occur with probabilities respectively. The mean of X, denoted by 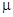is the number 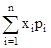. The mean of a random variable X is also called the expectation of X, denoted by E (X).
Variance: Let X be a random variable whose possible values occur with probabilities 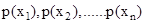respectively. Let be the mean of X. The variance of X, denoted by Var (X) or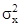 is defined as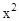 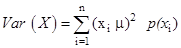or equivalently 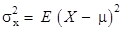. The non-negative number, 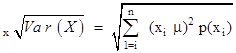is called the standard deviation of the random variable X.

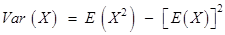
Bernoulli Trials: Trials of a random experiment are called Bernoulli trials, if they satisfy the following conditions:

(i) There should be a finite number of trials.

(ii) The trials should be independent.

(iii) Each trial has exactly two outcomes: success or failure.

(iv) The probability of success remains the same in each trial.

For Binomial distribution

Probability class 12 Notes

CBSE Revision notes (PDF Download) Free
CBSE Revision notes for Class 12 Mathematics PDF
CBSE Revision notes Class 12 Mathematics – CBSE
CBSE Revisions notes and Key Points Class 12 Mathematics
Summary of the NCERT books all chapters in Mathematics class 12
Short notes for CBSE class 12th Mathematics
Key notes and chapter summary of Mathematics class 12
Quick revision notes for CBSE board exams

CBSE Class-12 Revision Notes and Key Points

Probability class 12 Notes Mathematics. CBSE quick revision note for class-12 Chemistry Physics Math’s, Biology and other subject are very helpful to revise the whole syllabus during exam days. The revision notes covers all important formulas and concepts given in the chapter. Even if you wish to have an overview of a chapter, quick revision notes are here to do if for you. These notes will certainly save your time during stressful exam days.

To download Probability class 12 Notes Mathematics, sample paper for class 12 Physics, Chemistry, Biology, History, Political Science, Economics, Geography, Computer Science, Home Science, Accountancy, Business Studies and Home Science; do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT Probability, NCERT Exemplar Probability, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.

Test Generator

Create question paper PDF and online tests with your own name & logo in minutes.

Create Now

myCBSEguide

Question Bank, Mock Tests, Exam Papers, NCERT Solutions, Sample Papers, Notes