CBSE Class 12 Physics Chapter 2 Extra Questions

myCBSEguide App

Download the app to get CBSE Sample Papers 2023-24, NCERT Solutions (Revised), Most Important Questions, Previous Year Question Bank, Mock Tests, and Detailed Notes.

Install Now

CBSE Class 12 Physics Chapter 2 Extra Questions. We know Physics is tough subject within the consortium of science subjects physics is an important subject. But if you want to make career in these fields like IT Consultant, Lab Technician, Laser Engineer, Optical Engineer etc. You need to have strong fundamentals in physics to crack the exam. myCBSEguide has just released Chapter Wise Question Answers for class 12 Physics. There chapter wise Extra Questions with complete solutions are available for download in myCBSEguide website and mobile app. These Questions with solution are prepared by our team of expert teachers who are teaching grade in CBSE schools for years. There are around 4-5 set of solved Physics Extra Questions from each and every chapter. The students will not miss any concept in these Chapter wise question that are specially designed to tackle Board Exam. We have taken care of every single concept given in CBSE Class 12 Physics syllabus and questions are framed as per the latest marking scheme and blue print issued by CBSE for class 12.

Class 12 Physics Extra Questions

Download as PDF

Class – 12 Physics (Electrostatics Potential and Capacitance)
Answers

1. {tex}\epsilon_0\frac{A}{d}{/tex}Explanation: The capacitance of a parallel plate capacitor is given by {tex}C = \frac{{{\varepsilon _0}A}}{d}{/tex}
1. {tex}{3V \over 5}{/tex}Explanation: The charges on the capacitors after being charged to a potential V are Q1 = CV; Q2 = 2CV.
  After being filled with a material of dielectric K = 3 the capacitor which initially had a capacitance C has now the capacitance KC = 3C. The common potential
  {tex}{V_1} = \frac{{{\text{Total charge}}}}{{{\text{Total capacitance}}}} = \frac{{{Q_1} + {Q_2}}}{{3C + 2C}}{/tex}
  {tex} = \frac{{CV + 2CV}}{{5C}} = \frac{3}{5}V{/tex}
1. Decreasing the battery potential
  Explanation: An electroscope is a device which measures the potential difference. If it is connected in parallel to the capacitor, the potential across it will be equal to the potential across the capacitor, which is equal to the potential across the battery. On decreasing the battery potential, the potential difference across the electroscope reduces and hence the reading reduces. While the capacitor is connected to the battery, Placing a dielectric between the plates, or decreasing the distance between the plates or increasing the area of the plates will not change the potential difference across it; since it will always remain equal to the potential difference maintained by the battery. In the cases B, C and D, The capacitance of the capacitor, however increases; but this increase happens due to increase in the charge stored in the capacitor while the potential remains constant.
1. infinite
  Explanation: Capacitor does not allow DC to pass through it. The effective capacitance or the capacitive reactance {tex}{X_C} = \frac{1}{{C\omega }}{/tex},
  where ω is the frequency of voltage source.
  Since DC current is a constant current, its frequency is zero.
  The capacitive reactance is therefore infinity.
1. voltage
  Explanation: Voltage is an electrical potential difference, the difference in electric potential between two places. The unit for electrical potential difference, or voltage, is the volt.
The equipotential surfaces produced by a single point charge are spherically symmetrical surfaces which are concentric.
In concentric spheres which are as shown below in the figure, the lines of force point radially outwards, so they are perpendicular to the equipotential surfaces at all points.
If we place another unit positive charge at any point on these surfaces, that will repelled along the the direction of the electric field intensity i.e. radially outwards to the surfaces.
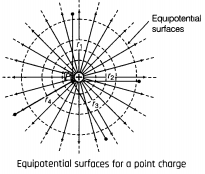
Equipotential surfaces due to a single point charge are concentric sphere having charge at the centre. Electric field lines are always normally outwards from the spherical surfaces.
Given, {tex}q _ { A } = 2 \mu C = 2 \times 10 ^ { – 6 } C{/tex}
{tex}q _ { g } = – 2 \mu C = – 2 \times 10 ^ { – 6 } C{/tex} and r = 5 cm

As, potential at point O is zero.
{tex}V = \frac { 2 \times 10 ^ { – 6 } } { 4 \pi \varepsilon _ { 0 } x \times 10 ^ { – 2 } } + \frac { – 2 \times 10 ^ { – 6 } } { 4 \pi \varepsilon _ { 0 } ( 5 – x ) \times 10 ^ { – 2 } } = 0{/tex}
or {tex}\frac { 2 \times 10 ^ { – 6 } } { 4 \pi \varepsilon _ { 0 } x \times 10 ^ { – 2 } } = \frac { 2 \times 10 ^ { – 6 } } { 4 \pi \varepsilon _ { 0 } ( 5 – x ) \times 10 ^ { – 2 } }{/tex}
or x = 5 – x {tex}\Rightarrow{/tex} x = 2.5 cm
Hence equipotential surface is a plane normal to the axis of the dipole and lies at the mid point of the axis.
{tex}\left[ {{M^{ – 1}}{L^{ – 2}}{A^2}{T^4}} \right]{/tex}
Initially, when there is vacuum between the two plates of the capacitor, then capacitance of the capacitor becomes {tex}C _ { 0 } = \frac { \varepsilon _ { 0 } A } { d }{/tex},
where, A is the area of each plate of the capacitor.
Suppose that the capacitor is connected to a battery, an electric field E₀ is produced.
Now, if we insert the dielectric slab of dielectric constant K and of thickness t = d/2, the electric field reduces to E = E₀/K.
Now, the gap between plates is divided in two parts, for distance t, there is electric field E and for the remaining distance (d – t) the electric field is E₀.
If V be the potential difference between the plates of the capacitor after inserting the dielectric slab, then V = Et + E₀(d – t)
{tex}\Rightarrow V = \frac { E d } { 2 } + \frac { E _ { 0 } d } { 2 } = \frac { d } { 2 } \left( E + E _ { 0 } \right) \quad \left[ \because t = \frac { d } { 2 } \right]{/tex}
{tex}\Rightarrow \quad V = \frac { d } { 2 } \left( \frac { E _ { 0 } } { K } + E _ { 0 } \right){/tex}
{tex}= \frac { d E _ { 0 } } { 2 K } ( K + 1 ){/tex} {tex}\left[ \text { As, } \frac { E _ { 0 } } { E } = K \right]{/tex}
Now, {tex}E _ { 0 } = \frac { \sigma } { \varepsilon _ { 0 } } = \frac { q } { \varepsilon _ { 0 } A } {/tex}, Hence the potential becomes {tex} V = \frac { d } { 2 K } \cdot \frac { q } { \varepsilon _ { 0 } A } ( K + 1 ){/tex}
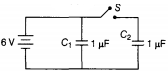
Again we know that, {tex}C = \frac { q } { V } = \frac { 2 K \varepsilon _ { 0 } A } { d ( K + 1 ) }{/tex}(substituting the value of V)
1. According to the diagram, when the switch S is closed, the two capacitors C₁ and C₂ in parallel will be charged by the same potential difference V.
  
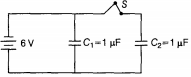
  So, charge on capacitor C₁{tex}q _ { 1 } = C _ { 1 } V = 1 \times 6 = 6 \mu \mathrm { C }{/tex} ….(i)
  and charge on capacitor C₂
  {tex}q _ { 2 } = C _ { 2 } V = 1 \times 6 = 6 \mu C{/tex} …(ii)
  Hence total charge, q = q₁ + q₂ = 6 + 6 = 12µC (before the switch S is closed) Now when S is left open, there will be no change in the stored charge value of C₂ and the change in charge value in C₁ is given in the answer (ii).
2. When switch S is opened and dielectric is introduced. Then
  
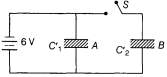
  Capacity of both the capacitors becomes K times
  i.e. {tex}C’ _ { 1 } = C _ { 2 } ^ { \prime } = K C = 3 \times 1 = 3 \mu F \left( \operatorname { as } C _ { 1 } ^ { \prime } = C’ _ { 2 } \right){/tex}
  Capacitor A remains connected to battery
  {tex}\therefore \quad V _ { 1 } ^ { \prime } = V = 6 \mathrm { V }{/tex}
  {tex}q _ { 1 } ^ { \prime } = K q _ { 1 } = 3 \times 6 \mu C = 18 \mu C{/tex} (charge in C₁ is increased to 3 times of the previous value)
  Capacitor B becomes isolated, charge in it will remain same
  {tex}\therefore q’ _ { 2 } = q _ { 2 } ~{ \Rightarrow } ~C’ _ { 2 } V’ _ { 2 } = C _ { 2 } V _ { 2 }{/tex} or {tex}( K C ) V’ _ { 2 } = C V{/tex} (C₂ = C, C’₂ = KC)
  or {tex}V _ { 2 } = \left( \frac { V } { K } \right) = \frac { 6 } { 3 } = 2 V{/tex} (potential difference of the capacitor B is reduced to a factor of 3)
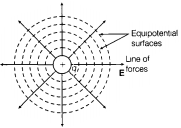
On the surface E = constant, V = constant. Inside the surface, E = 0, V = constant = potential on surface. Outside the balloon.
{tex}E \propto \frac{1}{{{r^2}}}{/tex} and {tex}V \propto \frac{1}{r}{/tex}
where r is the distance of the point from the centre of the balloon.
Initial energy stored in {tex}5\mu F{/tex} capacitor,
{tex}{U_i} = \frac{1}{2}C{V^2} = \frac{1}{2} \times 5 \times {10^{ – 6}} \times {(100)^2}{/tex}
{tex} = 2.5 \times {10^{ – 2}}J{/tex}
Charge on 5 mF capacitor {tex} = 5 \times {10^{ – 6}} \times 100{/tex}
{tex} = 5 \times {10^{ – 4}}C\left[ {\because q = CV} \right]{/tex}
The two capacitors attain a common potential V when they are connected together.
{tex}V = \frac{{Total\;ch\arg e}}{{total\;capacitor}} = \frac{{5 \times {{10}^{ – 4}}}}{{(5 + 3) \times {{10}^{ – 6}}}} = \frac{{125}}{2}V{/tex}
Final energy of the combination,
{tex}{U_f} = \frac{1}{2} \times (5 + 3) \times {10^{ – 6}} \times {\left( {\frac{{125}}{2}} \right)^2} = 1.56 \times {10^{ – 2}}J{/tex}
Electrostatic energy lost in the process of attaining the steady state
{tex} = {U_i} – {U_f} = (2.5 – 1.56) \times {10^{ – 2}}{/tex}
{tex} = 0.94 \times {10^{ – 2}}J{/tex}
1. Parallel plate capacitor consists of two thin conducting plates each of area A held parallel to each other at a suitable distance d. One of the plates is insulated and other is earthed. Say, there is vacuum or air between the plates. Structure of a parallel plate capacitor is shown below.
  
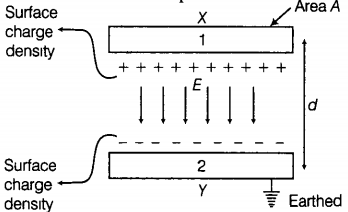
  Suppose, the plate X is given a charge of +q coulomb. By induction, -q coulomb of charge is produced on the inner surface of the plate Y and +q coulomb on the outer surface. Since, the plate Y is connected to the earth, hence the relatively weak charge +q residing far away i.e. on the outer surface flows to the earth. Thus, the plates X and Y have equal and opposite charges +q and -q respectively
  Suppose, the surface density of charge on each plate is {tex}\sigma{/tex}, We know that the intensity of electric field at a point between two plane parallel sheets of equal and opposite charges is = {tex}\frac {\sigma }{2 \epsilon _0} -(-\frac {\sigma }{2\epsilon _0}) ={/tex} {tex}\sigma/\varepsilon_0{/tex} [{tex}\frac {+ \sigma}{2\epsilon _0} ~and ~ \frac {- \sigma}{2 \epsilon_0}{/tex} are electric field intensities in between the two plates due to charges +q and -q on the two plates of the capacitor respectively], where {tex}\varepsilon_0{/tex} is the permittivity of free space. The intensity of electric field between the plates will be given by, {tex}E = \frac { \sigma } { \varepsilon _ { 0 } }{/tex}
  The charge on each plate is q and the area of each plate is A. Thus,
  {tex}\sigma = \frac { q } { A } \text { and } E = \frac { q } { \varepsilon _ { 0 } A }{/tex}…………(i)
  Now, let the potential difference between the two plates be V volt. Then, the electric field between the plates is given by
  {tex}E = \frac { V } { d } \quad \text { or } \quad V = E d{/tex}…..(ii)
  Substituting the value of E from equation (i) into equation (ii), we get
  {tex}V = \frac { q d } { \varepsilon _ { 0 } A }{/tex}
  Now capacitance of the parallel plate capacitor is
  {tex}C = \frac { q } { V } = \frac { q } { q d / \varepsilon _ { 0 } A } \quad \text { or } \quad C = \frac { \varepsilon _ { 0 } A } { d }{/tex}
  Where, {tex}\varepsilon _ { 0 } = 8.85 \times 10 ^ { – 12 } \mathrm { C } ^ { 2 } – \mathrm { Nm } ^ { – 2 }{/tex} is the permittivity of vacuum or air.
2. Surface charge density of a spherically charged body is given by
  {tex}\sigma = \frac { q } { 4 \pi R ^ { 2 } }{/tex}
  After connecting both the conductors, their potentials will become equal.
  {tex}\Rightarrow \frac { K q _ { 1 } } { R _ { \mathrm { l } } } = \frac { K q _ { 2 } } { R _ { 2 } }{/tex} [ For a spherically charged conductor with charge q potential is given by, {tex}V = \frac { 1 } { 4 \pi \varepsilon _ { 0 } } \frac { q } { R } \text { or } V = \frac { K q } { R }{/tex}] {tex}\therefore \frac { q _ { 1 } } { q _ { 2 } } = \frac { R _ { 1 } } { R _ { 2 } } ~Hence~ \frac { \sigma _ { 1 } } { \sigma _ { 2 } } = \frac { q _ { 1 } / 4 \pi R _ { 1 } ^ { 2 } } { q _ { 2 } / 4 \pi R _ { 2 } ^ { 2 } }{/tex}
  {tex}= \frac { q _ { 1 } } { q _ { 2 } } \left( \frac { R _ { 2 } } { R _ { 1 } } \right) ^ { 2 } = \frac {R_1}{R_2}× \left (\frac {R_2}{R_1} \right)^{2}= \frac { R _ { 2 } } { R _ { 1 } }{/tex}
1. 1. When a conductor is placed in an external electric field, the free charges present inside the conductor redistribute themselves in such a manner that the electric field due to induced charges opposes the external field within the conductor. This happens until a static situation is achieved, i.e. when the two fields cancels each other, then the net electrostatic field in the conductor becomes zero.
  2. Dielectrics are non-conducting substances. i.e. they have no charge carriers. Thus, in a dielectric, free movement of charges is not possible. When a dielectric is placed in an external electric field, the molecules are re-oriented and thus induces a net dipole moment in the dielectric. This produces an electric field. The diagram clearly shows that the net electric field in case of a conductor becomes zero, whereas in case of a dielectric net electric field intensity becomes non zero.
    
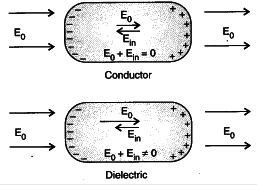
    However, the opposing field is so induced that does not exactly cancel the external field. It only reduces it. And the resultant electric field reduces by some value.
    Both polar and non-polar dielectric develop net dipole moment in the presence of an external field. The dipole moment per unit volume of the substance is called polarisation and is denoted by P for linear isotropic dielectrics. {tex}P = \chi E{/tex}, {tex}\chi {/tex} is the susceptibility.
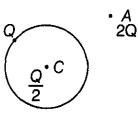
  1. At point C, inside the shell. The electric field inside a spherical shell is zero. Thus, the force experienced by the charge Q/2 kept at the centre C of the shell will also be zero.
    {tex}\because F _ { C } = q E \quad \left( E _ { \text { nside the shell } } = 0\right.{/tex})
    {tex}\therefore F _ { C } = 0{/tex}
    At point ‘A’, magnitude of the force experienced by charge +2Q there, IF_A= 2Q × {tex}\left( \frac { 1 } { 4 \pi \varepsilon _ { 0 } } \frac { 3 Q / 2 } { x ^ { 2 } } \right){/tex} (since 3Q/2 is the net charge of the shell)
    {tex}\therefore F = \frac { 3 Q ^ { 2 } } { 4 \pi \varepsilon _ { 0 } x ^ { 2 } }{/tex} , direction of this force is away from shell
  2. Electric flux through the shell, {tex}\phi = \frac { 1 } { \varepsilon _ { 0 } } {/tex} × magnitude of the net charge enclosed by the shell. (In this case there is no effect of the charge which lies above the shell)
    {tex}\Rightarrow \quad \phi = 1 / \varepsilon _ { 0 } \times Q / 2 = Q / 2 \varepsilon _ { 0 }{/tex}