Class 12 Applied Maths Sample Paper 2025

Table of Contents

myCBSEguide App

Download the app to get CBSE Sample Papers 2024-25, NCERT Solutions (Revised), Most Important Questions, Previous Year Question Bank, Mock Tests, and Detailed Notes.

Install Now

Class 12 Applied Maths Sample Papers 2025

Applied Maths is a newly introduced subject for commerce students in Class 12, offering an ideal choice for those who don’t plan on pursuing higher studies in Mathematics. CBSE Class 12 Applied Maths Sample Papers 2025 are now available for free download on the myCBSEguide app and website in PDF format. Teachers can easily create customized exam papers or mock papers using the Examin8 app, adding their name, logo, and branding. This app allows educators to design professional-quality question papers for practice or assessments. Enhance your teaching experience with Examin8 Website and streamline the paper creation process.

These model question papers are designed to help students familiarize themselves with the exam format and improve their problem-solving skills. By practicing with these Class 12 Applied Maths sample papers, students can enhance their chances of scoring higher grades in the CBSE board exams.

Class 12 Sample papers Download as PDF

The CBSE Class 12 Applied Maths exam 2025 will be conducted for 80 marks, with the remaining 20 marks allocated for internal assessments. These Mock Papers are a great tool for thorough preparation and boosting exam confidence.
Visit our Website myCBSEGuide

Model Papers of Applied Maths 2024-25

The Class 12 Applied Maths 2025 session follows a well-defined structure with five sections. The question paper is designed to assess a range of skills, with a total of 38 questions distributed across the sections:

Section A: This section consists of objective-type questions and is worth 20 marks. It tests students’ basic understanding of key concepts.
Section B: This section includes five very short answer questions, aimed at assessing quick recall and understanding of specific topics.
Section C: With 6 three-mark questions, Section C covers a range of concepts and requires students to apply their knowledge in more detailed answers.
Section D: This section includes 4 five-mark questions, focusing on more complex problem-solving and in-depth explanations.
Section E: The final section features 3 case study-based questions, designed to test students’ ability to apply mathematical concepts to real-world scenarios.

In total, the Class 12 Applied Maths question paper 2025 consists of 38 questions spread across these five sections. Understanding this structure will help students plan their time and approach more effectively during the exam.

Applied Maths Sample Question Paper 2025

Class 12 – Applied Maths
Sample Paper – 01 (2024-25)

Maximum Marks: 80
Time Allowed: : 3 hours

General Instructions:

Read the following instructions very carefully and strictly follow them:

This Question paper contains 38 questions. All questions are compulsory.
This Question paper is divided into five Sections – A, B, C, D and E.
In Section A, Questions no. 1 to 18 are multiple choice questions (MCQs) and Questions no. 19 and 20 are Assertion-Reason based questions of 1 mark each.
In Section B, Questions no. 21 to 25 are Very Short Answer (VSA)-type questions, carrying 2 marks each.
In Section C, Questions no. 26 to 31 are Short Answer (SA)-type questions, carrying 3 marks each.
In Section D, Questions no. 32 to 35 are Long Answer (LA)-type questions, carrying 5 marks each.
In Section E, Questions no. 36 to 38 are case study-based questions carrying 4 marks each.
There is no overall choice. However, an internal choice has been provided in 2 questions in Section B, 2 questions in Section C, 2 questions in Section D and one sub-part each in 2 questions of Section E.
Use of calculators is not allowed.

Section A
If A is a square matrix of order 3 and |A| = 2, then the value of |-AA’| is
a) 4
b) -2
c) -4
d) 2
A sample of 50 bulbs is taken at random. Out of 50 we found 15 bulbs are of Bajaj, 17 are of Surya and 18 are of Crompton. What is the point estimate of population proportion of Surya?
a) 0.3
b) 0.34
c) 0.36
d) 0.4
The assumption in calculating annuity is that every payment is
a) equal
b) marginal
c) nominal
d)different
The maximum value of Z = 3x + 4y subject to the constraints: x + y {tex}\leq{/tex} 4, x {tex}\geq{/tex} 0, y {tex}\geq{/tex} 0 is:
a)0
b)18
c)16
d)12
The matrix {tex}\left[\begin{array}{ccc} 0 & -5 & 3 \\ 5 & 0 & -7 \\ -3 & 7 & 0 \end{array}\right]{/tex} is a
a)symmetric matrix
b)skew-symmetric matrix
c)scalar matrix
d)diagonal matrix
If the mean and the variance of a probability distribution are 4 and 2 respectively, then the probability of two successes is
a){tex}\frac{219}{256}{/tex}
b){tex}\frac{7}{64}{/tex}
c){tex}\frac{1}{2}{/tex}
d){tex}\frac{37}{256}{/tex}
For the following probability distribution:
X -4 -3 -2 -1 0
P(X) 0.1 0.2 0.3 0.2 0.2
The value of E(X) is:
a)-1
b)0
c)-1.8
d)-2
Solution of the differential equation {tex}x \frac{d y}{d x}+2 y{/tex} = x² is
a){tex}y=\frac{x^2}{4}+\mathrm{C}{/tex}
b){tex}y=\frac{x^4+\mathrm{C}}{4 x^2}{/tex}
c){tex}y=\frac{x^2+C}{4 x^2}{/tex}
d){tex}y=\frac{x^2+C}{x^2}{/tex}
A pipe fills {tex}\frac{3}{7}^{\text {th }}{/tex} part of a tank in 1 hour. The rest of the tank can be filled in
a){tex}\frac{7}{3}{/tex} hours
b){tex}\frac{3}{4}{/tex} hours
c){tex}\frac{4}{3}{/tex} hours
d){tex}\frac{7}{4}{/tex} hours
If F(x) = {tex}\left[\begin{array}{ll} cos x & sin x \\ -sin x & cos x \end{array}\right]{/tex}, then F(x) F(y) is equal to:
a)F(x – y)
b)F(xy)
c)F(x)
d)F(x + y)
(09 : 30 + 16 : 40) in 24 hours clock is
a)2 : 10
b)26 : 10
c)25 : 70
d)03 : 10
The solution set of the inequation |x + 2| {tex}\leq{/tex} 5 is
a)(-7, 5)
b)|x| {tex}\leq{/tex} 5
c)[-5, 5]
d)[-7, 3]
In a 400 m race, A gives B a start of 5 seconds and beats him by 15 m. In another race of 400 m, A beats B by 7{tex}\frac 17{/tex}seconds. Their respective speeds are:
a)8 m/sec, 7 m/sec
b)5 m/sec, 7 m/sec
c)9 m/sec, 7 m/sec
d)6 m/sec, 7 m/sec
Comer points of the feasible region for an LPP are : (0, 2), (3, 0), (6, 0), (6, 8) and (0, 5). Let z = 4x + 6y be the objective function. Then, Max. z – Min. z =
a)48
b)42
c)60
d)18
The objective function of an LPP is
a)a relation between the variables
b)a function to be not optimized
c)a constrain
d)a function to be optimized
For the purpose of t-test of significance, a random sample of size (n) 34 is drawn from a normal population, then the degree of freedom (v) is
a)35
b){tex}\frac{1}{34}{/tex}
c)33
d)34
The value of {tex}\int \frac{1}{x+x \log x} d x{/tex} is
a)log (1 + log x)
b)x + log x
c)x log (1 + log x)
d)1 + log x
A factory production is delayed for three weeks due to breakdown of a machine and unavailability of spare parts. Under which trend oscillation does this situation fall?
a)Cyclical
b)Seasonal
c)Secular
d)Irregular
Let A and B be two square matrices of the same order.
Assertion (A): (A’BA) is symmetric if B is symmetric.
Reason (R): (A’BA) is skew-symmetric if B is skew-symmetric.
a)Both A and R are true and R is the correct explanation of A.
b)Both A and R are true but R is not the correct explanation of A.
c)A is true but R is false.
d)A is false but R is true.
Assertion (A): The tangent at x = 1 to the curve y = x³ – x² – x + 2 again meets the curve at x = -2.
Reason (R): When a equation of a tangent solved with the curve, repeated roots are obtained at point of tangency.
a)Both A and R are true and R is the correct explanation of A.
b)Both A and R are true but R is not the correct explanation of A.
c)A is true but R is false.
d)A is false but R is true.
Prepare for Exams with myCBSEguide App – Your Ultimate Study Companion
Visit our Website myCBSEGuide. To excel in your exams, download the myCBSEguide app today! This all-in-one app offers comprehensive study material for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams. Whether you’re preparing for school exams or competitive entrance tests, myCBSEguide provides a wealth of resources, including practice questions, solved papers, and more. With the Class 12 Applied Maths 2025 syllabus in mind, we have curated a list of essential resources to help students excel in both theory and application-based questions.
For teachers, the Examin8 app is the perfect tool to create customized practice papers with your branding, including your name and logo. Enhance your teaching experience and help students prepare better with personalized assessments.
Key Features of myCBSEguide:
- Complete study material for CBSE, JEE (Main), NEET-UG, and NDA.
- Practice papers, solved examples, and previous years’ question papers.
- Easy access to NCERT solutions and notes.
- User-friendly interface for quick learning.
Key Features of Examin8:
- Create customized exams with your own name and logo.
- Personalize the content for students.
- Streamlined paper generation for teachers.
Download the myCBSEguide App now to start preparing smarter and stay ahead of the competition! You can access detailed NCERT solutions for every chapter of Class 12 Applied Maths 2025 on our platform, ensuring you understand each concept thoroughly.
Explore Our Website for More Information & Resources
We invite you to visit our website and explore a wide range of resources designed to help you succeed in your academic journey. Whether you’re preparing for CBSE, JEE (Main), NEET-UG, or NDA exams, our platform offers comprehensive study material, practice papers, and much more to help you prepare effectively.
Why visit our website myCBSEGuide?
- Access high-quality study material and practice questions.
- Download useful apps like myCBSEguide and Examin8 for personalized learning.
- Stay updated with the latest resources and exam tips.
Don’t miss out on the opportunity to enhance your preparation! Visit us today and take the next step towards achieving your goals.
Section B
Rahul borrowed ₹100000 from a co-operative society at the rate of 10% p.a. for 2 years. Calculate his EMI using flat rate method.
OR
If money is worth 5% compare the present value of a perpetuity of ₹2,000 payable at the end of each year with that of an ordinary annuity of ₹2,000 per year for 100 years. (Given (1.05)^-100 = 0.0076)
Assuming a four yearly cycle, calculate the trend by the method of moving averages from the following data:
Year 1984 1985 1986 1987 1988 1989 1990 1991 1992 1993
Value 12 25 39 54 70 87 105 100 82 65
Evaluate: {tex}\int_\limits{1}^{2} \frac{1}{x\left(1+x^{2}\right)}{/tex}dx
If A is a non-singular square matrix of order 3 such that |adj A| = 225, find |A’|.
OR
If A = {tex}\left[\begin{array}{r} 1 \\ -4 \\ 3 \end{array}\right]{/tex} and B = [-1 2 1], verify that (AB)’ = B’A’.
Find the unit digit in 183! + 3¹⁸³.
Section C
A bond has issued with the face (Par) value of ₹ 1,000 at 10% coupon for three years The required rate of return is 8%. What is the value of the bond if the coupon amount is payable on half-yearly basis? Given (1.04)^-6 = 0.79031
The rate of increase of bacteria in a culture is proportional to the number of bacteria present and it is found that the number doubles in 6 hours. Prove that the bacteria becomes 8 times at the end of 18 hours.
OR
Show that the differential equation representing one parameter family of curves (x² – y²) = c(x² + y²)² is (x² – 3xy²) dx = (y² – 3x²y) dy
The marginal revenue of a company is given by MR = 100 + 20Q + 3Q², where Q is the amount of units sold for a period. Find the total revenue function if at Q = 2 it is equal to 260.
Fit a straight line trend by the method of least squares to the data given below:
Years 2012 2013 2014 2015 2016 2017 2018
Sales (in tones) 9 11 13 12 14 15 17
Ten cartons are taken at random from an automatic filling machine. The mean net weight of the cartons is 11.8 kg and the standard deviation 0.15 kg. Does the sample mean differ significantly from the intended weight of 12 kg? [Given that for d.f. = 9, t_0.05 = 2.26]
In a certain examination, the percentage of passes and distinction were 46 and 9 respectively. Estimate the average marks obtained by the candidate, the minimum pass and distinction marks being 40 and 75 respectively (assume the distribution of marks to be normal).
OR
A factory produces bulbs. The probability that one bulb is defective is {tex}\frac{1}{50}{/tex} and they are packed in boxes of 10. From a single box, find the probability that
1. none of the bulbs is defective
2. exactly two bulbs are defective
3. more than 8 bulbs work properly.
Section D
A manufacturer produces two models of bikes-model X and model Y. Model X takes a 6 man-hours to make per unit, while model Y takes 10 man-hours per unit. There is a total of 450 man-hours available per week. Handling and marketing costs are ₹2000 and ₹1000 per unit for Models X and Y respectively. The total funds available for these purposes are ₹80000 per week. Profit per unit for models X and Yare noon and ₹ 600 respectively. How many bikes of each model should the manufacturer produce so as to yield a maximum profit? Form an L.P.P. and solve it graphically using iso-profit/iso-cost method.
OR
Two tailors P and Q earn ₹ 150 and ₹ 200 per day respectively. P can stitch 6 shirts and 4 trousers a day, while Q can stitch 10 shirts and 4 trousers per day. How many days should each work to produce at least 60 shirts and 32 trousers at minimum labour cost?
Let X denote the number of hours a Class 12 student studies during a randomly selected school day. The probability that X can take the values x_i, for an unknown constant k:
{tex}P(X=k)=\left\{\begin{array}{ccc} 0 \cdot 1 & \text { if } & x_i=0 \\ k x_i & \text { if } & x_i=1 \text { or } 2 \\ k\left(5-x_i\right) & \text { if } & x_i=3 \text { or } 4 \end{array}\right.{/tex}
1. Find the value of k.
2. Determine the probability that the student studied for at least 2 hours.
3. Determine the probability that the student studied for at most 2 hours.
OR
Two numbers are selected at random (without replacement) from the first six positive integers. Let X denote the larger of the two numbers obtained. Find E(X).
Find the linear inequations for which the shaded area in figure is the solution set.
A machine costing ₹ 200000 has an effective life of 7 years and its scrap value is ₹ 30000. What amount should the company put into a sinking fund earning 5% per annum, so that it can replace the machine after its useful life? Assume that a new machine will cost ₹ 300000 after 7 years.
Section E
Read the following text carefully and answer the questions that follow:
Derivative of y = f(x) w.r.t. x (if exists) is denoted by {tex}\frac{d y}{d x}{/tex} or f'(x) and is called the first-order derivative of y. If we take the derivative of {tex}\frac{d y}{d x}{/tex} again, then we get {tex}\frac{d}{d x}\left(\frac{d y}{d x}\right)=\frac{d^{2} y}{d x^{2}}{/tex} or f”(x) and is called the second-order derivative of y. Similarly, {tex}\frac{d}{d x}\left(\frac{d^{2} y}{d x^{2}}\right){/tex} is denoted and defined as {tex}\frac{d^{3} y}{d x^{3}}{/tex} or f'”(x) and is known as the third-order derivative of y and so on.
1. If y = tan^-1{tex}\left(\frac{\log \left(e / x^{2}\right)}{\log \left(e x^{2}\right)}\right){/tex} + tan^-1{tex}\left(\frac{3+2 \log x}{1-6 \log x}\right){/tex},then find the value of{tex}\frac{d^{2}y}{d x^{2}}{/tex}. (1)
2. If u = x² + y² and x = s + 3t, y = 2s – t, then find the value of {tex}\frac{d^{2} u}{d s^{2}}{/tex}. (1)
3. If f(x) = 2 log sin x, then find f'(x). (2)
  OR
  If f(x) = e^xsinx, then find f”(x). (2)
Read the following text carefully and answer the questions that follow:
What Is a Sinking Fund?
A sinking fund contains money set aside or saved to pay off a debt or bond. A company that issues debt will need to pay that debt off in the future, and the sinking fund helps to soften the hardship of a large outlay of revenue. A sinking fund allows companies that have floated debt in the form of bonds gradually save money and avoid a large lump-sum payment at maturity.
Example:
- Cost of Machine: ₹2,00,000/-
- Effective Life: 7 Years
- Scrap Value: ₹30,000/-
- Sinking Fund Earning Rate: 5%
- The Expected Cost of New Machine: ₹3,00,000/-
1. What is the money required for a new machine after 7 years? (1)
2. What is the value of A, i and n here? (1)
3. What formula will you use to get the requisite amount? (2)
  OR
  What amount should the company put into a sinking fund earning 5% per annum to replace the machine after its useful life? (2)
Read the following text carefully and answer the questions that follow:
Two schools P and Q decided to award their selected students for the values of discipline and honesty in the form of prizes at the rate of ₹ x and ₹ y respectively. School P decided to award respectively 3, 2 students a total prize money of ₹ 2300 and school Q decided to award respectively 5, 3 students a total prize money of ₹ 3700.
1. Write the matrix equation representing the above situation. (1)
2. Find the value of the determinant of coefficients of x and y. (1)
3. Find the values of x and y respectively (use Cramer’s rule). (2)
  OR
  Find the inverse of matrix A. (2)
  Looking to practice more questions and prepare effectively for your exams? Download the myCBSEguide App today! It offers a complete range of study materials for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams. Whether you’re a student or a teacher, myCBSEguide provides you with the tools needed to ace your exams. Preparing for the Class 12 Applied Maths 2025 exam requires a structured study plan, and we provide the necessary tools and resources to help you stay on trackWhy Choose myCBSEguide?
  - Access curated study notes, NCERT solutions, previous year papers, and more.
  - Test your knowledge with a wide variety of practice questions to strengthen your understanding.
  - Easy navigation for seamless learning on-the-go.
  Examin8 App for Teachers Teachers can also benefit from the Examin8 App to create custom exam papers tailored with their name and logo. Personalize your practice papers to better serve your students and track their progress.
  Key Features for Teachers:
  - Create customized exam papers with your branding.
  - Generate personalized assessments to suit your teaching style.
  - Track student progress efficiently.
  Start preparing smarter and enhance your exam readiness. Download the myCBSEguide App and the Examin8 App now! Class 12 Applied Maths 2025 covers a wide range of topics, including matrices, determinants, and differential equations, all of which are critical for understanding advanced mathematics.

Class 12 – Applied Maths
Sample Paper – 01 (2024-25)

Solution

Section A
(c)
-4
Explanation: Given |A| = 2, order of A = 3.
So, |-AA’| = |-A| {tex}\cdot{/tex} |A’| = (-1)³| A|{tex}\cdot{/tex}|A|
= -1|A|² = -1(2)² = -4
(b)
0.34
Explanation: 0.34
(a)
equal
Explanation: equal
(c)
16
Explanation: 16
(b)
skew-symmetric matrix
Explanation: Let A = {tex}\left[\begin{array}{rrr} 0 & -5 & 3 \\ 5 & 0 & -7 \\ -3 & 7 & 0 \end{array}\right]{/tex}
So, A’ = {tex}\left[\begin{array}{rrr} 0 & 5 & -3 \\ -5 & 0 & 7 \\ 3 & -7 & 0 \end{array}\right]{/tex} {tex}=-\left[\begin{array}{rrr} 0 & -5 & 3 \\ 5 & 0 & -7 \\ -3 & 7 & 0 \end{array}\right]{/tex} = -A
{tex}\Rightarrow{/tex} A is a skew-symmetric matrix.
{tex}\therefore{/tex} Option (skew-symmetric matrix) is the correct answer.
(b)
{tex}\frac{7}{64}{/tex}
Explanation: Given np = 4 and npq = 2
{tex}\Rightarrow \frac{n p q}{n p}=\frac{2}{4} \Rightarrow q=\frac{1}{2}{/tex}
{tex}\therefore{/tex} p = 1{tex}-\frac{1}{2}=\frac{1}{2}{/tex}, so n {tex}\times{/tex} {tex}\frac{1}{2}{/tex} = 4 {tex}\Rightarrow{/tex} n = 8
Now, P(X = 2) = {tex}{ }^8 C _2\left(\frac{1}{2}\right)^2\left(\frac{1}{2}\right)^6=\frac{28}{256}=\frac{7}{64}{/tex}
(c)
-1.8
Explanation: E(X) = {tex}\sum {/tex}X P(X)
= -4 {tex}\times{/tex} (0.1) + (-3 {tex}\times{/tex} 0.2) + (-2 {tex}\times{/tex} 0.3) + (-1 +0.2) + (0 {tex}\times{/tex} 0.2)
= -0.4 – 0.6 – 0.6 – 0.2 = -1.8
(b)
{tex}y=\frac{x^4+\mathrm{C}}{4 x^2}{/tex}
Explanation: {tex}\frac{d y}{d x}+\frac{2}{x}{/tex}y = x {tex}\Rightarrow{/tex} I.F. = {tex}e^{\int \frac{2}{x} d x}{/tex} = {tex}e^{2 \log x}{/tex} = x²
{tex}\therefore{/tex} Solution is y {tex}\cdot{/tex} x² = {tex}\int{/tex} x {tex}\cdot{/tex} x² dx + C₁
y {tex}\cdot{/tex} x² = {tex}\frac{x^4}{4}{/tex} + C₁ {tex}\Rightarrow{/tex} y = {tex}\frac{x^4+C}{4 x^2}{/tex}
(c)
{tex}\frac{4}{3}{/tex} hours
Explanation: {tex}\frac {3}{7}{/tex}l {tex}\rightarrow{/tex} 1 min
remaining amount = {tex}1 – \frac {3}{7}{/tex} = {tex}\frac {4}{7}{/tex}ltr
since time taken to fill 1 litre
= {tex}\frac {3}{7}{/tex}min
So time required to fill {tex}\frac {4}{7}{/tex}litres
= {tex}\frac {7}{3} \times \frac {4}{7}{/tex} = {tex}\frac {4}{3}{/tex}min
(d)
F(x + y)
Explanation: F(x + y)
(a)
2 : 10
Explanation: (09: 30 + 16 : 40) (mod 24) = 26 : 10 (mod 24) = 2 : 10
(d)
[-7, 3]
Explanation: |x + 2| {tex}\le{/tex} 5
{tex}\Rightarrow{/tex} -5 {tex}\le{/tex} x + 2 {tex}\le{/tex} 5
{tex}\Rightarrow{/tex} -7 {tex}\le{/tex} x {tex}\le{/tex} 3
{tex}\Rightarrow{/tex} x {tex}\in{/tex} [-7, -3]
(a)
8 m/sec, 7 m/sec
Explanation: Suppose A covers 400 m in t seconds
Then, B covers 385 m in (t + 5) seconds
{tex}\therefore{/tex} B covers 400 m = {tex}\left\{\frac{(t+5)}{385} \times 400\right\}{/tex}sec
= {tex}\frac{80(t+5)}{77}{/tex} sec
Also, B covers 400 m = {tex}\left(t+7 \frac{1}{7}\right){/tex}sec
= {tex}\frac{(7 t+50)}{7}{/tex}sec
{tex}\therefore{/tex} {tex}\frac{80(t+5)}{77}=\frac{7 t+50}{7}{/tex}
{tex}\therefore{/tex} 80(t + 5) = 11(7t + 50)
{tex}\Rightarrow{/tex} (80t – 77t) = (550 – 400)
{tex}\Rightarrow{/tex} 3t = 150
{tex}\Rightarrow{/tex} t = 50
{tex}\therefore{/tex} A’s speed
= {tex}\frac{400}{50}{/tex} m/sec
= 8 m/sec
{tex}\therefore{/tex} B’s speed
= {tex}\frac {385}{55}{/tex} m/sec
= 7 m/sec
(c)
60
Explanation: Here the objective function is given by:
F = 4x + 6y
Corner points Z = 4x +6 y
(0, 2) 12 (Min.)
(3, 0) 12 (Min.)
(6, 0) 24
(6, 8) 72 (Max.)
(0, 5) 30
Maximum of F – Minimum of F = 72 – 12 = 60
(d)
a function to be optimized
Explanation: A Linear programming problem is a linear function (also known as an objective function) subjected to certain constraints for which we need to find an optimal solution (i.e. either a maximum/minimum value) depending on the requirement of the problem.
From the above definition, we can clearly say that the Linear programming problem’s objective is to either maximize/minimize a given objective function, which means to optimize a function to get an optimum solution.
(c)
33
Explanation: Given n = 34
{tex}\Rightarrow{/tex} degree of freedom (v) = 34 – 1 = 33
(a)
log (1 + log x)
Explanation: {tex}I=\int \frac{1}{x+x \log x} d x{/tex}
{tex}I=\int \frac{d x}{x(1+\log x)}{/tex}
Put 1 + log x = t
{tex}\Rightarrow \frac{1}{x} d x=d t{/tex}
{tex}I=\int \frac{1}{t} d t{/tex}
{tex}\Rightarrow{/tex} I = log |t| + C
I = log (1 + log x) + C
(d)
Irregular
Explanation: Irregular
(b)
Both A and R are true but R is not the correct explanation of A.
Explanation: Given A and B are square matrices of the same order, so, A’BA is defined.
Let B be symmetric, then B’ = B.
Now, (A’BA)’ = A’B'(A’)’ = A’BA {tex}\Rightarrow{/tex} A’BA is symmetric.
{tex}\therefore{/tex} Assertion is true.
Let B be skew-symmetric, then B’ = -B.
Now, (A’BA)’ = A’B'(A’)’ = A'(-B)A = -A’BA
{tex}\Rightarrow{/tex} A’BA is skew-symmetric.
{tex}\therefore{/tex} Both A and R are true but R is not the correct explanation of A.
{tex}\therefore{/tex} Option (Both A and R are true but R is not the correct explanation of A) is the correct answer.
(d)
A is false but R is true.
Explanation: When x = 1, then y = (1)³ – (1)³ – 1 + 2 = 1
{tex}\therefore \frac {dy}{dx}{/tex} = 3x² – 2x – 1 {tex}\left. \Rightarrow \frac {dy}{dx}\right|_{x = 1}{/tex} = 0
{tex}\therefore{/tex} Equation of tangent at point (1, 1) is
y – 1 = 0(x – 1) {tex}\Rightarrow{/tex} y = 1
Solving with the curve, x³ – x² – x + 2 = 1
{tex}\Rightarrow{/tex} x³ – x² – x + 1 = 0
{tex}\Rightarrow{/tex} (x – 1)(x² – 1) = 0 {tex}\Rightarrow{/tex} = 1, 1, -1 [here, 1 is repeated root] {tex}\therefore{/tex} Tangent meets the curve again at x = -1
{tex}\therefore{/tex} Assertion is false, Reason is true.
Prepare smarter and score better by downloading the myCBSEguide app today! Whether you’re gearing up for CBSE, NCERT, JEE (Main), NEET-UG, or NDA exams, myCBSEguide offers comprehensive study resources, including practice questions, solved papers, NCERT solutions, and more. With expertly curated content and easy-to-use features, the app is designed to help you achieve top scores with confidence. As Class 12 Applied Maths 2025 draws closer, students should prioritize understanding the application of mathematical concepts to real-world problems.
For teachers, the Examin8 app is an ideal solution to create custom exam papers. Personalize tests with your name, logo, and branding, making it easy to assess student progress and track performance.To excel in Class 12 Applied Maths 2025, it’s crucial to focus on problem-solving techniques that emphasize both theoretical knowledge and practical applications.
Why Choose myCBSEguide App?
- Complete study material for CBSE, JEE (Main), NEET-UG, and NDA exams.
- Access to NCERT solutions, practice papers, and exam preparation tips.
- User-friendly interface to enhance your learning experience anytime, anywhere.Our expert-created practice papers for Class 12 Applied Maths 2025 will help you familiarize yourself with the exam format and the types of questions you’ll encounter.
Key Benefits of Examin8 for Teachers:
- Easily create customized exam papers with your own name and logo.
- Design personalized assessments tailored to your students’ needs.
- Track student performance and streamline exam management.
Don’t wait – download myCBSEguide now and start your journey towards exam success!
Section B
P = ₹100000, i = {tex}\frac{10}{12 \times 100}=\frac{1}{120}{/tex}, n = 2 {tex}\times{/tex}12 = 24.
{tex}\therefore{/tex} EMI = {tex}\frac{\mathrm{P} \ \ + \ \ \mathrm{P} n i}{n}{/tex} = {tex}\frac{100000 \ \ + \ \ 100000 \ \ \times \ \ \frac{1}{120} \ \ \times \ \ 24}{24} {/tex}
= {tex}\frac{100000 \ \ + \ \ 20000}{24}{/tex} = {tex}\frac{120000}{24}{/tex} = ₹5000
OR
Let P be the present value of a perpetuity of ₹2,000 payable at the end of each year when money is worth 5%. It is given that
i = {tex}\frac{5}{100}{/tex} = 0.05 and R = 2,000
{tex}\therefore{/tex} P = {tex}\frac{R}{i} \Rightarrow{/tex} P = ₹{tex}\frac{2,000}{0.05}{/tex} = ₹40,000
Let P₁ be the present value of an ordinary annuity of ₹2,000 per year for 100 years. Then,
P₁ = R{tex}\left\{\frac{1-(1-i)^{-n}}{i}\right\}{/tex}
We have, R = 2,000, i = {tex}\frac{5}{100}{/tex} = 0.05 and n = 100
{tex}\therefore{/tex} P₁ = ₹2,000 {tex}\left\{\frac{1-(1.05)^{-100}}{0.05}\right\}{/tex} = ₹2,000{tex}\left(\frac{1-0.0076}{0.05}\right){/tex} = ₹{tex}\frac{2,000 \times 0.9924}{0.05}{/tex} = ₹39,696
We observe that the present value of the perpetuity is more than that of ordinary annuity.
Let {tex}\frac{1}{x\left(1+x^{2}\right)}=\frac{A}{x}+\frac{B x+C}{1+x^{2}}{/tex} …(i)
Then, 1 = A(1 + x²) + (Bx + C)x …(ii)
Putting x = 0 in (ii), we get A = 1
Comparing the coefficients of x² and x in (ii), we get
A + B = 0 and C = 0 {tex}\Rightarrow{/tex} B = -1 and C = 0 [{tex}\because{/tex} A = 1] Substituting the values of A, B and C in (i), we get
{tex}\frac{1}{x\left(1+x^{2}\right)}=\frac{1}{x}-\frac{x}{1+x^{2}}{/tex}
{tex}\therefore{/tex} I = {tex}\int_\limits{1}^{2} \frac{1}{x\left(1+x^{2}\right)}{/tex} dx = {tex}\int_\limits{1}^{2} \frac{1}{x}{/tex} dx – {tex}\frac{1}{2} \int_\limits{1}^{2} \frac{2 x}{1+x^{2}}{/tex} dx = {tex}[\log x]_{1}^{2}-\frac{1}{2}\left[\log \left(1+x^{2}\right)\right]_{1}^{2}{/tex}
{tex}\Rightarrow{/tex} I = (log 2 – log 1) – {tex}\frac{1}{2}{/tex} (log 5 – log 2) = log 2 – {tex}\frac{1}{2}{/tex} log 5 + {tex}\frac{1}{2}{/tex} log 2 = {tex}\frac{3}{2}{/tex} log 2 – {tex}\frac{1}{2}{/tex} log 5
As A is a non-singular square matrix of order 3, |adj A| = |A|²
{tex}\Rightarrow{/tex} 225 = |A|² {tex}\Rightarrow{/tex} |A| = 15, -15
{tex}\Rightarrow{/tex} |A’| = 15, -15 ({tex}\because{/tex} |A’| = |A|)
OR
AB = {tex}\left[\begin{array}{r} 1 \\ -4 \\ 3 \end{array}\right]{/tex} [-1 2 1 ] = {tex}\left[\begin{array}{rrr} -1 & 2 & 1 \\ 4 & -8 & -4 \\ -3 & 6 & 3 \end{array}\right]{/tex} {tex}\Rightarrow{/tex} (AB)’ = {tex}\left[\begin{array}{rrr} -1 & 4 & -3 \\ 2 & -8 & 6 \\ 1 & -4 & 3 \end{array}\right]{/tex} and B’A’ = [-1 2 1]’ {tex}\left[\begin{array}{r} 1 \\ -4 \\ 3 \end{array}\right]^{\prime}=\left[\begin{array}{r} -1 \\ 2 \\ 1 \end{array}\right]{/tex} [1 -4 3] {tex}= \left[\begin{array}{rrr} -1 & 4 & -3 \\ 2 & -8 & 6 \\ 1 & -4 & 3 \end{array}\right]{/tex}
{tex}\Rightarrow{/tex} (AB)’ = B’A’.
We have, 5! = 120, 6! = 720, 7! =5040 etc.
In fact units digit in n! for n {tex}\geq{/tex} 5 is 0. Therefore, units digit in 183! is 0. Consequently, units digit in 183! + 3¹⁸³ is same as the units digit in 3¹⁸³.
Now, 3² = 9 {tex}\equiv{/tex} -1 (mod 10) and, 3₁₈₃ = (3²)⁹¹ {tex}\times{/tex} 3
{tex}\therefore{/tex} 3² {tex}\equiv{/tex} -1 (mod 10)
{tex}\Rightarrow{/tex} (3²)⁹¹{tex}\equiv{/tex} (-1)⁹¹ (mod 10)
{tex}\Rightarrow{/tex} (3²)⁹¹ {tex}\equiv{/tex} -1 (mod 10)
{tex}\Rightarrow{/tex} (3²)⁹¹ {tex}\times{/tex} 3 {tex}\equiv{/tex} -3 (mod 10)
{tex}\Rightarrow{/tex} (3²)⁹¹ {tex}\times{/tex} 3 {tex}\equiv{/tex} 7 (mod 10)
{tex}\Rightarrow{/tex} 3¹⁸³ {tex}\equiv{/tex} 7 (mod 10)
{tex}\therefore{/tex} Units digit in 3¹⁸³ is 7.
Hence, the units digit in 183! + 3¹⁸³ is 7
Section C
Given, P = ₹ 1,000 Annual Coupon Payment
= ₹ 1,000 {tex}\times{/tex} 10% = ₹ 100
Semi-annual Coupon Payment,
C = ₹ 100 + 2 = ₹ 50
r = 8% + 2
= 4%
= 0.04
N = 3 year {tex}\times{/tex} 2 = 6 periods for semi-annual coupon payments
Bond Value = C {tex}\times \frac{1-(1+r)^{-N}}{r}+\frac{P}{(1+r)^{-N}}{/tex}
= 50 {tex}\times \frac{1-(1+0.04)^{-6}}{0.04}{/tex}{tex}+\frac{1000}{(1+0.04)^{6}}{/tex}
= 50 {tex}\times \frac{1-0.79031}{0.04}{/tex} + 1000 {tex}\times{/tex} 0.79031
= ₹ 262.1125 + 790.31
= ₹ 1053.42
Let A be the quantity of bacteria present in culture at any time t and initial quantity of bacteria is A₀
{tex}\frac{d A}{d A} \propto A{/tex}
{tex}\frac{d A}{d t}=\lambda A {/tex}
{tex}\frac{d A}{A}=\lambda d t {/tex}
{tex}\int \frac{d A}{A}= \int \lambda d t {/tex}
log A = {tex}\lambda{/tex}t + c …(i)
Initially, A = A₀, t = 0
log A₀ = 0 + c
log A₀ = c
Now equation (i) becomes,
log A = {tex}\lambda{/tex}t + log A₀
{tex}\log \left(\frac{A}{A_{0}}\right) = \lambda t{/tex} …(ii)
Given A = 2 A₀ when t = 6 hours
{tex}\log \left(\frac{A}{A_{0}}\right) = 6 \lambda{/tex}
{tex}\frac{\log 2}{6} = \lambda{/tex}
Now equation (ii) becomes,
{tex}\log \left(\frac{A}{A_{0}}\right) = \frac{\log 2}{6} t{/tex}
Now, A = 8 A₀
so, {tex}\log \left(\frac{8 A_{0}}{A_{0}}\right) = \frac{\log 2}{6} t{/tex}
log 2³ = {tex}\frac{\log 2}{6} t {/tex}
3 log 2 = {tex}\frac{\log 2}{6} t{/tex}
18 = t
Hence, Bacteria becomes 8 times in 18 hours.
OR
The given equation of one parameter family of curves is
x² – y² c(x² + y²)² …(i)
Differentiating (i) with respect to x, we get
2x – 2y{tex}\frac {dy}{dx}{/tex} = 2c(x² + y²)(2x + 2y{tex}\frac {dy}{dx}{/tex})
{tex}\Rightarrow{/tex} (x – y{tex}\frac {dy}{dx}{/tex}) = 2c(x² + y²)(x + y{tex}\frac {dy}{dx}{/tex}) …(ii)
On substituting the value of c obtained from (i) in (ii), we get,
{tex}\left(x-y \frac{d y}{d x}\right)=\frac{2\left(x^{2}-y^{2}\right)\left(x^{2}+y^{2}\right)}{\left(x^{2}+y^{2}\right)^{2}}\left(x+y \frac{d y}{d x}\right){/tex}
{tex}\Rightarrow{/tex} (x² + y²)(x – y{tex}\frac {dy}{dx}{/tex}) = 2(x² – y²)(x + y{tex}\frac {dy}{dx}{/tex})
{tex}\Rightarrow{/tex} {x(x² + y²) – 2x(x² – y²)} = {tex}\frac {dy}{dx}{/tex}{2y(x² – y²) + y(x² + y²)}
{tex}\Rightarrow{/tex} (3xy² – x³) = {tex}\frac {dy}{dx}{/tex}(3x²y – y³)
{tex}\Rightarrow{/tex} (x³ – 3 xy²) dx = (y³ – 3x²y) dy, which is the given differential equation.
We find the total revenue function TR by integrating the marginal revenue function MR:
TR (Q) = {tex}\int{/tex} MR (Q) dQ
= {tex}\int{/tex} (100 + 20Q + 3Q²) dQ
= 100Q + 10Q² + Q³ + C
The constant of integration C can be determined using the initial condition TR (Q=2) = 260. Hence,
200 + 40 + 8 + C = 260
C = 12
So, the total revenue function is given by
TR (Q) = 100Q + 10Q² + Q³ + 12

Years (t_i)	Sales (y_i)	x_i = t_i – 2015	{tex}x ^2_i{/tex}	x_iy_i
2012	9	-3	9	-27
2013	11	-2	4	-22
2014	13	-1	1	-13
2015	12	0	0	0
2016	14	1	1	14
2017	15	2	4	30
2018	17	3	9	51
n = 7	{tex}\sum y_i{/tex} = 91	{tex}\sum x_i{/tex} = 0	{tex}\sum x_i^2{/tex} = 28	{tex}\sum x_i y_i{/tex} = 33

{tex}a = \frac {\sum y _i}{n} = \frac {91}{7}{/tex} =13
{tex}b = \frac {\sum x_i y _i}{\sum x_i^2} = \frac {33}{28}{/tex} = 1.179
The equation of the straight line trend is
y = ax + b
{tex}\therefore{/tex} y = 13x + 1.179

{tex}\mu ={/tex} Population mean = 12 Kg
{tex}\overline{X}{/tex} = Sample mean = 11.8 Kg
n = 10
Sample standard deviation = s = 0.15
Null Hypothesis H₀= There is no significance between the sample mean
{tex}\overline{X}{/tex} and the population mean {tex}\mu {/tex}.
Alternate Hypothesis H₁ = There is significance between the sample mean {tex}\overline{X}{/tex} and the population mean {tex}\mu {/tex}
Let t be the test statistic given by
{tex}t = \frac{\overline{X}-\mu}{\frac{s}{\sqrt{n-1}}}{/tex}
{tex}t = \left(\frac{11.8-12}{0.15}\right)\times 3{/tex}
= -4
The test statistic t follows student t-distribution with (10-1)=9 degrees of freedom
It is given that t_0.05 = 2.26
We observe that,
|t| = 4>2.26
_{{tex}\implies{/tex}}Calculate |t| > tabulated t₉(0.05)
So, the null hypothesis is rejected at a 5% level of significance.
Hence there is a significance between the sample mean {tex}\overline{X}{/tex} and the population mean {tex}\mu{/tex}.
Let X denote the marks obtained by the candidates. Let {tex}\mu{/tex} be mean and {tex}\sigma {/tex} be the standard deviation of the normal distribution.
Let Z be the standard normal variate. Then,
Z = {tex}\frac{X-\mu}{\sigma}{/tex}
Now, X = 40 {tex}\Rightarrow{/tex} Z = {tex}\frac{40-\mu}{\sigma}{/tex} = Z₁ (say) and, X = 75 {tex}\Rightarrow{/tex} Z = {tex}\frac{75-\mu}{\sigma}{/tex} = Z₂ (say)
Now,
P(X {tex}\geq{/tex} 40) = 0.46 [Given] = P(Z {tex}\geq{/tex} Z₁) = 0.46
= P(Z {tex}\geq{/tex} 0) – P(0 {tex}\leq{/tex} Z {tex}\leq{/tex} Z₁) = 0.46 [{tex}\because{/tex} P(Z {tex}\leq{/tex} Z₁) < 0.5 {tex}\therefore{/tex} Z₁ > 0] = 0.5 – P(0 {tex}\leq{/tex} Z {tex}\leq{/tex} Z₁) = 0.46
= P(0 {tex}\leq{/tex} Z {tex}\leq{/tex} Z₁) = 0.04
= Z₁ = 0.1
= {tex}\frac{40-\mu}{\sigma}{/tex} = 0.1
= {tex}\mu{/tex} + 0.1 {tex}\sigma {/tex} = 40 …(i)

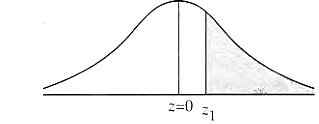
And,
P(X {tex}\geq{/tex} 75) = 0.09 [Given] = P(Z {tex}\geq{/tex} Z₂) = 0.09
= P(Z {tex}\geq{/tex} 0) – P(0 {tex}\leq{/tex} Z {tex}\leq{/tex} Z₂) = 0.09 [{tex}\because{/tex} P(Z {tex}\leq{/tex} Z₂) < 0.5 {tex}\therefore{/tex} Z₂ > 0] = 0.5 – P(0 {tex}\leq{/tex} Z {tex}\leq{/tex} Z₂) = 0.09
= P(0 {tex}\leq{/tex} Z {tex}\leq{/tex} Z₂) = 0.41
= Z₂ = 1.34
= {tex}\frac{75-\mu}{\sigma}{/tex} = 1.34
= {tex}\mu{/tex} + 1.34 {tex}\sigma {/tex} = 75 …(ii)

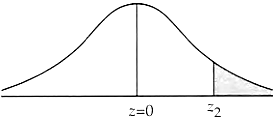
Solving (i) and (ii), we get {tex}\mu{/tex} = 37.18 and {tex}\sigma {/tex} = 28.22.
Thus, the average mark obtained by the candidates is 37
OR
Let X is the random variable that denotes that a bulb is defective.
Also, n = 10, {tex}p = \frac{1}{{50}}{/tex} and {tex}q = \frac{{49}}{{50}}{/tex} and P(X = r) {tex}{ = ^n}{C_r }{p^r}{q^{n – r }}{/tex}
1. None of the bulbs are defective i.e., r = 0
  {tex}\therefore P(X = r) = {P_{(0)}}{ = ^{10}}{C_0}{\left( {\frac{1}{{50}}} \right)^0}{\left( {\frac{{49}}{{50}}} \right)^{10 – 0}} = {\left( {\frac{{49}}{{50}}} \right)^{10}}{/tex}
2. Exactly two bulbs are defective i.e., r = 2
  {tex}\therefore P(X = r) = {P_{(2)}}{ = ^{10}}{C_2}{\left( {\frac{1}{{50}}} \right)^2}{\left( {\frac{{49}}{{50}}} \right)^8}{/tex}
  {tex} = \frac{{10!}}{{8!2!}}{\left( {\frac{1}{{50}}} \right)^2} \cdot {\left( {\frac{{49}}{{50}}} \right)^8} = 45 \times {\left( {\frac{1}{{50}}} \right)^{10}} \times {(49)^8}{/tex}
3. More than 8 bulbs work properly i.e., there are less than 2 bulbs that are defective.
  So, r < 2 {tex} \Rightarrow {/tex} r = 0,1
  {tex}\therefore {/tex} P(X = r) = P(r < 2) = P(0) + P(1)
  {tex}{ = ^{10}}{C_0}{\left( {\frac{1}{{50}}} \right)^0}{\left( {\frac{{49}}{{50}}} \right)^{10 – 0}}{ + ^{10}}{C_1}{\left( {\frac{1}{{50}}} \right)^1}{\left( {\frac{{49}}{{50}}} \right)^{10 – 1}}{/tex}
  {tex} = {\left( {\frac{{49}}{{50}}} \right)^{10}} + \frac{{10!}}{{1!9!}} \cdot \frac{1}{{50}} \cdot {\left( {\frac{{49}}{{50}}} \right)^9}{/tex}
  {tex} = {\left( {\frac{{49}}{{50}}} \right)^{10}} + \frac{1}{5} \cdot {\left( {\frac{{49}}{{50}}} \right)^9} = {\left( {\frac{{49}}{{50}}} \right)^9}\left( {\frac{{49}}{{50}} + \frac{1}{5}} \right){/tex}
  {tex} = {\left( {\frac{{49}}{{50}}} \right)^9}\left( {\frac{{59}}{{50}}} \right) = \frac{{59{{(49)}^9}}}{{{{(50)}^{10}}}}{/tex}

Section D
Let x and y be the number of bikes of model X and Y respectively, then the problem can be formulated as an L.P.P.as follows:
Maximize Z = 1000x + 600y subject to constraints
6x + 10y {tex}\leq{/tex} 450 (man hours constraint)
i.e. 3x + 5y {tex}\leq{/tex} 225
2000x + 1000 {tex}\leq{/tex} 80000 (handling and marketing constraints)
i.e. 2x + y {tex}\leq{/tex} 80
x {tex}\geq{/tex} 0, y {tex}\geq{/tex} 0 (non-negativity constraints)
Draw the lines 3x + 5y = 225 and 2x + y = 80 and shade the region satisfied by the above inequalities.

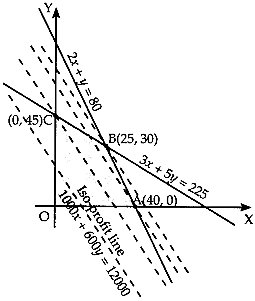
The feasible region OABC is convex and bounded.
The corner points are O(0, 0), A(40, 0), B(25, 30) and C(0, 45).
Now, let us give some value to Z say 12000 and draw a dotted line 1000x + 600y = 12000 which is iso-profit line. Move this line parallel to itself over the feasible region.
It passes through all corner points one by one. The farthest corner point it crosses is B(25, 30) which gives us the optimal solution.
Z = 1000 {tex}\times{/tex} 25 + 600 {tex}\times{/tex} 30 i.e. Z = 43000.
Hence, 25 bikes of model X and 30 bikes of model X should be manufactured to obtain maximum profit of ₹ 43000
OR
Let the tailor P work for x days and the tailor Q work for y days respectively.
Here, the problem can be formulated as an L.P.P. as follows:
Minimize Z = 150x + 200y
Subject to the constraints:
6x + 10y {tex}\geq{/tex} 60
or 3x + 5y {tex}\geq{/tex} 30 …(i)
4x + 4y {tex}\geq{/tex} 32
or x + y {tex}\geq{/tex} 8 …(ii)
and x {tex}\geq{/tex} 0, y {tex}\geq{/tex} 0
Converting them into equations we obtain the following equations:
3x + 5y = 30, x + y = 8
{tex}\Rightarrow{/tex} y = {tex}\frac{30-3 x}{5}{/tex}
{tex}\Rightarrow{/tex} y = 8 – x
x 0 10 5
y 6 0 3
x 0 8 5
y 8 0 3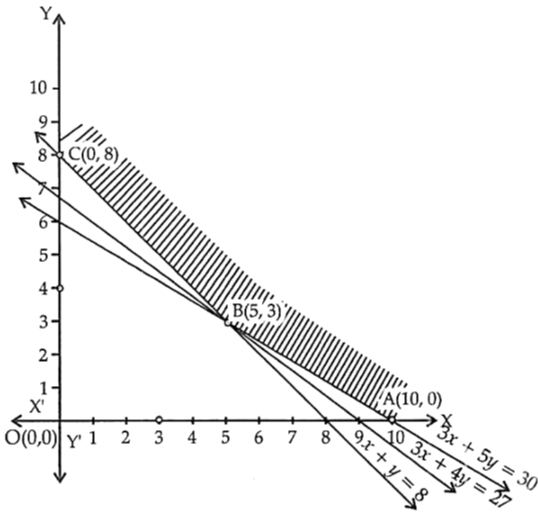

The shaded region in the diagram represent the feasible region.
The corner points are A(10, 0), B(5, 3) and C(0, 8)
At the corner point the value of Z = 150x + 200y
At (10, 0) Z = 1500
At B( 5, 3) Z = 150 {tex}\times{/tex} 5 + 200 {tex}\times{/tex} 3
= 750 + 600 = 1350
At C(0, 8) Z = 1600
As the feasible region is unbounded, we draw the graph of the half-plane.
150x + 200y < 1350
3x + 4y < 27
There is no point common with the feasible region, therefore, Z has minimum value.
Minimum value of Z is ₹ 1350 and it occurs at the point B(5, 3).
Hence, the labour cost is ₹ 1350 when P works for 5 days and Q works for 3 days.
1. 0.1 + k + 2k + 2k + k = 1
  {tex}\Rightarrow{/tex} 0.1 + 6k = 1
  {tex}\Rightarrow {k}=\frac{3}{20}{/tex}
2. {tex}{P}({X} \geq {2}){/tex} = P(2) + P(3) + P(4)
  = 2k + 2k + k
  = 5k = {tex}\frac{3}{4}{/tex}
3. {tex}{P}({X} \leq {2}){/tex} = P(0) + P(1) + P(2)
  = 0.1 + k + 2k
  {tex}=\frac{1}{10}+\frac{9}{20}=\frac{11}{20}{/tex}
OR
Given: first six positive integers.
Two numbers can be selected at random (without replacement) from the first six positive integer in 6 {tex}\times{/tex} 5 = 30 ways.
X denotes the larger of the two numbers obtained. Hence, X can take any value of 2, 3, 4, 5 or 6.
For X = 2, the possible observations are (1, 2) and (2, 1)
{tex}\Rightarrow P(X)=\frac{2}{30}=\frac{1}{15}{/tex}
For X = 3, the possible observations are (1, 3), (3, 1), (2, 3) and (3, 2).
{tex}\Rightarrow P(X)=\frac{4}{30}=\frac{2}{15}{/tex}
For X = 4, the possible observations are (1, 4), (4, 1), (2, 4), (4, 2), (3, 4) and (4, 3).
{tex}\Rightarrow P(X)=\frac{6}{30}=\frac{1}{5}{/tex}
For X = 5, the possible observations are (1, 5), (5, 1), (2, 5), (5, 2), (3, 5), (5, 3), (5, 4) and (4, 5).
{tex}\Rightarrow P(X)=\frac{8}{30}=\frac{4}{15}{/tex}
For X = 6, the possible observations are (1, 6), (6, 1), (2, 6), (6, 2), (3, 6), (6, 3) (6, 4), (4, 6), (5, 6) and (6, 5).
{tex}\Rightarrow P(X)=\frac{10}{30}=\frac{1}{3}{/tex}
Hence, the required probability distribution is,
X 2 3 4 5 6
P(X) {tex}\frac{1}{15}{/tex} {tex}\frac{2}{15}{/tex} {tex}\frac{1}{5}{/tex} {tex}\frac{4}{15}{/tex} {tex}\frac{1}{3}{/tex}
Therefore E(X) = {tex}2 \times \frac{1}{15}+3 \times \frac{2}{15}+4 \times \frac{1}{5}+5 \times \frac{4}{15}+6 \times \frac{1}{3}{/tex}
{tex}\Rightarrow E(X)=\frac{14}{3}{/tex}
Consider the line x + 2y = 8. We observe that the shaded region and the origin are on the same side of the line x + 2y = 8 and (0, 0) satisfies the linear constraint x + 2y {tex}\leq{/tex} 8. So, we must have one inequations as x + 2y {tex}\leq{/tex} 8.

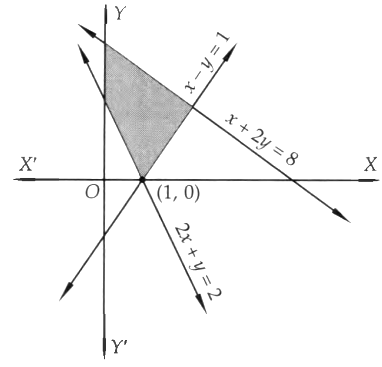
Now, consider the line 2x + y = 2. We find that the shaded region and the origin are on the opposite sides of the line 2x + y = 2 and (0, 0) does not satisfy the inequation 2x + y {tex}\geq{/tex} 2. So, the second inequations is 2x + y {tex}\geq{/tex} 2.
Finally, consider the line x – y = 1. We observe that the shaded region and the origin are on the same side of line x – y = 1. We observe that the shaded region and the origin are on the same side of line x – y = 1 and (0, 0) satisfies x – y {tex}\geq{/tex} 1. So, the third constraint is x – y {tex}\geq{/tex} 1.
We also notice that the shaded region is above x-axis and is on the right side of y-axis. So, we must have x {tex}\geq{/tex} 0 and y {tex}\geq{/tex} 0. Thus, the linear inequations corresponding to the given solution set are
x + 2y {tex}\leq{/tex} 8, 2x + y {tex}\geq{/tex} 2, x – y {tex}\leq{/tex} 1, x {tex}\geq{/tex} 0, y {tex}\leq{/tex} 0
Cost of new machine = ₹ 300000
Scrap value of old machine = ₹ 30000
Hence, the money required for new machine after 7 years
= ₹ 300000 – ₹ 30000 = ₹ 270000
So, we have A = ₹ 270000, i = {tex}\frac{5}{100}{/tex} = 0.05, n = 7
Using formula, A = R{tex}\left[\frac{(1+i)^{n}-1}{i}\right]{/tex}, we get
270000 = R{tex}\left[\frac{(1.05)^{7}-1}{0.05}\right]{/tex}
{tex}\Rightarrow{/tex} R = {tex}\frac{270000 \times 0.05}{(1.05)^{7}-1}{/tex} [Let x = (1.05)⁷{tex}\Rightarrow{/tex} x = 7 log 1.05 = 7 {tex}\times{/tex} 0.0212 = 0.1484 {tex}\Rightarrow{/tex} x = antilog 0.1484 {tex}\Rightarrow{/tex} x = 1.407] {tex}\Rightarrow{/tex} R = {tex}\frac{13500}{1.407-1}=\frac{13500}{0.407}{/tex}
{tex}\Rightarrow{/tex} R = 33169.53
Hence, the company should deposit ₹ 33169.53 at the end of each year for 7 years.

Section E
1. Given, y = tan^-1{tex}\left(\frac{\log \left(\frac{e}{x^{2}}\right)}{\log e x^{2}}\right){/tex} + tan^-1{tex}\left(\frac{3+2 \log x}{1-6 \log x}\right){/tex}
  = tan^-1{tex}\left(\frac{1-\log x^{2}}{1+\log x^{2}}\right){/tex}+ tan^-1{tex}\left(\frac{3+2 \log x}{1-6 \log x}\right){/tex}
  = tan^-1(1) – tan^-1(2log x) + tan^-1(3) + tan^-1(2log x)
  {tex}\Rightarrow{/tex} y = tan^-1(1) + tan^-1(3)
  {tex}\Rightarrow{/tex} {tex}\frac{d y}{d x}{/tex} = 0 {tex}\Rightarrow \frac{d^{2} y}{d x^{2}}{/tex} = 0
2. Given, x = s + 3t, y = 2s – t {tex}\Rightarrow{/tex} {tex}\frac{d x}{d s}=1{/tex},{tex}\frac{d y}{d s}=2 {/tex}
  Now, u = x² + y² {tex}\frac{d u}{d s}=2 x \frac{d x}{d s}+2 y \frac{d y}{d s}{/tex} = 2x + 4y
  {tex}\Rightarrow{/tex}{tex}\frac{d^{2} u}{d s^{2}}=2\left(\frac{d x}{d s}\right)+4\left(\frac{d y}{d s}\right) \Rightarrow \frac{d^{2} u}{d s^{2}}{/tex} = 2(1) + 4(2) = 10
3. We have,f{x) = 2 log sin x
  {tex}\Rightarrow{/tex} f'(x) = 2{tex}\cdot{/tex}{tex}\frac{1}{\sin x}{/tex}{tex}\cdot{/tex}cos x = 2 cos x
  {tex}\Rightarrow{/tex} {tex}f^{\prime \prime}{/tex}(x) = -2 cosec²x
  OR
  We have, f(x) = e^xsinx
  {tex}\Rightarrow{/tex} f'(x) = e^xcosx + e^xsinx = e^x(cosx + sinx)
  {tex}\Rightarrow{/tex} e^x(x) = e^x(cosx – sinx) + e^x(cosx + sinx) = 2e^x cosx
  {tex}\Rightarrow{/tex} f”(x) = 2[e^xcosx – e^xsinx] = 2e^x[cosx – sinx]
1. Cost of new machine = ₹ 300000
  Scrap value of old machine = ₹ 30000
  Hence, the money required for new machine after 7 years
  = ₹ 300000 – ₹ 30000 = ₹ 270000
2. A = ₹ 270000, i = {tex}\frac {5}{100}{/tex} = 0.05, n = 7
3. A = {tex}R\left[\frac{(1+i)^n-1}{i}\right]{/tex}
  OR
  Cost of new machine = ₹300000
  Scrap value of old machine = ₹30000
  Hence, the money required for new machine after 7 years
  = ₹300000 – ₹30000 = ₹270000
  So, we have A = ₹270000, i = {tex}\frac {5}{100}{/tex} = 0.05, n = 7
  Using formula, A = {tex}R\left[\frac{(1+i)^n-1}{i}\right]{/tex}, we get
  270000 = {tex}\mathrm{R}\left[\frac{(1.05)^7-1}{0.05}\right]{/tex}
  [Let x = (1.05)⁷
  {tex}\Rightarrow{/tex} log x = 7 log 1.05 = 7 {tex}\times{/tex} 0.0212 = 0.1484
  {tex}\Rightarrow{/tex} x = antilog 0.1484
  {tex}\Rightarrow{/tex} x = 1.407
  {tex}\Rightarrow R=\frac{270000 \times 0.05}{(1.05)^7-1}{/tex}
  {tex}\Rightarrow R=\frac{13500}{1.407-1}=\frac{13500}{0.407}{/tex}
  {tex}\Rightarrow{/tex} R = 33169.53
  Hence, the company should deposit ₹33169.53 at the end of each year for 7 years.
1. The linear equations representing the given situation are
  3x + 2y = 2300
  5x + 3y = 3700.
  The matrix equation representing these equations is
  {tex}\left[\begin{array}{ll} 3 & 2 \\ 5 & 3 \end{array}\right]{/tex}{tex}\left[\begin{array}{l} x \\ y \end{array}\right]{/tex} = {tex}\left[\begin{array}{l} 2300 \\ 3700 \end{array}\right]{/tex}
2. The determinant of the coefficients of x and y is {tex}\left|\begin{array}{ll}3 & 2 \\ 5 & 3\end{array}\right|{/tex}.
  Its value = 3 {tex}\times{/tex} 3 – 5 {tex}\times{/tex} 2 = -1.
3. D₁ = {tex}\left|\begin{array}{ll} 2300 & 2 \\ 3700 & 3 \end{array}\right|{/tex} = 2300 {tex}\times{/tex} 3 – 3700 {tex}\times{/tex} 2 = -500,
  D₂ = {tex}\left|\begin{array}{ll} 3 & 2300 \\ 5 & 3700 \end{array}\right|{/tex} = 3 {tex}\times{/tex} 3700 – 5 {tex}\times{/tex} 2300 = -400.
  x = {tex}\frac{\mathrm{D}_1}{\mathrm{D}}{/tex} = {tex}\frac{-500}{-1}{/tex} = 500, y = {tex}\frac{\mathrm{D}_2}{\mathrm{D}}{/tex} = {tex}\frac{-400}{-1}{/tex} = 400.
  OR
  |A| = {tex}\left|\begin{array}{ll} 3 & 2 \\ 5 & 3 \end{array}\right|{/tex} = 3 {tex}\times{/tex} 3 – 2 {tex}\times{/tex} 5 = -1.
  A^-1 = {tex}\frac{1}{|\mathrm{~A}|}{/tex} adj A = {tex}\frac{1}{-1}{/tex}{tex}\left[\begin{array}{rr} 3 & -2 \\ -5 & 3 \end{array}\right]{/tex} = {tex}\left[\begin{array}{rr} -3 & 2 \\ 5 & -3 \end{array}\right]{/tex}.
  Download the myCBSEguide App for Comprehensive Exam Preparation Prepare effectively for your exams by downloading the myCBSEguide app, offering complete study material for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams. With access to practice questions, sample papers, and detailed solutions, the app is designed to help you improve your skills and boost your exam performance. Class 12 Applied Maths 2025 students can benefit from our interactive video lessons that break down complex problems into easy-to-understand steps. To perform well in the Class 12 Applied Maths 2025 exam, it’s essential to practice regularly and solve as many problems as possible.
  Examin8 App: Create Customized Exam Papers for Teachers
  Teachers can streamline their exam preparation process with the Examin8 app. This tool allows educators to easily create customized exam papers with their own name, logo, and branding, offering a professional and efficient way to generate assessments tailored to their students’ needs. Stay ahead in your studies with our regularly updated content for Class 12 Applied Maths 2025, keeping you aligned with the latest exam trends and syllabus changes.

Class 12 Sample Papers 2025

You can download sample papers for other subjects as well. These model papers are available for download on myCBSEguide app. Please browse through the resources provided below to access CBSE class 12th Model question papers for all the subjects. We offer downloadable practice papers designed specifically for Class 12 Applied Maths 2025, giving you the perfect opportunity to test your knowledge.

Test Generator

Create question paper PDF and online tests with your own name & logo in minutes.

Create Now

myCBSEguide

Question Bank, Mock Tests, Exam Papers, NCERT Solutions, Sample Papers, Notes