Class 12 Maths sample papers 2025

 Class 12 Maths Sample Papers for 2025

Looking for CBSE Class 12 Mathematics sample papers for the 2024-25 academic year? If you’re preparing for the Class 12 Maths exams in 2025, downloading Class 12 Maths sample papers 2025 is an essential step in your exam preparation. These Class 12 Maths sample papers 2025 are designed to align with the latest CBSE syllabus and exam pattern, providing you with a clear understanding of what to expect. By practicing these Class 12 Maths sample papers 2025, you can get a good grasp of key topics, improve problem-solving skills, and boost your confidence. Additionally, solving these sample papers will help you assess your readiness and identify areas that need more focus. Downloading and practicing Class 12 Maths sample papers 2025 from reliable sources like the myCBSEguide app will ensure you’re fully prepared for the exam.Visit the myCBSEguide app to access high-quality sample papers with 100% genuine solutions in PDF format. These sample papers are carefully designed to help Class 12 students practice and master important concepts, ensuring effective preparation for the 2025 CBSE exam.

If you’re searching for the best CBSE sample papers for Class 12 Mathematics 2025, the myCBSEguide student dashboard is your one-stop solution. It offers free downloadable sample papers, NCERT solutions, chapter-wise practice tests, revision notes, and much more to meet all your study needs.

Start your exam preparation now by downloading the myCBSEguide app or logging into the myCBSEguide student dashboard. Get access to the best Class 12 Maths sample papers and prepare effectively for the 2025 CBSE Mathematics exam.

Sample Papers of Maths Class 12 – Free PDF Download

Download CBSE Class 12 Mathematics Sample Papers for 2025

The CBSE Class 12 Mathematics sample papers for 2025 are now available! With the release of the new CBSE marking scheme and blueprint for the 2025 Class 12 exams, students can now access high-quality sample papers to help them prepare effectively. These sample papers are available for free download in PDF format through the myCBSEguide app and website.

To excel in the Class 12 Maths exams 2025, practicing with Class 12 Maths sample papers 2025 is crucial for understanding the exam format and gaining confidence. These Class 12 Maths sample papers 2025 are designed to give students a clear picture of the types of questions that will appear on the exam and help improve time management skills. By regularly solving these Class 12 Maths sample papers 2025, you can identify your strengths and weaknesses, allowing you to focus on areas that need improvement. Additionally, working through Class 12 Maths sample papers 2025 will familiarize you with the marking scheme, ensuring that you’re fully prepared for every section of the exam. To get started, download the Class 12 Maths sample papers 2025 from trusted platforms like myCBSEguide, which offers updated papers according to the latest syllabus and exam pattern.

CBSE Sample Papers for Class 12 Mathematics come with solutions and are based on the latest CBSE syllabus, marking scheme, and exam blueprint. These sample papers provide a comprehensive preparation strategy and answer the most frequently asked question, “How to prepare for CBSE Class 12 board exams?” With these sample papers, you can practice and familiarize yourself with the exam format, ensuring you are fully prepared for the 2025 CBSE board exams.

Download the myCBSEguide app today to get the latest CBSE sample papers for Class 12 Mathematics with solutions. Start practicing now to enhance your exam preparation and perform well in your upcoming exams!

Sample Papers of Class 12 Maths 2025 with solution

Download as PDF

CBSE Sample Papers Class 12 Mathematics 2025

Class 12 – Mathematics
Sample Paper – 01 (2024-25)

---

Maximum Marks: 80
Time Allowed: : 3 hours

---

General Instructions:

1. This Question paper contains 38 questions. All questions are compulsory.
2. This Question paper is divided into five Sections – A, B, C, D and E.
3. In Section A, Questions no. 1 to 18 are multiple choice questions (MCQs) and Questions no. 19 and 20 are Assertion-Reason based questions of 1 mark each.
4. In Section B, Questions no. 21 to 25 are Very Short Answer (VSA)-type questions, carrying 2 marks each.
5. In Section C, Questions no. 26 to 31 are Short Answer (SA)-type questions, carrying 3 marks each.
6. In Section D, Questions no. 32 to 35 are Long Answer (LA)-type questions, carrying 5 marks each.
7. In Section E, Questions no. 36 to 38 are Case study-based questions, carrying 4 marks each.
8. There is no overall choice. However, an internal choice has been provided in 2 questions in Section B, 3 questions in Section C, 2 questions in Section D and one subpart each in 2 questions of Section E.
9. Use of calculators is not allowed.

---

1. Section A
2. If A = {tex}\left[\begin{array}{ll} 0 & 1 \\ 0 & 0 \end{array}\right]{/tex}, then A²⁰²³ is equal to

   a){tex}\left[\begin{array}{ll} 2023 & 0\\ 0 & 2023 \end{array}\right]{/tex}

   b){tex}\left[\begin{array}{ll} 0 & 1 \\ 0 & 0 \end{array}\right]{/tex}

   c){tex}\left[\begin{array}{ll} 0 & 2023 \\ 0 & 0 \end{array}\right]{/tex}

   d){tex}\left[\begin{array}{ll} 0 & 0 \\ 0 & 0 \end{array}\right]{/tex}

3. If A is a 3{tex}\times{/tex}3 matrix and |A| = -2, then value of |A (adj A)| is

   a)-2

   b)8

   c)2

   d)-8

4. The system of linear equations
   5x + ky = 5,
   3x + 3y = 5;
   will be consistent if:

   a)k = 5

   b)k = -5

   c)k {tex}\neq{/tex} -3

   d)k {tex}\neq{/tex} 5

5. If the matrix {tex}A=\left[\begin{array}{cc} 3-2 x & x+1 \\ 2 & 4 \end{array}\right]{/tex} is singular then x = ?.

   a)1

   b)0

   c)-1

   d)-2

6. The direction ratios of a line parallel to z-axis are:

   a)< 0, 0, 0 >

   b)< 1, 1, 0 >

   c)< 1, 1, 1 >

   d)< 0, 0, 1 >

7. If y = e^-x (A cos x + B sin x), then y is a solution of

   a){tex}\frac{d^{2} y}{d x^{2}}+2 \frac{d y}{d x}+2 y=0{/tex}

   b){tex}\frac{d^{2} y}{d x^{2}}-2 \frac{d y}{d x}+2 y=0{/tex}

   c){tex}\frac{d^{2} y}{d x^{2}}+2 y=0{/tex}

   d){tex}\frac{d^{2} y}{d x^{2}}+2 \frac{d y}{d x}=0{/tex}

8. In an LPP, if the objective function z = ax + by has the same maximum value on two corner points of the feasible region, then the number of points at which z_max occurs is:

   a)finite

   b)0

   c)infinite

   d)2

9. In {tex}\triangle\text {ABC}{/tex}, {tex}\overrightarrow{\mathrm{AB}}{/tex} = {tex}\hat {\text i} + \hat {\text j} + 2\hat {\text k}{/tex} and {tex}\overrightarrow{\mathrm{AC}}{/tex} = {tex}3\hat {\text i} – \hat {\text j} + 4\hat {\text k}{/tex}. If D is mid-point of BC, then vector {tex}\overrightarrow{\mathrm{AD}}{/tex} is equal to:

   a){tex}\hat{\text i}-\hat{\text j}+\hat{\text k}{/tex}

   b){tex}2 \hat{\text i}-2 \hat{\text j}+2 \hat{\text k}{/tex}

   c){tex}4 \hat{\text i}+6 \hat{\text k}{/tex}

   d){tex}2 \hat{\text i}+3 \hat{\text k}{/tex}

10. {tex}\int\limits_{0}^\frac{\pi}{8} {/tex} tan² (2x) is equal to

    a){tex}\frac{4+\pi}{8}{/tex}

    b){tex}\frac{4-\pi}{8}{/tex}

    c){tex}\frac{4-\pi}{2} {/tex}

    d){tex}\frac{4-\pi}{4} {/tex}

11. If A = {tex}\left[\begin{array}{ll} 1 & 0 \\ 0 & 0 \end{array}\right]{/tex} and B = {tex}\left[\begin{array}{ll} 1 & 1 \\ 0 & 0 \end{array}\right]{/tex}, then B’A’ is equal to:

    a){tex}\left[\begin{array}{ll} 1 & 1 \\ 0 & 0 \end{array}\right]{/tex}

    b){tex}\left[\begin{array}{ll} 1 & 1 \\ 1 & 1 \end{array}\right]{/tex}

    c){tex}\left[\begin{array}{ll} 1 & 0 \\ 1 & 0 \end{array}\right]{/tex}

    d){tex}\left[\begin{array}{ll} 0 & 0 \\ 0 & 0 \end{array}\right]{/tex}

12. Objective function of an LPP is

    a)a function to be optimized

    b)a function between the variables

    c)a constraint

    d)a relation between the variables

13. If the angle between the vectors {tex}\vec {\text a}{/tex} and {tex}\vec {\text b}{/tex} is {tex}\frac \pi 4{/tex} and {tex}|\vec{a} \times \vec{b}|{/tex} = 1, then {tex}\vec{a} \cdot \vec{b}{/tex} is equal to

    a)-1

    b){tex}\frac{1}{\sqrt{2}}{/tex}

    c){tex}\sqrt{2}{/tex}

    d)1

14. Find the area of the triangle with vertices (0,0), (4,2), and (1,1).

    a)1 sq.unit

    b)2 sq.unit

    c)0 sq.unit

    d)5 sq.unit

15. For any two events A and B, if {tex}\text P(\bar {\text A}){/tex} = {tex}\frac 12{/tex}, {tex}\text P(\bar {\text B}){/tex} = {tex}\frac 23{/tex} and P(A {tex}\cap{/tex} B) = {tex}\frac 14{/tex}, then {tex}\mathrm{P}\left(\frac{\overline{\mathrm{A}}}{\overline{\mathrm{B}}}\right){/tex} equals:

    a){tex}\frac 14{/tex}

    b){tex}\frac {3}{8}{/tex}

    c){tex}\frac 89{/tex}

    d){tex}\frac 18{/tex}

16. Which of the following transformations reduce the differential equation {tex}\frac{d z}{d x}+\frac{z}{x} \log z=\frac{z}{x^{2}}(\log z)^{2}{/tex} into the form {tex}\frac{d u}{d x}+P(x) u=Q(x){/tex}

    a)u = e^x

    b)u = log x

    c)u = (log z)²

    d)u = (log z)^-1

    Download myCBSEguide App for Comprehensive Exam Preparation

    To practice more questions and boost your exam preparation, download the myCBSEguide app. It provides complete study material for CBSE, NCERT, and competitive exams like JEE (Main), NEET-UG, and NDA. With access to sample papers, chapter-wise tests, NCERT solutions, and revision notes, myCBSEguide ensures that you’re fully equipped to succeed in your exams.

    For teachers, the Examin8.com app offers a convenient platform to create customized question papers with your own name and logo. This tool is ideal for teachers who want to create unique practice papers for their students, based on their specific syllabus and requirements.

    Start your exam preparation today by downloading the myCBSEguide app or visiting the myCBSEguide website and Examin8 website. Whether you’re a student or a teacher, myCBSEguide offers all the resources you need to excel.

    ABCD is a rhombus whose diagonals intersect at E. Then {tex}\vec{\mathrm{EA}}+\vec{\mathrm{EB}}+\vec{\mathrm{EC}}+ \vec{\mathrm{ED}}{/tex} equals

    a){tex}\vec 0{/tex}

    b){tex}\vec {\text {AD}}{/tex}

    c){tex}2\vec {\text {AD}}{/tex}

    d){tex}2\vec {\text {BC}}{/tex}

17. If {tex}y=\sqrt{\sin x+\sqrt{\sin x+\sqrt{\sin x+}}} \ldots \infty{/tex} then {tex}\frac {dy }{dx}{/tex} = ?

    a){tex}\frac{\sin x}{(2 y-1)}{/tex}

    b){tex}\frac{\cos x}{(y-1)}{/tex}

    c){tex}\frac{\cos x}{(2 y-1)}{/tex}

    d){tex}\frac{\sin x}{(2 y+1)}{/tex}

18. The direction ratios of two lines are a, b, c and (b – c), (c – a), (a – b) respectively. The angle between these lines is

    a){tex}\frac{\pi}{2}{/tex}

    b){tex}\frac{\pi}{4}{/tex}

    c){tex}\frac{\pi}{3}{/tex}

    d){tex}\frac{3 \pi}{4}{/tex}

19. Assertion (A): If two positive numbers are such that sum is 16 and sum of their cubes is minimum, then numbers are 8, 8.
    Reason (R): If f be a function defined on an interval I and c {tex}\in{/tex} l and let f be twice differentiable at c, then x = c is a point of local minima if f'(c) = 0 and f”(c) > 0 and f(c) is local minimum value of f.

    a)Both A and R are true and R is the correct explanation of A.

    b)Both A and R are true but R is not the correct explanation of A.

    c)A is true but R is false.

    d)A is false but R is true.

20. Assertion (A): A function f: N {tex}\to{/tex} N be defined by {tex}\ f(n) = \begin{cases} \frac n2 & \quad \text{if } n \text{ is even}\\ \frac {(n+1)}2 & \quad \text{if } n \text{ is odd} \end{cases} {/tex}  for all n {tex}\in{/tex} N; is one-one.
    Reason (R): A function f: A {tex}\to{/tex} B is said to be injective if a {tex}\not={/tex} b then f(a) {tex}\not={/tex} f(b).

    a)Both A and R are true and R is the correct explanation of A.

    b)Both A and R are true but R is not the correct explanation of A.

    c)A is true but R is false.

    d)A is false but R is true.

21. Section B
22. Find the value of {tex}\cos ^{-1} \frac{1}{2}+2 \sin ^{-1} \frac{1}{2}{/tex}.

    OR

    Find the value of {tex}{\tan ^{ – 1}}\left( { – \frac{1}{{\sqrt 3 }}} \right) + {\cot ^{ – 1}}\left( {\frac{1}{{\sqrt 3 }}} \right) + {\tan ^{ – 1}}\left[ {\sin \left( {\frac{{ – \pi }}{2}} \right)} \right]{/tex}.

23. Find the absolute maximum and minimum values of the function f given by f(x) = cos² x + sin x, x {tex}\in{/tex} [0, {tex}\pi{/tex}]
24. Find the rate of change of the area of a circle with respect to its radius r when
    1. r = 3 cm
    2. r = 4 cm

    OR

    Find the intervals of function f(x) = (x – 1)(x – 2)² is

    1. increasing
    2. decreasing.
25. Evaluate: {tex}\int_{1}^{4} f(x) d x{/tex}, where f(x) =|x – 1| + |x – 2| + |x – 3|
26. A ladder 13 m long is leaning against a vertical wall. The bottom of the ladder is dragged away from the wall along the ground at the rate of 2 cm/sec. How fast is the height on the wall decreasing when the foot of the ladder is 5 m away from the wall?
27. Section C
28. Evaluate {tex} \int \frac { 2 x + 5 } { \sqrt { 7 – 6 x – x ^ { 2 } } } d x.{/tex}
29. There are three coins. One is a coin having tails on both faces, another is a biased coin that comes up tails 70% of the time and the third is an unbiased coin. One of the coins is chosen at random and tossed, it shows tail. Find the probability that it was a coin with tail on both the faces.
30. Evaluate the definite integral {tex} \int _ { 1 } ^ { 2 } \frac { 5 x ^ { 2 } } { x ^ { 2 } + 4 x + 3 }{/tex}

    OR

    Evaluate: {tex}\int \limits_{-2}^1 \sqrt{5-4 x-x^2} \mathrm{~d} x{/tex}

31. Find the particular solution of the differential equation {tex}\left[x \sin ^{2}\left(\frac{y}{x}\right)-y\right]{/tex}dx + x dy = 0, given that y = {tex}\frac{\pi}{4}{/tex}when x = 1

    OR

    Find the general solution of {tex}x \log x \frac{d y}{d x}+y=\frac{2}{x} \log x{/tex}

32. Find the Maximum and Minimum value of 2x + y
    Subject to the constraints:
    x + 3y {tex}\ge{/tex} 6,
    x – 3y {tex}\ge{/tex} 3,
    3x + 4y {tex}\le{/tex} 24,
    – 3x + 2y {tex}\le{/tex} 6,
    5x + y {tex}\ge{/tex} 5,
    where non-negative restrictions are x, y {tex}\ge{/tex} 0.

    OR

    Solved the linear programming problem graphically:
    Maximize Z = 60x + 15y
    Subject to constraints
    x + y {tex}\le{/tex} 50
    3x + y {tex}\le{/tex} 90
    x, y {tex}\ge{/tex} 0

33. Find the values of a and b so that the function f given by {tex}f(x)=\left\{\begin{aligned} 1, & \text { if } x \leq 3 \\ a x+b, & \text { if } 3<x<5 \\ 7, & \text { if } x \geq 5 \end{aligned}\right.{/tex} is continuous at x = 3 and x = 5
34. Section D
35. Using integration, find the area of the triangle formed by positive X-axis and tangent and normal to the circle x² + y² = 4 at (1, {tex}\sqrt3{/tex}).
36. Show that the function f : R {tex}\rightarrow{/tex} {x {tex}\in{/tex} R : -1 < x < 1} defined by {tex}f(x) = \frac{x}{{1 + |x|}}{/tex}, x {tex}\in{/tex} R is one-one and onto function.

    OR

    ​Let N be the set of all natural numbers and let R be a relation on N {tex}\times{/tex} N, defined by
    {a, b) R (c, d) {tex}\Leftrightarrow{/tex} ad = bc for all (a, b), (c, d) {tex}\in{/tex} N {tex}\times{/tex} N
    Show that R is an equivalence relation on N {tex}\times{/tex} N. Also, find the equivalence class [(2, 6)]

37. If {tex}A = \left[ {\begin{array}{*{20}{c}} 2&{ – 3}&5 \\ 3&2&{ – 4} \\ 1&1&{ – 2} \end{array}} \right]{/tex}, find A^-1. Using A^-1 solve the system of equations 2x – 3y + 5z = 11; 3x + 2y – 4z = -5; x + y – 2z = -3
38. Show that the straight lines whose direction cosines are given by the equations al + bm + cn = 0 and ul² + vm² + wn² = 0 are perpendicular, if
    a² (v + w) + b² (u + w) + c² (u + v) = 0 and, parallel, if {tex}\frac{a^{2}}{u}+\frac{b^{2}}{v}+\frac{c^{2}}{w}{/tex} = 0

    OR

    Find the shortest distance between the lines l₁ and l₂ whose vector equations are
    {tex}\vec r = \hat i + \hat j + \lambda (2\hat i – \hat j + \hat k){/tex} …(1)
    and {tex}\vec r = 2\hat i + \hat j – \hat k + \mu (3\hat i – 5\hat j + 2\hat k){/tex} …(2)

39. Section E
40. Read the following text carefully and answer the questions that follow:
    Akshat and his friend Aditya were playing the snake and ladder game. They had their own dice to play the game. Akshat was having red dice whereas Aditya had black dice. In the beginning, they were using their own dice to play the game. But then they decided to make it faster and started playing with two dice together.
    
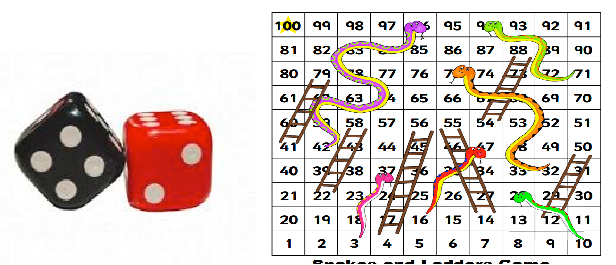
    Aditya rolled down both black and red die together.
    First die is black and second is red.
    1. Find the conditional probability of obtaining a sum greater than 9, given that the black die resulted in a 5. (1)
    2. Find the conditional probability of obtaining the sum 8, given that the red die resulted in a number less than 4. (1)
    3. Find the conditional probability of obtaining the sum 10, given that the black die resulted in even number. (2)
       OR
       Find the conditional probability of obtaining the doublet, given that the red die resulted in a number more than 4. (2)
41. Read the following text carefully and answer the questions that follow:
    The slogans on chart papers are to be placed on a school bulletin board at the points A, B and C displaying A (follow Rules), B (Respect your elders) and C (Be a good human). The coordinates of these points are (1, 4, 2), (3, -3, -2) and (-2, 2, 6), respectively.
    
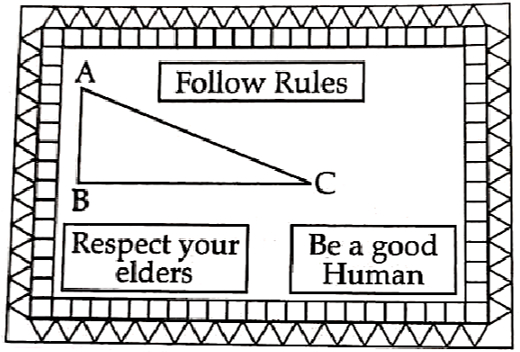
    1. If {tex}\vec a{/tex}, {tex}\vec b{/tex} and {tex}\vec c{/tex} be the position vectors of points A, B, C, respectively, then find {tex}|\vec{a}+\vec{b}+\vec{c}|{/tex}. (1)
    2. If {tex}\vec{a}=4 \hat{i}+6 \hat{j}+12 \hat{k}{/tex}, then find the unit vector in direction of {tex}\vec a{/tex}. (1)
    3. Find area of {tex}\triangle{/tex}ABC. (2)
       OR
       Write the triangle law of addition for {tex}\triangle{/tex}ABC. Suppose, if the given slogans are to be placed on a straight line, then the value of {tex}|\vec{a} \times \vec{b}+\vec{b} \times \vec{c}+\vec{c} \times \vec{a}|{/tex}. (2)
42. Read the following text carefully and answer the questions that follow:
    A gardener wants to construct a rectangular bed of garden in a circular patch of land. He takes the maximum perimeter of the rectangular region as possible. (Refer to the images given below for calculations)
    
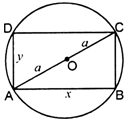
    1. Find the perimeter of rectangle in terms of any one side and radius of circle. (1)
    2. Find critical points to maximize the perimeter of rectangle? (1)
    3. Check for maximum or minimum value of perimeter at critical point. (2)
       OR
       If a rectangle of the maximum perimeter which can be inscribed in a circle of radius 10 cm is square, then the perimeter of region. (2)

       Download myCBSEguide App for Effective Exam Preparation

       For comprehensive exam preparation, download the myCBSEguide app. It offers complete study material for CBSE, NCERT, and top competitive exams like JEE (Main), NEET-UG, and NDA. The app provides access to sample papers, chapter-wise practice tests, NCERT solutions, revision notes, and important questions, ensuring you are fully prepared for your exams.If you’re a teacher, the Examin8 app is an excellent tool for creating customized question papers. With Examin8, you can design papers with your own name, logo, and content to suit your students’ needs.Download the myCBSEguide app now and start preparing for success in your CBSE, JEE, NEET, or NDA exams. For teachers,  visit myCBSEguide Website and Examin8 Website provides an easy and efficient way to generate tailored question papers.

Class 12 – Mathematics
Sample Paper – 01 (2024-25)

---

Solution

1. 1. Section A
   2. (d)

      {tex}\left[\begin{array}{ll} 0 & 0 \\ 0 & 0 \end{array}\right]{/tex}

      Explanation: {tex}\left[\begin{array}{ll} 0 & 0 \\ 0 & 0 \end{array}\right]{/tex}

   3. (d)

      -8

      Explanation: -8

   4. (d)

      k {tex}\neq{/tex} 5

      Explanation: We have, 5x + ky – 5 = 0
      and 3x + 3y – 5 = 0
      For consistent system
      {tex}\begin{equation} \frac{5}{3} \neq \frac{k}{3} \end{equation}{/tex}
      {tex}\Rightarrow{/tex} k {tex}\neq{/tex} 5

   5. (a)

      1

      Explanation: When a given matrix is singular then the given matrix determinant is 0.
      |A| = 0
      Given, {tex}A=\left(\begin{array}{cc} 3-2 x & x+1 \\ 2 & 4 \end{array}\right) {/tex}
      |A| = 0
      4(3 – 2x) – 2(x + 1) = 0
      12 – 8x – 2x – 2 = 0
      10 – 10x = 0
      10(1 – x) = 0
      x = 1

   6. (d)

      < 0, 0, 1 >

      Explanation: < 0, 0, 1 >

   7. (a)

      {tex}\frac{d^{2} y}{d x^{2}}+2 \frac{d y}{d x}+2 y=0{/tex}

      Explanation: Given that, y = e^-x (A cos x + sin x)
      Differentiating both sides w.r.t. x we get
      {tex}\frac{dy}{dx}{/tex} = -e^-x (A cos x + B sin x) + e^-x (-A sin x + B cos x)
      {tex}\frac{dy}{dx}{/tex} = -y + e^x (-A sin x + B cos x)
      Again, differentiating both sides w.r.t. x, we get
      {tex}\frac{d^{2} y}{d x^{2}}=-\frac{d y}{d x}{/tex} + e^-x (-A cos x – B sin x) – e^-x(-A sin x + b cos x)
      {tex}\Rightarrow \frac{d^{2} y}{d x^{2}}=-\frac{d y}{d x}-y-\left[\frac{d y}{d x}+y\right]{/tex}
      {tex}\Rightarrow \frac{d^{2} y}{d x^{2}}=-2 \frac{d y}{d x}-2 y{/tex}
      {tex}\Rightarrow \frac{d^{2} y}{d x^{2}}+2 \frac{d y}{d x}+2 y=0{/tex}

   8. (c)

      infinite

      Explanation: In a LPP, if the objective fⁿ Z = ax + by has the maximum value on two corner point of the feasible region then every point on the line segment joining these two points gives the same maximum value.
      hence, Z_max occurs at infinite no of times.

   9. (d)

      {tex}2 \hat{\text i}+3 \hat{\text k}{/tex}

      Explanation: {tex}2 \hat{\text i}+3 \hat{\text k}{/tex}

   10. (b)

       {tex}\frac{4-\pi}{8}{/tex}

       Explanation: I = {tex}\int_{0}^\frac{\pi}{8} {/tex} tan²(2 x) dx
       = {tex}\int_{0}^\frac{\pi}{8} {/tex}[sec² (2x) – 1]dx
       = {tex}[\frac {\tan 2x}2 – x]^{\frac {\pi}8}_0{/tex}
       = {tex}\left[\frac {\tan \frac {\pi}4}2 – \frac {\pi}8-\frac {\tan 0}2 – 0\right]{/tex}
       = {tex}\frac 12-\frac {\pi}8{/tex}
       = {tex}\frac{4-\pi}{8}{/tex}

   11. (c)

       {tex}\left[\begin{array}{ll} 1 & 0 \\ 1 & 0 \end{array}\right]{/tex}

       Explanation: {tex}\left[\begin{array}{ll} 1 & 0 \\ 1 & 0 \end{array}\right]{/tex}

   12. (a)

       a function to be optimized

       Explanation: a function to be optimized
       The objective function of a linear programming problem is either to be maximized or minimized i.e. objective function is to be optimized.

   13. (d)

       1

       Explanation: 1

   14. (a)

       1 sq.unit

       Explanation: {tex}\frac{1}{2}\left| {\begin{array}{*{20}{c}} 0&0&1 \\ 4&2&1 \\ 1&1&1 \end{array}} \right| = \frac{1}{2}[ 1(4 – 2)] = \frac{1}{2}(2) = 1{/tex}

   15. (b)

       {tex}\frac {3}{8}{/tex}

       Explanation: {tex}\frac {3}{8}{/tex}

   16. (d)

       u = (log z)^-1

       Explanation: We have ,

       {tex}\frac{d z}{d x}+\frac{z}{x} \log z=\frac{z}{x^{2}}(\log z)^{2}{/tex}
       {tex}\frac{d z}{d x}=\frac{z}{x^{2}}(\log z)^{2}-\frac{z}{x} \log z{/tex} ….(i)
       Put v = (logz)^-1
       {tex}\frac{d v}{d x}=\frac{-1}{(\log z)^{2}} \frac{1}{z} \frac{d z}{d x}{/tex}
       {tex}\frac{d z}{d x}=-z(\log z)^{2} \frac{d v}{d x}{/tex} ….(ii)
       From (i) and (ii)
       {tex}-z(\log z)^{2} \frac{d v}{d x}=\frac{z}{x^{2}}(\log z)^{2}-\frac{z}{x} \log z{/tex}
       {tex}(\log z) \frac{d v}{d x}=-\frac{1}{x^{2}}(\log z)+\frac{1}{x}{/tex}
       {tex}\frac{d v}{d x}=-\frac{1}{x^{2}}+\frac{u}{x}{/tex}
       {tex}\frac{d v}{d x}-\frac{u}{x}=-\frac{1}{x^{2}}{/tex}
       P(x) = {tex}-1 \over x{/tex}, q (x) = {tex}-1 \over x^2{/tex}
       Given differential equation can be reduced.
       using v = (log z)^-1

   17. (a)

       {tex}\vec 0{/tex}

       Explanation:ABCD is a rhombus and its diagonal intersects at E.
       
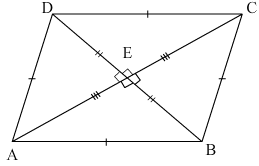
       According to the properties of rhombus,
       {tex}|\vec{\mathrm{AB}}|=|\vec{\mathrm{BC}}|=|\vec{\mathrm{CD}}|=|\vec{\mathrm{DA}}|{/tex}
       {tex}\vec{\mathrm{EA}}=-\vec{\mathrm{EC}}{/tex}
       {tex}\vec{\mathrm{ED}}=-\vec{\mathrm{EB}}{/tex}
       Consider the given expression {tex}\vec{\mathrm{EA}}+\vec{\mathrm{EB}}+\vec{\mathrm{EC}}+\vec{\mathrm{ED}}{/tex}
       {tex}=-\vec{\mathrm{EC}}+\vec{\mathrm{EB}}+\vec{\mathrm{EC}}-\vec{\mathrm{EB}}{/tex} ……({tex}\because\vec{\mathrm{EA}}=-\vec{\mathrm{EC}}{/tex} and {tex}\vec{\mathrm{ED}}=-\vec{\mathrm{EB}}{/tex})
       = 0
       Hence, the correct answer is 0.

       Boost Your Exam Preparation with the myCBSEguide App

       Ready to practice more and prepare efficiently for your exams? Download the myCBSEguide app today for access to comprehensive study material for CBSE, NCERT, and key entrance exams such as JEE (Main), NEET-UG, and NDA. Whether you’re looking for sample papers, chapter-wise practice tests, NCERT solutions, or important question papers, the myCBSEguide app offers everything you need to excel in your exams. If you’re aiming for success in the Class 12 Maths exams 2025, practicing with Class 12 Maths sample papers 2025 is essential. By regularly solving Class 12 Maths sample papers 2025, students can improve their speed and accuracy, which is crucial for scoring well. Moreover, Class 12 Maths sample papers 2025 are an excellent tool for identifying your strengths and weaknesses, allowing you to tailor your study plan effectively. To make your preparation even more comprehensive, download the best Class 12 Maths sample papers 2025 from trusted platforms like the myCBSEguide app, which provides high-quality papers with solutions to ensure thorough revision.

       For teachers, the Examin8.com app allows you to easily create customized question papers with your name and logo. This app is perfect for crafting personalized practice papers and assessments for your students.

       Start preparing smarter today—download the myCBSEguide app and explore a wide range of resources to help you succeed in your CBSE, NEET, JEE, or NDA exams. Teachers can also use Examin8 Website and myCBSEGuide Website to create tailored exam papers that fit their specific syllabus and requirements.

       (c)

   18. {tex}\frac{\cos x}{(2 y-1)}{/tex}

       Explanation: Given:
       {tex}\Rightarrow y=\sqrt{\sin x+\sqrt{\sin x+\sqrt{\sin x+}}}{/tex}
       We can write it as
       {tex}\Rightarrow y=\sqrt{\sin x+y}{/tex}
       Squaring we get
       {tex}\Rightarrow{/tex} y² = sin x + y
       Differentiating with respect to x,we get
       {tex}\Rightarrow 2 y \frac{d y}{d x}=\cos x+\frac{d y}{d x}{/tex}
       {tex}\Rightarrow \frac{d y}{d x}=\frac{\cos x}{(2 y-1)}{/tex}

   19. (a)

       {tex}\frac{\pi}{2}{/tex}

       Explanation:  Let’s consider the first parallel vector to be {tex}\vec{a}=a \hat{i}+b \hat{j}+c \hat{k}{/tex} and second parallel vector be {tex}\overrightarrow{\mathrm{b}}=(\mathrm{b}-\mathrm{c}) \hat{\mathrm{i}}+(\mathrm{c}-\mathrm{a}) \hat{\mathrm{j}}+(\mathrm{a}-\mathrm{b}) \hat{\mathrm{k}}{/tex}
       For the angle, we can use the formula {tex}\cos \alpha=\frac{\vec{a} \cdot \vec{b}}{|\vec{a}| \times|\vec{b}|}{/tex}
       For that, we need to find the magnitude of these vectors
       {tex}|\vec{a}|=\sqrt{a^{2}+b^{2}+(c)^{2}}{/tex}
       {tex}=\sqrt{a^{2}+b^{2}+c^{2}}{/tex}
       {tex}|\overrightarrow{\mathrm{b}}|=\sqrt{(\mathrm{b}-\mathrm{c})^{2}+(\mathrm{c}-\mathrm{a})^{2}+(\mathrm{a}-\mathrm{b})^{2}}{/tex}
       {tex}=\sqrt{2\left(a^{2}+b^{2}+c^{2}-a b-b c-c a\right)}{/tex}
       {tex}\Rightarrow{/tex} {tex}\cos \alpha=\frac{(a \hat{\imath}+b \hat{\jmath}+c \hat{k}) \cdot((b-c) \hat{\imath}+(c-a) \hat{\jmath}+(a-b) \hat{k})}{\sqrt{2\left(a^{2}+b^{2}+c^{2}-a b-b c-c a\right)} \times \sqrt{a^{2}+b^{2}+c^{2}}}{/tex}
       {tex}\Rightarrow{/tex} {tex}\cos \alpha=\frac{a b-a c+b c-b a+c a-c b}{\sqrt{2\left(a^{2}+b^{2}+c^{2}-a b-b c-c a\right)} \times \sqrt{a^{2}+b^{2}+c^{2}}}{/tex}
       {tex}\Rightarrow{/tex} {tex}\cos \alpha=\frac{0}{\sqrt{2\left(a^{2}+b^{2}+c^{2}-a b-b c-c a\right)} \times \sqrt{a^{2}+b^{2}+c^{2}}}{/tex}
       {tex}\Rightarrow{/tex} {tex}\alpha=\cos ^{-1}(0){/tex}
       {tex}\therefore{/tex} {tex}\alpha=\frac{\pi}{2}{/tex}

   20. (a)

       Both A and R are true and R is the correct explanation of A.

       Explanation: Let one number be x, then the other number will be (16 – x).
       Let the sum of the cubes of these numbers be denoted by S.
       Then, S = x³ + (16 – x)³
       On differentiating w.r.t. x, we get
       {tex}\frac{d S}{d x}{/tex} = 3x² + 3(16 – x)²(-1)
       = 3x² – 3(16 – x)²
       {tex}\Rightarrow \frac{d^2 S}{d x^2}{/tex} = 6x + 6(16 – x) = 96
       For minima put {tex}\frac {dS}{dx}{/tex} = 0.
       {tex}\therefore{/tex} 3x² – 3(16 – x)² = 0
       {tex}\Rightarrow{/tex} x² – (256 + x² – 32x) = 0
       {tex}\Rightarrow{/tex} 32x = 256
       {tex}\Rightarrow{/tex} x = 8
       At x = 8, {tex}\left(\frac{d^2 S}{d x^2}\right)_{x=8}{/tex} = 96 > 0
       By second derivative test, x = 8 is the point of local minima of S.
       Thus, the sum of the cubes of the numbers is the minimum when the numbers are 8 and 16 – 8 = 8
       Hence, the required numbers are 8 and 8.

   21. (d)

       A is false but R is true.

       Explanation: Assertion is false because distinct elements in N has equal images.
       for example f(1) = {tex}\frac {(1+1)}{2}{/tex} = 1
       f(2) = {tex}\frac {2}{2}{/tex} = 1
       Reason is true because for injective function if elements are not equal then their images should be unequal.

   22. Section B
   23. Let {tex}\cos ^{-1}\left(\frac{1}{2}\right)=x{/tex}. Then, {tex}\cos x=\frac{1}{2}=\cos \left(\frac{\pi}{3}\right){/tex}.
       {tex}\therefore \cos ^{-1}\left(\frac{1}{2}\right)=\frac{\pi}{3}{/tex}
       Let {tex}\sin ^{-1}\left(\frac{1}{2}\right)=y{/tex}. Then, {tex}\sin y=\frac{1}{2}=\sin \left(\frac{\pi}{6}\right){/tex}.
       {tex}\therefore \sin ^{-1}\left(\frac{1}{2}\right)=\frac{\pi}{6}{/tex}
       {tex}\therefore \cos ^{-1}\left(\frac{1}{2}\right)+2 \sin ^{-1}\left(\frac{1}{2}\right){/tex} {tex}=\frac{\pi}{3}+\frac{2 \pi}{6}=\frac{\pi}{3}+\frac{\pi}{3}=\frac{2 \pi}{3}{/tex}

       OR

       We have, {tex}{\tan ^{ – 1}}\left( { – \frac{1}{{\sqrt 3 }}} \right) + {\cot ^{ – 1}}\left( {\frac{1}{{\sqrt 3 }}} \right) + {\tan ^{ – 1}}\left[ {\sin \left( {\frac{{ – \pi }}{2}} \right)} \right]{/tex}.
       {tex} = {\tan ^{ – 1}}\left( {\tan \frac{{5\pi }}{6}} \right) + {\cot ^{ – 1}}\left( {\cot \frac{\pi }{3}} \right) + {\tan ^{ – 1}}( – 1){/tex}.
       {tex}= {\tan ^{ – 1}}\left[ {\tan \left( {\pi – \frac{\pi }{6}} \right)} \right] + {\cot ^{ – 1}}\left[ {\cot \left( {\frac{\pi }{3}} \right)} \right]{/tex}{tex}+ ta{n^{ – 1}}\left[ {\tan \left( {\pi – \frac{\pi }{4}} \right)} \right]{/tex}
       {tex}= {\tan ^{ – 1}}\left( { – \tan \frac{\pi }{6}} \right) + {\cot ^{ – 1}}\left( {\cot \frac{\pi }{3}} \right) + {\tan ^{ – 1}}{/tex}{tex}\left( { – \tan \frac{\pi }{4}} \right){/tex} {tex}\left[ \begin{gathered} \because {\tan ^{ – 1}}\left( {\tan x} \right) = x,x \in \left( { – \frac{\pi }{2},\frac{\pi }{2}} \right) \hfill \\ {\cot ^{ – 1}}(\cot x) = x,x \in (0,\;\pi ) \hfill \\ and\;{\tan ^{ – 1}}( – x) = – {\tan ^{ – 1}}x \hfill \\ \end{gathered} \right]{/tex}
       {tex}= – \frac{\pi }{6} + \frac{\pi }{3} – \frac{\pi }{4} = \frac{{ – 2\pi + 4\pi – 3\pi }}{{12}}{/tex}
       {tex} = \frac{{ – 5\pi + 4\pi }}{{12}} = \frac{{ – \pi }}{{12}}{/tex}

   24. It is given that f(x) = cos² x + sin x, {tex}x \in[0, \pi]{/tex}
       {tex}f^\prime{/tex}(x) = 2 cos x (-sin x) + cos x
       = -2 sin x cos x + cos x
       Now, if {tex}f^\prime{/tex}(x) = 0
       {tex}\Rightarrow{/tex} 2 sin x cos x = cos x
       {tex}\Rightarrow{/tex} cos x (2 sin x – 1) = 0
       {tex}\Rightarrow{/tex} sin x = {tex}\frac{1}{2}{/tex} or cos x = 0
       {tex}\Rightarrow{/tex} {tex}x=\frac{\pi}{6},\frac{5\pi}{6}{/tex} or {tex}\frac{\pi}{2}{/tex} as {tex}\mathrm{x} \in[0, \pi]{/tex}
       Next, evaluating the value of f at critical points {tex}x=\frac{\pi}{2}{/tex} and {tex}x=\frac{\pi}{6}{/tex} and at the end points of the interval {tex}[0, \pi]{/tex}, (i.e. at x = 0 and {tex}x=\pi{/tex} ), we get,
       {tex}f\left(\frac{\pi}{6}\right)=\cos ^{2} \frac{\pi}{6}+\sin \frac{\pi}{6}=\left(\frac{\sqrt{3}}{2}\right)^{2}+\frac{1}{2}=\frac{5}{4}{/tex}
       {tex}f(\frac{5\pi}{6}) = cos^2(\frac{5\pi}{6})+ sin(\frac{5\pi}{6}) = cos^2(\pi-\frac\pi6)+sin(\pi-\frac\pi6)=cos^2\frac\pi6-sin\frac\pi6=\frac54{/tex}
       f(0) = cos² 0 + sin 0 = 1 + 0 = 1
       {tex}f(\pi)=\cos ^{2} \pi+\sin \pi{/tex} = (-1)² + 0 = 1
       {tex}f\left(\frac{\pi}{2}\right)=\cos ^{2} \frac{\pi}{2}+\sin \frac{\pi}{2}=0+1=1{/tex}
       Therefore, the absolute maximum value of f is {tex}\frac{5}{4}{/tex} occurring at x = {tex}\frac{\pi}{6}{/tex} and the absolute minimum value of f is 1 occurring at x = 1, {tex}\frac{\pi}{2}{/tex} and {tex}\pi{/tex}.
   25. Let x denote the area of the circle of variable radius r.{tex}\because{/tex}Area of circle {tex}\left( x \right) = \pi {r^2}{/tex}{tex}\therefore {/tex} Rate of change of area x w.r.t. r{tex}\frac{{dx}}{{dr}} = \pi \left( {2r} \right) = 2\pi r{/tex}
       1. When r = 3 cm, then
          {tex}\frac{{dx}}{{dr}} = 2\pi \left( 3 \right) = 6\pi c{m^2}/\sec {/tex}
       2. When r = 4 cm, then
          {tex}\frac{{dx}}{{dr}} = 2\pi \left( 4 \right) = 8\pi c{m^2}/\sec {/tex}

       OR

       Given function is f(x) = (x – 1)(x – 2)² = x² – 4x + 4 (x – 1)
       = x³ – 4x² + 4x – x² + 4x – 4
       f(x) = x³ – 5x² + 8x – 4
       f’(x) = 3x² – 10x + 8
       f’(x) = 3x² – 6x – 4x + 8
       = 3x(x – 2) -4(x – 2)
       = (3x – 4)(x – 2)
       
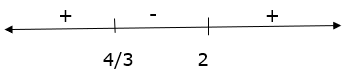
       Function f(x) is decreasing for x{tex}\in{/tex}[4/3, 2] and increasing in x{tex}\in{/tex}{tex}(-\infty, 4 / 3) \cup(2, \infty){/tex}.

   26. Let y = {tex}\int_{1}^{4} f(x) d x{/tex}, then we have
       {tex}y=\int_{1}^{2} f(x) d x+\int_{2}^{3} f(x) d x+\int_{3}^{4} f(x) d x{/tex}
       {tex}=\int_{1}^{2}[(x-1)-(x-2)-(x-3)] d x{/tex} {tex}+\int_{2}^{3}\{(x-1)+(x-2)-(x-3)\} d x{/tex}{tex}+\int_{3}^{4}\{(x-1)+(x-2)+(x-3)\} d x{/tex}
       {tex}=\int_{1}^{2}(-x+4) d x+\int_{2}^{3} x d x+\int_{3}^{4}(3 x-6) d x{/tex}
       {tex}=\left[\frac{-x^{2}}{2}+4 x\right]_{1}^{2}+\left[\frac{x^{2}}{2}\right]_{2}^{3}+\left[\frac{3 x^{2}}{2}-6 x\right]_{3}^{4}{/tex} {tex}=\left(\frac{5}{2}+\frac{5}{2}+\frac{9}{2}\right)=\frac{19}{2}{/tex}
   27. Let AB be the ladder & length of ladder is 5m
       i..e, AB = 5
       & OB be the wall & OA be the ground.
       
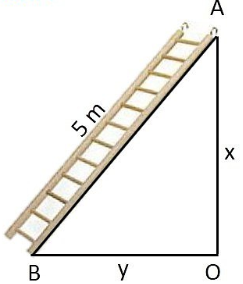
       Suppose OA = x & OB = y
       Given that
       The bottom of the ladder is pulled along the ground, away the wall at the rate of 2cm/s
       i.e., {tex}\frac{d x}{d t}{/tex} = 2cm/sec ….. (i)
       We need to calculate at which rate height of ladder on the wall.
       Decreasing when foot of the ladder is 4 m away from the wall
       i.e. we need to calculate {tex}\frac{d y}{d t}{/tex}  when x = 4 cm
       Wall OB is perpendicular to the ground OA
       
       Using Pythagoras theorem,we get
       (OB)² + (OA)² = (AB)²
       y² + x² = (5)²
       y² + x² = 24 …. (ii)
       Differentiating w.r.t. time,we get
       {tex}\frac{d\left(y^{2}+x^{2}\right)}{d t}=\frac{d(25)}{d t}{/tex}
       {tex}\frac{d\left(y^{2}\right)}{d t}+\frac{d\left(x^{2}\right)}{d t}=0{/tex}
       {tex}\frac{d\left(y^{2}\right)}{d t} \times \frac{d y}{d y}+\frac{d\left(x^{2}\right)}{d t} \times \frac{d x}{d x}=0{/tex}
       {tex}2 y \times \frac{d y}{d t}+2 x \times \frac{d x}{d t}=0{/tex}
       {tex}2 y \times \frac{d y}{d x}+2 x \times(2)=0{/tex}
       {tex}2 y \frac{d y}{d t}+4 x=0{/tex}
       {tex}2 y \frac{d y}{d t}=-4 x{/tex}
       {tex}\frac{d y}{d t}=\frac{-4 x}{2 y}{/tex}
       We need to find {tex}\frac{d y}{d t}{/tex} when x = 4cm
       {tex}\left.\frac{d y}{d t}\right|_{x=4}=\frac{-4 \times 4}{2 y}{/tex}
       {tex}\left.\frac{d y}{d t}\right|_{x=4}=\frac{-16}{2 y}{/tex} ….. (iii)
       Finding value of y
       From (ii)
       x² + y² = 25
       Putting x = 4
       (4)² + y² = 25
       y² = 9
       y = 3
   28. Section C
   29. According to the question,  {tex} I = \int \frac { 2 x + 5 } { \sqrt { 7 – 6 x – x ^ { 2 } } } d x{/tex}
       Above integral can be written as :{tex} I = \int \frac { 2 x + 5+1-1 } { \sqrt { 7 – 6 x – x ^ { 2 } } } d x{/tex}{tex} I = \int \frac { 2 x + 6-1 } { \sqrt { 7 – 6 x – x ^ { 2 } } } d x{/tex}
       {tex} I = \int \frac { – ( – 2 x – 6 ) – 1 } { \sqrt { 7 – 6 x – x ^ { 2 } } } d x{/tex}
       {tex} = – \int \frac { – 2 x – 6 } { \sqrt { 7 – 6 x – x ^ { 2 } } } d x – \int \frac { d x } { \sqrt { 7 – 6 x – x ^ { 2 } } }{/tex}
       {tex} let \ I = – I _ { 1 } – I _ { 2 }{/tex} …(i)
       {tex} I _ { 1 } = \int \frac { – 2 x – 6 } { \sqrt { 7 – 6 x – x ^ { 2 } } } d x{/tex}
       Put {tex}7 – 6x – x^2 = t{/tex}
       {tex} \Rightarrow{/tex} {tex}(-6 – 2x) dx = dt{/tex}
       {tex} \therefore \quad I _ { 1 } = \int \frac { d t } { \sqrt { t } } = \int t ^ { – 1 / 2 } d t= 2 \sqrt { t } + C_1{/tex}{tex} t = 7-6x – x^2{/tex}
       {tex} = 2 \sqrt { 7 – 6 x – x ^ { 2 } } + C _ { 1 }{/tex}
       {tex} I _ { 2 } = \int \frac { d x } { \sqrt { 7 – 6 x – x ^ { 2 } } }{/tex}{tex}=\int \frac { d x } { \sqrt { -(-7 + 6 x + x ^ { 2 } )} }{/tex}
       {tex} = \int \frac { d x } { \sqrt { – \left( – 7 + 6 x + x ^ { 2 } + 9 – 9 \right) } }{/tex}

       {tex} = \int \frac { d x } { \sqrt { – \left( 6 x + x ^ { 2 } + 9 – 16 \right) } }{/tex}
       {tex} = \int \frac { d x } { \sqrt { – \left[ ( x + 3 ) ^ { 2 } – 16 \right] } }{/tex}
       {tex} \Rightarrow \quad I _ { 2 } = \int \frac { d x } { \sqrt { ( 4 ) ^ { 2 } – ( x + 3 ) ^ { 2 } } }{/tex}
       {tex} = \sin ^ { – 1 } \left( \frac { x + 3 } { 4 } \right) + C _ { 2 }{/tex}{tex} \left[ \because \int \frac { d x } { \sqrt { a ^ { 2 } – x ^ { 2 } } } = \sin ^ { – 1 } \frac { x } { a } + c \right]{/tex}
       Putting the values of I₁ and I₂ in Equation (i),
       we get

       {tex}I= – 2 \sqrt { 7 – 6 x – x ^ { 2 } }-C_1- \sin ^ { – 1 } \left( \frac { x + 3 } { 4 } \right) -C_2{/tex}
       {tex}I= – 2 \sqrt { 7 – 6 x – x ^ { 2 } } – \sin ^ { – 1 } \left( \frac { x + 3 } { 4 } \right) -C_1-C_2{/tex}

       {tex}I= – 2 \sqrt { 7 – 6 x – x ^ { 2 } } – \sin ^ { – 1 } \left( \frac { x + 3 } { 4 } \right) + C \ [\because C = -C_1 – C_2]{/tex}

   30. E₁: Selected coin has tail on both faces
       E₂: Selected coin is biased
       E₃: Selected coin is unbiased
       A: Tail comes up
       P(E₁) = P(E₂) = P(E₃) = {tex}\frac {1} {3}{/tex}
       P(A|E₁) = 1, {tex}\mathrm{P}\left(\mathrm{AlE}_2\right)=\frac{7}{10}, \mathrm{P}\left(\mathrm{AlE}_3\right)=\frac{1}{2}{/tex}
       {tex}\mathrm{P}\left(\mathrm{E}_1 \mid \mathrm{A}\right)=\frac{\mathrm{P}\left(\mathrm{E}_1\right) \mathrm{P}\left(\mathrm{A} \mid E_1\right)}{\mathrm{P}\left(\mathrm{E}_1\right) \mathrm{P}\left(\mathrm{A} \mid E_1\right)+\mathrm{P}\left(\mathrm{E}_2\right) \mathrm{P}\left(\mathrm{A} \mid E_2\right)+\mathrm{P}\left(\mathrm{E}_3\right) \mathrm{P}\left(\mathrm{AlE}_3\right)}{/tex}
       {tex}=\frac{\frac{1}{3} \times 1}{\frac{1}{3} \times 1+\frac{1}{3} \times \frac{7}{10}+\frac{1}{3} \times \frac{1}{2}}{/tex}
       {tex}=\frac{10}{22}{/tex} or {tex}\frac{5}{11}{/tex}
   31. According to the question, {tex} I = \int _ { 1 } ^ { 2 } \frac { 5 x ^ { 2 } } { x ^ { 2 } + 4 x + 3 } d x{/tex}
       {tex} I = 5 \int _ { 1 } ^ { 2 } \left( 1 + \frac { – 4 x – 3 } { x ^ { 2 } + 4 x + 3 } \right) d x{/tex}
       {tex} = 5 \int _ { 1 } ^ { 2 } d x – 5 \int _ { 1 } ^ { 2 } \frac { 4 x + 3 } { x ^ { 2 } + 4 x + 3 } d x{/tex}
       {tex} \Rightarrow \quad I = 5 [ x ] _ { 1 } ^ { 2 } – 5 \int _ { 1 } ^ { 2 } \frac { 4 x + 3 } { ( x + 3 ) ( x + 1 ) } d x{/tex}
       Using partial fraction,
       let {tex} \frac { 4 x + 3 } { ( x + 3 ) ( x + 1 ) } = \frac { A } { x + 3 } + \frac { B } { x + 1 }{/tex}
       {tex} \Rightarrow \quad \frac { 4 x + 3 } { ( x + 3 ) ( x + 1 ) } = \frac { A ( x + 1 ) + B ( x + 3 ) } { ( x + 3 ) ( x + 1 ) }{/tex}
       {tex} \Rightarrow{/tex} {tex}4x + 3 = A(x + 1) + B(x + 3){/tex}
       Comparing the coefficients of like from both sides,
       {tex}\Rightarrow A + B = 4 \Rightarrow A = 4 – B{/tex}
       and A + 3B  = 3{tex} \Rightarrow{/tex} {tex}4 – B + 3B = 3{/tex}
       {tex} \Rightarrow \quad B = – \frac { 1 } { 2 },{/tex}then {tex} A = 4 + \frac { 1 } { 2 } = \frac { 9 } { 2 }{/tex}
       Now, from Equation (i), we get
       {tex} I = 5 ( 2 – 1 ) – 5 \int _ { 1 } ^ { 2 } \left( \frac { 9 / 2 } { x + 3 } + \frac { – 1 / 2 } { x + 1 } \right) d x{/tex}
       {tex} = 5 – 5 \left[ \frac { 9 } { 2 } \log | x + 3 | – \frac { 1 } { 2 } \log | x + 1 | \right] _ { 1 } ^ { 2 }{/tex}
       {tex} = 5 – 5\left. {\left[ {\left( {\frac{9}{2}\log 5 – \frac{1}{2}\log 3} \right)} \right. – \left( {\frac{9}{2}\log 4 – \frac{1}{2}\log 2} \right)} \right]{/tex}
       {tex} = 5 – 5 \left[ \frac { 9 } { 2 } ( \log 5 – \log 4 ) – \frac { 1 } { 2 } ( \log 3 – \log 4 ]\right.{/tex}
       {tex} = 5 – 5 \left[ \frac { 9 } { 2 } \log \frac { 5 } { 4 } – \frac { 1 } { 2 } \log \frac { 3 } { 2 } \right]{/tex}
       {tex} = 5 – \frac { 45 } { 2 } \log \frac { 5 } { 4 } + \frac { 5 } { 2 } \log \frac { 3 } { 2 }{/tex}

       OR

       {tex}I=\int\sqrt{5-4x-x^2}\;dx{/tex}
       {tex}I=\int\sqrt{-\left(x^2+4x+4-4-5\right)}\;dx{/tex}
       {tex}I=\int\sqrt{-\left(x+2\right)^2+9}\;dx{/tex}
       {tex}I=\int\sqrt{\left(3\right)^2-\left(x+2\right)^2}\;dx{/tex}
       As, {tex}\int\sqrt{\left(a\right)^2-\left(x\right)^2}\;dx{/tex} {tex}=\frac x2\sqrt{a^2-x^2}+\frac{a^2}2\sin^{-1}{\textstyle\frac xa}+C{/tex}
       So, {tex}\int\sqrt{\left(3\right)^2-\left(x+2\right)^2}\;dx{/tex} {tex}=\frac{x+2}2\sqrt{5-4x-x^2}+\frac92\sin^{-1}{\textstyle\frac{x+2}3}+C{/tex}

   32. We can rewrite the given differential equation as,
       {tex}\frac{d y}{d x}=\frac{y}{x}-\sin ^{2}\left(\frac{y}{x}\right){/tex}
       This is of the form {tex}\frac{d y}{d x}=f\left(\frac{y}{x}\right){/tex} So, it is homogeneous.
       Putting y = vx and {tex}\frac{d y}{d x}=v+x \frac{d v}{d x}{/tex} in (i), we get
       {tex}v+x \frac{d v}{d x}{/tex} = v -sin² v
       {tex}\Rightarrow \quad x \frac{d v}{d x}{/tex} = -sin² v
       {tex}\Rightarrow-cosec ^{2} v d v=\frac{1}{x} d x{/tex}
       {tex}\Rightarrow \int\left(-cosec ^{2} v\right) d v=\int \frac{1}{x} d x{/tex} [on integrating both sides] {tex}\Rightarrow {/tex} cot v = log |x| + C, where C is an arbitrary constant
       {tex}\Rightarrow {/tex} cot {tex}\frac{y}{x}{/tex} = log |x| + C …(ii) {tex}\left[\because v=\frac{y}{x}\right]{/tex}
       Putting x = 1 and y = {tex}\frac{\pi}{4}{/tex} in (ii), we get C = 1.
       {tex}\therefore{/tex} cot {tex}\frac{y}{x}{/tex}= log |x| + 1 is the desired solution.

       OR

       It is given that {tex}x \log x \frac{d y}{d x}+y=\frac{2}{x} \log x{/tex}
       {tex}\Rightarrow \frac{d y}{d x}+\frac{y}{x \log x}=\frac{2}{x^{2}}{/tex},
       This is equation in the form of {tex}\frac{d y}{d x}+p y=Q{/tex} (where, p = {tex}\frac{1}{x \log x}{/tex} and {tex}Q=\frac{2}{x^{2}}{/tex} )
       Now, I.F. = {tex}\mathrm{e}^{\int \mathrm{pdx}}=\mathrm{e}^{\int \frac{1}{\mathrm{xlog} \mathrm{x}} \mathrm{dx}}=\mathrm{e}^{\log |\log \mathrm{x}|}=\log \mathrm{x}{/tex}
       Thus, the solution of the given differential equation is given by the relation:
       {tex}\mathrm{y}(\mathrm{I}. \mathrm{F} )=\int(\mathrm{Q} \times \mathrm{I.F}) \mathrm{d} \mathrm{x}+\mathrm{C}{/tex}
       {tex}\Rightarrow \mathrm{y} . \log \mathrm{x}=\int\left[\frac{2}{\mathrm{x}^{2}} \cdot \log \mathrm{x}\right] \mathrm{d} \mathrm{x}+\mathrm{C}{/tex} ….(i)
       Now, {tex}\int\left[\frac{2}{x^{2}} \cdot \log x\right] d x=2 \int\left(\log x \cdot \frac{1}{x^{2}}\right) d x{/tex}
       = {tex}2\left[\log x . \int \frac{1}{x^{2}} d x-\int\left\{\frac{d}{d x}(\log x) \cdot \int \frac{1}{x^{2}} d x\right\} d x\right]{/tex}
       = {tex}2\left[\log _{\mathrm{X}}\left(-\frac{1}{\mathrm{x}}\right)-\int\left(\frac{1}{\mathrm{x}} \cdot\left(-\frac{1}{\mathrm{x}}\right)\right) \mathrm{d} \mathrm{x}\right]{/tex}
       = {tex}2\left[-\frac{\log x}{x}+\int \frac{1}{x^{2}} d x\right]{/tex}
       = {tex}2\left[-\frac{\log x}{x}-\frac{1}{x}\right]{/tex}
       = {tex}-\frac{2}{x}(1+\log x){/tex}
       Now, substituting the value in (i), we get,
       {tex}\Rightarrow \mathrm{y} \cdot \log \mathrm{x}=-\frac{2}{\mathrm{x}}(1+\log \mathrm{x})+\mathrm{C}{/tex}
       Therefore, the required general solution of the given differential equation is
       {tex}\text { y. } \log x=-\frac{2}{x}(1+\log x)+C{/tex}

   33. First, we will convert the given inequations into equations, we obtain the following equations:
       x + y = 4,
       x + y = 3,
       x – 2y = 2,
       x = 0 and y = 0
       The line x + 3y = 6 meets the coordinate axis at A(6, 0) and B(0, 2). Join these points to obtain the line x + 3y = 6
       Clearly, (0,0) does not satisfies the inequation x + 3y {tex}\geq{/tex} 6 . So, the region in xy-plane that does not contains the origin represents the solution set of the given equation.
       The line x – 3y = 3 meets the coordinate axis at C(3,0) and D(0, – 1). Join these points to obtain the line x – 3y = 3. Clearly, (0,0) satisfies the inequation x – 3y {tex}\leq{/tex} 3.50, the region in xy-plane that contains the origin represents the solution set of the given equation.
       The line 3x + 4 y = 24 meets the coordinate axis at E(8,0) and F(0,6). Join these points to obtain the line 3 x + 4 y = 24. Clearly, (0, 0) satisfies the inequation 3 x + 4 y {tex}\leq{/tex} 24. So, the region in xy-plane that contains the origin represents the solution set of the given equation.
       The line -3x + 2y = 6 meets the coordinate axis at G( – 2,0) and H(0,3). Join these points to obtain the line – 3 x + 2y = 6 Clearly, (0,0) satisfies the inequation – 3 x + 2y {tex}\leq{/tex} 6 . So, the region in xy-plane that contains the origin represents the solution set of the given equation.
       The line 5x + y = 5 meets the coordinate axis at x(1,0) and y(0,5) ) . Join these points to obtain line 5x + y = 5
       Clearly, (0,0) does not satisfies the inequation 5 x + y {tex}\geq{/tex} 5 . So, the region in xy-plane that does not contains the origin represents the solution set of the given equation.
       The region represented by x {tex}\geq{/tex} 0 and y {tex}\geq{/tex} 0 (non negative restrictions)since every point in the first quadrant satisfies these inequations. So, the  graph will be in first quadrant.These lines are drawn using a suitable scale.
       
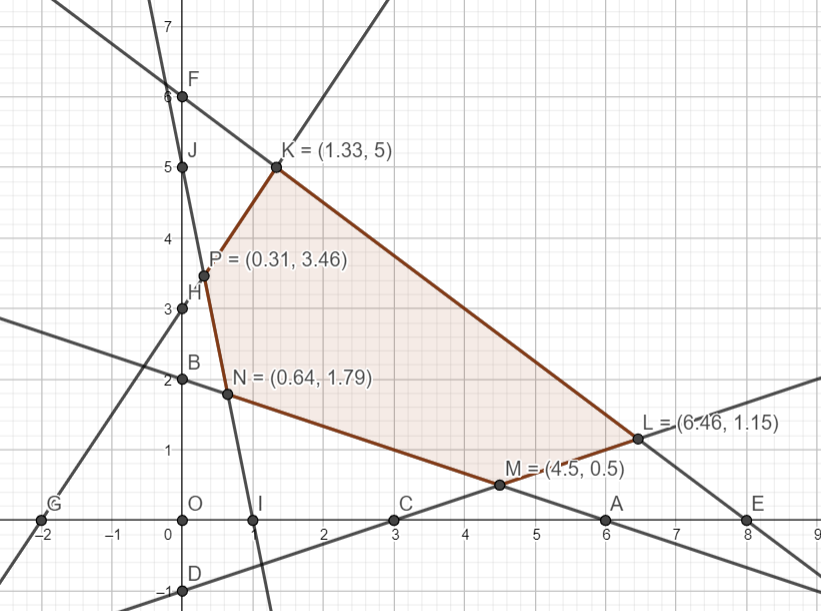
       The corner points of the feasible region are {tex}P\left(\frac{4}{13}, \frac{45}{13}\right){/tex} {tex}\mathrm{k}\left(\frac{4}{3}, 5\right){/tex}, {tex}L \left(\frac{84}{13}, \frac{15}{13}\right){/tex}, {tex}\mathrm{M}\left(\frac{9}{2}, \frac{1}{2}\right){/tex}, {tex}\mathrm{N}\left(\frac{9}{14}, \frac{25}{14}\right){/tex}
       The value of objective function Z = 2x + y at the corner point are as follows:
       {tex}P\left(\frac{4}{13}, \frac{45}{13}\right){/tex} : {tex}2 \times \frac{4}{13}+\frac{45}{13}=\frac{53}{13}{/tex}
       {tex}\mathrm{K}\left(\frac{4}{3}, 5\right){/tex}: {tex}2 \times \frac{4}{3}+5=\frac{23}{3}{/tex}
       {tex}L\left(\frac{84}{13}, \frac{15}{13}\right){/tex} ; {tex}2 \times \frac{84}{13}+\frac{15}{13}=\frac{183}{13}{/tex}
       {tex}M\left(\frac{9}{2}, \frac{1}{2}\right){/tex} : {tex}2 \times \frac{9}{2}+\frac{1}{2}=\frac{19}{2}{/tex}
       {tex}\mathrm{N}\left(\frac{9}{14}, \frac{25}{14}\right){/tex} : {tex}2 \times \frac{9}{14}+\frac{25}{14}=\frac{43}{14}{/tex}
       We see that the minimum value of the objective function Z is {tex}\frac{43}{14}{/tex} which is at {tex}\mathrm{N}\left(\frac{9}{14}, \frac{25}{14}\right){/tex}, and maximum value of the objective function is {tex}\frac{183}{13}{/tex} which is at {tex}L\left(\frac{84}{13}, \frac{15}{13}\right){/tex}.

       OR

       We have to maximize Z= 60 x + 15y First, we will convert the given inequations into equations, we obtain the following equations:
       x + y = 50, 3x + y = 90, x = 0 and y = 0
       Region represented by x + y {tex}\leq{/tex} 50 :
       The line x + y = 50 meets the coordinate axes at A(50, 0) and B(0, 50) respectively. By joining these points we obtain the line 3x + 5 y = 15 Clearly (0, 0) satisfies the inequation x + y {tex}\leq{/tex} 50 . Therefore, the region containing the origin represents the solution
       set of the inequation x + y {tex}\leq{/tex} 50
       Region represented by 3x + y {tex}\leq{/tex} 90 :
       The line 3x + y = 90 meets the coordinate axes at C(30, 0) and D(0, 90) respectively. By joining these points we obtain the line 3x + y = 90 Clearly (0, 0) satisfies the inequation 3x + y {tex}\leq{/tex} 90 . Therefore, the region containing the origin represents the solution set of the inequation 3x + y {tex}\leq{/tex} 90
       Region represented by x {tex}\geq{/tex} 0 and y {tex}\geq{/tex} 0 :
       since, every point in the first quadrant satisfies these inequations. Therefore, the first quadrant is the region represented by the inequations x {tex}\geq{/tex} 0, and y {tex}\geq{/tex} 0.
       The feasible region is given by {tex}{/tex}{tex}{/tex} {tex}{/tex}{tex}{/tex}
       
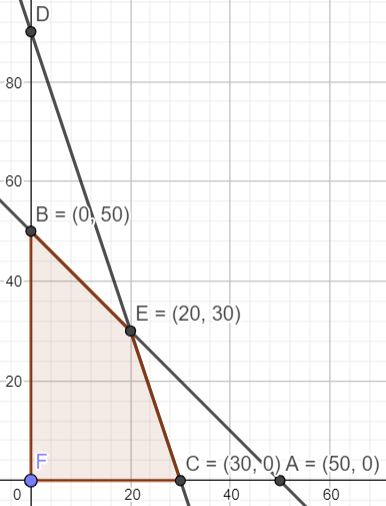
       The corner points of the feasible region are O(0, 0), C(30, 0) E(20, 30) and B(0, 50)
       The values of Z  at these corner points are as follows given by
       Corner point Z = 60 x + 15 y
       O(0, 0) : {tex}60 \times 0+15 \times 0=0{/tex}
       C(30, 0) : {tex}60 \times 30+15 \times 0=1800{/tex}
       E(20, 30) : {tex}60 \times 20+15 \times 30=1650{/tex}
       B(0, 50) : {tex}60 \times 0+15 \times 50=750{/tex}
       Therefore, the maximum value of Z is 1800 at the point (30, 0) Hence, x = 30 and y = 0 is the optimal solution of the given LPP. Thus, the optimal value of Z is 1800.This is the required solution.

   34. Given function is: {tex}f(x)=\left\{\begin{array}{l} 1, \text { if } x \leq 3 \\ a x+b, \text { if } 3<x<5 \\ 7, \text { if } x \geq 5 \end{array}\right.{/tex}
       We have,
       (LHL at x = 3) = {tex}\lim _\limits{x \rightarrow 3^{-}} f(x)=\lim _\limits{h \rightarrow 0} f(3-h){/tex}
       {tex}=\lim _\limits{h \rightarrow 0}(1)=1{/tex}
       (RHL at x = 3) = {tex}\lim _\limits{x \rightarrow 3^{+}} f(x)=\lim _\limits{h \rightarrow 0} f(3+h){/tex}
       {tex}=\lim _\limits{h \rightarrow 0} a(3+h)+b=3 a+b{/tex}
       (LHL at x = 5) = {tex}\lim _\limits{x \rightarrow 5^{-}} f(x)=\lim _\limits{h \rightarrow 0} f(5-h){/tex}
       {tex}=\lim _\limits{h \rightarrow 0}(a(5-h)+b)=5 a+b{/tex}
       (RHL at x = 5) = {tex}\lim _\limits{x \rightarrow 5^{+}} f(x)=\lim _\limits{h \rightarrow 0} f(5+h){/tex}
       {tex}=\lim _\limits{h \rightarrow 0}{/tex} 7 = 7
       If f(x) is continuous at x = 3 and 5, then
       {tex}\lim _\limits{x \rightarrow 3^{-}} f(x)=\lim _\limits{x \rightarrow 3^{+}} f(x) \text { and } \lim _\limits{x \rightarrow 5^{-}} f(x)=\lim _\limits{x \rightarrow 5^{+}} f(x){/tex}
       {tex}\Rightarrow{/tex} 1 = 3a + b …(i)
       and 5a + b = 7 …(ii)
       On solving eqs. (i) and (ii), we get,
       a = 3 and b = -8
   35. Section D
   36. According to the question ,Given equation of circle is {tex}x^2 + y^2 = 4{/tex}……(i)
       On differentiating Eq. (i) w.r.t. x , we get
       {tex}2x + 2y\frac{dy}{dx}{/tex}= 0
       {tex}\Rightarrow \quad x + y \frac { d } { d x } = 0{/tex}
       {tex}\Rightarrow \quad \frac { d y } { d x } = – \frac { x } { y }{/tex}
       {tex}\Rightarrow \quad \left( \frac { d y } { d x } \right) _ { ( 1 , \sqrt { 3 } ) } = – \frac { 1 } { \sqrt { 3 } }{/tex}
       {tex}\therefore m = – \frac { 1 } { \sqrt { 3 } }{/tex} ( ‘m’ is slope of tangent )
       Now, equation of tangent at point (1, {tex}\sqrt3{/tex}) is
       {tex}( y – \sqrt { 3 } ) = – \frac { 1 } { \sqrt { 3 } } ( x – 1 ){/tex}
       {tex}\Rightarrow \sqrt3 y – 3 = -x + 1{/tex}
       {tex}\Rightarrow \quad x + \sqrt { 3 } y = 4{/tex} …….(ii)
       equation of normal passing through point (1, {tex}\sqrt3{/tex}) andslope of normal = {tex}\frac {-1}{m_{(tangent )}} = \sqrt3{/tex}{tex}(y – \sqrt3) = \sqrt3 (x – 1){/tex}(y – {tex}\sqrt3{/tex}) = {tex}\sqrt3{/tex}x – {tex}\sqrt3{/tex} ……..(iii)
       Now, the Eqs. (i) and (ii) can be represented in the graph as shown below:
       
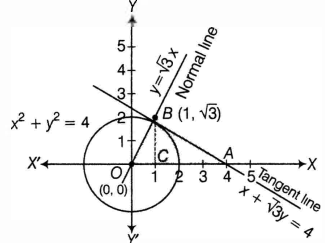
       On putting y = 0 in Eq. (i), we get
       x + 0 = 4
       {tex}\Rightarrow{/tex} x = 4
       {tex}\therefore{/tex} the tangent line x + {tex}\sqrt3{/tex}y = 4 cuts the X-axis at A(4,0).
       {tex}\therefore{/tex} Required area = Area of shaded region OAB
       {tex}= \int _ { 0 } ^ { 1 } y _ { ( \text { equation of normal) } } d x{/tex}+ {tex}\int _ { 1 } ^ { 4 } y _\text{ (equation of tangent) } d x{/tex}
       {tex}= \int _ { 0 } ^ { 1 } \sqrt { 3 } x d x + \int _ { 1 } ^ { 4 } \left( \frac { 4 – x } { \sqrt { 3 } } \right) d x{/tex}
       {tex}= \sqrt { 3 } \left[ \frac { x ^ { 2 } } { 2 } \right] _ { 0 } ^ { 1 } + \frac { 1 } { \sqrt { 3 } } \left[ 4 x – \frac { x ^ { 2 } } { 2 } \right] _ { 1 } ^ { 4 }{/tex}
       {tex}= \frac { \sqrt { 3 } } { 2 } + \frac { 1 } { \sqrt { 3 } } \left[ 16 – \frac { 16 } { 2 } – 4 + \frac { 1 } { 2 } \right]{/tex}
       {tex}= \frac { \sqrt { 3 } } { 2 } + \frac { 1 } { \sqrt { 3 } } \left[ 12 – \frac { 15 } { 2 } \right]{/tex}
       {tex}= \frac { \sqrt { 3 } } { 2 } + \frac { 1 } { \sqrt { 3 } } \left[ \frac { 9 } { 2 } \right]{/tex}
       {tex}= \frac { \sqrt { 3 } } { 2 } + \frac { 3 \sqrt { 3 } } { 2 }{/tex}
       {tex}= \frac { 4 \sqrt { 3 } } { 2 } {/tex}
       {tex}= 2 \sqrt { 3 }{/tex}sq units.
   37. f is one-one: For any x, y {tex}\in{/tex} R, we have f(x) : f(y)
       {tex}\Rightarrow \frac{x}{{1 + |x|}} = \frac{y}{{|y| + 1}}{/tex}
       {tex}\Rightarrow{/tex} xy + x = xy + y
       {tex}\Rightarrow{/tex} x = y
       Therefore, f is one-one function.
       If f is one-one, let y = R – {1}, then f(x) = y
       {tex}\Rightarrow \frac{x}{{x + 1}} = y{/tex}
       {tex}\Rightarrow x = \frac{y}{{1 – y}}{/tex}
       It is clear that x {tex}\in{/tex} R for all y = R – {1}, also x {tex}\ne{/tex}=-1
       Because x = -1
       {tex}\Rightarrow \frac{y}{{1 – y}} = – 1{/tex}
       {tex}\Rightarrow{/tex} y = -1 + y which is not possible.
       Thus for each R – {1} there exists {tex}x = \frac{y}{{1 – y}} \in{/tex} R – {1} such that
       {tex}f(x) = \frac{x}{{x + 1}} = \frac{{\frac{y}{{1 – y}}}}{{\frac{y}{{1 – y}} + 1}} = y{/tex}
       Therefore f is onto function.

       OR

       We observe the following properties of relation R.
       Reflexivity: Let (a, b) be an arbitrary element of N {tex}\times{/tex} N. Then,
       (a, b) {tex}\in{/tex} N {tex}\times{/tex} N
       {tex}\Rightarrow{/tex} a, b {tex}\in{/tex} N
       {tex}\Rightarrow{/tex} ab = ba [By commutativity of multiplication on N] {tex}\Rightarrow{/tex} (a, b) R (a, b)
       Thus, (a, b) R (a, b) for all (a, b) {tex}\in{/tex} N {tex}\times{/tex} N.
       So, R is reflexive on N {tex}\times{/tex} N.
       Symmetry: Let (a, b), (c, d) {tex}\in{/tex} N {tex}\times{/tex} N be such that (a, b) R (c, d). Then,
       (a, b) R (c, d)
       {tex}\Rightarrow{/tex} ad = bc
       {tex}\Rightarrow{/tex} cb = da [By commutativity of multiplication on N] {tex}\Rightarrow{/tex} (c,d) R (a, b)
       Thus, (a, b) R (c, d) {tex}\Rightarrow{/tex} (c, d) R (a, b) for all (a, b), (c, d ) {tex}\in{/tex} N {tex}\times{/tex} N .
       So, R is symmetric on N {tex}\times{/tex} N.
       Transitivity: Let (a, b), (c, d), (e, f) {tex}\in{/tex} N {tex}\times{/tex}N such that (a, b) R (c, d) and (c, d) R (e, f). Then,
       {tex}\begin{array}{l}{(a, b) R(c, d) \Rightarrow a d=b c} \\ {(c, d) R(e, f) \Rightarrow c f=d e}\end{array} \} \Rightarrow(a d)(c f){/tex}= (bc) (de) {tex}\Rightarrow{/tex} af = be {tex}\Rightarrow{/tex} (a, b) R (e, f)
       Thus, (a, b) R (c, d) and (c, d) R (e, f) {tex}\Rightarrow{/tex} (a, b) R (e, f) for all (a, b), (c, d), (e, f) {tex}\in{/tex} N{tex}\times{/tex} N.
       So, R is transitive on N {tex}\times{/tex} N.
       Hence, R being reflexive, symmetric and transitive, is an equivalence relation on N{tex}\times{/tex} N.
       [(2, 6)] = {(x, y) {tex}\in{/tex} N{tex}\times{/tex} N :(x, y) R (2, 6)}
       = {( x, y) {tex}\in{/tex} N {tex}\times{/tex} N :3x = y}
       = {(x, 3x ) : x {tex}\in{/tex} N ] ={(1, 3), (2, 6), (3, 9), (4,12),..}

   38. Given: Matrix {tex}A = \left[ {\begin{array}{*{20}{c}} 2&{ – 3}&5 \\ 3&2&{ – 4} \\ 1&1&{ – 2} \end{array}} \right]{/tex}
       {tex}\therefore{/tex} |A| = {tex}\left| {\begin{array}{*{20}{c}} 2&{ – 3}&5 \\ 3&2&{ – 4} \\ 1&1&{ – 2} \end{array}} \right|{/tex}
       {tex}\Rightarrow{/tex} |A| = 2(-4 + 4) – (-3)(-6 + 4) + 5(3 – 2) = 0 – 6 + 5 = -1 {tex} \ne{/tex} 0
       {tex}\therefore{/tex} A^-1 exists and A^-1 = {tex} \frac{1}{{\left| A \right|}}{/tex} (adj. A)…(i)
       Now, A₁₁ = 0, A₁₂ = 2, A₁₃ = 1 and A₂₁ = -1, A₂₂ = -9, A₂₃ = -5 and A₃₁ = 2, A₃₂ = 23, A₃₃ = 13
       {tex}\therefore{/tex} adj. A ={tex} \left[ {\begin{array}{*{20}{c}} 0&2&1 \\ { – 1}&{ – 9}&{ – 5} \\ 2&{23}&{13} \end{array}} \right]’ = \left[ {\begin{array}{*{20}{c}} 0&{ – 1}&2 \\ 2&{ – 9}&{23} \\ 1&{ – 5}&{13} \end{array}} \right]{/tex}
       From eq. (i),
       A^-1 = {tex} \frac{1}{{ – 1}}\left[ {\begin{array}{*{20}{c}} 0&{ – 1}&2 \\ 2&{ – 9}&{23} \\ 1&{ – 5}&{13} \end{array}} \right] = \left[ {\begin{array}{*{20}{c}} 0&1&{ – 2} \\ { – 2}&9&{ – 23} \\ { – 1}&5&{ – 13} \end{array}} \right]{/tex}
       Now, Matrix form of given equations is AX = B
       {tex}\Rightarrow \left[ {\begin{array}{*{20}{c}} 2&{ – 3}&5 \\ 3&2&{ – 4} \\ 1&1&{ – 2} \end{array}} \right]\left[ {\begin{array}{*{20}{c}} x \\ y \\ z \end{array}} \right] = \left[ {\begin{array}{*{20}{c}} {11} \\ { – 5} \\ { – 3} \end{array}} \right]{/tex}
       Here A ={tex} \left[ {\begin{array}{*{20}{c}} 2&{ – 3}&5 \\ 3&2&{ – 4} \\ 1&1&{ – 2} \end{array}} \right]{/tex}, X = {tex}\left[\begin{array}{l} x \\ y \\ z \end{array}\right]{/tex} and B ={tex} \left[ {\begin{array}{*{20}{c}} {11} \\ { – 5} \\ { – 3} \end{array}} \right]{/tex}
       Therefore, solution is unique and X = A^-1B
       {tex}\Rightarrow \left[ {\begin{array}{*{20}{c}} x \\ y \\ z \end{array}} \right] = \left[ {\begin{array}{*{20}{c}} 0&1&{ – 2} \\ { – 2}&9&{ – 23} \\ { – 1}&5&{ – 13} \end{array}} \right]\left[ {\begin{array}{*{20}{c}} {11} \\ { – 5} \\ { – 3} \end{array}} \right]{/tex}
       {tex}= \left[ {\begin{array}{*{20}{c}} {0 – 5 + 6} \\ { – 22 – 45 + 69} \\ { – 11 – 25 + 39} \end{array}} \right]{/tex}
       {tex}= \left[ {\begin{array}{*{20}{c}} 1 \\ 2 \\ 3 \end{array}} \right]{/tex}
       Therefore, x = 1, y = 2 and z = 3
   39. The given equations are
       al + bm + cn = 0 ….(i)
       and, ul² + vm² + wn² = 0 ..(ii)
       From (i), we get
       n = –{tex}\left(\frac{a l+b m}{c}\right){/tex}
       Substituting n = –{tex}\left(\frac{a l+b m}{c}\right){/tex} in (ii), we get
       ul² + vm² + w {tex}\frac{(a l+b m)^{2}}{c^{2}}{/tex} = 0
       {tex}\Rightarrow{/tex} (c²u + a²w)l² + 2abwlm + (c²v + b²w)m² = 0
       {tex}\Rightarrow{/tex} {tex}\left(a^{2} w+c^{2} u\right)\left(\frac{l}{m}\right)^{2}+2 a b w\left(\frac{l}{m}\right)+\left(b^{2} w+c^{2} v\right)=0{/tex} ….(iii)
       This is a quadratic equation in {tex}\frac{l}{m}{/tex}. So, it gives two values of {tex}\frac{l}{m}{/tex}. Suppose the two values be {tex}\frac{l_{1}}{m_{1}}{/tex} and {tex}\frac{l_{2}}{m_{2}}{/tex}.
       {tex}\therefore \quad \frac{l_{1}}{m_{1}}, \frac{l_{2}}{m_{2}}=\frac{b^{2} w+c^{2} v}{a^{2} w+c^{2} u} \Rightarrow \frac{l_{1} l_{2}}{b^{2} w+c^{2} v}=\frac{m_{1} m_{2}}{a^{2} w+c^{2} u}{/tex} …..(iv)
       Similarly, by making a quadratic equation in {tex}\frac{m}{n}{/tex}, we obtain
       {tex}\frac{m_{1} m_{2}}{a^{2} w+c^{2} u}=\frac{n_{1} n_{2}}{a^{2} v+b^{2} u}{/tex} ….(v)
       From (iv) and (v), we get
       {tex}\frac{l_{1} l_{2}}{b^{2} w+c^{2} v}=\frac{m_{1} m_{2}}{a^{2} w+c^{2} u}=\frac{n_{1} n_{2}}{a^{2} v+b^{2} u}=\lambda{/tex} (say)
       {tex}\Rightarrow{/tex} {tex}l_{1} l_{2}=\lambda\left(b^{2} w+c^{2} v\right), m_{1} m_{2}=\lambda\left(a^{2} w+c^{2} u\right), n_{1} n_{2}=\lambda\left(a^{2} v+b^{2} u\right){/tex}
       For the given lines to be perpendicular, we must have
       l₁ l₂ + m₁ m₂ + n₁ n₂ = 0
       {tex}\Rightarrow{/tex} {tex}\lambda\left(b^{2} w+c^{2} v\right)+\lambda\left(a^{2} w+c^{2} u\right)+\lambda\left(a^{2} v+b^{2} u\right)=0{/tex}
       {tex}\Rightarrow{/tex} a² (v + w) + b² (u + w) + c² (u + v) = 0
       For the given lines to be parallel, the direction cosines must be equal and so the roots of the equation (iii) must be equal.
       {tex}\therefore{/tex} 4a² b² w² − 4(a²w + c²u) (b²w + c²v) = 0 [On equating discriminant to zero] {tex}\Rightarrow{/tex} a² c² vw + b² c² uw + c⁴ uv = 0
       {tex}\Rightarrow{/tex} a² vw + b² c² uw + c²uv = 0
       {tex}\Rightarrow{/tex} {tex}\frac{a^{2}}{u}+\frac{b^{2}}{v}+\frac{c^{2}}{w}{/tex} = 0 [Dividing throughout by uvw] Hence the required result is proved

       OR

       {tex}{\vec a_1} = \hat i + \hat j,{\vec b_1} = 2\hat i – \hat j + \hat k{/tex}
       {tex}{\vec a_2} = 2\hat i + \hat j – \hat k,{\vec b_2} = 3\hat i – 5\hat j + 2\hat k{/tex}
       {tex}{\vec a_2} – {\vec a_1} = \hat i – \hat k{/tex}
       {tex}{\vec b_1} \times {\vec b_2} = \left| {\begin{array}{*{20}{c}} {\hat i}&{\hat j}&{\hat k} \\ 2&{ – 1}&1 \\ 3&{ – 5}&2 \end{array}} \right|{/tex}
       {tex}=\hat i (-2+5)-\hat j(4-3)+\hat k(-10+3){/tex}
       {tex} = 3\hat i – \hat j – 7\hat k{/tex}
       {tex}\left| {{{\vec b}_1} \times {{\vec b}_2}} \right|=\sqrt {9+1+49}= \sqrt {59} {/tex}
       Also, {tex}(\vec b_1×\vec b_2).(\vec a_2-\vec a_1)=(3\hat i-\hat j-7 \hat k)(\hat i-\hat k)=3+7+0=10{/tex}
       {tex}d=\left|\frac{(\vec b_1×\vec b_2).(\vec a_2-\vec a_1)}{|\vec b_1×\vec b_2|}\right|=\frac{10}{\sqrt{59}}{/tex}

   40. Section E
       1. Let A represents obtaining a sum greater than 9 and B represents black die resulted in a 5.
          n(S) = 36
          n(A) = {(4, 6), (5, 5), (5, 6), (6, 4), (6, 5), (6, 6)} = 6
          n(B) = {(5, 1), (5, 2), (5, 3), (5, 4), (5, 5)} = 6
          n(A {tex}\cap{/tex} B) = {(5, 5), (5, 6)} = 2
          P(A/B) = {tex}\frac{{P\left( {A \cap B} \right)}}{{P\left( B \right)}}{/tex} = {tex}\frac{{\frac{{n\left( {A \cap B} \right)}}{{n\left( S \right)}}}}{{\frac{{n\left( B \right)}}{{n\left( S \right)}}}}{/tex} = {tex}\frac{{\frac{2}{{36}}}}{{\frac{6}{{36}}}} = \frac{1}{3}{/tex}
       2. Let A represents obtaining a sum 8 and B represents red die resulted in number less than 4.
          n(S) = 36
          n(A) = {(2, 6), (3, 5), (4, 4), (5, 3), (6, 2) = 5
          n(B) = {(1, 1), (1, 2), (1, 3), (2, 1), (2, 2), (2, 3), (3, 1), (3, 2), (3, 3), (4, 1), (4, 2), (4, 3), (5, 1), (5, 2), (5, 3), (6, 1), (6, 2), (6, 3)} = 18
          n(A {tex}\cap{/tex} B) = {(5, 3), (6, 2)} = 2
          P(A/B) = {tex}\frac{{P\left( {A \cap B} \right)}}{{P\left( B \right)}}{/tex} = {tex}\frac{{\frac{{n\left( {A \cap B} \right)}}{{n\left( S \right)}}}}{{\frac{{n\left( B \right)}}{{n\left( S \right)}}}}{/tex} = {tex}\frac{{\frac{2}{{36}}}}{{\frac{{18}}{{36}}}} = \frac{1}{9}{/tex}
       3. Let A represents obtaining a sum 10 and B represents black die resulted in even number.
          n(S) = 36
          n(A) = {(4, 6), (6, 4), (5, 5)} = 3
          n(B) = {(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6), (4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6), (6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)} = 18
          n(A {tex}\cap{/tex} B) = {(4, 6), (6, 4)} = 2
          P(A/B) = {tex}\frac{{P\left( {A \cap B} \right)}}{{P\left( B \right)}} = \frac{{\frac{{n\left( {A \cap B} \right)}}{{n\left( S \right)}}}}{{\frac{{n\left( B \right)}}{{n\left( S \right)}}}}{/tex} = {tex}\frac{{\frac{2}{{36}}}}{{\frac{{18}}{{36}}}} = \frac{1}{9}{/tex}
          OR
          Let A represents getting doublet and B represents red die resulted in number greater than 4.
          n(S) = 36
          n(A) = {(1, 1), (2, 2), (3, 3), (4, 4), (5, 5), (6, 6)} = 6
          n(B) = {(1, 5), (1, 6), (2, 5), (2, 6), (3, 5), (3, 6), (4, 5), (4, 6), (5, 5), (5, 6), (6, 5), (6, 6)} = 12
          n(A {tex}\cap{/tex} B) = {(4, 4), (5, 5), (6, 6)} = 3
          P(A/B) = {tex}\frac{{P\left( {A \cap B} \right)}}{{P\left( B \right)}} = \frac{{\frac{{n\left( {A \cap B} \right)}}{{n\left( S \right)}}}}{{\frac{{n\left( B \right)}}{{n\left( S \right)}}}}{/tex} = {tex}\frac{{\frac{3}{{36}}}}{{\frac{{12}}{{36}}}} = \frac{1}{4}{/tex}

       1. Here,
          Position vector of A is {tex}\vec{a}=\hat{i}+4 \hat{j}+2 \hat{k} {/tex}
          Position vector of B is {tex}\vec{b}=3 \hat{i}-3 \hat{j}-2 \hat{k}{/tex}
          Position vector of C is {tex}\vec{c}=-2 \hat{i}+2 \hat{j}+6 \hat{k}{/tex}
          {tex}\therefore \vec{a}+\vec{b}+\vec{c}{/tex} = (1 + 3 – 2){tex}\hat i{/tex} + (4 – 3 + 2){tex}\hat j{/tex} + (2 – 2 + 6){tex}\hat k{/tex}
          = 2{tex}\hat i{/tex} + 3{tex}\hat j{/tex} + 6{tex}\hat k{/tex}
          Thus, {tex}|\vec{a}+\vec{b}+\vec{c}|{/tex} = {tex}\left|\sqrt{(2)^2+(3)^2+(6)^2}\right|{/tex}
          = {tex}|\sqrt{4+9+16}|{/tex}
          = {tex}\sqrt {29}{/tex}

       2. Given, {tex}\vec{a}=4 \hat{i}+6 \hat{j}+12 \hat{k}{/tex},
          {tex}|\vec{a}|=\sqrt{4^2+6^2+12^2}{/tex} = 14
          Therefore, the unit vector in direction of {tex}\vec a{/tex} is given by
          {tex}\hat{a} =\frac{\vec{a}}{|\vec{a}|}=\frac{4 \hat{i}+6 \hat{j}+12 \hat{k}}{14}{/tex}
          = {tex}\frac{4}{14} \hat{i}+\frac{6}{14} \hat{j}+\frac{12}{14} \hat{k}{/tex}
          = {tex}\frac{2}{7} \hat{i}+\frac{3}{7} \hat{j}+\frac{6}{7} \hat{k}{/tex}

       3. We have, A(1, 4, 2), B(3, -3, -2) and C(-2, 2, 6)
          Now, {tex}\overrightarrow{A B}=\vec{b}-\vec{a}=2 \hat{i}-7 \hat{j}-4 \hat{k}{/tex}
          and {tex}\overrightarrow{A C}=\vec{c}-\vec{a}=-3 \hat{i}-2 \hat{j}+4 \hat{k}{/tex}
          {tex}\therefore{/tex} {tex}\overrightarrow{A B} \times \overrightarrow{A C}{/tex} = {tex}\left|\begin{array}{ccc} \hat{i} & \hat{j} & \hat{k} \\ 2 & -7 & -4 \\ -3 & -2 & 4 \end{array}\right|{/tex}
          = {tex}\hat{i}(-28-8)-\hat{j}(8-12)+\hat{k}(-4-21){/tex}
          = – {tex}36 \hat{i}+4 \hat{j}-25 \hat{k}{/tex}
          Now, {tex}|\overrightarrow{A B} \times \overrightarrow{A C}|=\sqrt{(-36)^2+4^2+(-25)^2}{/tex}
          = {tex}|\sqrt{1296+16+625}|=\sqrt{1937}{/tex}
          {tex}\therefore{/tex} Area of {tex}\triangle{/tex}ABC = {tex}\frac{1}{2}|\overrightarrow{A B} \times \overrightarrow{A C}|{/tex}
          = {tex}\frac{1}{2} \sqrt{1937}{/tex} sq. units
          OR
          Triangle law of addition for {tex}\triangle{/tex}ABC is given by
          {tex}\overrightarrow{A B}+\overrightarrow{B C}+\overrightarrow{C A}{/tex} = {tex}\vec 0{/tex}
          If the given points lie on the straight line, then the points will be collinear and so area of {tex}\triangle{/tex}ABC = 0
          Then, {tex}|\vec{a} \times \vec{b}+\vec{b} \times \vec{c}+\vec{c} \times \vec{a}|{/tex} = 0.
          Also, if a, b, c are the position vector of the three vertices A, B and C of {tex}\triangle{/tex}ABC, then area of triangle is {tex}\frac{1}{2}|\vec{a} \times \vec{b}+\vec{b} \times \vec{c}+\vec{c} \times \vec{a}|{/tex}.

       1. Let ‘y’ be the breadth and ‘x’ be the length of rectangle and ‘a’ is radius of given circle.
          From fig 4a² = x² + y²
          {tex}\Rightarrow{/tex} y² = 4a² – x²
          {tex}\Rightarrow{/tex} y = {tex}\sqrt {4{a^2}\, – {x^2}}{/tex}
          Perimeter (P) = 2x + 2y = {tex}2\left( {x + \sqrt {4{a^2}\, – {x^2}} } \right){/tex}
       2. We know that P = {tex}2\left(x+\sqrt{4 a^2-x^2}\right){/tex}
          Critical points to maximize perimeter {tex}\frac{dP}{dx}{/tex} = 0
          {tex}\Rightarrow \frac{d p}{d x}=2\left(1+\frac{1}{2 \sqrt{4 a^2-x^2}}(-2 x)\right){/tex} = 0
          {tex}2\left(\frac{\sqrt{4 a^2-x^2}-x}{\sqrt{4 a^2-x^2}}\right){/tex} = 0
          {tex}\Rightarrow \sqrt{4 a^2-x^2}{/tex} = x
          {tex}\Rightarrow{/tex} 4a² – x² = x²
          {tex}\Rightarrow{/tex} 2a² = x²
          {tex}\Rightarrow{/tex} x = {tex}\pm \sqrt{2a}{/tex}
          when x = {tex}\sqrt{2a}{/tex}, y = {tex}\sqrt{2a}{/tex}
          when x = {tex}-\sqrt{2a}{/tex} not possible as ‘x’ is length critical point is ({tex}\sqrt{2a}{/tex}, {tex}\sqrt{2a}{/tex})
       3. {tex}\frac{d p}{d x}=2\left(1+\frac{1}{2 \sqrt{4 a^2-x^2}}(-2 x)\right){/tex}
          {tex}\frac{d^2 P}{d x^2}=-2\left(\frac{\sqrt{4 a^2-x^2}-(x)\left(\frac{-2 x}{2 \sqrt{4^2-x^2}}\right)}{\left(4 a^2-x^2\right)}\right){/tex}
          = {tex}-2\left(\frac{\left(4 a^2-x^2\right)+x^2}{\left(4 a^2-x^2\right)^{3 / 2}}\right){/tex}
          {tex}\left.\Rightarrow \frac{d^2 P}{d x^2}\right]_{x=a \sqrt{2}}{/tex} = {tex}-2\left(\frac{4 a^2}{\left(4 a^2-2 a^2\right)^{3 / 2}}\right)=\frac{-2}{(2 \sqrt{2}) a}<0{/tex}
          Perimeter is maximum at a critical point.
          OR
          From the above results know that x = y = {tex}\sqrt{2}a{/tex}
          a = radius
          Here, x = y = {tex}10\sqrt{2}{/tex}
          Perimeter = P = 4 {tex}\times{/tex} side = {tex}40\sqrt{2}{/tex} cm

Download myCBSEguide App for Complete Exam Preparation

To practice more questions and prepare effectively for your exams, download the myCBSEguide app. It offers comprehensive study material for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams. The app provides access to sample papers, previous year questions, chapter-wise practice tests, and more, helping you get ready for exams in a structured manner.

For teachers, the Examin8.com app allows you to easily create customized papers with your own name and logo, making exam preparation more efficient for your students.

Note: These are just the questions! To view and download the complete question paper with solutions, install the myCBSEguide app from the Google Play Store or log in to our Student Dashboard on the myCBSEguide website.

Get started today and ensure you are fully prepared for your exams with myCBSEguide!

Download myCBSEguide App

Sample Papers for Class 12 2025

• Physics
• Chemistry
• Mathematics
• Biology
• English Core
• Business Studies
• Economics
• Accountancy
• Computer Science
• Informatics Practices
• Hindi Core
• Hindi Elective
• History
• Political Science
• Geography
• Home Science
• Physical Education
• Other Subjects

Download CBSE Class 12 Sample Papers for All Subjects

Looking for Class 12 sample papers for Physics, Chemistry, Biology, History, Political Science, Economics, Geography, Computer Science, Home Science, Accountancy, Business Studies, and more? Visit the myCBSEguide app or the myCBSEguide website to download free sample papers with solutions in PDF format. For students preparing for the Class 12 Maths exams 2025, solving Class 12 Maths sample papers 2025 is one of the most effective ways to practice. By regularly working through Class 12 Maths sample papers 2025, students can familiarize themselves with key concepts and improve their problem-solving speed. Additionally, Class 12 Maths sample papers 2025 help students assess their preparation levels and pinpoint areas that need further revision. Download the Class 12 Maths sample papers 2025 from reliable sources like the myCBSEguide app, which provides updated papers based on the latest syllabus and exam guidelines.

myCBSEguide provides sample papers with solutions, chapter-wise test papers, NCERT solutions, NCERT Exemplar solutions, quick revision notes, CBSE guess papers, and important question papers. These resources are designed to help you prepare efficiently for your CBSE Class 12 exams.

All sample papers are updated according to the latest CBSE syllabus and marking scheme. With the best app for CBSE students and the myCBSEguide website, you can access comprehensive study material and enhance your exam preparation. Start practicing today and boost your chances of success in your Class 12 exams!