Class 10 Maths Sample Papers 2025

Table of Contents

myCBSEguide App

Download the app to get CBSE Sample Papers 2024-25, NCERT Solutions (Revised), Most Important Questions, Previous Year Question Bank, Mock Tests, and Detailed Notes.

Install Now

Class 10 Maths Standard Sample Papers 2025

CBSE offers two distinct courses in Class 10 Maths: Maths Basic and Maths Standard. This page provides Class 10 Maths Standard Sample Papers 2025 and their solution specifically tailored for students enrolled in the Maths Standard course. Sample Model Test Papers for Class 10 Maths with detailed solutions are available on the myCBSEguide app. Teachers can use the Examin8 app to create customized exam papers with their own branding, including name and logo. For students, the myCBSEguide website offers a range of study resources, sample papers, and solutions to enhance exam preparation. These sample papers are designed by the latest marking scheme and blueprint issued by CBSE for the 2024-25 academic session.

By practicing the Class 10 Maths Standard Sample Papers 2025, students can get a comprehensive understanding of the exam format, question types, and mark distribution. This practice will help improve problem-solving skills and enhance time management, ensuring students are thoroughly prepared for the Class 10 board exams.

Sample Paper of Maths Class 10 – PDF with Solution

Class 10 Maths Sample Papers 2025 – Practice with the Latest Marking Scheme and Blueprint

We are offering Class 10 Maths Sample Papers 2025 designed according to the latest marking scheme and blueprint for both Maths Basic and Maths Standard courses. Maths is a subject that requires consistent practice, but it is also one of the most scoring subjects in the CBSE board exams. Start practicing today with the Class 10 Maths Sample Papers 2025 and ensure you are fully prepared for your board exams

Maths Standard Sample Paper 2025 with Solution Download as PDF

Maths Basic Sample Paper 2025 with Solution Download as PDF

CBSE Sample Papers Class 10 Mathematics 2019

CBSE Class 10 Maths Sample Papers 2024-25

Download Class 10 Maths Sample Papers 2025 with Solutions – Free PDF on myCBSEguide

myCBSEguide Website offers Class 10 Maths Sample Papers 2025 with solutions in PDF format for free download. These sample papers are designed to help students understand the updated exam pattern and question types. You may notice that the format of the Class 10 Maths Sample Papers 2025 is slightly different from previous years, with a reduced number of straightforward questions and an increased focus on real-life application-based problems.

Many questions in the 2025 Maths sample papers(SQP) aim to assess your competency in applying concepts to practical situations.Start practicing today by downloading the Class 10 Maths Sample Papers 2025 with solutions on myCBSEguide to boost your confidence and score well in your board exams.

Model Papers Class 10 Maths Standard 2025

CBSE class 10 Maths Sample Papers

Class 10 – Mathematics
Maths Standard SP – 01 (2024-25)

Maximum Marks: 80
Time Allowed: : 3 hours

General Instructions:

This Question Paper has 5 Sections A, B, C, D and E.
Section A has 20 MCQs carrying 1 mark each
Section B has 5 questions carrying 02 marks each.
Section C has 6 questions carrying 03 marks each.
Section D has 4 questions carrying 05 marks each.
Section E has 3 case based integrated units of assessment carrying 04 marks each.
All Questions are compulsory. However, an internal choice in 2 Qs of 5 marks, 2 Qs of 3 marks and 2 Questions of 2 marks has been provided. An internal choice has been provided in the 2 marks questions of Section E
Draw neat figures wherever required. Take {tex}\pi=\frac{22}{7}{/tex} wherever required if not stated.

Section A
The HCF of the smallest 2-digit number and the smallest composite number is
a) 4
b) 10
c) 20
d) 2
In the given figure, graph of a polynomial p(x) is given. Number of zeroes of p(x) is:

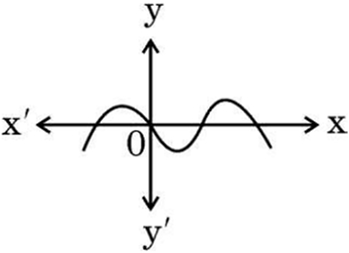a) 2
b) 4
c) 5
d) 3
The pair of linear equations y = 0 and y = – 6 has:

a) no solution
b) only solution (0, 0)
c) infinitely many solutions
d) a unique solution
The roots of the quadratic equation 2x² – x – 6 = 0 are
a) {tex}2, \frac{-3}{2}{/tex}
b) {tex}2, \frac{3}{2}{/tex}
c) {tex}-2, \frac{-3}{2}{/tex}
d) {tex}-2, \frac{3}{2}{/tex}
The 5th term of an AP is -3 and its common difference is -4. The sum of its first 10 terms is
a) 30
b) -50
c) -30
d) 50
The point on the x-axis which is equidistant from points (-1, 0) and (5, 0) is
a) (0, 3)
b) (2, 0)
c) (3, 0)
d) (0, 2)
The centre of the circle having end points of its one diameter as (-4, 2) and (4, -3) is
a) (2, -1)
b) (0, -1)
c) (1, -1)
d) (0,{tex}\frac{-1}{2}{/tex})
In the given figure, DE || BC. If AD = 2 units, DB = AE = 3 units and EC = x units, then the value of x is:

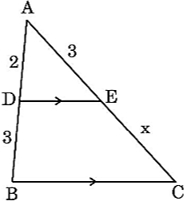a) 2
b) 3
c) {tex}\frac{9}{2}{/tex}
d) 5
In Figure, TP and TQ are tangents drawn to the circle with centre at O. If {tex}\angle \text { POQ}{/tex} = 115^o then {tex}\angle \text { PTQ}{/tex} is

a) 55^o
b) 115^o
c) 65^o
d) 57.5^o
Quadrilateral ABCD is circumscribed to a circle. If AB = 6 cm, BC = 7 cm and CD = 4 cm then the length of AD is
a) 6 cm
b) 4 cm
c) 7 cm
d) 3 cm
8 (cos² A + sin² A) is equal to:
a) 8
b) 0
c) 9
d) 1
If 2 tan A = 3, then the value of {tex}\frac{4 \sin A+3 \cos A}{4 \sin A-3 \cos A}{/tex} is
a) 3
b) does not exist
c) {tex}\frac{7}{\sqrt{13}}{/tex}
d) {tex}\frac{1}{\sqrt{13}}{/tex}
A kite is flying at a height of 30 m from the ground. The length of string from the kite to the ground is 60 m. Assuming that there is no slack in the string, the angle of elevation of the kite at the ground is
a) 30°
b) 45°
c) 90°
d) 60°
The area of the sector of a circle with radius 6 cm which subtends an angle of 60^o at the centre of the circle is:
a) {tex}\frac {152}{7}{/tex}cm²
b) {tex}\frac {132}{7}{/tex}cm²
c) {tex}\frac {142}{7}{/tex}cm²
d) {tex}\frac {122}{7}{/tex}cm²
The length of an arc that subtends an angle of 24^o at the centre of a circle with 5 cm radius is
a) {tex}\frac{3 \pi}{2} {/tex} cm
b) {tex}\frac{5 \pi}{3} {/tex} cm
c) {tex}\frac{\pi}{3} {/tex} cm
d) {tex}\frac{2 \pi}{3} {/tex} cm
The probablity that a number selected at random from the numbers 1, 2, 3, …, 15 is a multiple of 4, is
a) {tex}\frac{2}{15}{/tex}
b) {tex}\frac{1}{5}{/tex}
c) {tex}\frac{4}{15}{/tex}
d) {tex}\frac{1}{15}{/tex}
A box contains 90 discs, numbered from 1 to 90. If one disc is drawn at random from the box, the probability that it bears a prime number less than 23, is
a) {tex}\frac{10}{90}{/tex}
b) {tex}\frac{7}{90}{/tex}
c) {tex}\frac{9}{89}{/tex}
d) {tex}\frac{4}{45}{/tex}
The cumulative frequency table is useful in determining
a) All of these
b) Mean
c) Median
d) Mode
Assertion (A): In a solid hemisphere of radius 10 cm, a right cone of same radius is removed out. The surface area of the remaining solid is 570.74 cm² [Take {tex}\pi{/tex} = 3.14 and {tex}\sqrt 2{/tex} = 1.4] Reason (R): Reason (R): Expression used here to calculate Surface area of remaining solid = Curved surface area of hemisphere + Curved surface area of cone
a) Both A and R are true and R is the correct explanation of A.
b) Both A and R are true but R is not the correct explanation of A.
c) A is true but R is false.
d) A is false but R is true.
Assertion (A): If n^th term of an A.P. is 7 – 4n, then its common difference is -4.
Reason (R): Common difference of an A.P. is given by d = a_{n – 1} – a_n
a) Both A and R are true and R is the correct explanation of A.
b) Both A and R are true but R is not the correct explanation of A.
c) A is true but R is false.
d) A is false but R is true.
Download myCBSEguide App for Comprehensive Exam PreparationFor effective exam preparation, download the myCBSEguide.com app, which offers complete study material for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams. With a wide range of practice questions, sample papers, and detailed solutions, myCBSEguide helps students enhance their learning and perform better in exams.Teachers can also utilize the Examin8.com app to create customized exam papers with their own branding, including name and logo, making it easy to assess and engage students. Start preparing today with the myCBSEguide Website and Examin8 Website for a more streamlined and effective study experience.
Section B
An army contingent of 612 members is to march behind an army band of 48 members in a parade. The two groups are to march in the same number of columns. What is the maximum number of columns in which they can march?
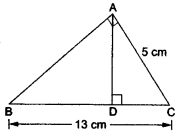
{tex}\triangle ABC{/tex} is a right triangle right-angled at A and {tex}A D \perp B C{/tex}. If BC = 13 cm and AC = 5 cm, find the ratio of the areas of {tex}\triangle ABC{/tex} and {tex}\triangle ADC{/tex}.
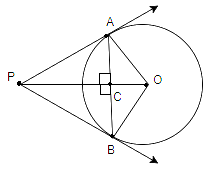
If PA and PB are two tangents drawn from a point P to a circle with centre O touching it at A and B, prove that OP is perpendicular bisector of AB.
Prove that:
{tex}\frac{1+\tan \text A}{2 \sin \text A}+\frac{1+\cot\text A}{2 \cos \text A}{/tex} = cosec A + sec A
OR
If sin{tex}\theta{/tex} + cos {tex}\theta{/tex} = {tex}\sqrt{3}{/tex} , then prove that tan {tex}\theta{/tex} + cot {tex}\theta{/tex} = 1
Find the area of the sector of a circle of radius 7 cm and of central angle 90^o. Also, find the area of corresponding major sector.
OR
A horse is tethered to one corner of a field which is in the shape of an equilateral triangle of side 12 m. If the length of the rope is 7 m, find the area of the field which the horse cannot graze. Take {tex}\sqrt3{/tex} = 1.732. Write the answer correct to 2 places of decimal.
Section C
Prove that {tex}( 5 + 3 \sqrt { 2 } ){/tex} is irrational.
Find the zeroes of the quadratic polynomial 6x²– 3 – 7x and verify the relationship between the zeroes and the coefficients of the polynomial.
How many terms of the AP 9,17,25,… must be taken so that their sum is 636?
OR
If the 8th term of an AP is 37 and the 15th term is 15 more than the 12th term. Find the AP. Hence find the sum of first 15 terms of the AP.
Two concentric circles are of diameters 30 cm and 18 cm. Find the length of the chord of the larger circle which touches the smaller circle.
OR
In figure, O is the centre of a circle. PT and PQ are tangents to the circle from an external point P. If {tex}\angle{/tex}TPQ = 70°, find {tex}\angle{/tex}TRQ.
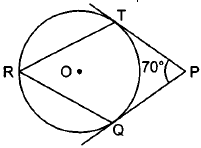
If sec{tex}\theta{/tex} + tan{tex}\theta{/tex} = p, show that {tex}\frac { p ^ { 2 } – 1 } { p ^ { 2 } + 1 } = \sin \theta{/tex}
The frequency distribution given below shows the weight of 40 students of a class. Find the median weight of the students.
Weight (in kg) Number of Students
40 – 45 9
45 – 50 5
50 – 55 8
55 – 60 9
60 – 65 6
65 – 70 3
Section D
Two water taps together can fill a tank in 6 hours. The tap of larger diameter takes 9 hours less than the smaller one to fill the tank separately. Find the time in which each tap can separately fill the tank.
OR
A train covered a certain distance at a uniform speed. If the train would have been 6 km/h faster, it would have taken 4 hours less than the scheduled time. And, if the train were slower by 6 km/h, it would have taken 6 hours more than the scheduled time. Find the length of the journey.
From the top of a 7 m high building, the angle of elevation of the top of a cable tower is 60^o and the angle of depression of its foot is 30^o. Determine the height of the tower.
A toy is in the form of a cylinder of diameter {tex}2 \sqrt { 2 } m{/tex} and height 3.5 m surmounted by a cone whose vertical angle is 90°. Find total surface area of the toy.
OR
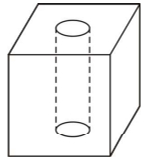
In Figure, from a solid cube of side 7 cm, a cylinder of radius 2.1 cm and height 7 cm is scooped out. Find the total surface area of the remaining solid.
A survey conducted on 20 families in a locality by a group of students resulted in the following frequency table for the number of family members in a family.
Family size
1 – 3
3 – 5
5 – 7
7 – 9
9 – 11
Number of families
7
8
2
4
1
Determine the mean and mode of the above data.
Section E
Read the following text carefully and answer the questions that follow:

Lokesh, a production manager in Mumbai, hires a taxi everyday to go to his office. The taxi charges in Mumbai consists of a fixed charges together with the charges for the distance covered. His office is at a distance of 10 km from his home. For a distance of 10 km to his office, Lokesh paid ₹ 105. While coming back home, he took another route. He covered a distance of 15 km and the charges paid by him were ₹ 155.
1. What are the fixed charges? (1)
2. What are the charges per km? (1)
3. If fixed charges are ₹ 20 and charges per km are ₹ 10, then how much Lokesh have to pay for travelling a distance of 10 km? (2)
  OR
  Find the total amount paid by Lokesh for travelling 10 km from home to office and 25 km from office to home. [Fixed charges and charges per km are as in (i) & (ii). (2)
Read the following text carefully and answer the questions that follow:
Two poles, 30 feet and 50 feet tall, are 40 feet apart and perpendicular to the ground. The poles are supported by wires attached from the top of each pole to the bottom of the other, as in the figure. A coupling is placed at C where the two wires cross.
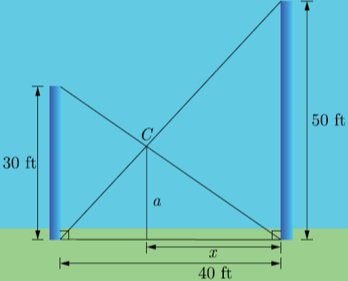
1. What is the horizontal distance from C to the taller pole? (1)
2. How high above the ground is the coupling? (1)
3. How far down the wire from the smaller pole is the coupling? (2)
  OR
  Find the length of line joining the top of the two poles. (2)
Read the following text carefully and answer the questions that follow:
Reena has a 10 m {tex}\times{/tex} 10 m kitchen garden attached to her kitchen. She divides it into a 10 {tex}\times{/tex} 10 grid and wants to grow some vegetables and herbs used in the kitchen. She puts some soil and manure in that and sow a green chilly plant at A, a coriander plant at B and a tomato plant at C. Her friend Kavita visited the garden and praised the plants grown there. She pointed out that they seem to be in a straight line. See the below diagram carefully:
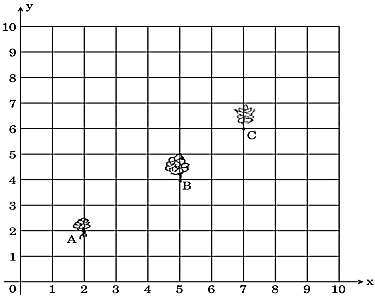
1. Find the distance between A and B? (1)
2. Find the mid-point of the distance AB? (1)
3. Find the distance between B and C? (2)
  OR
  Find the mid point of BC. (2)

Enhance Your Exam Preparation with myCBSEguide App

Looking for the best tools to boost your exam preparation? Download the myCBSEguide.com app, your one-stop solution for comprehensive study material for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams. The app offers a vast collection of practice questions, sample papers, NCERT solutions, and more to help you master every topic and perform better in your exams.

For educators, the Examin8.com app is an ideal platform to create customized question papers with personal branding, including name and logo. Also myCBSEguide and Examin8 apps provide all the resources you need to succeed, whether you’re a student or a teacher.

Class 10 – Mathematics
Maths Standard SP – 01 (2024-25)

Solution

Section A
(d) 2
Explanation: Smallest two digit number is 10 and smallest composite number is 4 .
Clearly, 2 is the greatest factor of 4 and 10, so their H.C.F. is 2.
(b) 4
Explanation: Number of zeros= number of times the graph touches x-axis.
Here the graph touches x-axis 4 times.
(a) no solution
Explanation: Since, we have y = 0 and y = -6 are two parallel lines.
therefore, no solution exists.
(a) {tex}2, \frac{-3}{2}{/tex}
Explanation: The given quadratic equation is 2x² – x – 6 = 0
2x² – x – 6 = 0
{tex}\Rightarrow{/tex} 2x²– 4x + 3x – 6 = 0
{tex}\Rightarrow{/tex} 2x (x-2) + 3 (x-2) = 0
{tex}\Rightarrow{/tex} x – 2 = 0 or 2x + 3 = 0
{tex}\Rightarrow{/tex} x = 2 or x = -3/2
Thus, the roots of the given equation are 2 and -3/2
(b) -50
Explanation: a + 4d = -3 and d = -4
{tex}\Rightarrow{/tex} a = -3 + 16
So, a = 13.
{tex}\therefore{/tex} {tex}S_{10}=\frac{10}{2}[2 a+9 d]=5[2 \times 13+9 \times(-4)]=-50{/tex}.
(b) (2, 0)
Explanation: Let the required point be P(x, 0). Then,
{tex}P A^{2}=P B^{2} \Rightarrow(x+1)^{2}=(x-5)^{2}\\ \Rightarrow x^{2}+2 x+1=x^{2}-10 x+25\\ \Rightarrow 12 x=24 \Rightarrow x=2{/tex}
So, the required point is P(2, 0).
(d) (0,{tex}\frac{-1}{2}{/tex})
Explanation: Centare of circle is equal to mid point of diameter.

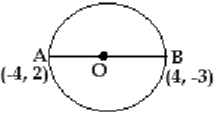
Midpoint (O) = (0,{tex}\frac{-1}{2}{/tex})
Coordinate of O = (0,{tex}\frac{-1}{2}{/tex})
(c) {tex}\frac{9}{2}{/tex}
Explanation: According to Basic Proportionality theorem.
{tex}\frac {\text {AD}}{\text {DB}}{/tex} = {tex}\frac {\text {AE}}{\text {EC}}{/tex}
{tex}\frac 23{/tex} = {tex}\frac 3x{/tex}
x = {tex}\frac 92{/tex}
(c) 65^o
Explanation: OPTQ is a quadrilateral
{tex}\angle{/tex} O+{tex}\angle{/tex} P + {tex}\angle{/tex}T + {tex}\angle{/tex}Q = 360^o
115^o + 90^o + {tex}\angle{/tex}PTQ + 90^o = 360^o
{tex}\angle{/tex} PTQ = 360^o – 295^o
{tex}\angle{/tex} PTQ = 65^o
(d) 3 cm
Explanation: A quadrilateral ABCD is circumscribed to a circle with centre O.
AB = 6 cm, BC = 7 cm, CD = 4 cm, AD = 7 cm
ABCD circumscribed to a circle.
AB + CD = BC + AD
{tex}\Rightarrow{/tex} 6 + 4 = 7 + AD
{tex}\Rightarrow{/tex} 10 = 7 + AD
AD = 10 – 7 = 3 cm
(a) 8
Explanation: 8(cos² A + sin² A) = 8(1) = 8
(a) 3
Explanation: 2tan A = 3
tan A = {tex}\frac32{/tex} ={tex}\frac Pb{/tex}
h = {tex}\sqrt{P^2+b^2}{/tex}
= {tex}\sqrt{3^2+2^2}{/tex}
= {tex}\sqrt{13}{/tex}
Now, Sin A = {tex}\frac Ph{/tex} = {tex}\frac{3}{\sqrt{13}}{/tex}
Cos A = {tex}\frac bh{/tex} = {tex}\frac{2}{\sqrt{13}}{/tex}
{tex}\frac{4Sin\ A + 3Cos\ A}{4Sin\ A-3cos\ A}{/tex}= {tex}\frac {4(\frac {3}{\sqrt {13}}) + 3(\frac {2}{\sqrt {13}})}{4(\frac{3}{\sqrt{13}}) – 3(\frac{2}{\sqrt{13}})}{/tex}
= {tex}\frac{\frac{12}{\sqrt{13}}+\frac{6}{\sqrt{13}}}{\frac{12}{\sqrt{13}} – {\frac{6}{\sqrt{13}}}}{/tex}
= {tex}\frac{18}{6}{/tex} = 3
(a) 30°
Explanation: Let AB be the tower and B be the kite.
Let AC be the horizontal and let BC {tex}\perp{/tex} AC.
Let {tex}\angle{/tex}CAB = {tex}\theta{/tex}.
BC = 30 m and AB = 60 m. Then,
{tex}\frac{B C}{A B}{/tex} = sin {tex}\theta{/tex} {tex}\Rightarrow{/tex} sin {tex}\theta{/tex} = {tex}\frac{30}{60}=\frac{1}{2} \Rightarrow{/tex} sin {tex}\theta{/tex} = sin 30^o {tex}\Rightarrow{/tex} {tex}\theta{/tex} = 30^o.
(b) {tex}\frac {132}{7}{/tex}cm²
Explanation: Angle of the sector is 60°
Area of sector = ({tex}\frac {\theta}{360^o}{/tex}) {tex}\times{/tex} {tex}\pi{/tex}r²
{tex}\therefore{/tex} Area of the sector with angle 60^o = ({tex}\frac {60^o}{360^o}{/tex}) {tex}\times{/tex} {tex}\pi{/tex}r² cm²
= ({tex}\frac {36}{6}{/tex}){tex}\pi{/tex} cm²
= 6 {tex}\times{/tex} ({tex}\frac {22}{7}{/tex}) cm²
= {tex}\frac {132}{7}{/tex} cm²
(d) {tex}\frac{2 \pi}{3} {/tex} cm
Explanation: Given that, {tex}\theta{/tex} = 30^o and r = 4 cm
Length of an arc {tex}=\frac{\theta}{360^{\circ}}(2 \pi r){/tex}
{tex}=\frac{30^{\circ}}{360^{\circ}} \times 2 \pi \times 4{/tex}
{tex}=\frac{2 \pi \times 4}{12}{/tex}
{tex}\therefore{/tex} Length of an arc {tex}=\frac{2 \pi}{3}{/tex}
(b) {tex}\frac{1}{5}{/tex}
Explanation: Total outcomes = 15
({tex}\because{/tex} 15 numbers are given)
Favourable outcomes for a multiple of 4 = 3 (i.e. 4, 8, 12)
{tex}\therefore{/tex} Probability of selecting a number which is a multiple of 4 = {tex}\frac{3}{15}=\frac{1}{5}{/tex}
(d) {tex}\frac{4}{45}{/tex}
Explanation: Number of disc in a box = 90
Numbered on it are 1 to 90 Prime numbers less than 23 are = 2, 3, 5, 7, 11, 13, 17, 19 = 8
Probability of a number being a prime less than {tex}23=\frac{8}{90}=\frac{4}{45}{/tex}
(c) Median
Explanation: Median
(d) A is false but R is true.
Explanation: A is false but R is true.
(a) Both A and R are true and R is the correct explanation of A.
Explanation: Both are correct. Reason is the correct explanation.
Assertion,
a_n = 7 – 4n
d = a_{n – 1} – a_n
= 7 – 4(n + 1) – (7 – 4n)
= 7 – 4n – 4 – 7 + 4n = -4
Download myCBSEguide App for Comprehensive Exam PreparationTo enhance your exam preparation, download the myCBSEguide app today! It offers complete study materials for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams. With an extensive collection of practice questions, sample papers, NCERT solutions, and detailed explanations, the app is designed to help you study smarter and perform better. By regularly practicing Class 10 Maths Sample Papers 2025, students can gain a clear understanding of the exam format and question types.For teachers, the Examin8 app provides an excellent platform to create personalized question papers with your own name, logo, and branding. Whether you’re a student or an educator, myCBSEguide and Examin8 are the perfect apps to simplify your exam preparation and assessment process and for more visit myCBSEGuide Website and Examin8 Website.
Section B
Clearly, the maximum number of columns = HCF (612, 48).

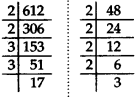
Now, {tex} 612 {/tex} ={tex}2 \times 2 \times 3 \times 3 \times 17{/tex} {tex}= \left( 2 ^ { 2 } \times 3 ^ { 2 } \times 17 \right){/tex}
and {tex} 48= 2 \times 2 \times 2 \times 2 \times 3 = \left( 2 ^ { 4 } \times 3 \right){/tex}.
{tex} \therefore{/tex} HCF (612, 48) {tex} = \left( 2 ^ { 2 } \times 3 \right) = ( 4 \times 3 ) {/tex}
{tex} \therefore{/tex} HCF (612, 48)= 12.
{tex} \therefore{/tex} Maximum number of columns in which they can march = 12.
Given, BC = 13 cm and AC = 5 cm and {tex}A D \perp B C{/tex}

In {tex}\triangle BAC{/tex} and {tex}\triangle A D C {/tex}
{tex}\angle A = \angle D =90^\circ{/tex}
{tex}\angle A C B = \angle D C A{/tex} (common)
{tex}\triangle \mathrm { BAC } \sim \triangle \mathrm { ADC }{/tex}[by AA similarity] {tex}\therefore \frac {AC}{AD} = \frac {BC}{AC} = \frac {AB}{DC}{/tex}
{tex}\therefore \frac { \operatorname { ar } ( \triangle A B C ) } { \operatorname { ar } ( \triangle A D C ) }{/tex}{tex}=\frac {\frac{1}{2} AB . AC}{\frac {1}{2}DC. AD} = \frac { B C ^ { 2 } } { A C ^ { 2 } }{/tex}
{tex}= \frac { 13 ^ { 2 } } { 5 ^ { 2 } } = \frac { 169 } { 25 }{/tex}
Therefore, the ratio of the areas of {tex}\triangle ABC{/tex} and {tex}\triangle ADC{/tex} {tex}= 169 : 25.{/tex}
Let OP intersect AB at a point C,

Clearly, {tex}\angle APO=\angle BPO{/tex}…(i) [{tex}\because{/tex} O is bisector of {tex}\angle APB{/tex}] Now, in {tex}\triangle ACP{/tex} and {tex}\triangle BCP{/tex}.
{tex}AP\;=\;BP{/tex} [{tex}\because{/tex} length of tangents drawn from an external point to a circle are equal ] {tex}PC\;=\;PC{/tex} [{tex}\because{/tex} common sides] and {tex}\angle APO=\angle BPO{/tex} [From Eq.(i)] {tex}\therefore \triangle ACP \cong \triangle BCP{/tex} [by SAS congruence rule] Then, {tex}AC\;=\;BC{/tex} [by congruence side of congruence triangle] and {tex}\angle ACP=\angle BCP{/tex} [by congruence angle of congruence triangle] {tex}\angle ACP=\angle BCP{/tex}{tex}=\frac{1}{2}\times 180^o=90^o{/tex} [AB is a straight line] Hence, OP is a perpendicular bisector of AB.
LHS = {tex}\frac{1+\tan A}{2 \sin A}+\frac{1+\cot A}{2 \cos A}{/tex}
= {tex}\frac{\cos A+\sin A}{2 \sin A \cos A}+\frac{\sin A+\cos A}{2 \cos A \sin A}{/tex}
= {tex}\frac{2(\cos A+\sin A)}{2 \sin A \cos A}{/tex}
= cosec A + sec A
= RHS
OR
Given that: sin {tex}\theta{/tex} + cos {tex}\theta{/tex} ={tex}\sqrt{3}{/tex}
or (sin {tex}\theta{/tex} + cos {tex}\theta{/tex})² = 3
or sin² {tex}\theta{/tex} + cos² {tex}\theta{/tex} + 2sin{tex}\theta{/tex} cos{tex}\theta{/tex} = 3
2sin{tex}\theta{/tex} cos{tex}\theta{/tex} = 2 [As sin² {tex}\theta{/tex} + cos² {tex}\theta{/tex} = 1] or sin {tex}\theta{/tex} cos {tex}\theta{/tex} = 1 = sin² {tex}\theta{/tex} + cos²{tex}\theta{/tex}
LHS=1
RHS={tex}\frac{Sin \theta}{Cos \theta} + \frac{Cos \theta}{Sin \theta}{/tex}
Taking LCM
Substitute the value of LHS in RHS we get,
tan{tex}\theta{/tex} + cot{tex}\theta{/tex} = 1
Given that,
Radius of circle = 7 cm
Central angle = 90^o
Now, area of minor sector of circle
= {tex}\frac{\pi r^2 \theta}{360^{\circ}}{/tex}
= {tex}\frac{\pi(7)^2}{4}{/tex}
= {tex}\frac{22 \times 7 \times 7}{7 \times 4}{/tex}
= 38.5 cm²
Area of complete circle
= {tex}\pi{/tex}r²
= {tex}\pi{/tex}(7)²
= 154 cm²
Now, area of major sector
= Area of complete circle – Area of minor sector
= 154 – 38.5
= 115.5 cm²
OR

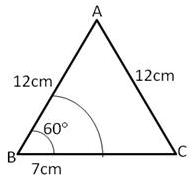
Area which cannot be grazed = (area of equilateral {tex}\triangle ABC{/tex} – (area of the sector with r = 7m,{tex}\theta = 60 ^ { \circ }{/tex})
{tex}= \left[ \frac { \sqrt { 3 } } { 4 } \times ( 12 ) ^ { 2 } – \frac { 22 } { 7 } \times ( 7 ) ^ { 2 } \times \frac { 60 } { 360 } \right] \mathrm { m } ^ { 2 }{/tex}
{tex}= \left[ ( \sqrt { 3 } \times 12 \times 3 ) – \frac { ( 22 \times 7 ) } { 6 } \right]{/tex}
= 62.35 – 25.66 m²
= 36.68 m²
Section C
Let {tex}5 + 3 \sqrt { 2 }{/tex} is rational. It can be written in the form {tex} \frac pq{/tex}.
{tex}(5 + 3 \sqrt { 2 }) = \frac pq{/tex}
{tex} 3 \sqrt { 2 } = \frac pq – 5{/tex}
{tex} 3 \sqrt { 2 } = \frac{ p – 5q}q {/tex}
{tex} \sqrt { 2 } = \frac{ p – 5q}{3q} {/tex}
As p – 5q and 3q are integers .
So, {tex}\frac{ p – 5q}{3q} {/tex} is rational number .
But {tex} \sqrt { 2 }{/tex} is not rational number .
Since a rational number cannot be equal to an irrational number. Our assumption that {tex}5 + 3 \sqrt { 2 }{/tex} is rational wrong.
Hence, {tex}5 + 3 \sqrt { 2 }{/tex} is irrational.
The given polynomial is
p(x) = 6x²-7x -3
Factorize the above quadratic polynomial, we have
6x²-7x -3 = 6x²-9x + 2x – 3
= 3x(2x – 3) + 1(2x – 3)
= (3x + 1)(2x – 3)
For p(x) = 0, either 3x + 1 = 0 or 2x – 3 = 0
{tex}\Rightarrow \quad x = \frac { – 1 } { 3 } \text { or } x = \frac { 3 } { 2 }{/tex}
Verification:we have a = 6, b = -7, c = -3
Sum of zeroes = {tex}\frac { – 1 } { 3 } + \frac { 3 } { 2 } = \frac { 7 } { 6 }{/tex}
Also, {tex}\frac { – b } { a } = \frac { – ( – 7 ) } { 6 } = \frac { 7 } { 6 }{/tex}
Now, product of zeroes= {tex}\left( – \frac { 1 } { 3 } \right) \times \frac { 3 } { 2 } = \frac { – 1 } { 2 }{/tex}
Also, {tex}\frac { c } { a } = \frac { – 3 } { 6 } = \frac { – 1 } { 2 } {/tex}
According to the question,we have,
a=9. Therefore, common difference d =17-9=8
let the required number of terms be n.
Therefore, S_n=636
{tex}\Rightarrow{/tex}{tex}\frac{n}{2}{/tex}[2a+(n-1)d]=636
{tex}\Rightarrow{/tex}{tex}\frac{n}{2}{/tex}[2(9)+(n-1)8]=636
{tex}\Rightarrow{/tex}n[18+8n-8]=1272
{tex}\Rightarrow{/tex}8n²+10n-1272=0
{tex}\Rightarrow{/tex}4n²+5n-636=0
{tex}\Rightarrow{/tex}4n²+53n-48n-636=0
{tex}\Rightarrow{/tex}n(4n+53)-12(4n+53)=0
{tex}\Rightarrow{/tex}(4n+53)(n-12)=0
{tex}\Rightarrow{/tex}4n+53=0 or n-12=0
{tex}\Rightarrow{/tex}n={tex}\frac{{ – 53}}{4}{/tex} or n=12
Since number of terms cannot neither be negative nor fraction, n=12
hence, the required number of terms is 12.
OR
Let 1st term of the AP be a and common difference be d.
Now a₈ = 37
{tex}\Rightarrow{/tex} a + 7d = 37
{tex}\Rightarrow{/tex} a = 37 – 7d ..(i)
Also, a₁₅ = a₁₂+ 15
{tex}\Rightarrow{/tex} a + 14d = a + 11d + 15
{tex}\Rightarrow{/tex} 3d = 15 {tex}\Rightarrow{/tex} d = 5
Substituting in eq. (i), we get
a = 37 – 7(5) = 2
AP. is 2, 7, 12, 17, …
S₁₅ = {tex}\frac{15}{2}[2 \times 2+14 \times 5]{/tex}
= 15(37) = 555
Let {tex}OA = R = \frac{{30}}{2} = 15cm{/tex}, {tex}OB = r = \frac{{18}}{2} = 9cm{/tex}

Since AC is the tangent to the circle with radius 9 cm, we have OB⊥AC.
Hence, by applying the Pythagoras Theorem, we have,
OA² = OB² + AB²
{tex}\Rightarrow{/tex} 15² = 9² + AB²
{tex}\Rightarrow{/tex} AB² = 15² – 9²
{tex}\Rightarrow{/tex} AB² = 225 – 81 = 144
{tex}\therefore{/tex} AB = 12 cm
We know that the perpendicular drawn from the centre of a circle to any chord of the circle bisects the chord.
Here, OB is the perpendicular and AC is the length of the chord of the circle with radius 15 cm.
So,
AC = 2 {tex}\times{/tex} AB = 2 {tex}\times{/tex} 12 = 24 cm
Length of the chord of the larger circle which touches the smaller circle = 24 cm.
OR

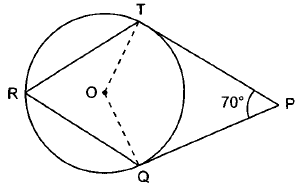
we know that , angle subtended by an arc at centre of the circle is twice the angle subtended by it in alternate segment.
{tex}\angle \mathrm { TOQ } + \angle \mathrm { TPQ } = 180 ^ { \circ }{/tex}
{tex}\Rightarrow{/tex}{tex}\angle \mathrm { TOQ } = 110 ^ { \circ }{/tex}
{tex}\angle \mathrm { TOQ } = 2 \angle \mathrm { TRQ }{/tex}
{tex}\Rightarrow{/tex} {tex}110 ^ { \circ } = 2 \angle \mathrm { TRQ } \Rightarrow \angle \mathrm { TRQ } = 55 ^ { \circ }{/tex}
We have,
{tex}\mathrm { LHS } = \frac { p ^ { 2 } – 1 } { p ^ { 2 } + 1 } = \frac { ( \sec \theta + \tan \theta ) ^ { 2 } – 1 } { ( \sec \theta + \tan \theta ) ^ { 2 } + 1 }{/tex}
{tex}\Rightarrow \quad \mathrm { LHS } = \frac { \sec ^ { 2 } \theta + \tan ^ { 2 } \theta + 2 \sec \theta \tan \theta – 1 } { \sec ^ { 2 } \theta + \tan ^ { 2 } \theta + 2 \sec \theta \tan \theta + 1 }{/tex}
{tex}\Rightarrow \quad \mathrm { LHS } = \frac { \left( \sec ^ { 2 } \theta – 1 \right) + \tan ^ { 2 } \theta + 2 \sec \theta \tan \theta } { \sec ^ { 2 } \theta + 2 \sec \theta \tan \theta + \left( 1 + \tan ^ { 2 } \theta \right) }{/tex}
{tex}\Rightarrow \quad \mathrm { LHS } = \frac { \tan ^ { 2 } \theta + \tan ^ { 2 } \theta + 2 \sec \theta \tan \theta } { \sec ^ { 2 } \theta + 2 \sec \theta \tan \theta + \sec ^ { 2 } \theta }{/tex}
{tex}\Rightarrow \quad \mathrm { LHS } = \frac { 2 \tan ^ { 2 } \theta + 2 \tan \theta \sec \theta } { 2 \sec ^ { 2 } \theta + 2 \sec \theta \tan \theta } = \frac { 2 \tan \theta ( \tan \theta + \sec \theta ) } { 2 \sec \theta ( \sec \theta + \tan \theta ) } = \frac { \tan \theta } { \sec \theta } = \frac { \sin \theta } { \cos \theta \cdot \sec \theta } = \sin \theta{/tex}=RHS
The frequency distribution table for calculations of median is as follow:
Class Interval Frequency (f) Cumulative frequency
40-45 9 9
45-50 5 14
50-55 8 22
55-60 9 31
60-65 6 37
65-70 3 40
We know,
Median = {tex}l+\left\{h \times \frac{\frac{N}{2}-c f}{f}\right\}{/tex}
Here,
l denotes lower limit of median class
h denotes width of median class
f denotes frequency of median class
cf denotes cumulative frequency of the class preceding the median class
N denotes sum of frequency
{tex}\frac {N}{2}{/tex} = 20
cumulative frequency just greater than 20 is 22
Therefore, median class is 50-55
Median class is 50 – 55
So,
l = 50,
h = 5,
f = 8,
cf = cf of preceding class = 14
{tex}\frac {N}{2}{/tex} = 20
O substituting all the above values in the formula, we get
Median = {tex}l+\left\{\mathrm{h} \times \frac{\frac{\mathrm{N}}{2}-c f}{f}\right\}{/tex}
Median = {tex}50+\left\{5 \times \frac{20-14}{8}\right\}{/tex}
Median = {tex}50+\left\{5 \times \frac{6}{8}\right\}{/tex}
Median = {tex}50+\left\{5 \times \frac{3}{4}\right\}{/tex}
Median = {tex}50+\left\{\frac{15}{4}\right\}{/tex}
Median = 50 + 3.75
{tex}\Rightarrow{/tex} Median = 53.75
Section D
Let the time taken by the smaller pipe to fill the tank be x hr.
The time is taken by the larger pipe = (x – 9)hr.
Part of the tank filled by a smaller pipe in 1 hour = {tex}\frac { 1 } { x }{/tex}
Part of the tank filled by a larger pipe in 1 hour = {tex}\frac { 1 } { x – 9 }{/tex}
According to question,
{tex}\Rightarrow \frac { 1 } { x } + \frac { 1 } { x – 9 } = \frac { 1 } { 6 }{/tex}
{tex}\Rightarrow \frac { x – 9 + x } { x ^ { 2 } – 9 x } = \frac { 1 } { 6 }{/tex}
{tex}\Rightarrow{/tex} 6(2x – 9) = x² – 9x
{tex}\Rightarrow{/tex} 12x – 54 = x²– 9x
{tex}\Rightarrow{/tex} x² – 21x + 54 = 0
{tex}\Rightarrow{/tex} x² – 18x – 3x + 54 = 0
{tex}\Rightarrow{/tex} x(x – 18) – 3(x – 18) = 0
{tex}\Rightarrow{/tex} x – 18 = 0 or x – 3 = 0
{tex}\Rightarrow{/tex} x = 18 or x = 3
As time can’t be less than 9. Then, x {tex}\neq{/tex} 3.
{tex}\therefore{/tex} x = 18
{tex}\Rightarrow{/tex} x – 9 = 18 – 9 = 9
Therefore, the smaller pipe and larger pipe take 18 hours and 9 hours to fill the tank respectively.
OR
Suppose the actual speed of the train be x km/hr and the actual time taken be y hours. Then,
Distance covered =(xy) km ………..(i) [{tex}\therefore{/tex} Distance = Speed {tex}\times{/tex}Time] If the speed is increased by {tex}6km/hr{/tex}, then time of journey is reduced by 4 hours i.e., when speed is {tex}(x + 6)km/hr{/tex}, time of journey is {tex}(y-4)hours.{/tex}
{tex}\therefore{/tex} Distance covered = {tex}(x + 6) (y – 4){/tex}
{tex}\Rightarrow{/tex}{tex}xy = (x + 6) (y – 4){/tex} [Using (i)] {tex}\Rightarrow{/tex}{tex}-4x + 6y -24=0{/tex}
{tex}\Rightarrow{/tex}{tex}-2x + 3y -12=0{/tex} ……….(ii)
when the speed is reduced by 6 km/hr, then the time of journey is increased by 6 hours i.e.,
when speed is {tex}(x-6)km/hr{/tex}, time of journey is {tex}(y – 6)hours.{/tex}
{tex}\therefore{/tex} Distance covered ={tex}(x -6) (y + 6){/tex}
{tex}\Rightarrow{/tex} {tex}xy = (x – 6) (y + 6){/tex} [Using (i)] {tex}\Rightarrow{/tex} {tex}6x – 6y -36 =0{/tex}
{tex}\Rightarrow{/tex} {tex}x – y -6=0 {/tex}………..(iii)
Thus, we obtain the following system of equations:
{tex}-2x + 3y -12 =0{/tex}
{tex}x – y – 6=0{/tex}
By using cross-multiplication, we have
{tex}\frac { x } { 3 \times – 6 – ( – 1 ) \times – 12 } = \frac { – y } { – 2 \times – 6 – 1 \times – 12 } = \frac { 1 } { – 2 \times – 1 – 1 \times 3 }{/tex}
{tex}\Rightarrow \quad \frac { x } { – 30 } = \frac { – y } { 24 } = \frac { 1 } { – 1 }{/tex}
{tex}\Rightarrow{/tex} {tex}x =30\ and\ y=24.{/tex}
Putting the values of x and y in equation (i), we obtain
Distance= {tex}(30\times24)km =720km.{/tex}
Hence, the length of the journey is {tex}720 km{/tex}.
Given,

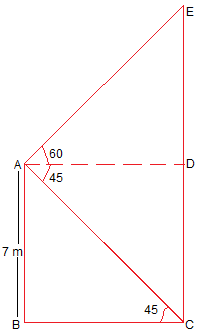
AB be the building.
EC be the tower.
A is the point from where elevation of tower is 60° and the angle of depression of its foot is 45^o
{tex}EC = DE + CD{/tex}
also, {tex}CD = AB = 7 m.{/tex}
and {tex} BC = AD{/tex}
In right {tex}\triangle{/tex}ABC,
tan45^o ={tex}\frac{AB}{BC}{/tex}
{tex}\Rightarrow{/tex} 1 = {tex}\frac{7}{BC}{/tex}
{tex}\Rightarrow{/tex}{tex} BC = 7 m = AD{/tex}
In right {tex}\triangle{/tex}ADE,
{tex}\tan 60^{\circ}{/tex} = {tex}\frac{DE}{AD}{/tex}
{tex}\Rightarrow{/tex} {tex}\sqrt 3{/tex} = {tex}\frac{DE}{7}{/tex}
{tex}\Rightarrow{/tex} DE = 7{tex}\sqrt 3{/tex}
Height of the tower{tex} = EC = DE + CD = {/tex}(7{tex}\sqrt 3{/tex} + 7) m = 7({tex}\sqrt 3{/tex} + 1) m.
According to question, {tex}\angle C = 90 ^ { \circ }{/tex}
Let, AC = BC = x
According to pythagoras theorem,
{tex}\therefore A B ^ { 2 } = A C ^ { 2 } + B C ^ { 2 }{/tex}
{tex}A B ^ { 2 } = x ^ { 2 } + x ^ { 2 }{/tex}
{tex}\therefore 2 x ^ { 2 } = ( 2 \sqrt { 2 } ) ^ { 2 }{/tex}
or, x = 2m
{tex}r = \sqrt { 2 } \mathrm { m }{/tex} (given)
Radius of the cylinder = Radius of the cone
{tex}\therefore{/tex} Slant height of conical portion, x = 2 m
Total surface area of toy = curved surface area of cylinder + Area of base of cylinder + Curved surface area of cone
{tex}= 2 \pi r h + \pi r ^ { 2 } + \pi r l{/tex}
{tex}= \pi r( 2h + r + l){/tex}
{tex}= \pi r [ 7 + \sqrt { 2 } + 2 ] m ^ { 2 }{/tex}
{tex}= \pi \sqrt { 2 } [ 9 + \sqrt { 2 } ] m ^ { 2 }{/tex}
{tex}= \pi [ 2 + 9 \sqrt { 2 } ] \mathrm { m } ^ { 2 }{/tex}
The total surface area of the toy {tex}= \pi [ 2 + 9 \sqrt { 2 } ] \mathrm { m } ^ { 2 }{/tex}
OR
We have;
A Cube,
Cube’s {tex}\frac {length}{Edge}{/tex}, a = 7 cm
A Cylinder:
Cylinder’s Radius, r = 2.1 cm or r = {tex}\frac {21}{10}{/tex}cm
Cylinder’s Height, h = 7 cm
{tex}\because{/tex} A cylinder is scooped out from a cube,
{tex}\therefore{/tex} TSA of the resulting cuboid:
= TSA of whole Cube – 2 {tex}\times{/tex} (Area of upper circle or Area of lower circle) + CSA of the scooped out Cylinder
= 6a² + 2{tex}\pi{/tex}rh – 2 {tex}\times{/tex} ({tex}\pi{/tex}r²)
= 6 {tex}\times{/tex} (7)² + 2 {tex}\times{/tex} (22 {tex}\div{/tex} 7 {tex}\times{/tex} 2.1 {tex}\times{/tex} 7) – 2 {tex}\times{/tex} [22 {tex}\div{/tex} 7 {tex}\times{/tex} (2.1)²] = 6 {tex}\times{/tex} 49 + (44 {tex}\div{/tex} 7 {tex}\times{/tex} 14.7) – (44 {tex}\div{/tex} 7 {tex}\times{/tex} 4.41)
= 294 + 92.4 – 27.72
= 294 + 64.68
= 358.68 cm²
Hence, the total surface area of the remaining solid is 358.68 cm²
Family size X_i F_i f_ix_i
1 – 3 2 7 14
3 – 5 4 8 32
5 – 7 6 2 12
7 – 9 8 2 16
9 – 11 10 1 10
20 84
Mean = {tex}\frac{\sum \mathrm{f}_{\mathrm{i}} \mathrm{x}_{\mathrm{i}}}{\sum \mathrm{f}_{\mathrm{i}}}{/tex} = {tex}\frac{84}{20}{/tex} = 4.2
Mode : Modal Class = 3-5
I = 3, f1 = 8, f0 = 7, f2 = 2, h = 2
Mode = l + {tex}\left(\frac{\mathrm{f}_1-\mathrm{f}_0}{2 \mathrm{f}_1-\mathrm{f}_0-\mathrm{f}_2}\right){/tex} {tex}\times{/tex} h
= 3 + {tex}\left(\frac{8-7}{16-7-2}\right){/tex} {tex}\times{/tex} 2
= {tex}\frac{23}{7}{/tex} or 3.287
Section E
1. Let the fixed charge be ₹ x and per kilometer charge be ₹ y
  {tex}\therefore{/tex} x + 10y = 105 …(i)
  x + 15y = 155 …(ii)
  From (i) and (ii)
  5y = 50
  y = {tex}\frac{50}{5}{/tex} = 10
  From equation (i)
  x + 100 = 105
  x = 105 – 100 = 5
  Fixed charges = ₹ 5
2. Let the fixed charge be ₹ x and per kilometer charge be ₹ y
  {tex}\therefore{/tex} x + 10y = 105 …(1)
  x + 15y = 155 …(2)
  From (1) and (2)
  5y = 50
  y = {tex}\frac{50}{5}{/tex} = 10
  From equation (1)
  x + 100 = 105
  x = 105 – 100 = 5
  Per km charges = ₹ 10
3. Let the fixed charge be ₹ a and per kilometer charge be ₹ b
  a + 10b
  20 + 10 {tex}\times{/tex} 10 = ₹ 120
  OR
  Total amount = x + 10y + x + 25y
  = 2x + 35y
  = 2 {tex}\times{/tex} 5 + 35 {tex}\times{/tex} 10
  = 10 + 350
  = ₹ 360
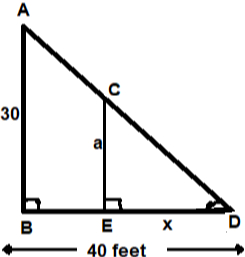
1. {tex}\triangle{/tex}ABD {tex}\sim{/tex} {tex}\triangle{/tex}CED (by AA criteria)
  {tex}\frac{30}{a}=\frac{40}{x}{/tex}
  {tex}\frac{x}{a}=\frac{40}{30}{/tex}
  a = {tex}\frac{30}{40}x{/tex}
  Again
  
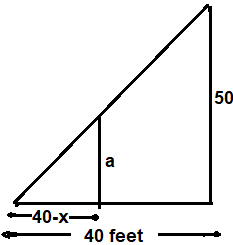
  {tex}\frac{40-x}{40}=\frac{a}{50}{/tex}
  {tex}\frac{40-x}{40}=\frac{30\times x}{40\times50}{/tex}
  8000 – 200x = 120x
  8000 = 320x
  {tex}\therefore{/tex} x = 25 feet
2. {tex}\frac{x}{40}=\frac{a}{30}{/tex}
  {tex}\frac{25}{40}=\frac{a}{30}{/tex}
  {tex}\frac{25\times30}{40}=a{/tex}
  {tex}\frac{75}{4}{/tex} = a
  a = 18.75 feet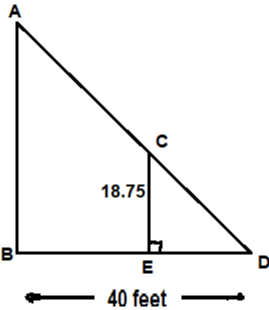
3. AD = {tex}\sqrt{{30^2+40}^2}{/tex}
  {tex}= \sqrt{900+1600}{/tex}
  {tex}=\sqrt{2500}{/tex}
  AD = 50 feet
  In {tex}\triangle{/tex}CED
  CD = {tex}\sqrt{18.75^2+25^2}{/tex}
  {tex}= \sqrt{976.5625}{/tex}
  = 31.25 feet
  AC = AD – CD
  = 50 – 31.25
  = 18.75 feet
  OR
  {tex}\sqrt{40^2+20^2}{/tex}
  {tex}= \sqrt{1600+400}{/tex}
  {tex}=\sqrt{2000}{/tex}
  {tex}= 20\sqrt{5}{/tex} feet
1. AB = {tex}\sqrt{{(5-2)}^2+{(4-2)^2}}{/tex}
  {tex}= \sqrt{9+4}{/tex}
  {tex}= \sqrt{13}{/tex}
2. Middle point AB = {tex}\left(\frac{2+5}2,\frac{2+4}2\right){/tex}
  = (3.5, 3)
3. BC = {tex}\sqrt{{(7-5)^2}+{(6-4)^2}}{/tex}
  {tex}= \sqrt{4+4}{/tex}
  {tex}= 2 \sqrt{2}{/tex}
  OR
  
  Middle point of BC = {tex}\left(\frac{5+7}2,\frac{4+6}2\right){/tex}
  = (6, 5)

Class Interval	Frequency (f)	Cumulative frequency
40-45	9	9
45-50	5	14
50-55	8	22
55-60	9	31
60-65	6	37
65-70	3	40

Boost Your Exam Preparation with myCBSEguide App

For thorough exam practice and effective preparation, download the myCBSEguide app. It offers complete study material for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams. The app provides access to an extensive range of practice questions, sample papers, and NCERT solutions to help you prepare comprehensively and improve your exam performance. The Class 10 Maths Sample Papers 2025 are an essential resource for students preparing for their upcoming exams.

Teachers can also take advantage of the Examin8 app, which allows them to create customized exam papers featuring their own name and logo, making it easier to conduct personalized assessments. Whether you’re a student aiming to excel or a teacher designing tailored exams, myCBSEguide and Examin8 Website are your go-to tools for academic success.

Login into our student dashboard for more.

Download myCBSEguide App

Sample Papers for Class 10 2024-25 in PDF

Download Class 10 Sample Papers 2025 for Mathematics, Science, English, and More on myCBSEguide

To practice effectively and excel in your exams, download the Sample Papers for Class 10 Mathematics 2025, Science, Social Science, English Communicative, English Language and Literature, Hindi Course A, and Hindi Course B from the myCBSEguide app or myCBSEguide website. myCBSEguide offers sample papers with solutions, chapter-wise test papers, NCERT solutions, and NCERT Exemplar solutions to help students improve their understanding and problem-solving skills.

Additionally, the app provides quick revision notes, CBSE guess papers, and important question papers, ensuring you are well-prepared for the board exams. Available through the myCBSEguide app and website, these resources are perfect for CBSE students who want to perform at their best in the upcoming exams.

Test Generator

Create question paper PDF and online tests with your own name & logo in minutes.

Create Now

myCBSEguide

Question Bank, Mock Tests, Exam Papers, NCERT Solutions, Sample Papers, Notes