CBSE Class 12 Physics Chapter 13 Nuclei Important Questions

CBSE Class 12 Physics Chapter 13 Nuclei Important Questions. We know Physics is tough subject within the consortium of science subjects physics is an important subject. But if you want to make career in these fields like IT Consultant, Lab Technician, Laser Engineer, Optical Engineer etc. You need to have strong fundamentals in physics to crack the exam. myCBSEguide has just released Chapter Wise Question Answers for class 12 Physics. There chapter wise Extra Questions with complete solutions are available for download in myCBSEguide website and mobile app. These Questions with solution are prepared by our team of expert teachers who are teaching grade in CBSE schools for years. There are around 4-5 set of solved Physics Extra Questions from each and every chapter. The students will not miss any concept in these Chapter wise question that are specially designed to tackle Board Exam. We have taken care of every single concept given in CBSE Class 12 Physics syllabus and questions are framed as per the latest marking scheme and blue print issued by CBSE for class 12.

Class 12 Physics Extra Questions

Download as PDF

Extra Questions for Class 12 Physics Chapter 13

Class – 12 Physics (Nuclei)

---

1. The half life of I¹³¹ is 8 days. Given a sample of I¹³¹ at time t = 0, we can assert that:

   1. a given nucleus may decay after t = 0
   2. no nucleus will decay before t = 8 days
   3. no nucleus will decay before t = 4 days
   4. all nuclei will decay before t = 16 days

2. The radioactive decay of uranium into thorium is represented by the equation {tex}{}_{92}^{238}U \to {}_{90}^{234}Th + x{/tex} What is x?

   1. an alpha particle
   2. an electron
   3. a neutron
   4. proton

3. The binding energy of the nucleus {tex}E_b{/tex} is related to mass defect Δm by

   1. {tex}{{\rm{E}}_{\rm{b}}} = {\rm{ }}\Delta {\rm{m }}{{\rm{c}}^{\rm{3}}}{/tex}
   2. {tex}{{\rm{E}}_{\rm{b}}} = {\rm{ }}\Delta {\rm{m }}{{\rm{c}}^{\rm{2}}}{/tex}
   3. {tex}{{\rm{E}}_{\rm{b}}} = {\rm{ }}\Delta {\rm{m }}{{\rm{c}}^{\rm{4}}}{/tex}
   4. {tex}{{\rm{E}}_{\rm{b}}} = {\rm{ 2}}\Delta {\rm{m }}{{\rm{c}}^{\rm{2}}}{/tex}

4. Plutonium decays with half life of 24000 years. If plutonium is stored for 72000 years, the fraction of it that remains is

   1. 1/8
   2. 1/4
   3. 1/3
   4. 1/2

5. Which of the following particles has similar mass to that of the protons?

   1. Neutrino
   2. Neutron
   3. Positron
   4. Proton

6. Two nuclei have mass numbers in the ratio 1: 8. What is the ratio of their nuclear radii?

7. A nucleus {tex}_{92}^{238}U{/tex} undergoes through {tex}\alpha {/tex}-decay and transforms to thorium. What is

   1. the mass number
   2. atomic number of the daughter nucleus produced?

8. Draw a plot of the binding energy per nucleon as a function of mass number for a large number of nuclei 20 < A < 240. How do you explain the constancy of binding energy per nucleon in the range of 30 < A < 170 using the property that nuclear force is short-ranged?

9. A nucleus with mass number A = 240 and BE/A= 7.6 MeV breaks into two fragments each of A = 120 with BE/ A= 8.5 MeV. Calculate the released energy.

10. How the size of a nucleus is experimentally determined? Write the relation between the radius and mass number of the nucleus. Show that the density of the nucleus is independent of its mass number.

11. Obtain approximately the ratio of the nuclear radii of the gold isotope {tex}_{79}^{197}Au{/tex} and the silver isotope {tex}_{47}^{107}Ag{/tex}.

12. Draw a plot of potential energy between a pair of nucleons as a function of their separation. Mark the regions where potential energy is

    1. positive and
    2. negative.

13. Explain by giving necessary reactions, how energy is released during (i) fission? (ii) fusion?

14. A given coin has a mass of 3.0 g. Calculate the nuclear energy that would be required to separate all the neutrons and protons from each other. For simplicity assume that the coin is entirely made of {tex}_{29}^{63}Cu{/tex} atoms ( of mass 62.92960 u)

15. Distinguish between nuclear fission and fusion. Show how in both these processes energy is released.
    Calculate the energy release in MeV in the deuterium-tritium fusion reaction.
    {tex}_ { 1 } ^ { 2 } \mathrm { H } + _ { 1 } ^ { 3 } \mathrm { H } \longrightarrow _ { 2 } ^ { 4 } \mathrm { He } + n{/tex}
    Using the data
    {tex}m \left( 1 ^ { 2 } H \right) = 2.014102 u , m \left( _ { 1 } ^ { 3 } H \right) = 3.016049 u{/tex}
    {tex}m \left( _ { 2 } ^ { 4 } \mathrm { He } \right) = 4.002603 \mathrm { u } , \mathrm { m } _ { n } = 1.008665 \mathrm { u }{/tex}
    {tex}1 \mathrm { u } = 931.5 \frac { \mathrm { MeV } } { \mathrm { c } ^ { 2 } }{/tex}

Class – 12 Physics (Nuclei)
Answers

---

1. 1. a given nucleus may decay after t = 0
      Explanation: The sample will be halved in t = 8 days and will be {tex}\frac{1}{4}{/tex}th of the initial size in t = 16 days. So any given nucleus may decay after t = 0.
   1. an alpha particle
      Explanation: {tex}\lambda – decay{/tex}
      {tex}_Z{X^A}{ \to _{Z – 2}}{Y^{A – 4}}{ + _2}H{e^4} + \mathop Q\limits_{(Energy)} {/tex}
      {tex}_{92}{U^{238}}{ \to _{90}}T{h^{254}} + \mathop {_2H{e^4}}\limits_{\lambda \;particle} + \mathop Q\limits_{(Energy)} {/tex}
   2. {tex}{{\rm{E}}_{\rm{b}}} = {\rm{ }}\Delta {\rm{m }}{{\rm{c}}^{\rm{2}}}{/tex}
      Explanation: This follows from the mass energy equivalence relation of the special theory of relativity, proposed by Einstein.
   1. 1/8
      Explanation: {tex}\frac{N}{{{N_o}}} = {\left( {\frac{1}{2}} \right)^n}{/tex}
      {tex}n = \frac{t}{{{T_{1/2}}}} = \frac{{72000}}{{24000}}{/tex}
      {tex}\frac{N}{{{N_o}}} = {\left( {\frac{1}{2}} \right)^3} = \frac{1}{8}{/tex}
   2. Neutron
      Explanation: Neutrons and protons have equal masses which is 1.6 × 10^-27Kg.
2. Radius of nucleus is related to its mass number as {tex}R = R _ { 0 } A ^ { 1 / 3 }{/tex}
   where, R₀ = base radius (1.2 femtometre) and A = mass number
   {tex}\therefore \quad \frac { R _ { 1 } } { R _ { 2 } } = \left( \frac { A _ { 1 } } { A _ { 2 } } \right) ^ { 1 / 3 } = \left( \frac { 1 } { 8 } \right) ^ { 1 / 3 } = \frac { 1 } { 2 }{/tex}
   {tex}R _ { 1 } \cdot R _ { 2 } = 1 : 2{/tex}
   so nuclear radius of latter will be two times that of former as masses are in ratio 1:8.
3. When any nucleus goes through {tex}\alpha {/tex}-decay, the mass number of parent nucleus decreases by 4 units and atomic number decreases by 2 units.
   {tex}\therefore {\quad ^{238}}{{\text{U}}_{92}}{\xrightarrow{{\alpha – decay}}^{234}}{\text{T}}{{\text{h}}_{90}}{ + _2}{\text{H}}{{\text{e}}^4}{/tex}
   1. Mass number of the nucleus produced= 238-4=234
   2. Atomic number of nucleus produced = 92-2=90

   so daughter nucleus can be expressed as ₉₀Th²³⁴ .

4. The binding energy per nucleon curve is shown as below
   
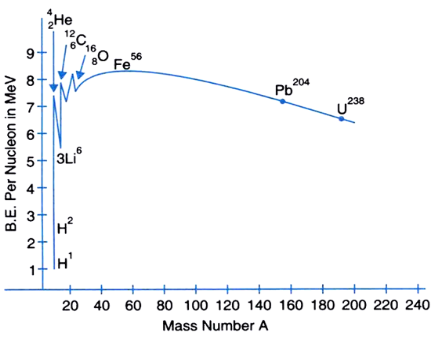
   1. Excluding the lighter nuclei, the average binding energy per nucleon is about 8 MeV.
   2. The maximum binding energy per nucleon occurs at around mass number A = 50, and corresponds to the most stable nuclei. Iron nucleus Fe⁵⁶ is located close to the peak with a binding energy per nucleon value of approximately 8.8 MeV. It’s one of the most stable nuclides that exist.
   3. Nuclei with very low or very high mass numbers have lesser binding energy per nucleon and are less stable because the lesser the binding energy per nucleon, the easier it is to separate the nucleus into its constituent nucleons.
   4. Nuclei with low mass numbers may undergo nuclear fusion, where light nuclei are joined together under certain conditions so that the final product may have a greater binding energy per nucleon.
5. Let the Parent nucleus was P whose binding enegy per nucleon was 7.6 Mev which decays into daughter nucleus Q having binding energy per nucleon is 8.5 Mev .According to question,
   {tex}P \to Q + Q{/tex}
   BE/A of element P = 7.6 MeV (given)
   So, {tex}\mathrm { BE } \text { of } P = 7.6 \times 240 \mathrm { MeV }{/tex}
   BE/A of element Q = 8.5 MeV
   So, BE of {tex}Q = 8.5 \times 120 \mathrm { MeV }{/tex}
   Now, energy released in the process will be difference of the Total binding energy and can be calculated as = 2(BE of Q) – BE of P
   {tex}= 85 \times 120 \times 2 – 7.6 \times 240{/tex}
   {tex}\Rightarrow \quad = ( 2040 – 1824 \mathrm { MeV }{/tex}
   = 216 MeV
6. The size of the nucleus is experimentally determined using Rutherford’s a-scattering experiment and the distance of closest approach and impact parameter and the findings can be stated as below –
   The relation between radius and mass number of the nucleus is R =R₀ A^1/3
   Where, R₀ = 1.2 fmR = radius of nucleus and A = mass number
   Nuclear density,
   {tex}\rho = \frac { \text { Mass of nucleus } } { \text { Volume of nucleus } } = \frac { m A } { \frac { 4 } { 3 } \pi \left( R _ { 0 } A ^ { 1 / 3 } \right) ^ { 3 } }{/tex}
   where, m = mass of each nucleon(proton and neutron)
   {tex}\rho = \frac { m A } { \frac { 4 } { 3 } \pi R _ { 0 } ^ { 3 } A } \Rightarrow \rho = \frac { m } { \frac { 4 } { 3 } \pi R _ { 0 } ^ { 3 } }{/tex}
   So as per above formula, density of nucleus does not depend on mass number of nucleus rather it is same for all the atoms and it is roughly in the order of 10¹⁷ kg/m³ which is very large as compared to our everyday observed densities.
7. As, {tex}R \approx {A^{1/3}}{/tex}
   {tex}\therefore \frac{{{R_1}}}{{{R_2}}} = {\left( {\frac{{{A_1}}}{{{A_2}}}} \right)^{1/3}}{/tex}
   {tex} = {\left( {\frac{{197}}{{107}}} \right)^{1/3}} = {(1.84)^{1/3}}{/tex}
   {tex} \Rightarrow {\log _{10}}\left( {\frac{{{R_1}}}{{{R_2}}}} \right) = {\log _{10}}{(1.84)^{1/3}}{/tex}
   {tex}\Rightarrow {\log _{10}}\left( {\frac{{{R_1}}}{{{R_2}}}} \right) = \frac{1}{3}{\log _{10}}(1.84){/tex}
   {tex} = \frac{1}{3} \times 0.2648{/tex}
   = 0.08827
   {tex}\Rightarrow \frac{{{R_1}}}{{{R_2}}}{/tex}= antilog (0.08827)
   = 1.23
8. potential energy of a pair of nucleons as a function of their separation is indicated in the diagram
   
   1. When distance is less than 0.8 fm, negative potential energy decreases to zero and then becomes positive which indicates high amount of repulsive forces.
   2. For distance greater than 0.8 fm, negative potential energy goes on decreasing and shows attractive interaction between the nuclides.
   1. Nuclear Fission is a process in nuclear physics in which the nucleus of an atom splits into two or more smaller nuclei as fission products, and usually some by-product particles. Hence, fission is a form of elemental transmutation
      e.g. {tex}_{92}^{235}U + _0^1n \to _{56}^{141}Ba + _{36}^{92}Kr + 3_0^1n + Q{/tex}
      Masses of reactant
      = 235.0439 amu + 1.0087 amu
      = 236.0526 amu
      Masses of product
      = 140.9139 + 91.8973 + 3.0261
      = 235.8373 amu
      Mass defect = 236.0526 – 235.8373
      = 0.2153 amu
      {tex}\because {/tex} 1 amu = 931 MeV
      {tex} \Rightarrow {/tex} Energy released= 0.2153 {tex} \times {/tex} 931 = 200MeV nearly
      Thus, energy is liberated in nuclear fission
      If {tex}_{92}^{235}U{/tex}.
   2. In nuclear physics, nuclear fusion is a reaction in which two or more atomic nuclei are combined to form one or more different atomic nuclei and subatomic particles (neutrons or protons). The difference in mass between the reactants and products is manifested as either the release or absorption of energy..
      e.g. {tex}_ { 1 } H ^ { 1 } + _ { 1 } H ^ { 1 } \longrightarrow _1H ^ { 2 } + e ^ { + } + v + 0.42 \mathrm { MeV }{/tex}
      Also,
      {tex}_ { 1 } H ^ { 2 } + _ { 1 } H ^ { 2 } \longrightarrow _ { 1 } H ^ { 3 } + _ { 1 } H ^ { 1 } + 4.03 M e V{/tex}
9. Mass of atom = 62.92960 u
   Mass of 29 electrons {tex} = 29 \times 0.000548 u{/tex}
   = 0.015892 u
   Mass of nucleus = (62.9296 – 0.015892)u
   = 62.913708 u
   Mass of 29 protons {tex} = 29 \times 1.007825\;u{/tex}
   = 29.226925 u
   Mass of (63 – 29) i.e. 34 neutrons
   {tex} = 34 \times 1.008665\;u{/tex}
   = 34.29461 u
   Total mass of protons and neutrons
   = (29.226925 + 34.29461) u
   = 63.521535 u
   Binding energy = {tex}\left( {63.521535 – 62.913708} \right) \times 931.5\;MeV{/tex}
   {tex} = 0.607827 \times 931.5\;MeV{/tex}
   Required energy {tex} = \frac{{6.023 \times {{10}^{23}}}}{{63}} \times 3 \times 0.607827 \times 931.5\;MeV{/tex}
   {tex} = 1.6 \times {10^{25}}MeV = 2.6 \times {10^{12}}J{/tex}
10. Nuclear fission is a process in nuclear physics in which the nucleus of an atom splits into two or more smaller nuclei as fission products, and usually some by-product particles. When these radioactive nuclides dissociate the total binding energy of product is less than that of reactants which is released energy in radioactive decay.
    {tex}\mathrm { U } _ { 92 } ^ { 235 } + n _ { 0 } ^ { 1 } \longrightarrow \mathrm { Ba } _ { 56 } ^ { 141 } + \mathrm { Kr } _ { 36 } ^ { 92 } + 3 n _ { 0 } ^ { 1 } + Q{/tex}
    In this case, the energy released per fission of {tex}U _ { 92 } ^ { 215 }{/tex} is 200.4 MeV.
    if light nuclei are forced together, they will fuse with a yield of energy because the mass of the combination will be less than the sum of the masses of the individual nuclei. If the combined nuclear mass is less than that of iron at the peak of the binding energy curve, then the nuclear particles will be more tightly bound than they were in the lighter nuclei, and that decrease in mass comes off in the form of energy according to the Einstein relationship.
    e.g. {tex}_1^1H + _1^1H \to _1^2He + {e^ + } + v + 042MeV{/tex}
    {tex}_1^2H + _1^2H \to _1^3He + n + 3.27MeV{/tex}
    {tex}_ { 1 } ^ { 2 } H + _ { 1 } ^ { 2 } H \longrightarrow _ { 1 } H ^ { 3 } + _ { 1 } ^ { 1 } H + 4.03 M e ^ { V }{/tex}
    According to the question,
    {tex}_ { 1 } ^ { 2 } \mathrm { H } + \mathrm { H } \longrightarrow _ { 2 } ^ { 4 } \mathrm { He } + n \mathrm { MeV }{/tex}
    {tex}\Delta m = ( 2014102 + 3.016049 ){/tex}{tex}- ( 4.002603 + 1.008665 ){/tex}
    = 0.018883.u
    Energy released, {tex}Q = 0.018883 \times 9315 \frac { \mathrm { MeV } } { c ^ { 2 } }{/tex}
    = 17.589 MeV

Chapter Wise Extra Questions of Class 12 Physics Part I & Part II

1. Electric Charges and Fields
2. Electrostatic Potential and Capacitance
3. Current Electricity
4. Moving Charges and Magnetism
5. Magnetism and Matter
6. Electromagnetic Induction
7. Alternating Current
8. Electromagnetic Waves
9. Ray Optics and Optical
10. Wave Optics
11. Dual Nature of Radiation and Matter
12. Atoms
13. Nuclei
14. Electronic Devices