NCERT Solutions for Class 9 Maths Exercise 10.5 book solutions are available in PDF format for free download. These ncert book chapter wise questions and answers are very helpful for CBSE board exam. CBSE recommends NCERT books and most of the questions in CBSE exam are asked from NCERT text books. Class 9 Maths chapter wise NCERT solution for Maths Book for all the chapters can be downloaded from our website and myCBSEguide mobile app for free.
NCERT solutions for Class 9 Maths Circles Download as PDF

NCERT Solutions for Class 9 Mathematics Circles
1. In figure, A, B, C are three points on a circle with centre O such that  BOC =
BOC = 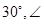 AOB =
AOB = 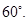 If D is a point on the circle other than the arc ABC, find
If D is a point on the circle other than the arc ABC, find  ADC.
ADC.
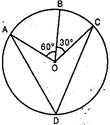
Ans.  AOC =
AOC =  AOB +
AOB +  BOC
BOC

 AOC =
AOC = 
Now  AOC = 2
AOC = 2 ADC
ADC
 Angled subtended by an arc, at the centre of the circle is double the angle subtended by the same arc at any point in the remaining part of the circle]
Angled subtended by an arc, at the centre of the circle is double the angle subtended by the same arc at any point in the remaining part of the circle]

 ADC =
ADC = 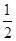
 AOC
AOC

 ADC =
ADC = 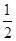
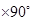 =
= 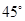
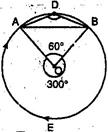
2. A chord of a circle is equal to the radius of the circle. Find the angle subtended by the chord on a point on the minor arc and also at a point on the major arc.
Ans. Let AB be the minor arc of circle.
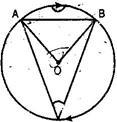
 Chord AB = Radius OA = Radius OB
Chord AB = Radius OA = Radius OB
 AOB is an equilateral triangle.
AOB is an equilateral triangle.

 AOB =
AOB = 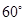
Now 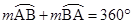

 AOB +
AOB +  BOA =
BOA = 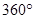

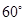 +
+  BOA =
BOA = 

 BOA =
BOA = 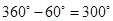
D is a point in the minor arc.
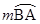 = 2
= 2 BDA
BDA

 BOA = 2
BOA = 2 BDA
BDA

 BDA =
BDA = 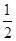
 BOA =
BOA = 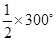

 BDA =
BDA = 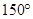
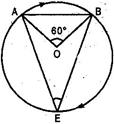
Thus angle subtended by major arc,  at any point D in the minor arc is
at any point D in the minor arc is 
Let E be a point in the major arc 
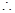
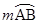 = 2
= 2 AEB
AEB

 AOB = 2
AOB = 2 AEB
AEB

 AEB =
AEB = 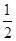
 AOB
AOB

 AEB =
AEB = 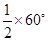 =
= 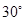
3. In figure,  PQR =
PQR =  where P, Q, R are points on a circle with centre O. Find
where P, Q, R are points on a circle with centre O. Find  OPR.
OPR.
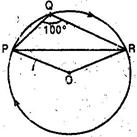
Ans. In the figure, Q is a point in the minor arc 
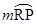 = 2
= 2 PQR
PQR

 ROP = 2
ROP = 2 PQR
PQR

 ROP =
ROP = 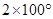 =
= 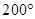
Now 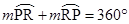

 POR +
POR +  ROP =
ROP = 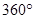

 POR +
POR + 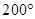 =
= 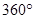

 POR =
POR = 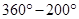 =
=  …..(i)
…..(i)
Now 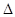 OPR is an isosceles triangle.
OPR is an isosceles triangle.
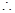 OP = OR [radii of the circle]
OP = OR [radii of the circle]

 OPR =
OPR =  ORP [angles opposite to equal sides are equal] …..(ii)
ORP [angles opposite to equal sides are equal] …..(ii)
Now in isosceles triangle OPR,
 OPR +
OPR +  ORP +
ORP +  POR =
POR = 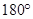

 OPR +
OPR +  ORP +
ORP +  =
= 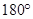
 2
2 OPR =
OPR =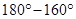 [Using (i) & (ii)]
[Using (i) & (ii)]
 2
2 OPR =
OPR = 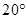

 OPR =
OPR = 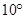
4. In figure,  ABC =
ABC = 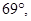
 ACB =
ACB =  find
find  BDC.
BDC.
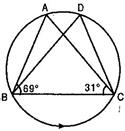
Ans. In triangle ABC,
 BAC +
BAC +  ABC +
ABC +  ACB =
ACB = 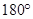

 BAC +
BAC + 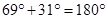

 BAC =
BAC = 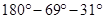

 BAC =
BAC =  …….(i)
…….(i)
Since, A and D are the points in the same segment of the circle.
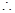
 BDC =
BDC =  BAC
BAC

 BDC =
BDC =  [Using (i)]
[Using (i)]
NCERT Solutions for Class 9 Maths Exercise 10.5
5. In figure, A, B, C, D are four points on a circle. AC and BD intersect at a point E such that  BEC =
BEC = 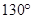 and
and
 ECD =
ECD =  Find
Find  BAC.
BAC.
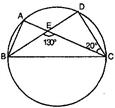
Ans. Given:  BEC =
BEC = 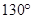 and
and  ECD =
ECD = 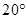
 DEC =
DEC =  BEC =
BEC = 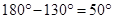 [Linear pair]
[Linear pair]
Now in  DEC,
DEC,
 DEC +
DEC +  DCE +
DCE +  EDC =
EDC = 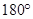 [Angle sum property]
[Angle sum property]

 EDC =
EDC = 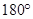

 EDC =
EDC = 

 BAC =
BAC =  EDC =
EDC =  [Angles in same segment]
[Angles in same segment]
NCERT Solutions for Class 9 Maths Exercise 10.5
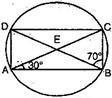
6. ABCD is a cyclic quadrilateral whose diagonals intersect at a point E.  DBC =
DBC = 
 BAC is
BAC is 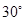 find
find BCD. Further if AB = BC, find
BCD. Further if AB = BC, find  ECD.
ECD.
Ans. For chord CD
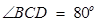
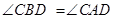 (Angles in same segment)
(Angles in same segment)
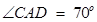
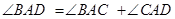
= 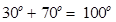
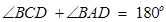 (Opposite angles of a cyclic quadrilateral)
(Opposite angles of a cyclic quadrilateral)
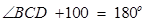
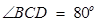
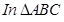
AB = BC (given)
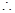
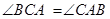 (Angles opposite to equal sides of a triangle)
(Angles opposite to equal sides of a triangle)
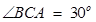
We have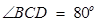
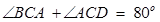
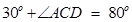
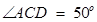

NCERT Solutions for Class 9 Maths Exercise 10.5
7. If diagonals of a cyclic quadrilateral are diameters of the circle through the vertices of the quadrilateral, prove that it is a rectangle.
Ans. Let ABCD a cyclic quadrilateral having diagonals as BD and AC intersecting each other at point O.

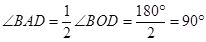
(Consider BD as a chord)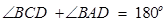 (Cyclic quadrilateral)
(Cyclic quadrilateral)
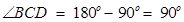
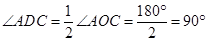
(Considering AC as a chord)
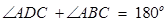 (Cyclic quadrilateral)
(Cyclic quadrilateral)
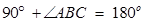
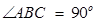
Here, each interior angle of cyclic quadrilateral is of . Hence it is a rectangle.
. Hence it is a rectangle.
NCERT Solutions for Class 9 Maths Exercise 10.5
8. If the non-parallel sides of a trapezium are equal, prove that it is cyclic.
Ans. Given: A trapezium ABCD in which AB CD and AD = BC.
CD and AD = BC.
To prove: The points A, B, C, D are concyclic.
Construction: Draw DE CB.
CB.
Proof: Since DE CB and EB
CB and EB DC.
DC.

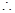 EBCD is a parallelogram.
EBCD is a parallelogram.
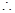 DE = CB and
DE = CB and  DEB =
DEB =  DCB
DCB
Now AD = BC and DA = DE

 DAE =
DAE =  DEB
DEB
But  DEA +
DEA +  DEB =
DEB = 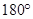

 DAE +
DAE +  DCB =
DCB = 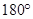

 DEA =
DEA =  DAE and
DAE and  DEB =
DEB =  DCB]
DCB]

 DAB +
DAB +  DCB =
DCB = 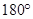

 A +
A +  C =
C = 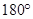
Hence, ABCD is a cyclic trapezium.
NCERT Solutions for Class 9 Maths Exercise 10.5
9. Two circles intersect at two points B and C. Through B, two line segments ABD and PBQ are drawn to intersect the circles at A, D, P, Q respectively (see figure). Prove that  ACP =
ACP =  QCD.
QCD.
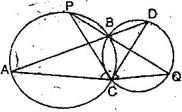
Ans. In triangles ACD and QCP,
 A =
A =  P and
P and  Q =
Q =  D [Angles in same segment]
D [Angles in same segment]
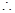
 ACD =
ACD =  QCP [Third angles] ……….(i)
QCP [Third angles] ……….(i)
Subtracting  PCD from both the sides of eq. (i), we get,
PCD from both the sides of eq. (i), we get,
 ACD –
ACD –  PCD =
PCD =  QCP –
QCP –  PCD
PCD

 ACPO =
ACPO =  QCD
QCD
Hence proved.
NCERT Solutions for Class 9 Maths Exercise 10.5
10. If circles are drawn taking two sides of a triangle as diameters, prove that the point of intersection of these circles lie on the third side.
Ans. Given: Two circles intersect each other at points A and B. AP and AQ be their respective diameters.
To prove: Point B lies on the third side PQ.
Construction: Join A and B.
Proof: AP is a diameter.
 1 =
1 = 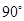
AlsoAQ is a diameter.
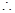
 2 =
2 = 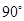
 1 +
1 +  2 =
2 = 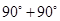

 PBQ =
PBQ = 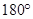
 PBQ is a line.
PBQ is a line.
Thus point B. i.e. point of intersection of these circles lies on the third side i.e., on PQ.
NCERT Solutions for Class 9 Maths Exercise 10.5
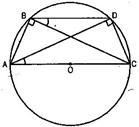
11. ABC and ADC are two right triangles with common hypotenuse AC. Prove that  CAD =
CAD =  ABD.
ABD.
Ans. We have ABC and ADC two right triangles, right angled at B and D respectively.

 ABC = ADC [Each
ABC = ADC [Each 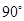 ]
]
If we draw a circle with AC (the common hypotenuse) as diameter, this circle will definitely passes through of an arc AC, Because B and D are the points in the alternate segment of an arc AC.
Now we have 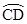 subtending
subtending  CBD and
CBD and  CAD in the same segment.
CAD in the same segment.
 CAD =
CAD =  CBD
CBD
Hence proved.
NCERT Solutions for Class 9 Maths Exercise 10.5
NCERT Solutions for Class 9 Maths PDF (Download) Free from myCBSEguide app and myCBSEguide website. Ncert solution class 9 Maths includes text book solutions from Mathematics Book. NCERT Solutions for CBSE Class 9 Maths have total 15 chapters. 9 Maths NCERT Solutions in PDF for free Download on our website. Ncert Maths class 9 solutions PDF and Maths ncert class 9 PDF solutions with latest modifications and as per the latest CBSE syllabus are only available in myCBSEguide.
CBSE app for Class 9
To download NCERT Solutions for Class 9 Maths, Computer Science, Home Science,Hindi ,English, Social Science do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT solutions, NCERT Exemplar solutions, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.