NCERT Solutions for Class 9 Maths Exercise 2.4

NCERT Solutions for Class 9 Maths Exercise 2.4 book solutions are available in PDF format for free download. These ncert book chapter wise questions and answers are very helpful for CBSE board exam. CBSE recommends NCERT books and most of the questions in CBSE exam are asked from NCERT text books. Class 9 Maths chapter wise NCERT solution for Maths Book for all the chapters can be downloaded from our website and myCBSEguide mobile app for free.

NCERT solutions for Class 9 Maths Polynomials Download as PDF

 NCERT Solutions for Class 9 Maths Polynomials

1. Determine which of the following polynomials has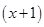a factor:

(i)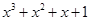

(ii)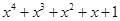

(iii)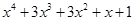

(iv)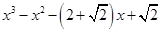

Ans. (i)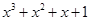

While applying the factor theorem, we get

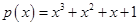
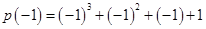
=-1+1-1+1

=0

We conclude that on dividing the polynomial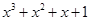by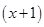, we get the remainder as0.

Therefore, we conclude that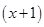is a factor of.

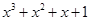(ii)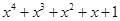

While applying the factor theorem, we get

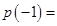
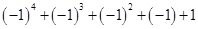
=1-1+1-1+1

=1

We conclude that on dividing the polynomial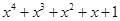by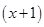, we will get the remainder as1, which is not 0.

Therefore, we conclude that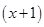is not a factor of.

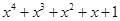(iii)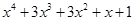

While applying the factor theorem, we get

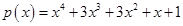
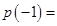
=1-3+3-1+1

=1

We conclude that on dividing the polynomial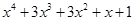by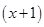, we will get the remainder as 1, which is not 0.

Therefore, we conclude that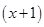is not a factor of.

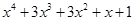(iv)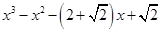

While applying the factor theorem, we get

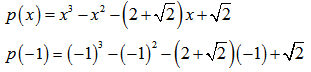
We conclude that on dividing the polynomial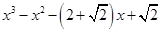by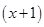, we will get the remainder as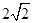, which is not 0.

Therefore, we conclude that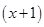is not a factor of.

---

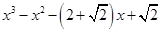NCERT Solutions for Class 9 Maths Exercise 2.4

2. Use the Factor Theorem to determine whether g(x) is a factor of p(x) in each of the

following cases:

(i)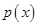

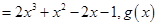
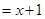
(ii)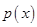

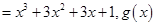
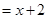
(iii)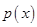

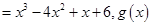
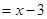
Ans. (i)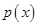

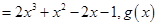
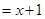
We know that according to the factor theorem,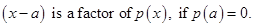

We can conclude that g(x) is a factor of p(x), if p(-1)=0.

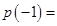
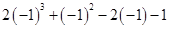
=2+1-1-2

=0

Therefore, we conclude that the g(x) is a factor of p(x).

(ii)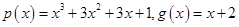

We know that according to the factor theorem,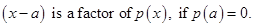

We can conclude that g(x) is a factor of p(x), if p(-2)=0.

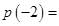
=-8+12-6+1

=-1

Therefore, we conclude that the g(x) is not a factor of p(x).

(iii)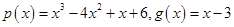

We know that according to the factor theorem,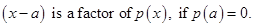

We can conclude that g(x) is a factor of p(x), if p(3)=0.

=27-36+3+6

=0

Therefore, we conclude that the g(x) is a factor of p(x).

---

NCERT Solutions for Class 9 Maths Exercise 2.4

3. Find the value of k, if x – 1 is a factor of p(x) in each of the following cases:

(i)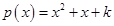

(ii)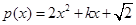

(iii)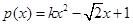

(iv)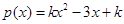

Ans. (i)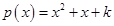

We know that according to the factor theorem

.

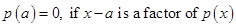We conclude that if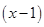is a factor of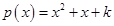, then.

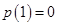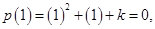or

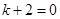
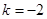
Therefore, we can conclude that the value of k is.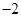

(ii)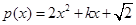

We know that according to the factor theorem

.

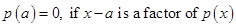We conclude that if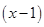is a factor of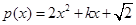, then.

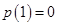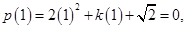or

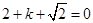
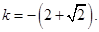
Therefore, we can conclude that the value of k is.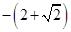

(iii)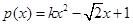

We know that according to the factor theorem

.

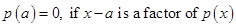We conclude that if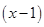is a factor of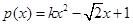, then.

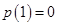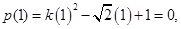or

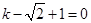
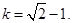
Therefore, we can conclude that the value of k is.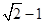

(iv)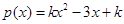

We know that according to the factor theorem

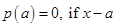is a factor of p(x)

We conclude that if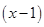is a factor of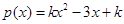, then.

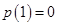or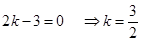

Therefore, we can conclude that the value of k is.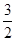

---

NCERT Solutions for Class 9 Maths Exercise 2.4

4. Factorize:

(i)

(ii)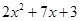

(iii)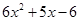

(iv)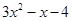

Ans. (i)

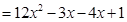
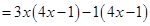
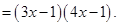
Therefore, we conclude that on factorizing the polynomial, we get.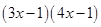

(ii)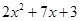

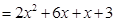
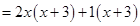
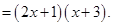
Therefore, we conclude that on factorizing the polynomial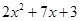, we get.

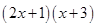(iii)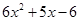

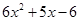
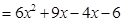
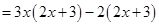
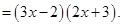
Therefore, we conclude that on factorizing the polynomial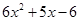, we get.

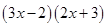(iv)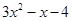

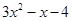
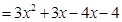
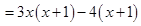
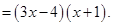
Therefore, we conclude that on factorizing the polynomial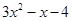, we get.

---

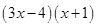NCERT Solutions for Class 9 Maths Exercise 2.4

5. Factorize:

(i)

(ii)

(iii)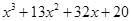

(iv)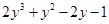

Ans. (i)

We need to consider the factors of 2, which are.

Let us substitute 1 in the polynomial, to get

=1-1-2+2=0

Thus, according to factor theorem, we can conclude that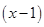is a factor of the polynomial.

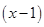Let us divide the polynomialby, to get

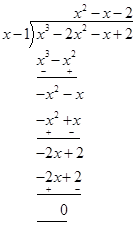
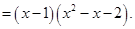
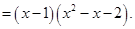
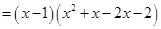
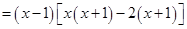
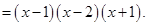
Therefore, we can conclude that on factorizing the polynomial, we get .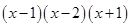

(ii)

We need to consider the factors of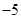, which are.

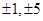Let us substitute 1 in the polynomial, to get

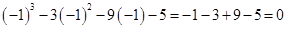
Thus, according to factor theorem, we can conclude that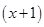is a factor of the polynomial.

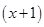Let us divide the polynomialby, to get

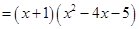
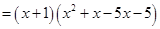
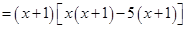
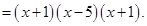
Therefore, we can conclude that on factorizing the polynomial, we get .

(iii)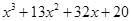

We need to consider the factors of 20, which are.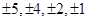

Let us substitute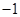in the polynomial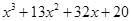, to get

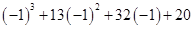=-1+13-32+20=-20+20=0

Thus, according to factor theorem, we can conclude that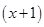is a factor of the polynomial.

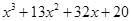Let us divide the polynomial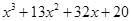by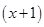, to get

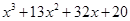
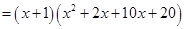
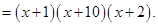
Therefore, we can conclude that on factorizing the polynomial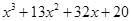, we get.

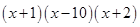(iv)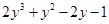

We need to consider the factors of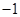, which are.

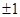Let us substitute 1 in the polynomial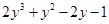, to get

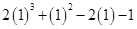=2+1-2-1=3-3=0

Thus, according to factor theorem, we can conclude that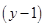is a factor of the polynomial.

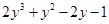Let us divide the polynomial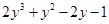by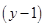, to get

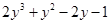
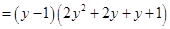
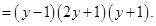
Therefore, we can conclude that on factorizing the polynomial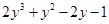, we get.

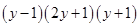NCERT Solutions for Class 9 Maths Exercise 2.4

NCERT Solutions Class 9 Maths PDF (Download) Free from myCBSEguide app and myCBSEguide website. Ncert solution class 9 Maths includes text book solutions from Mathematics Book. NCERT Solutions for CBSE Class 9 Maths have total 15 chapters. 9 Maths NCERT Solutions in PDF for free Download on our website. Ncert Maths class 9 solutions PDF and Maths ncert class 9 PDF solutions with latest modifications and as per the latest CBSE syllabus are only available in myCBSEguide.

CBSE app for Class 9

To download NCERT Solutions for Class 9 Maths, Computer Science, Home Science,Hindi ,English, Social Science do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT solutions, NCERT Exemplar solutions, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.