myCBSEguide App
Download the app to get CBSE Sample Papers 2023-24, NCERT Solutions (Revised), Most Important Questions, Previous Year Question Bank, Mock Tests, and Detailed Notes.
Install NowExercise 13.1
Evaluate the following limits in Exercises 1 to 22.
1. 
Ans. 
3 + 3 = 6
2. 
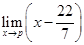
Ans.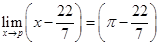 
3. 
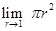
Ans.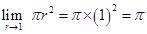 
4. 
Ans. 
5. 
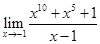
Ans.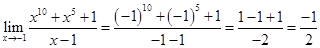 
6. 
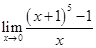
Ans.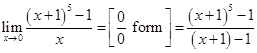 
Putting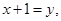 
as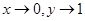 
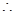
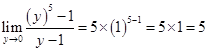
7. 
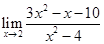
Ans.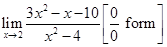 
= 
= 
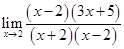
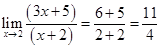
8. 
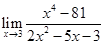
Ans.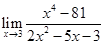
=
= 
= 
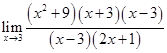
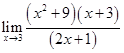
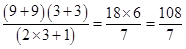
9. 
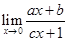
Ans.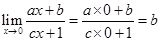 
10. 
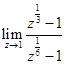
Ans. 
= 
= 
= 
= 
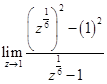
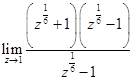
= 1 + 1 = 2
11. 
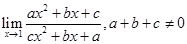
Ans.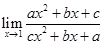 
= 
= 
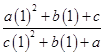
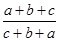= 1
12. 
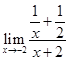
Ans. =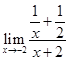 
= 
= 
= 
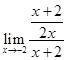
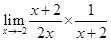
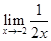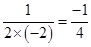
13. 
Ans.
= 
= 
= 
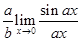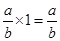
14. 
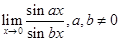
Ans. 
= 
= 
= 
= 
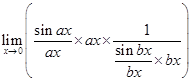
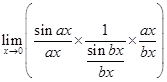
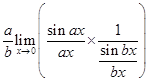
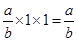
15. 
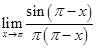
Ans.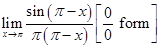 
Putting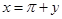 
as 
= 
= 
= 
= 
= 
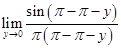
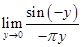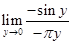
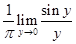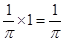
16. 
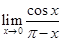
Ans. =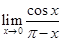 
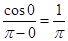
17. 
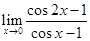
Ans. =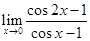 
= 
= 
= 
= 
= 
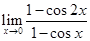
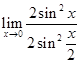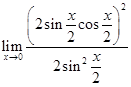
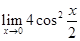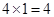
18. 
Ans.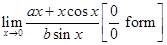 
= 
= 
= 
= 
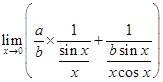
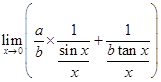
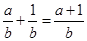
19. 
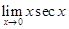
Ans. =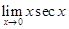 
= 
= 
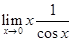
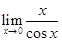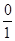= 0
20. 
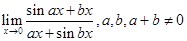
Ans.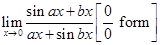 
Dividing numerator and denominator by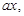 
= 
= 
= 
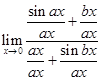
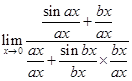= 1
21. 
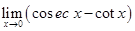
Ans. Given: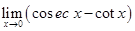
= 
= 
= 
= 
= 
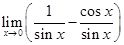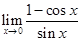
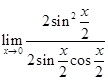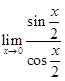
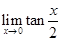= 0
22. 
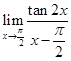
Ans. Given: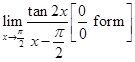
Putting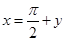 
as 
= 
= 
= 
= 
= 
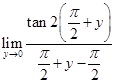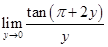
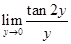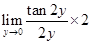
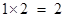
23. Fi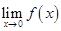nd 
and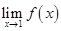 
where 
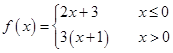
Ans. Given: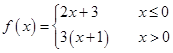
Now
= 
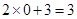
And
= 3 (1 + 1) =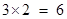 
24. Find 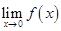where 
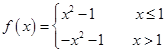
Ans. Given: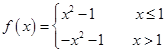
L.H.L.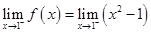
Putting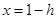 
as 
= 
= 
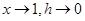
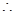
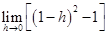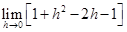
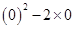= 0
R.H.L.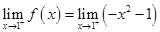
Putting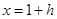 
as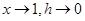
= 
= 
= 
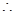
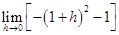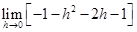
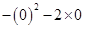25. Evaluate 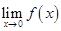where 
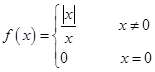
Ans. Given: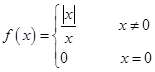
L.H.L.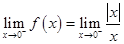
Putting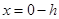 
as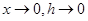 
= 
= 
= 
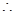
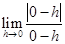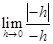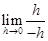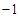
R.H.L.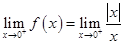
Putting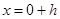 
as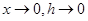
= 
= 
= 
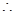
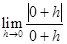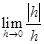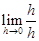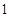
Here, L.H.L.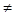 
R.H.L.
Therefore, this limit does not exist at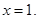 
26. Find 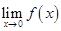where 
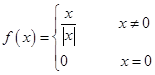
Ans. Given: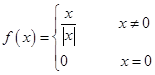
L.H.L.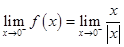
Putting 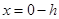as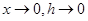
= 
= 
=
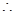
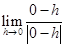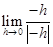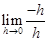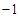
R.H.L.
Putting 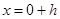as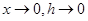
= 
= =
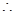
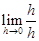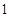
Here, L.H.L. 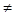R.H.L.
Therefore, this limit does not exist at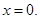 
27. Find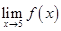 
where 
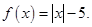
Ans. Given: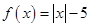
L.H.L.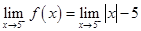
Putting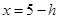 
as 
= 
= 
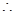
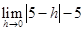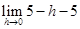
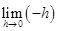= 0
R.H.L.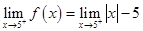
Putting 
as
= 
= 
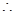
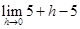
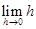= 0
Here, L.H.L. = R.H.L.
Therefore, this limit exists at and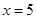 
28. Suppose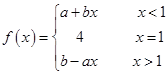 
and if 
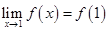what are possible values of 
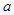and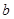 
?
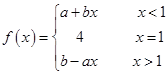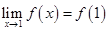
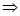
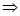
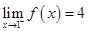Ans. Given: and
and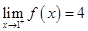
……… (i)
Now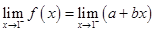
Putting 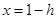as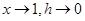
= 
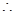
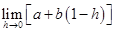
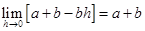……….(ii)
Again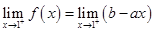
Putting 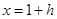as
= 
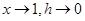
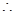
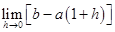
……….(ii)
Putting values from eq. (ii) and (iii) in eq. (i), we get
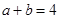and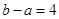 
On solving these equation, we get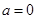
and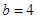 
29. Let 
be fixed real numbers and define a function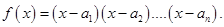 
What is 
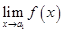? For some 
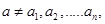compute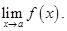 
Ans. Given: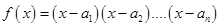
Now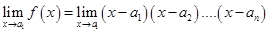
= 
= 
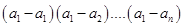
= 0
Also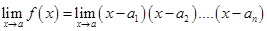
= 
30. If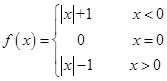 
for what values of 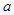does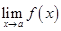 
exists?
Ans. Given: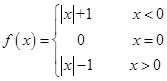 
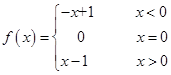
exists for all 
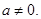
Now L.H.L.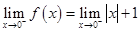
Putting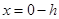 as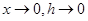
= 
= 
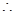
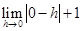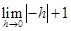
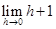= 0 + 1 = 1
Also R.H.L.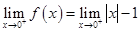
Putting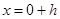 as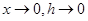
= 
= 
= 
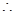
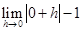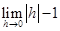
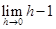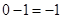
Here, L.H.L. 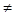R.H.L.
Therefore, this limit does not exist.
31. If the function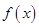 
satisfies 
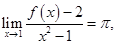valuate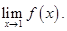 
Ans. Since
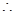
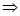
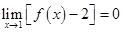
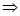
32. If 
for what integer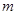 
and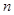 
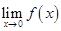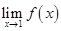does both and exist?
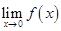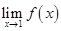Ans. Both and exist.
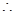
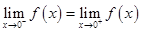and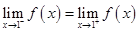
Now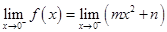
= 
= 
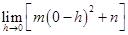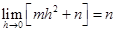
And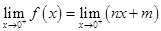
= 
= 
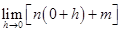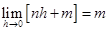
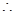
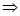
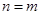……….(i)
Therefore, for 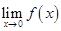exists we need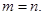 
Again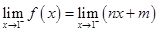
= 
= 
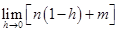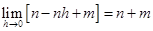
And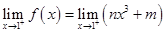
= 
= 
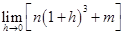
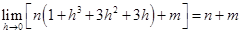
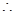
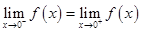
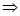
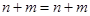
Therefore, 
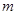exists for any integral value of and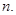 
Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create NowmyCBSEguide
Question Bank, Mock Tests, Exam Papers, NCERT Solutions, Sample Papers, Notes
Install Now
Nice???
it very good for teaching
very nice :aapka ye app hame bahut achha laga
mai ese bahut use karta hu
I like your appp and this app helps me very much???
Thank you…….. This app help me very much