
myCBSEguide App
Download the app to get CBSE Sample Papers 2025-26, NCERT Solutions (Revised), Most Important Questions, Previous Year Question Bank, Mock Tests, and Detailed Notes.
Install NowExercise 10.3
1. Reduce the following equations into slope-intercept form and find their slopes and the 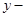 intercepts.
intercepts.
(i) 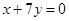
(ii) 
(iii) 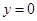
Ans. (i) Given: 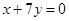



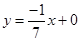 ……….(i)
……….(i)
Comparing with 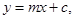 we have
we have 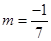 and
and 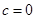
(ii) Given: 



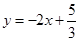 ……….(i)
……….(i)
Comparing with 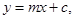 we have
we have  and
and 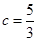
(iii) Given: 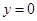

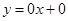 ……….(i)
……….(i)
Comparing with 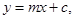 we have
we have 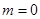 and
and 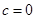
2. Reduce the following equations into intercept form and find their intercepts on the axis:
(i) 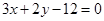
(ii) 
(iii) 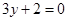
Ans. (i) Given: 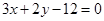



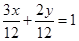

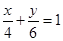
Comparing with 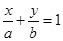 , we have
, we have 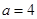 and
and 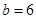
(ii) Given: 

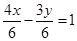

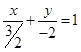
Comparing with 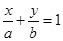 , we have
, we have 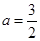 and
and 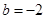
(iii) Given: 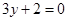

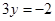

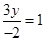



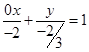
Comparing with 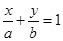 , we have
, we have  and
and 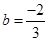
3. Reduce the following equations into normal form. Find their perpendicular distances from the origin and angle between perpendicular 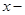 axis:
axis:
(i) 
(ii) 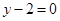
(iii) 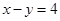
Ans. (i) Given: 

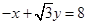
Dividing both sides by 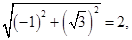 we have
we have
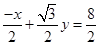

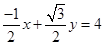
Putting  and
and 

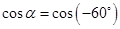

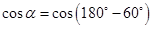

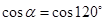

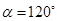 =
= 
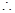 Equation of line in normal form is
Equation of line in normal form is 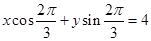
Comparing with  we have
we have 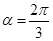 and
and 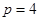
(ii) Given: 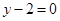

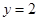


Dividing both sides by 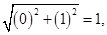 we have
we have 
Putting  and
and 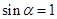


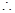 Equation of line in normal form is
Equation of line in normal form is 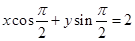
Comparing with  we have
we have  and
and 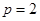
(iii) Given: 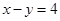
Dividing both sides by 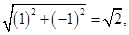 we have
we have
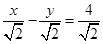

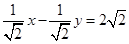
Putting 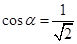 and
and 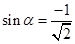



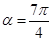
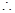 Equation of line in normal form is
Equation of line in normal form is 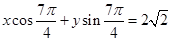
Comparing with  we have
we have 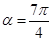 and
and 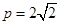
4. Find the distance of the point 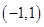 from the line
from the line 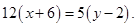
Ans. Given: A line 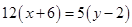

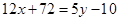

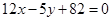
Now, perpendicular distance of the point 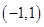 from the line
from the line 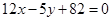 is
is
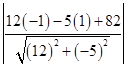 =
= 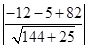 =
= 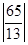 = 5 units
= 5 units
5. Find the points on the 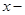 axis, where distances from the line
axis, where distances from the line 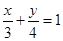 are 4 units.
are 4 units.
Ans. Let the coordinates of the point on 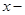 axis be
axis be 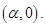
Now, perpendicular distance of the point 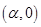 from the line
from the line 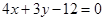 is
is
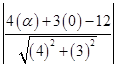 =
= 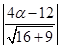 =
= 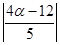
According to question, 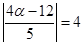

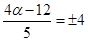

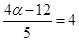 or
or 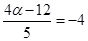

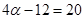 or
or 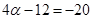

 or
or 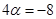

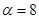 or
or 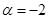
Therefore, the points on 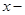 axis are (8, 0) and
axis are (8, 0) and 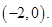
6. Find the distance between parallel lines:
(i)  and
and 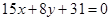
(ii) 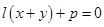 and
and 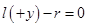
Ans. (i) Given: Two equations  and
and 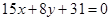
Here, 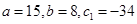 and
and 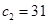
Distance between two parallel lines 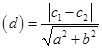 =
= 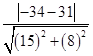
= 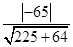 =
= 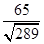 =
= 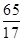 units
units
(ii) Given: Two equations 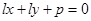 and
and 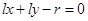
Here, 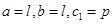 and
and 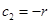
Distance between two parallel lines 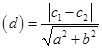 =
= 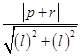
= 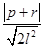 =
= 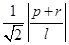 units
units
7. Find equation of the line parallel to the line  and passing through the point
and passing through the point 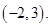
Ans. Given: Equation of a line which is parallel to the line  is
is 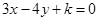 .
.
Since the line passes through point  .
.
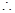
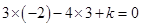

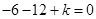

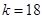
Therefore, the equation of required line is 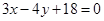 .
.
8. Find the equation of the line perpendicular to the line 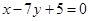 and having
and having 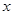 intercept 3.
intercept 3.
Ans. Given: Equation of a line which is perpendicular to the line 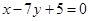 is
is 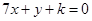 .
.
Since the line passes through point 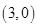 .
.
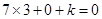

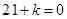

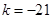
Therefore, the equation of required line is 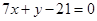 .
.
9. Find the angles between the lines 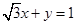 and
and 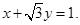
Ans. Given: 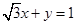


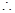

Also 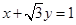


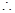

Let 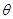 be the angle between the lines.
be the angle between the lines.
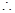
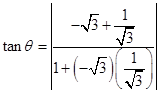
= 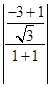 =
= 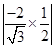 =
= 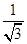

 and
and 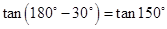

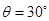 and
and 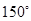
10. The line through the points 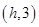 and (4, 1) intersects the line
and (4, 1) intersects the line 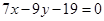 at right angle. Find the value of
at right angle. Find the value of 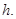
Ans. Slope of the line passing through the points 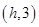 and (4, 1) =
and (4, 1) = 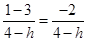
Also slope of the line 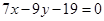 is
is 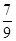
Since both lines are perpendicular to each other.
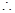
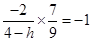

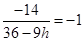

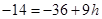

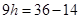

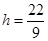
11. Prove that the line through the point 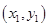 and parallel to the line
and parallel to the line 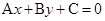 is
is  .
.
Ans. Equation of the line parallel to the line 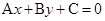 is
is 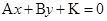 …..(i)
…..(i)
Since line (i) passes through 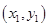 , therefore
, therefore  …..(ii)
…..(ii)
Subtracting eq. (ii) from eq. (i), we have 
12. Two lines passing through the point (2, 3) intersects each other at an angle of 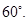 If slope of the line is 2, find equation of the other line.
If slope of the line is 2, find equation of the other line.
Ans. Given: 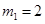 and
and 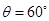
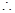
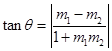



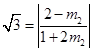

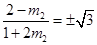
Taking 

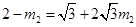

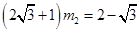

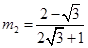
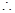 Equation of required line is
Equation of required line is 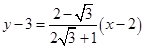

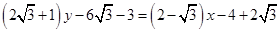

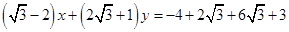

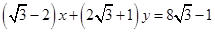
Taking 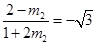

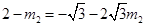

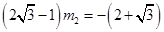

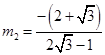
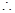 Equation of required line is
Equation of required line is 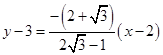

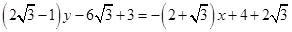

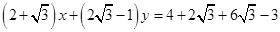

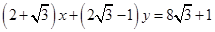
13. Find the equation of the right bisector of the line segment joining the points (3, 4) and 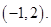
Ans. Mid-point of the line segment joining the points 93, 4) and 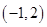 =
= 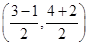 = (1, 3)
= (1, 3)
Slope of the line joining points (3, 4) and 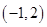 =
= 
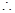 Slope of the required line is
Slope of the required line is 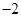
Therefore, the required line passes through point (1, 3) having slope 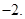
 Equation of the required line
Equation of the required line 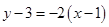

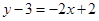

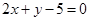
14. Find the coordinates of the foot of perpendicular from the point  on the line
on the line 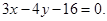
Ans. Let Q be the foot of perpendicular drawn from P on the line
on the line 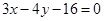

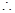 Equation of a line perpendicular to
Equation of a line perpendicular to 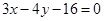 is
is 
Since the line passes through 
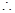
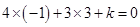

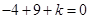

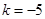
Therefore, Q is a point of intersection A
of the lines 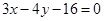 and
and 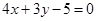
Solving both the equations, we have 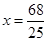 and
and 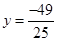
Therefore, coordinates of foot of perpendicular are 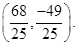
15. The perpendicular from the origin to the line 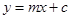 meets it at the point
meets it at the point 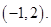 Find the value of
Find the value of  and
and 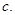
Ans. Equation of the line PQ

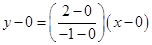

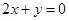
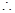 Slope of the required line which is perpendicular to this line is
Slope of the required line which is perpendicular to this line is 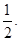
 Equation of the line AB is
Equation of the line AB is
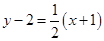

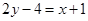



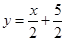
Comparing with 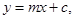 we have
we have 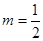 and
and 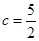
16. If 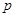 and
and  are the lengths of perpendiculars from the origin to the line
are the lengths of perpendiculars from the origin to the line  and
and 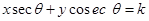 respectively, prove that
respectively, prove that 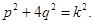
Ans. Length of perpendicular from origin to line 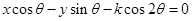 is
is
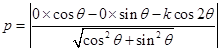
= 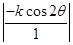 =
= 
And Length of perpendicular from origin to line 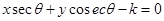 is
is
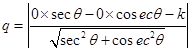
= 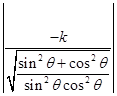
= 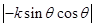 =
= 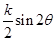
Now, 
= 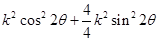
= 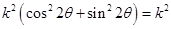
17. In the triangle ABC with vertices A (2, 3), B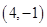 and C (1, 2), find the equation and length of altitude from the vertex A.
and C (1, 2), find the equation and length of altitude from the vertex A.
Ans. Slope of BC 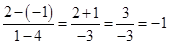
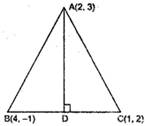
Since AD 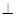 BC, therefore slope of AD = 1
BC, therefore slope of AD = 1
 Equation of AD is
Equation of AD is
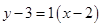

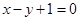
And Equation of BC is
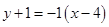

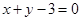
 Length of AD =
Length of AD = 
= 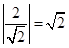 units
units
18. If 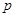 is the length of perpendicular from the origin to the line whose intercepts on the axes are
is the length of perpendicular from the origin to the line whose intercepts on the axes are 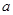 and
and 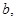 then show that
then show that 
Ans. Given: Line 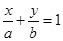

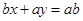

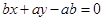
Now, 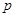 is the length of perpendicular from origin to
is the length of perpendicular from origin to 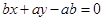 .
.
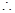
 =
= 

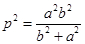

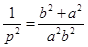




Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
Learn8 App
Practice unlimited questions for Entrance tests & government job exams at ₹99 only
Install Now