NCERT Solutions for Class 7 Maths Exercise 9.1

NCERT Solutions for Class 7 Maths Exercise 9.1 book solutions are available in PDF format for free download. These ncert book chapter wise questions and answers are very helpful for CBSE exam. CBSE recommends NCERT books and most of the questions in CBSE exam are asked from NCERT text books. Class 7 Maths chapter wise NCERT solution for Maths Book all the chapters can be downloaded from our website and myCBSEguide mobile app for free.

NCERT solutions for Maths Rational Numbers Download as PDF

NCERT Solutions for Class 7 Maths Rational Numbers

Class –VII Mathematics (Ex. 9.1)

Question 1.List five rational numbers between:

(i) {tex} – 1{/tex} and 0

(ii) {tex} – 2{/tex} and {tex} – 1{/tex}

(iii) {tex}\frac{{ – 4}}{5}{/tex} and {tex}\frac{{ – 2}}{3}{/tex}

(iv) {tex}\frac{{ – 1}}{2}{/tex} and {tex}\frac{2}{3}{/tex}

Answer:

(i) {tex} – 1{/tex} and 0

Let us write {tex} – 1{/tex} and 0 as rational numbers with denominator 6.

{tex} \Rightarrow {/tex} {tex} – 1 = \frac{{ – 6}}{6}{/tex} and 0 = {tex}\frac{0}{6}{/tex}

{tex}\therefore {/tex} {tex}\frac{{ – 6}}{6} < \frac{{ – 5}}{6} < \frac{{ – 4}}{6} < \frac{{ – 3}}{6} < \frac{{ – 2}}{6} < \frac{{ – 1}}{6} < 0{/tex}

{tex} \Rightarrow {/tex} {tex} – 1 < \frac{{ – 5}}{6} < \frac{{ – 2}}{3} < \frac{{ – 1}}{2} < \frac{{ – 1}}{3} < \frac{{ – 1}}{6} < 0{/tex}

Therefore, five rational numbers between {tex} – 1{/tex} and 0 would be

{tex}\frac{{ – 5}}{6},\frac{{ – 2}}{3},\frac{{ – 1}}{2},\frac{{ – 1}}{3},\frac{{ – 1}}{6}{/tex}

(ii) {tex} – 2{/tex} and {tex} – 1{/tex}

Let us write {tex} – 2{/tex} and {tex} – 1{/tex} as rational numbers with denominator 6.

{tex} \Rightarrow {/tex} {tex} – 2 = \frac{{ – 12}}{6}{/tex} and {tex} – 1 = \frac{{ – 6}}{6}{/tex}

{tex}\therefore {/tex} {tex}\frac{{ – 12}}{6} < \frac{{ – 11}}{6} < \frac{{ – 10}}{6} < \frac{{ – 9}}{6} < \frac{{ – 8}}{6} < \frac{{ – 7}}{6} < \frac{{ – 6}}{6}{/tex}

{tex} \Rightarrow {/tex} {tex} – 2 < \frac{{ – 11}}{6} < \frac{{ – 5}}{3} < \frac{{ – 3}}{2} < \frac{{ – 4}}{3} < \frac{{ – 7}}{6} < – 1{/tex}

Therefore, five rational numbers between {tex} – 2{/tex} and {tex} – 1{/tex} would be

{tex}\frac{{ – 11}}{6},\frac{{ – 5}}{3},\frac{{ – 3}}{2},\frac{{ – 4}}{3},\frac{{ – 7}}{6}{/tex}

(iii) {tex}\frac{{ – 4}}{5}{/tex} and {tex}\frac{{ – 2}}{3}{/tex}

Let us write {tex}\frac{{ – 4}}{5}{/tex} and {tex}\frac{{ – 2}}{3}{/tex} as rational numbers with the same denominators.

{tex} \Rightarrow {/tex} {tex}\frac{{ – 4}}{5} = \frac{{ – 36}}{{45}}{/tex} and {tex}\frac{{ – 2}}{3} = \frac{{ – 30}}{{45}}{/tex}

{tex}\therefore {/tex} {tex}\frac{{ – 36}}{{45}} < \frac{{ – 35}}{{45}} < \frac{{ – 34}}{{45}} < \frac{{ – 33}}{{45}} < \frac{{ – 32}}{{45}} < \frac{{ – 31}}{{45}} < \frac{{ – 30}}{{45}}{/tex}

{tex} \Rightarrow {/tex} {tex}\frac{{ – 4}}{5} < \frac{{ – 7}}{9} < \frac{{ – 34}}{{45}} < \frac{{ – 11}}{{15}} < \frac{{ – 32}}{{45}} < \frac{{ – 31}}{{45}} < \frac{{ – 2}}{3}{/tex}

Therefore, five rational numbers between {tex}\frac{{ – 4}}{5}{/tex} and {tex}\frac{{ – 2}}{3}{/tex} would be

{tex}\frac{{ – 7}}{9},\frac{{ – 34}}{{45}},\frac{{ – 11}}{{15}},\frac{{ – 32}}{{45}},\frac{{ – 31}}{{45}},\frac{{ – 2}}{3}{/tex}

(iv) {tex}\frac{{ – 1}}{2}{/tex} and {tex}\frac{2}{3}{/tex}

Let us write {tex}\frac{{ – 1}}{2}{/tex} and {tex}\frac{2}{3}{/tex} as rational numbers with the same denominators.

{tex} \Rightarrow {/tex} {tex}\frac{{ – 1}}{2} = \frac{{ – 3}}{6}{/tex} and {tex}\frac{2}{3} = \frac{4}{6}{/tex}

{tex}\therefore {/tex} {tex}\frac{{ – 3}}{6} < \frac{{ – 2}}{6} < \frac{{ – 1}}{6} < 0 < \frac{1}{6} < \frac{2}{6} < \frac{3}{6} < \frac{4}{6}{/tex}

{tex} \Rightarrow {/tex} {tex}\frac{{ – 1}}{2} < \frac{{ – 1}}{3} < \frac{{ – 1}}{6} < 0 < \frac{1}{6} < \frac{1}{3} < \frac{1}{2} < \frac{2}{3}{/tex}

Therefore, five rational numbers between {tex}\frac{{ – 1}}{2}{/tex} and {tex}\frac{2}{3}{/tex} would be {tex}\frac{{ – 1}}{3},\frac{{ – 1}}{6},0,\frac{1}{6},\frac{1}{3}{/tex}.

---

NCERT Solutions for Class 7 Maths Exercise 9.1

Question 2.Write four more rational numbers in each of the following patterns:

(i) {tex}\frac{{ – 3}}{5},\frac{{ – 6}}{{10}},\frac{{ – 9}}{{15}},\frac{{ – 12}}{{20}},………{/tex}

(ii) {tex}\frac{{ – 1}}{4},\frac{{ – 2}}{8},\frac{{ – 3}}{{12}},……….{/tex}

(iii) {tex}\frac{{ – 1}}{6},\frac{2}{{ – 12}},\frac{3}{{ – 18}},\frac{4}{{ – 24}},………{/tex}

(iv) {tex}\frac{{ – 2}}{3},\frac{2}{{ – 3}},\frac{4}{{ – 6}},\frac{6}{{ – 9}},……….{/tex}

Answer:

(i) {tex}\frac{{ – 3}}{5},\frac{{ – 6}}{{10}},\frac{{ – 9}}{{15}},\frac{{ – 12}}{{20}},………{/tex}

{tex} \Rightarrow {/tex} {tex}\frac{{ – 3 \times 1}}{{5 \times 1}},\frac{{ – 3 \times 2}}{{5 \times 2}},\frac{{ – 3 \times 3}}{{5 \times 3}},\frac{{ – 3 \times 4}}{{5 \times 4}},………{/tex}

Therefore, the next four rational numbers of this pattern would be

{tex}\frac{{ – 3 \times 5}}{{5 \times 5}},\frac{{ – 3 \times 6}}{{5 \times 6}},\frac{{ – 3 \times 7}}{{5 \times 7}},\frac{{ – 3 \times 8}}{{5 \times 8}}{/tex} = {tex}\frac{{ – 15}}{{25}},\frac{{ – 18}}{{30}},\frac{{ – 21}}{{35}},\frac{{ – 24}}{{40}}{/tex}

(ii) {tex}\frac{{ – 1}}{4},\frac{{ – 2}}{8},\frac{{ – 3}}{{12}},……….{/tex}

{tex} \Rightarrow {/tex} {tex}\frac{{ – 1 \times 1}}{{4 \times 1}},\frac{{ – 1 \times 2}}{{4 \times 2}},\frac{{ – 1 \times 3}}{{4 \times 3}},……….{/tex}

Therefore, the next four rational numbers of this pattern would be

{tex}\frac{{ – 1 \times 4}}{{4 \times 4}},\frac{{ – 1 \times 5}}{{4 \times 5}},\frac{{ – 1 \times 6}}{{4 \times 6}},\frac{{ – 1 \times 7}}{{4 \times 7}}{/tex} = {tex}\frac{{ – 4}}{{16}},\frac{{ – 5}}{{20}},\frac{{ – 6}}{{24}},\frac{{ – 7}}{{28}}{/tex}

(iii) {tex}\frac{{ – 1}}{6},\frac{2}{{ – 12}},\frac{3}{{ – 18}},\frac{4}{{ – 24}},………{/tex}

{tex} \Rightarrow {/tex} {tex}\frac{{ – 1 \times 1}}{{6 \times 1}},\frac{{1 \times 2}}{{ – 6 \times 2}},\frac{{1 \times 3}}{{ – 6 \times 3}},\frac{{1 \times 4}}{{ – 6 \times 4}},………{/tex}

Therefore, the next four rational numbers of this pattern would be

{tex}\frac{{1 \times 5}}{{ – 6 \times 5}},\frac{{1 \times 6}}{{ – 6 \times 6}},\frac{{1 \times 7}}{{ – 6 \times 7}},\frac{{1 \times 8}}{{ – 6 \times 8}}{/tex} = {tex}\frac{5}{{ – 30}},\frac{6}{{ – 36}},\frac{7}{{ – 42}},\frac{8}{{ – 48}}{/tex}

(iv) {tex}\frac{{ – 2}}{3},\frac{2}{{ – 3}},\frac{4}{{ – 6}},\frac{6}{{ – 9}},……….{/tex}

{tex} \Rightarrow {/tex} {tex}\frac{{ – 2 \times 1}}{{3 \times 1}},\frac{{2 \times 1}}{{ – 3 \times 1}},\frac{{2 \times 2}}{{ – 3 \times 2}},\frac{{2 \times 3}}{{ – 3 \times 3}},……….{/tex}

Therefore, the next four rational numbers of this pattern would be

{tex}\frac{{2 \times 4}}{{ – 3 \times 4}},\frac{{2 \times 5}}{{ – 3 \times 5}},\frac{{2 \times 6}}{{ – 3 \times 6}},\frac{{2 \times 7}}{{ – 3 \times 7}}{/tex} = {tex}\frac{8}{{ – 12}},\frac{{10}}{{ – 15}},\frac{{12}}{{ – 18}},\frac{{14}}{{ – 21}}{/tex}

---

NCERT Solutions for Class 7 Maths Exercise 9.1

Question 3.Give four rational numbers equivalent to:

(i) {tex}\frac{{ – 2}}{7}{/tex}

(ii) {tex}\frac{5}{{ – 3}}{/tex}

(iii) {tex}\frac{4}{9}{/tex}

Answer:

(i) {tex}\frac{{ – 2}}{7}{/tex}

{tex}\frac{{ – 2 \times 2}}{{7 \times 2}} = \frac{{ – 4}}{{14}},{/tex}{tex}\frac{{ – 2 \times 3}}{{7 \times 3}} = \frac{{ – 6}}{{21}},{/tex}{tex}\frac{{ – 2 \times 4}}{{7 \times 4}} = \frac{{ – 8}}{{28}},{/tex}{tex}\frac{{ – 2 \times 5}}{{7 \times 5}} = \frac{{ – 10}}{{35}}{/tex}

Therefore, four equivalent rational numbers are {tex}\frac{{ – 4}}{{14}},\frac{{ – 6}}{{21}},\frac{{ – 8}}{{28}},\frac{{ – 10}}{{35}}{/tex}.

(ii) {tex}\frac{5}{{ – 3}}{/tex}

{tex}\frac{{5 \times 2}}{{ – 3 \times 2}} = \frac{{10}}{{ – 6}},{/tex}{tex}\frac{{5 \times 3}}{{ – 3 \times 3}} = \frac{{15}}{{ – 9}},{/tex}{tex}\frac{{5 \times 4}}{{ – 3 \times 4}} = \frac{{20}}{{ – 12}},{/tex}{tex}\frac{{5 \times 5}}{{ – 3 \times 5}} = \frac{{25}}{{ – 15}}{/tex}

Therefore, four equivalent rational numbers are {tex}\frac{{10}}{{ – 6}},\frac{{15}}{{ – 9}},\frac{{20}}{{ – 12}},\frac{{25}}{{ – 15}}{/tex}.

(iii) {tex}\frac{4}{9}{/tex}

{tex}\frac{{4 \times 2}}{{9 \times 2}} = \frac{8}{{18}},{/tex}{tex}\frac{{4 \times 3}}{{9 \times 3}} = \frac{{12}}{{27}},{/tex}{tex}\frac{{4 \times 4}}{{9 \times 4}} = \frac{{16}}{{36}},{/tex}{tex}\frac{{4 \times 5}}{{9 \times 5}} = \frac{{20}}{{45}}{/tex}

Therefore, four equivalent rational numbers are {tex}\frac{8}{{18}},\frac{{12}}{{27}},\frac{{16}}{{36}},\frac{{20}}{{45}}{/tex}.

---

Question 4.Draw the number line and represent the following rational numbers on it:

(i) {tex}\frac{3}{4}{/tex}

(ii) {tex}\frac{{ – 5}}{8}{/tex}

(iii) {tex}\frac{{ – 7}}{4}{/tex}

(iv) {tex}\frac{7}{8}{/tex}

Answer:

(i) {tex}\frac{3}{4}{/tex}

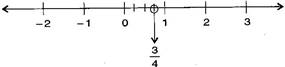
(ii) {tex}\frac{{ – 5}}{8}{/tex}

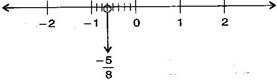
(iii) {tex}\frac{{ – 7}}{4}{/tex}

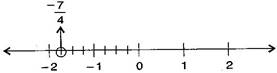
(iv) {tex}\frac{7}{8}{/tex}

---

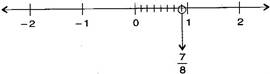
NCERT Solutions for Class 7 Maths Exercise 9.1

Question 5.The points P, Q, R, S, T, U, A and B on the number line are such that, TR = RS = SU and AP = PQ = QB. Name the rational numbers represented by P, Q, R and S.

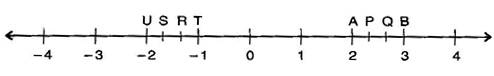
Answer:

Each part which is between the two numbers is divided into 3 parts.

Therefore, A = {tex}\frac{6}{3},{/tex} P = {tex}\frac{7}{3},{/tex} Q = {tex}\frac{8}{3}{/tex} and B = {tex}\frac{9}{3}{/tex}

Similarly T = {tex}\frac{{ – 3}}{3},{/tex} R = {tex}\frac{{ – 4}}{3},{/tex} S = {tex}\frac{{ – 5}}{3}{/tex} and U = {tex}\frac{{ – 6}}{3}{/tex}

Thus, the rational numbers represented P, Q, R and S are {tex}\frac{7}{3},\frac{8}{3},\frac{{ – 4}}{3}{/tex} and {tex}\frac{{ – 5}}{3}{/tex} respectively.

---

NCERT Solutions for Class 7 Maths Exercise 9.1

Question 6.Which of the following pairs represent the same rational numbers:

(i) {tex}\frac{{ – 7}}{{21}}{/tex} and {tex}\frac{3}{9}{/tex}

(ii) {tex}\frac{{ – 16}}{{20}}{/tex} and {tex}\frac{{20}}{{ – 25}}{/tex}

(iii) {tex}\frac{{ – 2}}{{ – 3}}{/tex} and {tex}\frac{2}{3}{/tex}

(iv) {tex}\frac{{ – 3}}{5}{/tex} and {tex}\frac{{ – 12}}{{20}}{/tex}

(v) {tex}\frac{8}{{ – 5}}{/tex} and {tex}\frac{{ – 24}}{{15}}{/tex}

(vi) {tex}\frac{1}{3}{/tex} and {tex}\frac{{ – 1}}{9}{/tex}

(vii) {tex}\frac{{ – 5}}{{ – 9}}{/tex} and {tex}\frac{5}{{ – 9}}{/tex}

Answer:

(i) {tex}\frac{{ – 7}}{{21}}{/tex} and {tex}\frac{3}{9}{/tex}

{tex} \Rightarrow {/tex} {tex}\frac{{ – 7}}{{21}}{/tex} = {tex}\frac{{ – 1}}{3}{/tex} and {tex}\frac{3}{9}{/tex} = {tex}\frac{1}{3}{/tex} [Converting into lowest term]

{tex}\because {/tex} {tex}\frac{{ – 1}}{3}{/tex}{tex} \ne {/tex}{tex}\frac{1}{3}{/tex}

{tex}\therefore {/tex} {tex}\frac{{ – 7}}{{21}}{/tex}{tex} \ne {/tex}{tex}\frac{3}{9}{/tex}

(ii) {tex}\frac{{ – 16}}{{20}}{/tex} and {tex}\frac{{20}}{{ – 25}}{/tex}

{tex} \Rightarrow {/tex} {tex}\frac{{ – 16}}{{20}}{/tex} = {tex}\frac{{ – 4}}{5}{/tex} and {tex}\frac{{20}}{{ – 25}}{/tex} = {tex}\frac{4}{{ – 5}} = \frac{{ – 4}}{5}{/tex}

[Converting into lowest term]

{tex}\because {/tex} {tex}\frac{{ – 4}}{5}{/tex} = {tex}\frac{{ – 4}}{5}{/tex}

{tex}\therefore {/tex} {tex}\frac{{ – 16}}{{20}}{/tex} = {tex}\frac{{20}}{{ – 25}}{/tex}

(iii) {tex}\frac{{ – 2}}{{ – 3}}{/tex} and {tex}\frac{2}{3}{/tex}

{tex} \Rightarrow {/tex} {tex}\frac{{ – 2}}{{ – 3}}{/tex} = {tex}\frac{2}{3}{/tex} and {tex}\frac{2}{3}{/tex} = {tex}\frac{2}{3}{/tex} [Converting into lowest term]

{tex}\because {/tex} {tex}\frac{2}{3}{/tex} = {tex}\frac{2}{3}{/tex}

{tex}\therefore {/tex} {tex}\frac{{ – 2}}{{ – 3}}{/tex} = {tex}\frac{2}{3}{/tex}

(iv) {tex}\frac{{ – 3}}{5}{/tex} and {tex}\frac{{ – 12}}{{20}}{/tex}

{tex} \Rightarrow {/tex} {tex}\frac{{ – 3}}{5}{/tex} = {tex}\frac{{ – 3}}{5}{/tex} and {tex}\frac{{ – 12}}{{20}}{/tex} = {tex}\frac{{ – 3}}{5}{/tex} [Converting into lowest term]

{tex}\because {/tex} {tex}\frac{{ – 3}}{5}{/tex} = {tex}\frac{{ – 3}}{5}{/tex}

{tex}\therefore {/tex} {tex}\frac{{ – 3}}{5}{/tex} = {tex}\frac{{ – 12}}{{20}}{/tex}

(v) {tex}\frac{8}{{ – 5}}{/tex} and {tex}\frac{{ – 24}}{{15}}{/tex}

{tex} \Rightarrow {/tex} {tex}\frac{8}{{ – 5}}{/tex} = {tex}\frac{{ – 8}}{5}{/tex} and {tex}\frac{{ – 24}}{{15}}{/tex} = {tex}\frac{{ – 8}}{5}{/tex} [Converting into lowest term]

{tex}\because {/tex} {tex}\frac{{ – 8}}{5}{/tex} = {tex}\frac{{ – 8}}{5}{/tex}

{tex}\therefore {/tex} {tex}\frac{8}{{ – 5}}{/tex} = {tex}\frac{{ – 24}}{{15}}{/tex}

(vi) {tex}\frac{1}{3}{/tex} and {tex}\frac{{ – 1}}{9}{/tex}

{tex} \Rightarrow {/tex} {tex}\frac{1}{3}{/tex} = {tex}\frac{1}{3}{/tex} and {tex}\frac{{ – 1}}{9}{/tex} = {tex}\frac{{ – 1}}{9}{/tex} [Converting into lowest term]

{tex}\because {/tex} {tex}\frac{1}{3}{/tex}{tex} \ne {/tex}{tex}\frac{{ – 1}}{9}{/tex}

{tex}\therefore {/tex} {tex}\frac{1}{3}{/tex}{tex} \ne {/tex}{tex}\frac{{ – 1}}{9}{/tex}

(vii) {tex}\frac{{ – 5}}{{ – 9}}{/tex} and {tex}\frac{5}{{ – 9}}{/tex}

{tex} \Rightarrow {/tex} {tex}\frac{{ – 5}}{{ – 9}}{/tex} = {tex}\frac{5}{9}{/tex} and {tex}\frac{5}{{ – 9}}{/tex} = {tex}\frac{5}{9}{/tex} [Converting into lowest term]

{tex}\because {/tex} {tex}\frac{5}{9}{/tex}{tex} \ne {/tex}{tex}\frac{5}{{ – 9}}{/tex}

{tex}\therefore {/tex} {tex}\frac{{ – 5}}{{ – 9}}{/tex}{tex} \ne {/tex}{tex}\frac{5}{{ – 9}}{/tex}

---

NCERT Solutions for Class 7 Maths Exercise 9.1

Question 7.Rewrite the following rational numbers in the simplest form:

(i) {tex}\frac{{ – 8}}{6}{/tex}

(ii) {tex}\frac{{25}}{{45}}{/tex}

(iii) {tex}\frac{{ – 44}}{{72}}{/tex}

(iv) {tex}\frac{{ – 8}}{{10}}{/tex}

Answer:

(i) {tex}\frac{{ – 8}}{6}{/tex} = {tex}\frac{{ – 8 \div 2}}{{6 \div 2}}{/tex} = {tex}\frac{{ – 4}}{3}{/tex} [H.C.F. of 8 and 6 is 2]

(ii){tex}\frac{{25}}{{45}}{/tex} = {tex}\frac{{25 \div 5}}{{45 \div 5}}{/tex} = {tex}\frac{5}{9}{/tex}

[H.C.F. of 25 and 45 is 5]

(iii){tex}\frac{{ – 44}}{{72}}{/tex} = {tex}\frac{{ – 44 \div 4}}{{72 \div 4}}{/tex} = {tex}\frac{{ – 11}}{{18}}{/tex} [H.C.F. of 44 and 72 is 4]

(iv) {tex}\frac{{ – 8}}{{10}}{/tex} = {tex}\frac{{ – 8 \div 2}}{{10 \div 2}}{/tex} = {tex}\frac{{ – 4}}{5}{/tex} [H.C.F. of 8 and 10 is 2]

---

NCERT Solutions for Class 7 Maths Exercise 9.1

Question 8.Fill in the boxes with the correct symbol out of <, > and =:

(i) {tex}\frac{{ – 5}}{7}\boxed{{\text{ }}}\frac{2}{3}{/tex}

(ii) {tex}\frac{{ – 4}}{5}\boxed{{\text{ }}}\frac{{ – 5}}{7}{/tex}

(iii) {tex}\frac{{ – 7}}{8}\boxed{{\text{ }}}\frac{{14}}{{ – 16}}{/tex}

(iv) {tex}\frac{{ – 8}}{5}\boxed{{\text{ }}}\frac{{ – 7}}{4}{/tex}

(v) {tex}\frac{1}{{ – 3}}\boxed{{\text{ }}}\frac{{ – 1}}{4}{/tex}

(vi) {tex}\frac{5}{{ – 11}}\boxed{{\text{ }}}\frac{{ – 5}}{{11}}{/tex}

(vii) {tex}0\boxed{{\text{ }}}\frac{{ – 7}}{6}{/tex}

Answer:

(i) {tex}\frac{{ – 5}}{7}\boxed{{\text{ < }}}\frac{2}{3}{/tex} Since, the positive number if greater than negative number.

(ii) {tex}\frac{{ – 4 \times 7}}{{5 \times 7}}\boxed{{\text{ }}}\frac{{ – 5 \times 5}}{{7 \times 5}}{/tex} {tex} \Rightarrow {/tex} {tex}\frac{{ – 28}}{{35}}\boxed{{\text{ < }}}\frac{{ – 25}}{{35}}{/tex} {tex} \Rightarrow {/tex} {tex}\frac{{ – 4}}{5}\boxed{{\text{ < }}}\frac{{ – 5}}{7}{/tex}

(iii) {tex}\frac{{ – 7 \times 2}}{{8 \times 2}}\boxed{{\text{ }}}\frac{{14 \times \left( { – 1} \right)}}{{ – 16 \times \left( { – 1} \right)}}{/tex} {tex} \Rightarrow {/tex} {tex}\frac{{ – 14}}{{16}}\boxed{{\text{ = }}}\frac{{ – 14}}{{16}}{/tex} {tex} \Rightarrow {/tex}{tex}\frac{{ – 7}}{8}\boxed{{\text{ = }}}\frac{{14}}{{ – 16}}{/tex}

(iv) {tex}\frac{{ – 8 \times 4}}{{5 \times 4}}\boxed{{\text{ }}}\frac{{ – 7 \times 5}}{{4 \times 5}}{/tex} {tex} \Rightarrow {/tex} {tex}\frac{{ – 32}}{{20}}\boxed{{\text{ > }}}\frac{{ – 35}}{{20}}{/tex} {tex} \Rightarrow {/tex} {tex}\frac{{ – 8}}{5}\boxed{{\text{ > }}}\frac{{ – 7}}{4}{/tex}

(v) {tex}\frac{1}{{ – 3}}\boxed{{\text{ }}}\frac{{ – 1}}{4}{/tex} {tex} \Rightarrow {/tex}{tex}\frac{1}{{ – 3}}\boxed{{\text{ < }}}\frac{{ – 1}}{4}{/tex}

(vi) {tex}\frac{5}{{ – 11}}\boxed{{\text{ }}}\frac{{ – 5}}{{11}}{/tex} {tex} \Rightarrow {/tex}{tex}\frac{5}{{ – 11}}\boxed{{\text{ = }}}\frac{{ – 5}}{{11}}{/tex}

(vii) {tex}0\boxed{{\text{ > }}}\frac{{ – 7}}{6}{/tex} Since, 0 is greater than every negative number.

---

NCERT Solutions for Class 7 Maths Exercise 9.1

Question 9.Which is greater in each of the following:

(i) {tex}\frac{2}{3},\frac{5}{2}{/tex}

(ii) {tex}\frac{{ – 5}}{6},\frac{{ – 4}}{3}{/tex}

(iii) {tex}\frac{{ – 3}}{4},\frac{2}{{ – 3}}{/tex}

(iv) {tex}\frac{{ – 1}}{4},\frac{1}{4}{/tex}

(v) {tex} – 3\frac{2}{7}, – 3\frac{4}{5}{/tex}

Answer:

(i) {tex}\frac{{2 \times 2}}{{3 \times 2}} = \frac{4}{6}{/tex} and {tex}\frac{{5 \times 3}}{{2 \times 3}} = \frac{{15}}{6}{/tex}

Since {tex}\frac{4}{6}\boxed{{\text{ < }}}\frac{{15}}{6}{/tex}

Therefore {tex}\frac{2}{3}\boxed{{\text{ }} < {\text{ }}}\frac{5}{2}{/tex}

(ii) {tex}\frac{{ – 5 \times 1}}{{6 \times 1}} = \frac{{ – 5}}{6}{/tex} and {tex}\frac{{ – 4 \times 2}}{{3 \times 2}} = \frac{{ – 8}}{6}{/tex}

Since {tex}\frac{{ – 5}}{6}\boxed{{\text{ > }}}\frac{{ – 8}}{6}{/tex} Therefore {tex}\frac{{ – 5}}{6}\boxed{{\text{ > }}}\frac{{ – 4}}{3}{/tex}

(iii) {tex}\frac{{ – 3 \times 3}}{{4 \times 3}} = \frac{{ – 9}}{{12}}{/tex} and {tex}\frac{{2 \times \left( { – 4} \right)}}{{ – 3 \times \left( { – 4} \right)}} = \frac{{ – 8}}{{12}}{/tex}

Since {tex}\frac{{ – 9}}{{12}}\boxed{{\text{ < }}}\frac{{ – 8}}{{12}}{/tex}

Therefore {tex}\frac{{ – 3}}{4}\boxed{{\text{ }} < {\text{ }}}\frac{2}{{ – 3}}{/tex}

(iv) {tex}\frac{{ – 1}}{4}\boxed{{\text{ < }}}\frac{1}{4}{/tex} Since positive number is always greater than negative number.

(v) {tex} – 3\frac{2}{7} = \frac{{ – 23}}{7} = \frac{{ – 23 \times 5}}{{7 \times 5}} = \frac{{ – 115}}{{35}}{/tex} and {tex} – 3\frac{4}{5} = \frac{{ – 19}}{5} = \frac{{ – 19 \times 7}}{{5 \times 7}} = \frac{{ – 133}}{{35}}{/tex}

Since {tex}\frac{{ – 115}}{{35}}\boxed{{\text{ > }}}\frac{{ – 133}}{{35}}{/tex}

Therefore{tex} – 3\frac{2}{7}\boxed{{\text{ > }}} – 3\frac{4}{5}{/tex}

---

NCERT Solutions for Class 7 Maths Exercise 9.1

Question 10.Write the following rational numbers in ascending order:

(i) {tex}\frac{{ – 3}}{5},\frac{{ – 2}}{5},\frac{{ – 1}}{5}{/tex}

(ii) {tex}\frac{1}{3},\frac{{ – 2}}{9},\frac{{ – 4}}{3}{/tex}

(iii) {tex}\frac{{ – 3}}{7},\frac{{ – 3}}{2},\frac{{ – 3}}{4}{/tex}

Answer:

(i) {tex}\frac{{ – 3}}{5},\frac{{ – 2}}{5},\frac{{ – 1}}{5}{/tex}{tex} \Rightarrow {/tex} {tex}\frac{{ – 3}}{5} < \frac{{ – 2}}{5} < \frac{{ – 1}}{5}{/tex}

(ii) {tex}\frac{1}{3},\frac{{ – 2}}{9},\frac{{ – 4}}{3}{/tex} {tex} \Rightarrow {/tex}{tex}\frac{3}{9},\frac{{ – 2}}{9},\frac{{ – 12}}{9}{/tex} [Converting into same denominator]

Now {tex}\frac{{ – 12}}{9} < \frac{{ – 2}}{9} < \frac{3}{9}{/tex} {tex} \Rightarrow {/tex} {tex}\frac{{ – 4}}{3} < \frac{{ – 2}}{9} < \frac{1}{3}{/tex}

(iii) {tex}\frac{{ – 3}}{7},\frac{{ – 3}}{2},\frac{{ – 3}}{4}{/tex}

{tex} \Rightarrow {/tex} {tex}\frac{{ – 3}}{2} < \frac{{ – 3}}{4} < \frac{{ – 3}}{7}{/tex}

NCERT Solutions for Class 7 Maths Exercise 9.1

NCERT Solutions Class 7 Maths PDF (Download) Free from myCBSEguide app and myCBSEguide website. Ncert solution class 7 Maths includes text book solutions from Class 7 Maths Book . NCERT Solutions for CBSE Class 7 Maths have total 15 chapters. 7 Maths NCERT Solutions in PDF for free Download on our website. Ncert Maths class 7 solutions PDF and Maths ncert class 7 PDF solutions with latest modifications and as per the latest CBSE syllabus are only available in myCBSEguide.

CBSE app for Students

To download NCERT Solutions for Class 7 Maths, Social Science Computer Science, Home Science, Hindi English, Maths Science do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT solutions, NCERT Exemplar solutions, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.