NCERT Solutions for Class 9 Maths Exercise 9.4 book solutions are available in PDF format for free download. These ncert book chapter wise questions and answers are very helpful for CBSE board exam. CBSE recommends NCERT books and most of the questions in CBSE exam are asked from NCERT text books. Class 9 Maths chapter wise NCERT solution for Maths Book for all the chapters can be downloaded from our website and myCBSEguide mobile app for free.
NCERT solutions for Class 9 Maths Areas of Parallelograms and Triangles Download as PDF

NCERT Solutions for Class 9 Mathematics Areas of Parallelograms and Triangles
1. Parallelogram ABCD and rectangle ABEF are on the same base AB and have equal areas. Show that the perimeter of the parallelogram is greater than that of the rectangle.
Ans. Given: Parallelogram ABCD and rectangle ABEF are on same base AB and between the same parallels AB and CF.

 ar (
ar ( gm ABCD) = ar (rect. ABEF)
gm ABCD) = ar (rect. ABEF)
To prove: AB + BC + CD + AD > AB + BE + EF + AF
Proof: AB = CD [ opposites sides of a parallelogram are always equal]
opposites sides of a parallelogram are always equal]
AB = EF [ opposites sides of a rectangle are always equal]
opposites sides of a rectangle are always equal]
 CD = EF
CD = EF
Adding AB both sides,
AB + CD = AB + EF ……….(i)
 Off all the segments that can be drawn to a given line from a point not lying on it, the perpendicular segment is the shortest.
Off all the segments that can be drawn to a given line from a point not lying on it, the perpendicular segment is the shortest.
 BE < BC and AF < AD
BE < BC and AF < AD
 BC > BE and AD > AF
BC > BE and AD > AF
 BC + AD > BE + AF ……….(ii)
BC + AD > BE + AF ……….(ii)
From eq. (i) and (ii),
AB + CD + BC + AD = AB + EF + BE + AF
2. In figure, D and E are two points on BC such that BD = DE = EC. Show that ar (ABD) = ar (ADE) = ar (AEC). Can you know answer the question that you have left in the ‘introduction’ of this chapter, whether the field of Budhia has been actually divided into three parts of equal area?
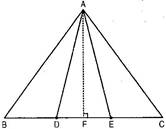
Ans. In  ABC, points D and E divides BC in three equal parts such that BD = DE = EC.
ABC, points D and E divides BC in three equal parts such that BD = DE = EC.
 BD = DE = EC =
BD = DE = EC = 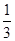 BC
BC
Draw AF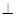 BC
BC
ar ( ABC) =
ABC) = 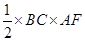 ……….(i)
……….(i)
and ar ( ABD) =
ABD) =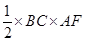 ……….(ii)
……….(ii)
= 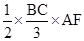 =
= 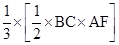
= 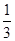 ar (
ar ( ABC) ……….(iii)
ABC) ……….(iii)
And ar ( AEC) =
AEC) = 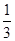 ar (
ar ( ABC) ……….(iv)
ABC) ……….(iv)
From (ii), (iii) and (iv),
ar ( ABD) = ar (
ABD) = ar ( ADE) = ar (
ADE) = ar ( AEC)
AEC)
NCERT Solutions for Class 9 Maths Exercise 9.4
3. In figure, ABCD, DCFE and ABFE are parallelograms. Show that ar (ADE) = ar (BCF).
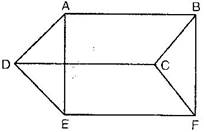
Ans. As we know that opposite sides of a parallelogram are always equal.
 In parallelogram ABFE, AE = BF and AB = EF
In parallelogram ABFE, AE = BF and AB = EF
In parallelogram DCFE, DE = CF and DC = EF
In parallelogram ABCD, AD = BC and AB = DC
Now in  ADE and
ADE and  BCF,
BCF,
AE = BF [Opposite sides of parallelogram ABFE]
DE = CF [Opposite sides of parallelogram DCFE]
And AD = BC [Opposite sides of parallelogram ABCD]

 ADE
ADE 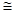
 BCF [By SSS congruency]
BCF [By SSS congruency]
 ar (
ar ( ADE) = ar (
ADE) = ar ( BCF)
BCF)
[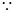 Area of two congruent figures is always equal]
Area of two congruent figures is always equal]
NCERT Solutions for Class 9 Maths Exercise 9.4
4. In figure, ABCD is a parallelogram and BC is produced to a point Q such that AD = CQ. If AQ intersects DC at P, show that ar (BPC) = ar (DPQ).

Ans. Join A and C.
 APC and
APC and  BPC are on the same base PC and between the same parallels PC and AB.
BPC are on the same base PC and between the same parallels PC and AB.
 ar (
ar ( APC) = ar (
APC) = ar ( BPC) ……….(i)
BPC) ……….(i)
Now ACBD is a parallelogram.
AD = BC [opposite sides of a parallelogram are always equal]
Also BC = CQ [given]
 AD = CQ
AD = CQ
Now AD  CQ [Since CQ is the extension of BC]
CQ [Since CQ is the extension of BC]
And AD = CQ
 ADQC is a parallelogram.
ADQC is a parallelogram.
[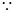 If one pair of opposite sides of a quadrilateral is equal and parallel then it is a parallelogram]
If one pair of opposite sides of a quadrilateral is equal and parallel then it is a parallelogram]
Since diagonals of a parallelogram bisect each other.
 AP = PQ and CP = DP
AP = PQ and CP = DP
Now in  APC and
APC and  DPQ,
DPQ,
AP = PQ [Proved above]
 APC =
APC =  DPQ [Vertically opposite angles]
DPQ [Vertically opposite angles]
PC = PD [Prove above]

 APC
APC 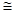
 DPQ ……….(ii)
DPQ ……….(ii)
 ar (
ar ( APC) = ar (
APC) = ar ( DPQ) [area of congruent figures is always equal]
DPQ) [area of congruent figures is always equal]
From eq. (i) and (ii),
ar ( BPC) = ar (
BPC) = ar ( DPQ)
DPQ)
NCERT Solutions for Class 9 Maths Exercise 9.4
5. In figure, ABC and BDF are two equilateral triangles such that D is the mid-point of BC. If AE intersects BC at F, show that:

(i) ar (BDE) = 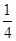 ar (ABC)
ar (ABC)
(ii) ar (BDE) =  ar (BAE)
ar (BAE)
(iii) ar (ABC) = 2 ar (BEC)
(iv) ar (BFE) = ar (AFD)
(v) ar (BFE) = 2 ar (FED)
(vi) ar (FED) = 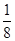 ar (AFC)
ar (AFC)
Ans. Join EC and AD.
Since  ABC is an equilateral triangle.
ABC is an equilateral triangle.

 A =
A =  B =
B =  C =
C = 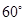
Also  BDE is an equilateral triangle.
BDE is an equilateral triangle.

 B =
B =  D =
D =  E =
E = 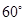
If we take two lines, AC and BE and BC as a transversal.
Then  B =
B =  C =
C = 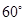 [Alternate angles]
[Alternate angles]
 BE
BE  AC
AC
Similarly, for lines AB and DE and BF as transversal.
Then  B =
B =  C =
C = 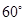 [Alternate angles]
[Alternate angles]
 BE
BE  AC
AC
(i) Area of equilateral triangle BDE = 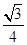 (BD)2 ……….(i)
(BD)2 ……….(i)
Area of equilateral triangle ABC = 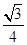 (BC)2 ……….(ii)
(BC)2 ……….(ii)
Dividing eq. (i) by (ii),
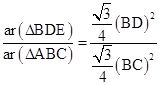

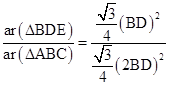 [
[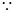 BD = DC]
BD = DC]


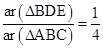
 ar (
ar ( BDE) =
BDE) = 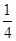 ar (
ar ( ABC)
ABC)
(ii) In  BEC, ED is the median.
BEC, ED is the median.
 ar (
ar ( BEC) = ar (
BEC) = ar ( BAE) ……….(i)
BAE) ……….(i)
[Median divides the triangle in two triangles having equal area]
Now BE  AC
AC
And  BEC and
BEC and  BAE are on the same base BE and between the same parallels BE and AC.
BAE are on the same base BE and between the same parallels BE and AC.
 ar (
ar ( BEC) = ar (
BEC) = ar ( BAE) ……….(ii)
BAE) ……….(ii)
Using eq. (i) and (ii), we get
Ar ( BDE) =
BDE) =  ar (
ar ( BAE)
BAE)
(iii) We have ar ( BDE) =
BDE) = 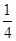 ar (
ar ( ABC) [Proved in part (i)] ……….(iii)
ABC) [Proved in part (i)] ……….(iii)
ar ( BDE) =
BDE) = 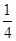 ar (
ar ( BAE) [Proved in part (ii)]
BAE) [Proved in part (ii)]
ar ( BDE) =
BDE) = 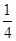 ar (
ar ( BEC) [Using eq. (iii)] ……….(iv)
BEC) [Using eq. (iii)] ……….(iv)
From eq. (iii) and (iv), we het
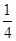 ar (
ar ( ABC) =
ABC) = 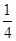 ar (
ar ( BEC)
BEC)
 ar (
ar ( ABC) = 2 ar (
ABC) = 2 ar ( BEC)
BEC)
(iv)  BDE and
BDE and  AED are on the same base DE and between same parallels AB and DE.
AED are on the same base DE and between same parallels AB and DE.
 ar (
ar ( BDE) = ar (
BDE) = ar ( AED)
AED)
Subtracting  FED from both the sides,
FED from both the sides,
ar ( BDE) – ar (
BDE) – ar ( FED) = ar (
FED) = ar ( AED) – ar (
AED) – ar ( FED)
FED)
 ar (
ar ( BFE) = ar (
BFE) = ar ( AFD) ……….(v)
AFD) ……….(v)
(v) An in equilateral triangle, median drawn is also perpendicular to the side,
 AD
AD  BC
BC
Now ar ( AFD) =
AFD) =  ……….(vi)
……….(vi)
Draw EG 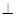 BC
BC
 ar (
ar ( FED) =
FED) =  ……….(vii)
……….(vii)
Dividing eq. (vi) by (vii), we get
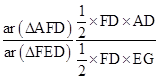

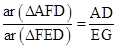

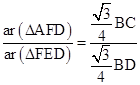 [Altitude of equilateral triangle =
[Altitude of equilateral triangle = 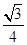 side]
side]

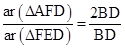 [D is the mid-point of BC]
[D is the mid-point of BC]

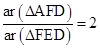
 ar (
ar ( AFD) = 2 ar (
AFD) = 2 ar ( FED) ……(viii)
FED) ……(viii)
Using the value of eq. (viii) in eq. (v),
Ar ( BFE) = 2 ar (
BFE) = 2 ar ( FED)
FED)
(vi) ar ( AFC) = ar (
AFC) = ar ( AFD) + ar (
AFD) + ar ( ADC) = 2 ar (
ADC) = 2 ar ( FED) +
FED) +  ar (
ar ( ABC) [using (v)
ABC) [using (v)
= 2 ar ( FED) +
FED) +  [4
[4 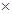 ar (
ar ( BDE)] [Using result of part (i)]
BDE)] [Using result of part (i)]
= 2 ar ( FED) + 2 ar (
FED) + 2 ar ( BDE) = 2 ar (
BDE) = 2 ar ( FED) + 2 ar (
FED) + 2 ar ( AED)
AED)
[ BDE and
BDE and  AED are on the same base and between same parallels]
AED are on the same base and between same parallels]
= 2 ar ( FED) + 2 [ar (
FED) + 2 [ar ( AFD) + ar (
AFD) + ar ( FED)]
FED)]
= 2 ar ( FED) + 2 ar (
FED) + 2 ar ( AFD) + 2 ar (
AFD) + 2 ar ( FED) [Using (viii)]
FED) [Using (viii)]
= 4 ar ( FED) + 4 ar (
FED) + 4 ar ( FED)
FED)
 ar (
ar ( AFC) = 8 ar (
AFC) = 8 ar ( FED)
FED)
 ar (
ar ( FED) =
FED) = 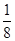 ar (
ar ( AFC)
AFC)
NCERT Solutions for Class 9 Maths Exercise 9.4
6. Diagonals AC and BD of a quadrilateral ABCD intersect each other at P. Show that:
ar (APB) 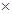 ar (CPD) = ar (APD)
ar (CPD) = ar (APD) 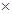 ar (BPC)
ar (BPC)
Ans. Given: A quadrilateral ABCD, in which diagonals AC and BD intersect each other at point E.
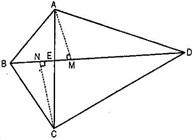
To Prove: ar ( AED)
AED)  ar (
ar ( BEC)
BEC)
= ar ( ABE)
ABE)  ar (
ar ( CDE)
CDE)
Construction: From A, draw AM 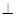 BD and from C, draw CN
BD and from C, draw CN 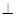 BD.
BD.
Proof: ar ( ABE) =
ABE) = 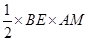 ……….(i)
……….(i)
And ar ( AED) =
AED) = 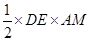 ……….(ii)
……….(ii)
Dividing eq. (ii) by (i), we get,
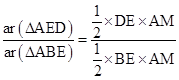

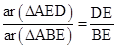 ……….(iii)
……….(iii)
Similarly  ……….(iv)
……….(iv)
From eq. (iii) and (iv), we get
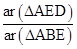 =
= 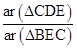
 ar (
ar ( AED)
AED)  ar (
ar ( BEC) = ar (
BEC) = ar ( ABE)
ABE)  ar (
ar ( CDE)
CDE)
Hence proved.
NCERT Solutions for Class 9 Maths Exercise 9.4
7. P and Q are respectively the mid-points of sides AB and BC or a triangle ABC and R is the mid-point of AP, show that:
(i) ar (PRQ) =  ar (ARC)
ar (ARC)
(ii) ar (RQC) = 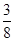 ar (ABC)
ar (ABC)
(iii) ar (PBQ) = ar (ARC)
Ans. (i) PC is the median of  ABC.
ABC.
 ar (
ar ( BPC) = ar (
BPC) = ar ( APC) ……….(i)
APC) ……….(i)
RC is the median of  APC.
APC.
 ar (
ar ( ARC) =
ARC) =  ar (
ar ( APC) ……….(ii)
APC) ……….(ii)
[Median divides the triangle into two triangles of equal area]
PQ is the median of  BPC.
BPC.

 ar (
ar ( PQC) =
PQC) =  ar (
ar ( BPC) ……….(iii)
BPC) ……….(iii)
From eq. (i) and (iii), we get,
ar ( PQC) =
PQC) =  ar (
ar ( APC) ……….(iv)
APC) ……….(iv)
From eq. (ii) and (iv), we get,
ar ( PQC) = ar (
PQC) = ar ( ARC) ……….(v)
ARC) ……….(v)
We are given that P and Q are the mid-points of AB and BC respectively.
 PQ
PQ  AC and PA =
AC and PA =  AC
AC
 ar (
ar ( APQ) = ar (
APQ) = ar ( PQC) ……….(vi) [triangles between same parallel are equal in area]
PQC) ……….(vi) [triangles between same parallel are equal in area]
From eq. (v) and (vi), we get
ar ( APQ) = ar (
APQ) = ar ( ARC) ……….(vii)
ARC) ……….(vii)
R is the mid-point of AP. Therefore RQ is the median of  APQ.
APQ.
 ar (
ar ( PRQ) =
PRQ) =  ar (
ar ( APQ) ……….(viii)
APQ) ……….(viii)
From (vii) and (viii), we get,
ar ( PRQ) =
PRQ) =  ar (
ar ( ARC)
ARC)
(ii) PQ is the median of  BPC
BPC
 ar (
ar ( PQC) =
PQC) =  ar (
ar ( BPC) =
BPC) = 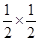 ar (
ar ( ABC) =
ABC) = 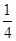 ar (
ar ( ABC) ……….(ix)
ABC) ……….(ix)
Also ar ( PRC) =
PRC) =  ar (
ar ( APC) [Using (iv)]
APC) [Using (iv)]
 ar (
ar ( PRC) =
PRC) = 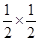 ar (
ar ( ABC) =
ABC) = 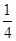 ar (
ar ( ABC) ……….(x)
ABC) ……….(x)
Adding eq. (ix) and (x), we get,
ar ( PQC) + ar (
PQC) + ar ( PRC) =
PRC) = 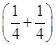 ar (
ar ( ABC)
ABC)
 ar (quad. PQCR) =
ar (quad. PQCR) =  ar (
ar ( ABC) ……….(xi)
ABC) ……….(xi)
Subtracting ar ( PRQ) from the both sides,
PRQ) from the both sides,
ar (quad. PQCR) – ar ( PRQ) =
PRQ) =  ar (
ar ( ABC) – ar (
ABC) – ar ( PRQ)
PRQ)
 ar (
ar ( RQC) =
RQC) =  ar (
ar ( ABC) –
ABC) –  ar (
ar ( ARC) [Using result (i)]
ARC) [Using result (i)]
 ar (
ar ( ARC) =
ARC) =  ar (
ar ( ABC) –
ABC) – 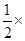
 ar (
ar ( APC)
APC)
 ar (
ar ( RQC) =
RQC) =  ar (
ar ( ABC) –
ABC) – 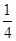 ar (
ar ( APC)
APC)
 ar (
ar ( RQC) =
RQC) =  ar (
ar ( ABC) –
ABC) – 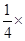
 ar (
ar ( ABC) [PC is median of
ABC) [PC is median of  ABC]
ABC]
 ar (
ar ( RQC) =
RQC) =  ar (
ar ( ABC) –
ABC) – 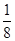 ar (
ar ( ABC)
ABC)
 ar (
ar ( RQC) =
RQC) = 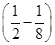
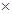 ar (
ar ( ABC)
ABC)
 ar (
ar ( RQC) =
RQC) = 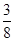 ar (
ar ( ABC)
ABC)
(iii) ar ( PRQ) =
PRQ) =  ar (
ar ( ARC) [Using result (i)]
ARC) [Using result (i)]  2 ar (
2 ar ( PRQ) = ar (
PRQ) = ar ( ARC) ..(xii)
ARC) ..(xii)
ar ( PRQ) =
PRQ) =  ar (
ar ( APQ) [RQ is the median of
APQ) [RQ is the median of  APQ] ……….(xiii)
APQ] ……….(xiii)
But ar ( APQ) = ar (
APQ) = ar ( PQC) [Using reason of eq. (vi)] ……….(xiv)
PQC) [Using reason of eq. (vi)] ……….(xiv)
From eq. (xiii) and (xiv), we get,
ar ( PRQ) =
PRQ) =  ar (
ar ( PQC) ……….(xv)
PQC) ……….(xv)
But ar ( BPQ) = ar (
BPQ) = ar ( PQC) [PQ is the median of
PQC) [PQ is the median of  BPC] ……….(xvi)
BPC] ……….(xvi)
From eq. (xv) and (xvi), we get,
ar ( PRQ) =
PRQ) =  ar (
ar ( BPQ) ……….(xvii)
BPQ) ……….(xvii)
Now from (xii) and (xvii), we get,
2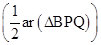 = ar (
= ar ( ARC)
ARC)  ar (
ar ( BPQ) = ar (
BPQ) = ar ( ARC)
ARC)
NCERT Solutions for Class 9 Maths Exercise 9.4
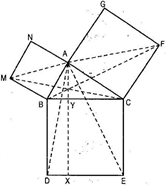
8. In figure, ABC is a right triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB respectively. Line segment AX DE meets BC at Y. Show that:
DE meets BC at Y. Show that:
(i)  MBC
MBC  ABD
ABD
(ii) ar (BYXD) = 2 ar (MBC)
(iii) ar (BYXD) = ar (ABMN)
(iv)  FCB
FCB  ACE
ACE
(v) ar (CYXE) = 2 ar (FCB)
(vi) ar (CYXE) = ar (ACFG)
(vii) ar (BCED) = ar (ABMN) + ar (ACFG)
Ans. (i)  ABM =
ABM =  CBD =
CBD = 
Adding  ABC both sides, we get,
ABC both sides, we get,
 ABM +
ABM +  ABC =
ABC =  CBD +
CBD +  ABC
ABC 
 MBC = ABD ……….(i)
MBC = ABD ……….(i)
Now in  MBC and
MBC and  ABD,
ABD,
MB = AB [equal sides of square ABMN]
BC = BD [sides of square BCED]
 MBC =
MBC =  ABD [proved above]
ABD [proved above]

 MBC
MBC  ABD [By SAS congruency]
ABD [By SAS congruency]
(ii) From above,  MBC
MBC  ABD
ABD
 ar (
ar ( MBC) = ar (
MBC) = ar ( ABD)
ABD)  ar (
ar ( MBC) = ar (trap. ABDX) – ar (
MBC) = ar (trap. ABDX) – ar ( ADX)
ADX)
 ar (
ar ( MBC) =
MBC) =  (BD + AX) BY –
(BD + AX) BY –  DX.AX
DX.AX
 ar (
ar ( MBC) =
MBC) =  BD.BY +
BD.BY +  AX.BY –
AX.BY –  DX.AX
DX.AX
 ar (
ar ( MBC) =
MBC) =  BD.BY +
BD.BY +  AX (BY – DX)
AX (BY – DX)
 ar (
ar ( MBC) =
MBC) =  BD.BY +
BD.BY +  AX. 0 [BY = DX]
AX. 0 [BY = DX]
 ar (
ar ( MBC) =
MBC) =  BD.BY
BD.BY
 2 ar (
2 ar ( MBC) = BD.BY
MBC) = BD.BY  2 ar (
2 ar ( MBC) = ar (rect. BYXD)
MBC) = ar (rect. BYXD)
Hence ar (BYXD) = 2 ar ( MBC)
MBC)
(iii) Join AM. ABMN is a square.
Therefore, NA  MB
MB  AC
AC  MB
MB
Now  AMB and
AMB and  MBC are on the same base and between the same parallels MB and AC.
MBC are on the same base and between the same parallels MB and AC.
 ar (
ar ( AMB) = ar (
AMB) = ar ( MBC) ……….(ii)
MBC) ……….(ii)
From result (ii), we have ar (BYXD) = 2 ar ( MBC) ……….(iii)
MBC) ……….(iii)
Using eq. (ii) and (iii), we get, ar (BYXD) = 2 ar ( AMB)
AMB)
 ar (BYXD) = ar (square ABMN)
ar (BYXD) = ar (square ABMN)
[Diagonal AM of square ABMN divides it in two triangles of equal area]
(iv) In  FCB and
FCB and  ACE,
ACE,
FC = AC [sides of square ACFG]
BC = CE [sides of square BCED]
 BCF =
BCF =  ACE [
ACE [
 ACF =
ACF =  BCE =
BCE =  ]
]
Adding  ACB both sides,
ACB both sides,
 BCF +
BCF +  ACB =
ACB =  ACE +
ACE +  ACB
ACB 
 BCF =
BCF =  ACE
ACE

 FCB
FCB  ACE [By SAS congruency]
ACE [By SAS congruency]
(v) From (iv), we have,  FCB
FCB  ACE
ACE
 ar (
ar ( FCB) = ar (
FCB) = ar ( ACE)
ACE)  ar (
ar ( FCB) = ar (trap. ACEX) – ar (
FCB) = ar (trap. ACEX) – ar ( AEX)
AEX)
 ar (
ar ( FCB) =
FCB) =  (CE + AX) CY –
(CE + AX) CY –  XE.AX
XE.AX
 ar (
ar ( FCB) =
FCB) =  CE.CY +
CE.CY +  AX.CY –
AX.CY –  XE.AX
XE.AX
 ar (
ar ( FCB) =
FCB) =  CE.CY +
CE.CY +  AX (CY – XE)
AX (CY – XE)
 ar (
ar ( FCB) =
FCB) =  CE.CY +
CE.CY +  AX. 0 [CY = XE]
AX. 0 [CY = XE]
 ar (
ar ( FCB) =
FCB) =  CE.CY
CE.CY
 2 ar (
2 ar ( FCB) = CE.CY
FCB) = CE.CY  2 ar (
2 ar ( FCB) = ar (rect. CYXE)
FCB) = ar (rect. CYXE)
Hence ar (BYXD) = 2 ar ( FCB)
FCB)
NCERT Solutions for Class 9 Maths Exercise 9.4
(vi) Join AF. ACFG is a square.
 FC
FC  AG
AG  FC
FC  AB
AB
Now  ACF and
ACF and  FCB are on the same base FC and between the same parallels FC and AB.
FCB are on the same base FC and between the same parallels FC and AB.
 ar (
ar ( ACF) = ar (
ACF) = ar ( FCB) ……….(v)
FCB) ……….(v)
From result (v), we get, ar (CYXE) = 2 ar ( FCB) ……….(vi)
FCB) ……….(vi)
Using eq. (v) in (vi), we get, ar (CYXE) = 2 ar ( ACF)
ACF)
Diagonal AF of square ACFG divides it in two triangles of equal area.
 ar (CYXE) = ar (sq. ACFG) ……….(vii)
ar (CYXE) = ar (sq. ACFG) ……….(vii)
(vii) Adding eq. (iv) and (vii), we get,
ar (BYXD) + ar (CYXE) = ar (ABMN) + ar (ACFG)
 ar (BCED) = ar (ABMN) + ar (ACFG)
ar (BCED) = ar (ABMN) + ar (ACFG)
NCERT Solutions for Class 9 Maths Exercise 9.4
NCERT Solutions for Class 9 Maths Exercise 9.4 PDF (Download) Free from myCBSEguide app and myCBSEguide website. Ncert solution class 9 Maths includes text book solutions from Mathematics Book. NCERT Solutions for CBSE Class 9 Maths have total 15 chapters. 9 Maths NCERT Solutions in PDF for free Download on our website. Ncert Maths class 9 solutions PDF and Maths ncert class 9 PDF solutions with latest modifications and as per the latest CBSE syllabus are only available in myCBSEguide.
CBSE app for Class 9
To download NCERT Solutions for Class 9 Maths, Computer Science, Home Science,Hindi ,English, Social Science do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT solutions, NCERT Exemplar solutions, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.

Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
Learn8 App
Practice unlimited questions for Entrance tests & government job exams at ₹99 only
Install Now