NCERT Solutions for Class 9 Maths Exercise 9.3 book solutions are available in PDF format for free download. These ncert book chapter wise questions and answers are very helpful for CBSE board exam. CBSE recommends NCERT books and most of the questions in CBSE exam are asked from NCERT text books. Class 9 Maths chapter wise NCERT solution for Maths Book for all the chapters can be downloaded from our website and myCBSEguide mobile app for free.
NCERT solutions for Class 9 Maths Areas of Parallelograms and Triangles Download as PDF

NCERT Solutions for Class 9 Mathematics Areas of Parallelograms and Triangles
1. In figure, E is any point on median AD of a  ABC. Show that ar (
ABC. Show that ar ( ABE) = ar (
ABE) = ar ( ACE).
ACE).
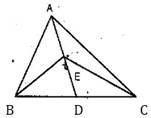
Ans. In  ABC, AD is a median.
ABC, AD is a median.
ar ( ABD) = ar (
ABD) = ar ( ACD) ……….(i)
ACD) ……….(i)
[ Median divides a
Median divides a  into two
into two  s of equal area]
s of equal area]
Again in  EBC, ED is a median
EBC, ED is a median
ar ( EBD) = ar (
EBD) = ar ( ECD) ……….(ii)
ECD) ……….(ii)
Subtracting eq. (ii) from (i),
ar ( ABD) – ar (
ABD) – ar ( EBD) = ar (
EBD) = ar ( ACD) – ar (
ACD) – ar ( ECD)
ECD)
 ar (
ar ( ABE) = ar (
ABE) = ar ( ACE)
ACE)
2. In a triangle ABC, E is the mid-point of median AD. Show that ar (BED) = 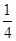 ar (ABC).
ar (ABC).
Ans. Given: A  ABC, AD is the median and E is the mid-point of median AD.
ABC, AD is the median and E is the mid-point of median AD.
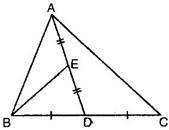
To prove: ar ( BED) =
BED) = 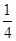 ar (
ar ( ABC)
ABC)
Proof: In  ABC, AD is the median.
ABC, AD is the median.
 ar (
ar ( ABD) = ar (
ABD) = ar ( ADC)
ADC)
[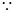 Median divides a
Median divides a  into two
into two  s of equal area]
s of equal area]
 ar (
ar ( ABD) =
ABD) = 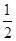 ar (ABC) ……….(i)
ar (ABC) ……….(i)
In  ABD, BE is the median.
ABD, BE is the median.
 ar (
ar ( BED) = ar (
BED) = ar ( BAE)
BAE)
 ar (
ar ( BED) =
BED) = 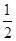 ar (
ar ( ABD)
ABD)
 ar (
ar ( BED) =
BED) =  ar (
ar ( ABC) =
ABC) = 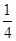 ar (
ar ( ABC)
ABC)
3. Show that the diagonals of a parallelogram divide it into four triangles of equal area.
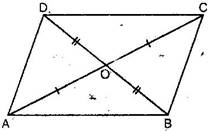
Ans. Let parallelogram be ABCD and its diagonals AC and BD intersect each other at O.
In  ABC and
ABC and  ADC,
ADC,
AB = DC [Opposite sides of a parallelogram]
BC = AD [Opposite sides of a parallelogram]
And AC = AC [Common]

 ABC
ABC 
 CDA [By SSS congruency]
CDA [By SSS congruency]
Since, diagonals of a parallelogram bisect each other.
 O is the mid-point of bisection.
O is the mid-point of bisection.
Now in  ADC, DO is the median.
ADC, DO is the median.
 ar (
ar ( AOD) = ar (
AOD) = ar ( COD) ……….(i)
COD) ……….(i)
[Median divides a triangle into two equal areas]
Similarly, in  ABC, OB is the median.
ABC, OB is the median.
 ar (
ar ( AOB) = ar (
AOB) = ar ( BOC) ……….(ii)
BOC) ……….(ii)
And in  AOB and
AOB and  AOD, AO is the median.
AOD, AO is the median.
 ar (
ar ( AOB) = ar (
AOB) = ar ( AOD) ……….(iii)
AOD) ……….(iii)
From eq. (i), (ii) and (iii),
ar ( AOB) = ar (
AOB) = ar ( AOD) = ar (
AOD) = ar ( BOC) = ar (
BOC) = ar ( COD)
COD)
Thus diagonals of parallelogram divide it into four triangles of equal area.
NCERT Solutions for Class 9 Maths Exercise 9.3
4. In figure, ABC and ABD are two triangles on the same base AB. If line-segment CD is bisected by AB at O, show that ar (ABC) = ar (ABD).
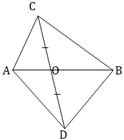
Ans. Draw CM AB and DN
AB and DN AB.
AB.
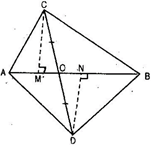
In  CMO and
CMO and  DNO,
DNO,
 CMO =
CMO = 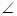 DNO =
DNO = 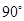 [By construction]
[By construction]
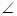 COM =
COM = 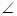 DON [Vertically opposite]
DON [Vertically opposite]
OC = OD [Given]

 CMO
CMO 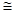
 DNO [By ASA congruency]
DNO [By ASA congruency]
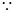 AM = DN [By CPCT] ……(i)
AM = DN [By CPCT] ……(i)
Now ar ( ABC) =
ABC) = 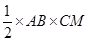 ……….(ii)
……….(ii)
ar ( ADB) =
ADB) = 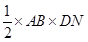 ……….(iii)
……….(iii)
Using eq. (i) and (iii),
ar ( ADB) =
ADB) = 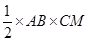 ……….(iv)
……….(iv)
From eq. (ii) and (iv),
ar ( ABC) = ar (
ABC) = ar ( ADB)
ADB)
NCERT Solutions for Class 9 Maths Exercise 9.3
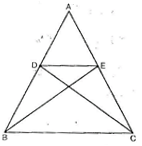
5. D, E and F are respectively the mid-points of the sides BC, CA and AB of a  ABC. Show that:
ABC. Show that:
(i) BDEF is a parallelogram.
(ii) ar (DEF) = 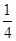 ar (ABC)
ar (ABC)
(iii) ar (BDEF) = 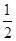 ar (ABC)
ar (ABC)
Ans. (i) F is the mid-point of AB and E is the mid-point of AC.
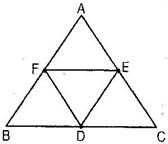
 FE
FE BC and FE =
BC and FE = 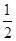 BD
BD
[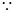 Line joining the mid-points of two sides of a triangle is parallel to the third and half of it]
Line joining the mid-points of two sides of a triangle is parallel to the third and half of it]
 FE
FE BD [BD is the part of BC]
BD [BD is the part of BC]
And FE = BD
Also, D is the mid-point of BC.
 BD =
BD = 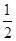 BC
BC
And FE BC and FE = BD
BC and FE = BD
Again E is the mid-point of AC and D is the mid-point of BC.
 DE
DE AB and DE =
AB and DE = 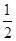 AB
AB
 DE
DE AB [BF is the part of AB]
AB [BF is the part of AB]
And DE = BF
Again F is the mid-point of AB.
 BF =
BF = 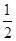 AB
AB
But DE = 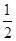 AB
AB
 DE = BF
DE = BF
Now we have FE BD and DE
BD and DE BF
BF
And FE = BD and DE = BF
Therefore, BDEF is a parallelogram.
(ii) BDEF is a parallelogram.
 ar (
ar ( BDF) = ar (
BDF) = ar ( DEF) ……….(i)
DEF) ……….(i)
[diagonals of parallelogram divides it in two triangles of equal area]
DCEF is also parallelogram.
 ar (
ar ( DEF) = ar (
DEF) = ar ( DEC) ……….(ii)
DEC) ……….(ii)
Also, AEDF is also parallelogram.
 ar (
ar ( AFE) = ar (
AFE) = ar ( DEF) ……….(iii)
DEF) ……….(iii)
From eq. (i), (ii) and (iii),
ar ( DEF) = ar (
DEF) = ar ( BDF) = ar (
BDF) = ar ( DEC) = ar (
DEC) = ar ( AFE) ……….(iv)
AFE) ……….(iv)
Now, ar ( ABC) = ar (
ABC) = ar ( DEF) + ar (
DEF) + ar ( BDF) + ar (
BDF) + ar ( DEC) + ar (
DEC) + ar ( AFE) ……….(v)
AFE) ……….(v)
 ar (
ar ( ABC) = ar (
ABC) = ar ( DEF) + ar (
DEF) + ar ( DEF) + ar (
DEF) + ar ( DEF) + ar (
DEF) + ar ( DEF)
DEF)
[Using (iv) & (v)]
 ar (
ar ( ABC) = 4
ABC) = 4 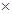 ar (
ar ( DEF)
DEF)
 ar (
ar ( DEF) =
DEF) = 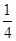 ar (
ar ( ABC)
ABC)
(iii) ar ( gm BDEF) = ar (
gm BDEF) = ar ( BDF) + ar (
BDF) + ar ( DEF) = ar (
DEF) = ar ( DEF) + ar (
DEF) + ar ( DEF) [Using (iv)]
DEF) [Using (iv)]
 ar (
ar ( gm BDEF) = 2 ar (
gm BDEF) = 2 ar ( DEF)
DEF)
 ar (
ar ( gm BDEF) =
gm BDEF) = 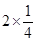 ar (
ar ( ABC)
ABC)
 ar (
ar ( gm BDEF) =
gm BDEF) = 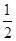 ar (
ar ( ABC)
ABC)
NCERT Solutions for Class 9 Maths Exercise 9.3
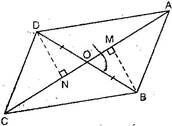
6. In figure, diagonals AC and BD of quadrilateral ABCD intersect at O such that OB = OD. If AB = CD, then show that:

(i) ar (DOC) = ar (AOB)
(ii) ar (DCB) = ar (ACB)
(iii) DA CB or ABCD is a parallelogram.
CB or ABCD is a parallelogram.
Ans. (i) Draw BM  AC and DN
AC and DN  AC.
AC.
In  DON and
DON and  BOM,
BOM,
OD = OB [Given]
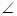 DNO =
DNO = 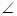 BMO =
BMO = 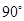 [By construction]
[By construction]
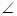 DON =
DON = 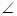 BOM [Vertically opposite]
BOM [Vertically opposite]

 DON
DON 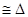 BOM [By RHS congruency]
BOM [By RHS congruency]
 DN = BM [By CPCT]
DN = BM [By CPCT]
Also ar ( DON) = ar (
DON) = ar ( BOM) ……….(i)
BOM) ……….(i)
Again, In  DCN and
DCN and  ABM,
ABM,
CD = AB [Given]
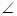 DNC =
DNC = 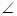 BMA =
BMA =  [By construction]
[By construction]
DN = BM [Prove above]

 DCN
DCN  BAM [By RHS congruency]
BAM [By RHS congruency]
 ar (
ar ( DCN) = ar (
DCN) = ar ( BAM) ……….(ii)
BAM) ……….(ii)
Adding eq. (i) and (ii),
ar ( DON) + ar (
DON) + ar ( DCN) = ar (
DCN) = ar ( BOM) + ar (
BOM) + ar ( BAM)
BAM)
 ar (
ar ( DOC) = ar (
DOC) = ar ( AOB)
AOB)
(ii) Since ar ( DOC) = ar (
DOC) = ar ( AOB)
AOB)
Adding ar  BOC both sides,
BOC both sides,
ar ( DOC) + ar
DOC) + ar  BOC = ar (
BOC = ar ( AOB) + ar
AOB) + ar  BOC
BOC
 ar (
ar ( DCB) = ar (
DCB) = ar ( ACB)
ACB)
(iii) Since ar ( DCB) = ar (
DCB) = ar ( ACB)
ACB)
Therefore, these two triangles in addition to be on the same base CB lie between two same parallels CB and DA.
 DA
DA CB
CB
Now AB = CD and DA CB
CB
Therefore, ABCD is a parallelogram.
NCERT Solutions for Class 9 Maths Exercise 9.3
7. D and E are points on sides AB and AC respectively of  ABC such that ar (DBC) = ar (EBC). Prove that DE
ABC such that ar (DBC) = ar (EBC). Prove that DE BC.
BC.
Ans. Given: ar ( DBC) = ar (
DBC) = ar ( EBC)
EBC)
Since two triangles of equal area have common base BC.
Therefore, DE BC [
BC [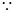 Two triangles having same base (or equal bases) and equal areas lie between the same parallel]
Two triangles having same base (or equal bases) and equal areas lie between the same parallel]
NCERT Solutions for Class 9 Maths Exercise 9.3
8. XY is a line parallel to side BC of triangle ABC. If BE AC and CF
AC and CF AB meet XY at E and F respectively, show that ar (ABE) = ar (ACF).
AB meet XY at E and F respectively, show that ar (ABE) = ar (ACF).
Ans.  ABE and parallelogram BCYE lie on the same base BE and between the same parallels BE and AC.
ABE and parallelogram BCYE lie on the same base BE and between the same parallels BE and AC.
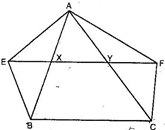
 ar (
ar ( ABE) =
ABE) = 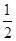 ar (
ar ( gm BCYE) ……….(i)
gm BCYE) ……….(i)
Also  ACF and
ACF and  gm BCFX lie on the same base CF and between same parallel BX and CF.
gm BCFX lie on the same base CF and between same parallel BX and CF.
 ar (
ar ( ACF) =
ACF) = 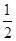 ar (
ar ( gm BCFX) ……….(ii)
gm BCFX) ……….(ii)
But  gm BCYE and
gm BCYE and  gm BCFX lie on the same base BC and between the same parallels BC and EF.
gm BCFX lie on the same base BC and between the same parallels BC and EF.
 ar (
ar ( gm BCYE) = ar (
gm BCYE) = ar ( gm BCFX) ……….(iii)
gm BCFX) ……….(iii)
From eq. (i), (ii) and (iii), we get,
ar ( ABE) = ar (
ABE) = ar ( ACF)
ACF)
9. The side AB of parallelogram ABCD is produced to any point P. A line through A and parallel to CP meets CB produced at Q and then parallelogram PBQR is completed. Show that ar (ABCD) = ar (PBQR).
Ans. Given: ABCD is a parallelogram, CP AQ and PBQR is a parallelogram.
AQ and PBQR is a parallelogram.
To prove: ar (ABCD) = ar (PBQR)
Construction: Join AC and QP.
Proof: Since AQ CP
CP
 ar (
ar ( AQC) = ar (
AQC) = ar ( AQP)
AQP)
[Triangles on the same base and between the same parallels are equal in area]
Subtracting ar ( ABQ) from both sides, we get
ABQ) from both sides, we get
ar ( AQC – ar (
AQC – ar ( ABQ) = ar (
ABQ) = ar ( AQP) – ar (
AQP) – ar ( ABQ)
ABQ)
 ar (
ar ( ABC) = ar (
ABC) = ar ( QBP) ……….(i)
QBP) ……….(i)
Now ar ( ABC) =
ABC) = 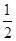 ar (
ar ( gm ABCD)
gm ABCD)
[Diagonal divides a parallelogram in two parts of equal area]
And ar ( PQB) =
PQB) = 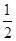 ar (
ar ( gm PBQR)
gm PBQR)
From eq. (i), (ii) and (iii), we get
ar ( gm ABCD) = ar (
gm ABCD) = ar ( gm PBQR)
gm PBQR)
NCERT Solutions for Class 9 Maths Exercise 9.3
10. Diagonals AC and BD of a trapezium ABCD with AB DC intersect each other at O. Prove that ar(AOD) = ar (BOC).
DC intersect each other at O. Prove that ar(AOD) = ar (BOC).
Ans.  ABD and
ABD and  ABC lie on the same base AB and between the same parallels AB and DC.
ABC lie on the same base AB and between the same parallels AB and DC.
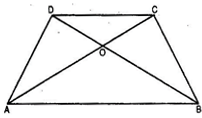
 ar (
ar ( ABD) = ar (
ABD) = ar ( ABC)
ABC)
Subtracting ar ( AOB) from both sides,
AOB) from both sides,
ar ( ABD) – ar (
ABD) – ar ( AOB)
AOB)
= ar ( ABC) – ar (
ABC) – ar ( AOB)
AOB)
 ar (
ar ( AOD) = ar (
AOD) = ar ( BOC)
BOC)
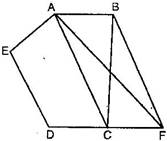
11. In figure, ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that:
(i) ar (ACB = ar (ACF)
(ii) ar (AEDF) = ar (ABCDE)
Ans. (i) Given that BF AC
AC
 ACB and
ACB and  ACF lie on the same base AC and between the same parallels AC and BF.
ACF lie on the same base AC and between the same parallels AC and BF.
 ar (
ar ( ACB) = ar (
ACB) = ar ( ACF) ……….(i)
ACF) ……….(i)
(ii) Now ar (ABCDE) = ar (trap. AEDC) + ar ( ABC) ……….(ii)
ABC) ……….(ii)
 ar (ABCDE) = ar (trap. AEDC) + ar (
ar (ABCDE) = ar (trap. AEDC) + ar ( ACF) = ar (quad. AEDF) [Using (i)]
ACF) = ar (quad. AEDF) [Using (i)]
 ar (AEDF) = ar (ABCDE)
ar (AEDF) = ar (ABCDE)
NCERT Solutions for Class 9 Maths Exercise 9.3
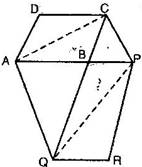
12. A villager Itwaari has a plot of land of the shape of quadrilateral. The Gram Panchyat of two villages decided to take over some portion of his plot from one of the corners to construct a health centre. Itwaari agrees to the above personal with the condition that he should be given equal amount of land in lieu of his land adjoining his plot so as to form a triangular plot. Explain how this proposal will be implemented.
Ans. Let Itwari has land in shape of quadrilateral PQRS.
Draw a line through 5 parallel to PR, which meets QR produced at M.
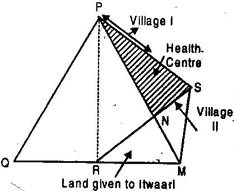
Let diagonals PM and RS of new formed quadrilateral intersect each other at point N.
We have PR SM [By construction]
SM [By construction]
 ar (
ar ( PRS) = ar (
PRS) = ar ( PMR)
PMR)
[Triangles on the same base and same parallel are equal in area]
Subtracting ar ( PNR) from both sides,
PNR) from both sides,
ar ( PRS) – ar (
PRS) – ar ( PNR) = ar (
PNR) = ar ( PMR) – ar (
PMR) – ar ( PNR)
PNR)
 ar (
ar ( PSN) = ar (
PSN) = ar ( MNR)
MNR)
It implies that Itwari will give corner triangular shaped plot PSN to the Grampanchayat for health centre and will take equal amount of land (denoted by  MNR) adjoining his plot so as to form a triangular plot PQM.
MNR) adjoining his plot so as to form a triangular plot PQM.
NCERT Solutions for Class 9 Maths Exercise 9.3
13. ABCD is a trapezium with AB DC. A line parallel to AC intersects AB at X and BC at Y. Prove that ar (ADX) = ar (ACY).
DC. A line parallel to AC intersects AB at X and BC at Y. Prove that ar (ADX) = ar (ACY).
Ans. Join CX,  ADX and ACX lie on the same base XA and between the same parallels XA and DC.
ADX and ACX lie on the same base XA and between the same parallels XA and DC.
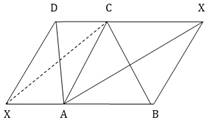
 ar (
ar ( ADX) = ar (
ADX) = ar ( ACX) ……….(i)
ACX) ……….(i)
Also  ACX and
ACX and  ACY lie on the same base
ACY lie on the same base
AC and between same parallels CY and XA.
 ar (
ar ( ACX) = ar (
ACX) = ar ( ACY) ……….(ii)
ACY) ……….(ii)
From (i) and (ii),
ar ( ADX) = ar (
ADX) = ar ( ACY)
ACY)
NCERT Solutions for Class 9 Maths Exercise 9.3
14. In figure, AP BQ
BQ CR. Prove that ar (AQC) = ar (PBR).
CR. Prove that ar (AQC) = ar (PBR).
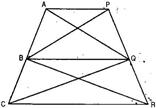
Ans.  ABQ and BPQ lie on the same base BQ and between same parallels AP and BQ.
ABQ and BPQ lie on the same base BQ and between same parallels AP and BQ.
 ar (
ar ( ABQ) = ar (
ABQ) = ar ( BPQ) ……….(i)
BPQ) ……….(i)
 BQC and
BQC and  BQR lie on the same base BQ and between same parallels BQ and CR.
BQR lie on the same base BQ and between same parallels BQ and CR.
 ar (
ar ( BQC) = ar (
BQC) = ar ( BQR) ……….(ii)
BQR) ……….(ii)
Adding eq (i) and (ii), ar ( ABQ) + ar (
ABQ) + ar ( BQC) = ar (
BQC) = ar ( BPQ) + ar (
BPQ) + ar ( BQR)
BQR)
 ar (
ar ( AQC) = ar (
AQC) = ar ( PBR)
PBR)
NCERT Solutions for Class 9 Maths Exercise 9.3
15. Diagonals AC and BD of a quadrilateral ABCD intersect at O in such a way that ar (AOD) = ar(BOC). Prove that ABCD is a trapezium.
Ans. Given that ar ( AOD) = ar (
AOD) = ar ( BOC)
BOC)
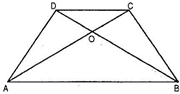
Adding  AOB both sides,
AOB both sides,
ar ( AOD) + ar (
AOD) + ar ( AOB) = ar (
AOB) = ar ( BOC) + ar (
BOC) + ar ( AOB)
AOB)
 ar (
ar ( ABD) = ar (
ABD) = ar ( ABC)
ABC)
Since if two triangles equal in area, lie on the same base then, they lie between same parallels. We have  ABD and
ABD and  ABC lie on common base AB and are equal in area.
ABC lie on common base AB and are equal in area.
 They lie in same parallels AB and DC.
They lie in same parallels AB and DC.
 AB
AB DC
DC
Now in quadrilateral ABCD, we have AB DC
DC
Therefore, ABCD is trapezium. [ In trapezium one pair of opposite sides is parallel]
In trapezium one pair of opposite sides is parallel]
NCERT Solutions for Class 9 Maths Exercise 9.3
16. In figure, ar (DRC) = ar (DPC) and ar (BDP) = ar (ARC). Show that both the quadrilaterals ABCD and DCPR are trapeziums.
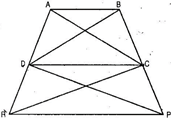
Ans. Given that  DRC and
DRC and  DPC lie on the same base DC and ar (
DPC lie on the same base DC and ar ( DPC) = ar (
DPC) = ar ( DRC) …..(i)
DRC) …..(i)
 DC
DC RP
RP
[If two triangles equal in area, lie on the same base then, they lie between same parallels]
Therefore, DCPR is trapezium. [ In trapezium one pair of opposite sides is parallel]
In trapezium one pair of opposite sides is parallel]
Also ar ( BDP) = ar (
BDP) = ar ( ARC) ……….(ii)
ARC) ……….(ii)
Subtracting eq. (i) from (ii),
ar ( BDP) – ar (
BDP) – ar ( DPC) = ar (
DPC) = ar ( ARC) – ar (
ARC) – ar ( DRC)
DRC)
 ar (
ar ( BDC) = ar (
BDC) = ar ( ADC)
ADC)
Therefore, AB DC [If two triangles equal in area, lie on the same base then, they lie between same parallels]
DC [If two triangles equal in area, lie on the same base then, they lie between same parallels]
Therefore, ABCD is trapezium.
NCERT Solutions for Class 9 Maths Exercise 9.3
NCERT Solutions for Class 9 Maths PDF (Download) Free from myCBSEguide app and myCBSEguide website. Ncert solution class 9 Maths includes text book solutions from Mathematics Book. NCERT Solutions for CBSE Class 9 Maths have total 15 chapters. 9 Maths NCERT Solutions in PDF for free Download on our website. Ncert Maths class 9 solutions PDF and Maths ncert class 9 PDF solutions with latest modifications and as per the latest CBSE syllabus are only available in myCBSEguide.
CBSE app for Class 9
To download NCERT Solutions for Class 9 Maths, Computer Science, Home Science,Hindi ,English, Social Science do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT solutions, NCERT Exemplar solutions, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.

Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
Learn8 App
Practice unlimited questions for Entrance tests & government job exams at ₹99 only
Install Now