NCERT Solutions for Class 9 Maths Exercise 9.2 book solutions are available in PDF format for free download. These ncert book chapter wise questions and answers are very helpful for CBSE board exam. CBSE recommends NCERT books and most of the questions in CBSE exam are asked from NCERT text books. Class 9 Maths chapter wise NCERT solution for Maths Book for all the chapters can be downloaded from our website and myCBSEguide mobile app for free.
NCERT solutions for Class 9 Maths Areas of Parallelograms and Triangles Download as PDF

NCERT Solutions for Class 9 Mathematics Areas of Parallelograms and Triangles
1. In figure, ABCD is a parallelogram. AE  DC and CF
DC and CF  AD. If AB = 16 cm, AE = 8 cm and CF = 10 cm, find AD.
AD. If AB = 16 cm, AE = 8 cm and CF = 10 cm, find AD.
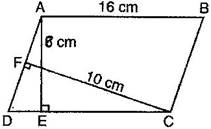
Ans. ABCD is a parallelogram.
 DC = AB
DC = AB  DC = 16 cm
DC = 16 cm
AE 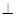 DC [Given]
DC [Given]
Now Area of parallelogram ABCD = Base x Corresponding height

Using base AD and height CF, we can find,
Area of parallelogram 
 128
128 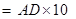
 AD =
AD = 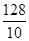 = 12.8 cm
= 12.8 cm
2. If E, F, G and H are respectively the mid-points of the sides of a parallelogram ABCD, show that ar (EFGH) =  ar (ABCD).
ar (ABCD).
Ans. Given: A parallelogram ABCD. E, F, G and H are mid-points of AB, BC, CD and DA respectively.
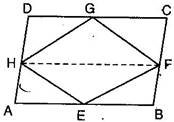
To prove: ar (EFGH) =  ar (ABCD)
ar (ABCD)
Construction: Join HF
Proof: ar ( GHF) =
GHF) =  ar (
ar ( gm HFCD) ……….(i)
gm HFCD) ……….(i)
And ar ( HEF) =
HEF) =  ar (
ar ( gm HABF) ……….(ii)
gm HABF) ……….(ii)
[If a triangle and a parallelogram are on the same base and between the same parallel then the area of triangle is half of area of parallelogram]
Adding eq. (i) and (ii),
ar ( GHF) + ar (
GHF) + ar ( HEF)
HEF)
=  ar (
ar ( gm HFCD) +
gm HFCD) +  ar (
ar ( gm HABF)
gm HABF)
 ar (
ar ( gm HEFG) =
gm HEFG) =  ar (
ar ( gm ABCD)
gm ABCD)
NCERT Solutions for Class 9 Maths Exercise 9.2
3. P and Q are any two points lying on the sides DC and AD respectively of a parallelogram ABCD. Show that ar (APB) = ar (BQC).
Ans. Given: ABCD is a parallelogram. P is a point on DC and Q is a point on AD.
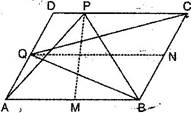
To prove: ar ( APB) = ar (
APB) = ar ( BQC)
BQC)
Construction: Draw PM  BC and QN
BC and QN  DC.
DC.
Proof: Since QC is the diagonal of parallelogram QNCD.
 ar (
ar ( QNC) =
QNC) =  ar (
ar ( gm QNCD) ……….(i)
gm QNCD) ……….(i)
Again BQ is the diagonal of parallelogram ABNQ.
 ar (
ar ( BQN) =
BQN) =  ar (
ar ( gm ABNQ) ……….(ii)
gm ABNQ) ……….(ii)
Adding eq. (i) and (ii),
ar ( QNC) + ar (
QNC) + ar ( BQN)
BQN)
=  ar (
ar ( gm QNCD) +
gm QNCD) +  ar (
ar ( gm ABNQ)
gm ABNQ)
 ar (
ar ( BQC) =
BQC) =  ar (
ar ( gm ABCD) ………..(iii)
gm ABCD) ………..(iii)
Again AP is the diagonal of  gm AMPD.
gm AMPD.
 ar (
ar ( APM) =
APM) =  ar (
ar ( gm AMPD) ……….(iv)
gm AMPD) ……….(iv)
And PB is the diagonal of  gm PCBM.
gm PCBM.
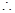 ar (
ar ( PBM) =
PBM) =  ar (
ar ( gm PCBM) ……….(v)
gm PCBM) ……….(v)
Adding eq. (iv) and (v),
ar ( APM) + ar (
APM) + ar ( PBM)
PBM)
=  ar (
ar ( gm AMPD) +
gm AMPD) +  ar (
ar ( gm PCBM)
gm PCBM)
 ar (
ar ( APB) =
APB) =  ar (
ar ( gm ABCD) ……….(vi)
gm ABCD) ……….(vi)
From eq. (iii) and (vi),
ar ( BQC) = ar (
BQC) = ar ( APB) or ar (
APB) or ar ( APB) = ar (
APB) = ar ( BQC)
BQC)
NCERT Solutions for Class 9 Maths Exercise 9.2
4. In figure, P is a point in the interior of a parallelogram ABCD.
Show that:
(i) ar (APB) + ar (PCD) =  ar (ABCD)
ar (ABCD)
(ii) ar (APD) + ar (PBC) = ar (APB) + ar (PCD)
Ans. (i) Draw a line passing through point P and parallel to AB which intersects AD at Q and BC at R respectively.
Now  APB and parallelogram ABRQ are on the same base AB and between same parallels AB and QR.
APB and parallelogram ABRQ are on the same base AB and between same parallels AB and QR.
 ar (
ar ( APB) =
APB) =  ar (
ar ( gm ABRQ) ……….(i)
gm ABRQ) ……….(i)
Also  PCD and parallelogram DCRQ are on the same base AB and between same parallels AB and QR.
PCD and parallelogram DCRQ are on the same base AB and between same parallels AB and QR.
 ar (
ar ( PCD) =
PCD) =  ar (
ar ( gm DCRQ) ……….(ii)
gm DCRQ) ……….(ii)
Adding eq. (i) and (ii),
ar ( APB) + ar (
APB) + ar ( PCD)
PCD)
=  ar (
ar ( gm ABRQ) +
gm ABRQ) +  ar (
ar ( gm DCRQ)
gm DCRQ)
 ar (
ar ( APB) =
APB) =  ar (
ar ( gm ABCD) ……….(iii)
gm ABCD) ……….(iii)
(ii) Draw a line through P and parallel to AD which intersects AB at M and DC at N. Now  APD and parallelogram AMND are on the same base AD and between same parallels AD and MN.
APD and parallelogram AMND are on the same base AD and between same parallels AD and MN.
 ar (
ar ( APD) =
APD) =  ar (
ar ( gm AMND) ……….(iv)
gm AMND) ……….(iv)
Also  PBC and parallelogram MNCB are on the same base BC and between same parallels BC and MN.
PBC and parallelogram MNCB are on the same base BC and between same parallels BC and MN.
 ar (
ar ( PBC) =
PBC) =  ar (
ar ( gm MNCB) ……….(v)
gm MNCB) ……….(v)
Adding eq. (i) and (ii),
ar ( APD) + ar (
APD) + ar ( PBC)
PBC)
=  ar (
ar ( gm AMND) +
gm AMND) +  ar (
ar ( gm MNCB)
gm MNCB)
 ar (
ar ( APD) =
APD) =  ar (
ar ( gm ABCD) ……….(vi)
gm ABCD) ……….(vi)
From eq. (iii) and (vi), we get,
ar ( APB) + ar (
APB) + ar ( PCD) = ar (
PCD) = ar ( APD) + ar (
APD) + ar ( PBC)
PBC)
or ar ( APD) + ar (
APD) + ar ( PBC) = ar (
PBC) = ar ( APB) + ar (
APB) + ar ( PCD)
PCD)
Hence proved.
NCERT Solutions for Class 9 Maths Exercise 9.2
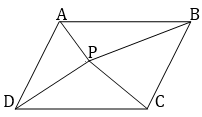
5. In figure, PQRS and ABRS are parallelograms and X is any point on the side BR. Show that:

(i) ar (PQRS) = ar (ABRS)
(ii) ar (AXS) =  ar (PQRS)
ar (PQRS)
Ans. (i) Parallelogram PQRS and ABRS are on the same base SR and between the same parallels SR and PB.
 ar (
ar ( gm PQRS) =
gm PQRS) =  ar (
ar ( gm ABRS) ……….(i)
gm ABRS) ……….(i)
[ parallelograms on the same base and between the same parallels are equal
parallelograms on the same base and between the same parallels are equal
in area]
(ii)  AXS and
AXS and  gm ABRS are on the same base AS and between the same parallels AS and BR.
gm ABRS are on the same base AS and between the same parallels AS and BR.
 ar (
ar ( AXS) =
AXS) =  ar (
ar ( gm ABRS) ……….(ii)
gm ABRS) ……….(ii)
Using eq. (i) and (ii),
ar ( AXS) =
AXS) =  ar (
ar ( gm PQRS)
gm PQRS)
NCERT Solutions for Class 9 Maths Exercise 9.2
6. a farmer was having a field in the form of a parallelogram PQRS. She took any point A on RS and joined it to points P and Q. In how many parts the field is divided? What are the shapes of these parts? The farmer wants to sow wheat and pulses in equal portions of the field separately. How should she do it?
Ans. When A is joined with P and Q; the field is divided into three parts viz.  PAS,
PAS,  APQ and
APQ and  AQR.
AQR. APQ and parallelogram PQRS are on the same base PQ and between same parallels PQ and SR.
APQ and parallelogram PQRS are on the same base PQ and between same parallels PQ and SR.
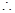 ar (
ar ( APQ) =
APQ) =  ar (
ar ( gm PQRS)
gm PQRS)
It implies that triangular region APQ covers half portion of parallelogram shaped field PQRS.
So if farmer sows wheat in triangular shaped field APQ then she will definitely sow pulses in other two triangular parts PAS and AQR.
Or
When she sows pulses in triangular shaped field APQ then she will sow wheat in other two triangular parts PAS and AQR.

NCERT Solutions for Class 9 Maths Exercise 9.2
NCERT Solutions for Class 9 Maths PDF (Download) Free from myCBSEguide app and myCBSEguide website. Ncert solution class 9 Maths includes text book solutions from Mathematics Book. NCERT Solutions for CBSE Class 9 Maths have total 15 chapters. 9 Maths NCERT Solutions in PDF for free Download on our website. Ncert Maths class 9 solutions PDF and Maths ncert class 9 PDF solutions with latest modifications and as per the latest CBSE syllabus are only available in myCBSEguide.
CBSE app for Class 9
To download NCERT Solutions for Class 9 Maths, Computer Science, Home Science,Hindi ,English, Social Science do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT solutions, NCERT Exemplar solutions, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.

Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
Learn8 App
Practice unlimited questions for Entrance tests & government job exams at ₹99 only
Install Now