NCERT Solutions class-11 Maths Exercise 9.2

Exercise 9.2

1. Find the sum of odd integers from 1 to 2001.

Ans. Odd integers from 1 to 2001 are 1, 3, 5, 7, …….., 2001.

Here,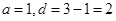 and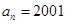

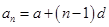
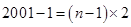
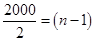
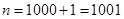
Now,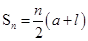

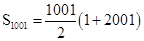
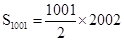= 1002001

---

2. Find the sum of all natural numbers lying between 100 and 1000 which are multiples of 5.

Ans. According to question, series is105, 110, 115, 120, ………, 995

Here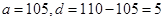 and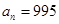

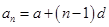
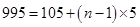
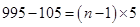
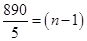
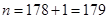
Now,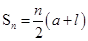

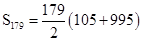
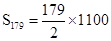= 98450

---

3. In an A.P. the first term is 2 and the sum of the first five terms is one-fourth of the next five terms. Show that 20^th term is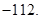

Ans. According to question,

---

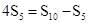
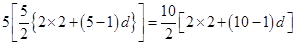
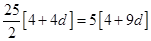
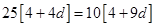
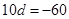
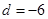
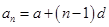
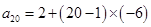
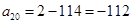
4. How many terms of the A.P., are needed to give the sum ?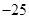

Ans. Here,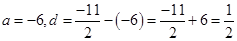

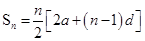
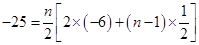
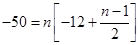
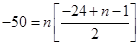
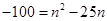
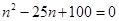
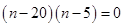
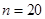or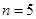

---

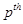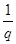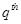5. In an A.P., if term is and term is 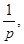prove that the sum of first 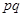terms is 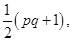where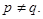

Ans. Let 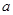be the first term and 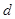be the common difference of given A.P.

And 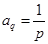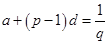and

and

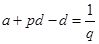…..(i) and…..(ii)

Subtracting eq. (ii) from eq. (i), we get

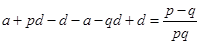
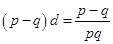
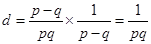
Putting value of 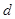in eq. (i), we get

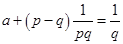
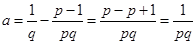
Now,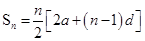

=

---

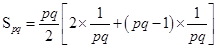
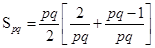
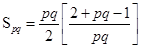
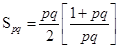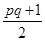
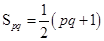
6. If the sum of a certain number of terms of the A.P. 25, 22, 19, ….. is 116, find the last term.

Ans. Here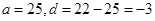 and

=

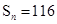
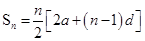
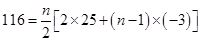
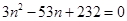
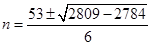
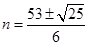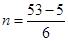
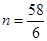or

or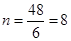

But 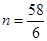is not possible. Therefore,

Now,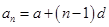

---

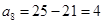
7. Find the sum of 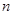terms of an A.P. whose 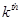term is

Ans. Given: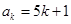

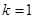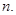
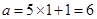Putting and

and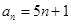

---

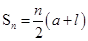
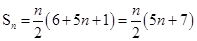
8. If the sum of 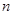terms of an A.P. is 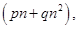where 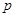and 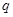are constants, find the common difference.

Ans. Given:

Replacing 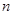by

=

=

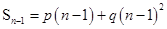
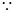
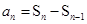
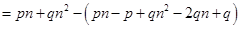
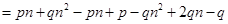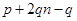
Again here replacing 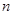by

=

=

---

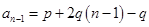
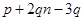
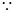
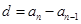
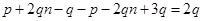
9. The sums of 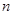terms of two arithmetic progressions are on the ratio 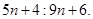Find the ratio of their 18^th terms.

Ans. Let 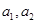and 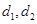be the first terms and common differences of two A.P’s respectively.

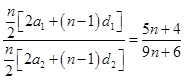
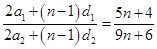
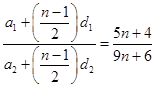
Now, to get 18^th term,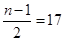

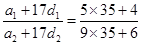
Therefore, the ratio of 18^th terms of two A.P.’s is 179: 321.

---

10. If the sum of first 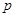terms of an A.P. is equal to the sum of the first 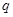terms, then find the sum of the first 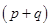terms.

Ans. Let 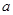be the first term and 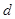be the common difference of given A.P.

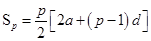and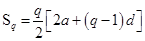

According to question,

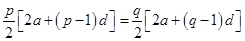
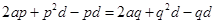
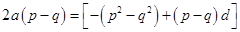
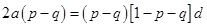
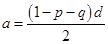
Now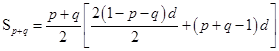

=

---

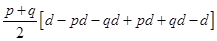
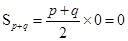
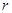11. Sum of the first and terms of an A.P. are 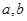and respectively. Prove that

Ans. Let A be the first term and 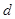be the common difference of given A.P.

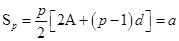
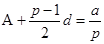……….(i)

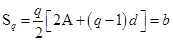
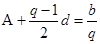……….(ii)

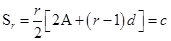
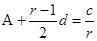……….(iii)

Now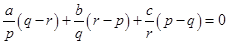

Putting the values of 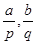and 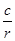from eq. (i), (ii) and (iii), we get

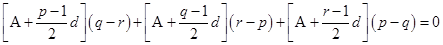
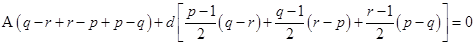
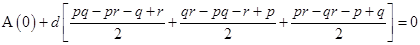
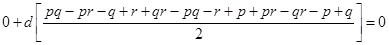
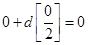
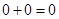
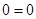
L.H.S. = R.H.S. Proved.

---

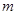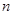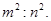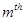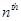12. The ratio of the sum of and terms of an A.P. is Show that the ratio of and term is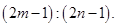

Ans. Let 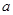be the first term and 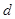be the common difference of given A.P.

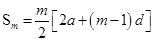and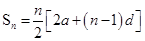

According to question,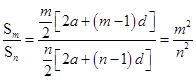

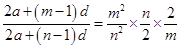
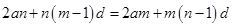
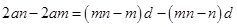
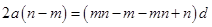
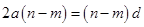
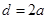
Now, =

=

---

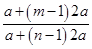
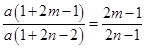
13. If the sum of 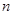terms of an A.P. is 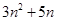and its 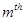term is 164, find the value of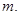

Ans. Given: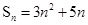 and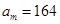

Replacing 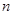by in 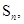we get

= =

=

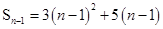
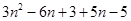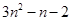
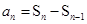
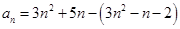
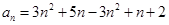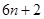
And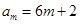

---

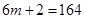
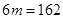
14. Insert five numbers between 8 and 26 so that the resulting sequence is an A.P.

Ans. Let A₁, A₂, A₃, A₄ and A₅ be five numbers between 8 and 26.

8, A₁, A₂, A₃, A₄, A₅, 26

Here,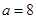 and 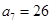and let 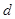be the common difference.

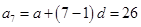
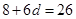
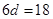
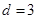
Now, A₁ =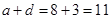

A₂ =

A₃ =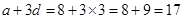

A₄ =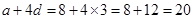

A₅ =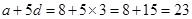

---

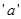15. If is the A.M. between and 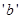then find the value of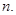

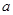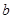Ans. Since, A.M. between and is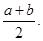

---

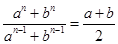
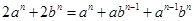
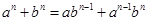
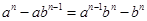
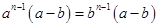
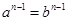
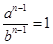
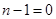
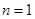
16. Between 1 and 31, 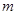numbers have been inserted in such a way that resulting sequence is an A.P. and the ratio of 7^th and 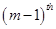numbers is 5: 9. Find the value of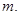

Ans. Let A₁, A₂, A₃, A₄, …….., A_m be 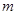numbers between 1 and 31.

Here,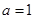 and let the common difference be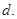

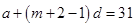
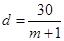
Now,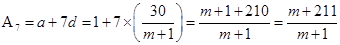

And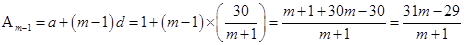

According to question,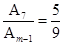

---

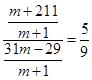
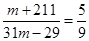
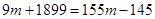
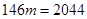
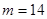
17. A man starts repaying a loan as first installment of Rs. 100. If he increases the installment by Rs. 5 every month, what amount he will pay in the 30^th installment?

Ans. Amount of 1^st installment = Rs. 100 and Amount of 2^nd installment = Rs. 105

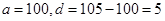and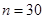

Now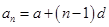

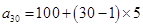
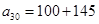= Rs. 245

Therefore, the amount of 30^th installment is Rs. 245.

---

18. The difference between any two consecutive interior angles of a polygon is 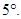If the smallest angle is 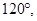find the number of the sides of the polygon.

Ans. Let the number of sides of polygon be 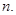The interior angles of the polygon form an A.P.

Here,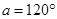 and

Since Sum of interior angles of a polygon with 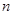sides is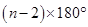

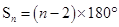
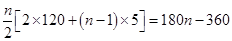
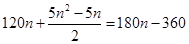
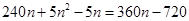
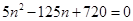
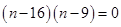
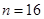or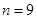

But 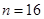not possible because == >

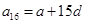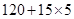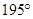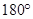
Therefore, number of sides of the polygon are 9.