NCERT Solutions for Class 10 Maths Exercise 11.2 Class 10 Maths book solutions are available in PDF format for free download. These ncert book chapter wise questions and answers are very helpful for CBSE board exam. CBSE recommends NCERT books and most of the questions in CBSE exam are asked from NCERT text books. Class 10 Maths chapter wise NCERT solution for Maths Book for all the chapters can be downloaded from our website and myCBSEguide mobile app for free.
NCERT solutions for Maths Constructions Download as PDF

NCERT Solutions for Class 10 Maths Constructions
Exercise 11.2
In each of the following, give the justification of the construction also:
1. Draw a circle of radius 6 cm. From a point 10 cm away from its centre, construct the pair of tangents to the circle and measure their lengths.
Ans. Given: A circle whose centre is O and radius is 6 cm and a point P is 10 cm away from its centre.
To construct: To construct the pair of tangents to the circle and measure their lengths.
Steps of Construction:
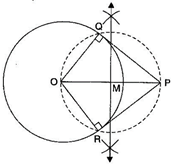
(a) Join PO and bisect it. Let M be the mid-point of PO.
(b) Taking M as centre and MO as radius, draw a circle. Let it intersects the given circle at the points Q and R.
(c) Join PQ and PR.
Then PQ and PR are the required two tangents.
By measurement, PQ = PR = 8 cm
Justification: Join OQ and OR.
Since  OQP and
OQP and  ORP are the angles in semicircles.
ORP are the angles in semicircles.
 OQP
OQP  =
=  ORP
ORP
Also, since OQ, OR are radii of the circle, PQ and PR will be the tangents to the circle at Q and R respectively.
 We may see that the circle with OP as diameter increases the given circle in two points. Therefore, only two tangents can be draw.
We may see that the circle with OP as diameter increases the given circle in two points. Therefore, only two tangents can be draw.
NCERT Solutions for Class 10 Maths Exercise 11.2
2. Construct a tangent to a circle of radius 4 cm from a point on the concentric circle of radius 6 cm and measure its length. Also verify the measurement by actual calculation.
Ans. To construct: To construct a tangent to a circle of radius 4 cm from a point on the concentric circle of radius 6 cm and measure its lengths. Also to verify the measurements by actual calculation.
Steps of Construction:
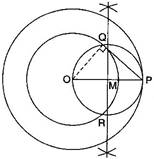
(a) Join PO and bisect it. Let M be the mid-point of PO.
(b) Taking M as centre and MO as radius, draw a circle. Let it intersects the given circle at the point Q and R.
(c) Join PQ.
Then PQ is the required tangent.
By measurement, PQ = 4.5 cm
By actual calculation,
PQ = 
= 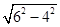 =
= 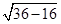
= 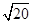 = 4.47 cm
= 4.47 cm
Justification: Join OQ. Then  PQO is an angle in the semicircle and therefore,
PQO is an angle in the semicircle and therefore,
 PQO =
PQO = 
 PQ
PQ  OQ
OQ
Since, OQ is a radius of the given circle, PQ has to be a tangent to the circle.
NCERT Solutions for Class 10 Maths Exercise 11.2
3. Draw a circle of radius 3 cm. Take two points P and Q on one of its extended diameter each at a distance of 7 cm from its centre. Draw tangents to the circle from these two points P and Q.
Ans. To construct: A circle of radius 3 cm and take two points P and Q on one of its extended diameter each at a distance of 7 cm from its centre and then draw tangents to the circle from these two points P and Q.
Steps of Construction:
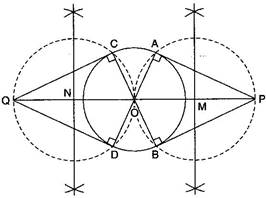
(a) Bisect PO. Let M be the mid-point of PO.
(b) Taking M as centre and MO as radius, draw a circle. Let it intersects the given circle at the points A and B.
(c) Join PA and PB.
Then PA and PB are the required two tangents.
(d) Bisect QO. Let N be the mid-point of QO.
(e) Taking N as centre and NO as radius, draw a circle. Let it intersects the given circle at the points C and D.
(f) Join QC and QD.
Then QC and QD are the required two tangents.
Justification: Join OA and OB.
Then  PAO is an angle in the semicircle and therefore
PAO is an angle in the semicircle and therefore  PAO =
PAO =  .
.
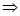 PA
PA  OA
OA
Since OA is a radius of the given circle, PA has to be a tangent to the circle. Similarly, PB is also a tangent to the circle.
Again join OC and OD.
Then  QCO is an angle in the semicircle and therefore
QCO is an angle in the semicircle and therefore  QCO =
QCO =  .
.
Since OC is a radius of the given circle, QC has to be a tangent to the circle. Similarly, QD is also a tangent to the circle.
NCERT Solutions for Class 10 Maths Exercise 11.2
4. Draw a pair of tangents to a circle of radius 5 cm which are inclined to each other at an angle of 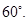
Ans. To construct: A pair of tangents to a circle of radius 5 cm which are inclined to each other at an angle of 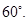
Steps of Construction:
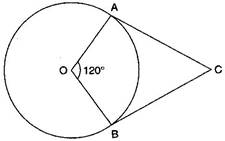
(a) Draw a circle of radius 5 cm with centre O.
(b) Draw an angle AOB of 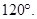
(c) At A and B, draw  angles which meet at C.
angles which meet at C.
Then AC and BC are the required tangents which are inclined to each other at an angle of 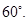
Justification:
 OAC =
OAC =  and OA is a radius.
and OA is a radius.
[By construction]
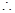 AC is a tangent to the circle.
AC is a tangent to the circle.
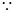
 OBC =
OBC =  and OB is a radius.
and OB is a radius.
[By construction]
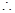 BC is a tangent to the circle.
BC is a tangent to the circle.
Now, in quadrilateral OACB,
 AOB +
AOB +  OAC +
OAC +  OBC +
OBC +  ACB =
ACB = 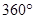
[Angle sum property of a quadrilateral]
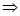
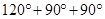 +
+  ACB =
ACB = 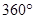
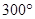 +
+  ACB =
ACB = 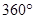
 ACB =
ACB = 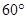
NCERT Solutions for Class 10 Maths Exercise 11.2
5. Draw a line segment AB of length 8 cm. Taking A as centre, draw a circle of radius 4 cm and taking B as centre, draw another circle of radius 3 cm. Construct tangents to each circle from the centre of the other circle.
Ans. To construct: A line segment of length 8 cm and taking A as centre, to draw a circle of radius 4 cm and taking B as centre, draw another circle of radius 3 cm. Also, to construct tangents to each circle from the centre to the other circle.
Steps of Construction:
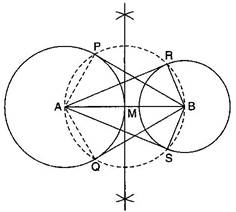
(a) Bisect BA. Let M be the mid-point of BA.
(b) Taking M as centre and MA as radius, draw a circle. Let it intersects the given circle at the points P and Q.
(c) Join BP and BQ.
Then, BP and BQ are the required two tangents from B to the circle with centre A.
(d) Again, Let M be the mid-point of AB.
(e) Taking M as centre and MB as radius, draw a circle. Let it intersects the given circle at the points R and S.
(f) Join AR and AS.
Then, AR and AS are the required two tangents from A to the circle with centre B.
Justification: Join BP and BQ.
Then  APB being an angle in the semicircle is
APB being an angle in the semicircle is 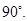
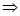 BP
BP  AP
AP
Since AP is a radius of the circle with centre A, BP has to be a tangent to a circle with centre A. Similarly, BQ is also a tangent to the circle with centre A.
Again join AR and AS.
Then  ARB being an angle in the semicircle is
ARB being an angle in the semicircle is 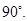
 AR
AR  BR
BR
Since BR is a radius of the circle with centre B, AR has to be a tangent to a circle with centre B. Similarly, AS is also a tangent to the circle with centre B.
NCERT Solutions for Class 10 Maths Exercise 11.2
6. Let ABC be a right triangle in which AB = 6 cm, BC = 8 cm and  B =
B =  BD is the perpendicular from B on AC. The circle through B, C, D is drawn. Construct the tangents from A to this circle.
BD is the perpendicular from B on AC. The circle through B, C, D is drawn. Construct the tangents from A to this circle.
Ans. To construct: A right triangle ABC with AB = 6 cm, BC = 8 cm and  B =
B =  BD is the perpendicular from B on AC and the tangents from A to this circle.
BD is the perpendicular from B on AC and the tangents from A to this circle.
Steps of Construction:
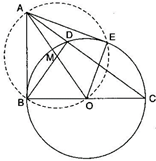
(a) Draw a right triangle ABC with AB = 6 cm, BC = 8 cm and  B =
B = 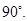 Also, draw perpendicular BD on AC.
Also, draw perpendicular BD on AC.
(b) Join AO and bisect it at M (here O is the centre of circle through B, C, D).
(c) Taking M as centre and MA as radius, draw a circle. Let it intersects the given circle at the points B and E.
(d) Join AB and AE.
Then AB and AE are the required two tangents.
Justification: Join OE.
Then,  AEO is an angle in the semicircle.
AEO is an angle in the semicircle.
 AEO =
AEO = 
 AE
AE  OE
OE
Since OE is a radius of the given circle, AE has to be a tangent to the circle. Similarly, AB is also a tangent to the circle.
NCERT Solutions for Class 10 Maths Exercise 11.2
7. Draw a circle with the help of a bangle. Take a point outside the circle. Construct the pair of tangents from this point to the circle.
Ans. To construct: A circle with the help of a bangle. Take a point outside the circle. Construct the pair of tangents from this point to the circle.
Steps of Construction:
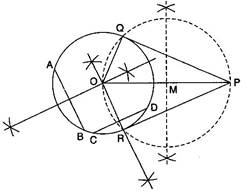
(a) Draw a circle with the help of a bangle.
(b) Take two non-parallel chords AB and CD of this circle.
(c) Draw the perpendicular bisectors of AB and CD. Let these intersect at O. Then O is the centre of the circle draw.
(d) Take a point P outside the circle.
(e) Join PO and bisect it. Let M be the mid-point of PO.
(f) Taking M as centre and MO as radius, draw a circle. Let it intersects the given circle at the points Q and R.
(g) Join PQ and PR.
Then PQ and PR are the required two tangents.
Justification: Join OQ and OR.
Then,  PQO is an angle in the semicircle.
PQO is an angle in the semicircle.
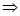
 PQO =
PQO = 
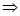 PQ
PQ  OQ
OQ
Since OQ is a radius of the given circle, PQ has to be a tangent to the circle. Similarly, PR is also a tangent to the circle.
NCERT Solutions for Class 10 Maths Exercise 11.2
NCERT Solutions for Class 10 Maths Exercise 11.2 PDF (Download) Free from myCBSEguide app and myCBSEguide website. Ncert solution class 10 Maths includes text book solutions from Mathematics Book. NCERT Solutions for CBSE Class 10 Maths have total 15 chapters. 10 Maths NCERT Solutions in PDF for free Download on our website. Ncert Maths class 10 solutions PDF and Maths ncert class 10 PDF solutions with latest modifications and as per the latest CBSE syllabus are only available in myCBSEguide.
CBSE app for Class 10
To download NCERT Solutions for Class 10 Maths, Computer Science, Home Science,Hindi ,English, Social Science do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT solutions, NCERT Exemplar solutions, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE

Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
Learn8 App
Practice unlimited questions for Entrance tests & government job exams at ₹99 only
Install Now