Ask questions which are clear, concise and easy to understand.
Ask QuestionPosted by Total Game Fire 1 year, 9 months ago
- 4 answers
Posted by Yashvi Stu 1 year, 9 months ago
- 1 answers
Posted by Aaisha Rabia Shabuddin 1 year, 9 months ago
- 1 answers
Preeti Dabral 1 year, 9 months ago
{tex}\begin{aligned} & \Longrightarrow \frac{2 \sqrt{6}}{\sqrt{2}+\sqrt{3}} \times \frac{\sqrt{2}-\sqrt{3}}{\sqrt{2}-\sqrt{3}}+\frac{6 \sqrt{2}}{\sqrt{6}+\sqrt{3}} \times \frac{\sqrt{6}-\sqrt{3}}{\sqrt{6}-\sqrt{3}}-\frac{8 \sqrt{3}}{\sqrt{6}+\sqrt{2}} \times \frac{\sqrt{6}-\sqrt{2}}{\sqrt{6}-\sqrt{2}} \\ & \Longrightarrow \frac{4 \sqrt{3}-6 \sqrt{2}}{2-3}+\frac{12 \sqrt{3}-6 \sqrt{6}}{6-3}-\frac{24 \sqrt{2}-8 \sqrt{6}}{6-2} \\ & \Longrightarrow-4 \sqrt{3}+6 \sqrt{2}+\frac{12 \sqrt{3}-6 \sqrt{6}}{3}-\frac{24 \sqrt{2}-8 \sqrt{6}}{4} \\ & \Longrightarrow-4 \sqrt{3}+6 \sqrt{2}+4 \sqrt{3}-2 \sqrt{6}-6 \sqrt{2}+2 \sqrt{6} \\ & \Longrightarrow-4 \sqrt{3}+6 \sqrt{2}+4 \sqrt{3}-2 \sqrt{6}-6 \sqrt{2}+2 \sqrt{6} \\ & \Longrightarrow 0 \end{aligned}{/tex}
Posted by Ujjwal Thakur 1 year, 9 months ago
- 0 answers
Posted by Ayisha A 1 year, 9 months ago
- 1 answers
Aryan Chauhan 1 year, 9 months ago
Posted by Ashutosh Yadav 1 year, 9 months ago
- 1 answers
Posted by S. Kolvalai 1 year, 9 months ago
- 2 answers
Preeti Dabral 1 year, 9 months ago
SAS congruence criterion: If two sides and included an angle of one triangle are equal to the corresponding two sides and included angle of another triangle then the two triangles are said to be congruent.
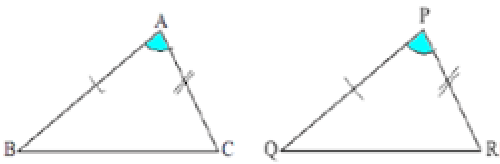
Consider {tex}\triangle{/tex}ABC, {tex}\triangle{/tex}PQR
here AB = PQ, AC = PR and {tex}\angle{/tex}A = {tex}\angle{/tex}P
Hence {tex}\triangle{/tex}ABC {tex}\cong{/tex} {tex}\triangle{/tex}PQR by SAS congruence criterion.
Posted by Yogesh Shekhadar 1 year, 9 months ago
- 1 answers
Posted by Sumedh Wani 1 year, 9 months ago
- 1 answers
Posted by Harini Murugan Harini 1 year, 9 months ago
- 1 answers
Preeti Dabral 1 year, 9 months ago
For class room : l = 7m, b = 6.5 m, h = 4 m
∴ Area of walls of the room = 2(l + b)h = 2(7 + 6.5)4 = 108 m2
Area of door = 3 × 1.4 = 4.2 m2
Area of one window = 2 × 1 = 2 m2
∴ Area of 3 windows = 3 × 2 = 6 m2
∴ Area of the walls of the room to be colour washed = 108 – (4.2 + 6)
= 108 – 10.2 = 97.8 m2
∴ Cost of colour washing the classroom at Rs. 15 per square metre = Rs 97.8 × 15 = Rs. 1467.
Posted by Sakshi Jaiswal 1 year, 9 months ago
- 1 answers
Preeti Dabral 1 year, 9 months ago
Constructing triangle ABC in which AB = 5.8 cm , BC + CA = 8.4 cm and ∠B = 45°.
Step 1: Draw a line segment AB 5.8 cm.
Step 2 : Draw ∠B = 45°.
Step 3 : With Center B and radius 8.4 cm, make an arc which intersects BX at D.
Step 4 : Join D to A.
Step 5 : Draw a perpendicular bisector of segment DA it intersect the line segment BD at point C.
Step 6 : Join C to A.
ABC is the required triangle.
Posted by Archita Singh 1 year, 10 months ago
- 2 answers
Posted by Rishika Minj 1 year, 10 months ago
- 1 answers
Posted by Amit Chaudhary 1 year, 10 months ago
- 0 answers
Posted by Aashika Bi 1 year, 9 months ago
- 1 answers
Preeti Dabral 1 year, 9 months ago
Since we know that rational number between two rational numbers is obtained by {tex}\frac{1}{2}{/tex}[a+b]
A rational number lying between -2 and -3 is {tex}\frac{1}{2}{/tex}[(-2) + (-3)],
i.e.,{tex}-\frac{5}{2}{/tex}
Now, a rational number lying between -2 and{tex}-\frac{5}{2}{/tex}is
{tex}\left.\frac{1}{2}[(-2)+\left(-\frac{5}{2}\right)\right ]{/tex}
= {tex} \frac{1}{2} \times\left(-\frac{9}{2}\right) {/tex}
= {tex}-\frac{9}{4} {/tex}
And, a rational number lying between {tex}-\frac{5}{2}{/tex} and -3 is
{tex}\frac{1}{2}\left(\left(-\frac{5}{2}\right)+(-3)\right) {/tex}
{tex}\frac{1}{2} \times\left(-\frac{11}{2}\right){/tex}
{tex}-\frac{11}{4} {/tex}
Thus, we have {tex}-2>-\frac{9}{4}>-\frac{5}{2}>-\frac{11}{4}>3{/tex}
Hence, three rational numbers between -2 and -3 are {tex}-\frac{9}{4},-\frac{5}{2}{/tex} and {tex}-\frac{11}{4}{/tex}
Posted by Mukund Vishwakarma 1 year, 10 months ago
- 1 answers
Posted by Bonty Clock Gaming 1 year, 9 months ago
- 1 answers
Preeti Dabral 1 year, 9 months ago
From the figure, we observe that when different pairs of circles are drawn, each pair have two points (say A and B) in common.
Maximum number of common points are two in number.
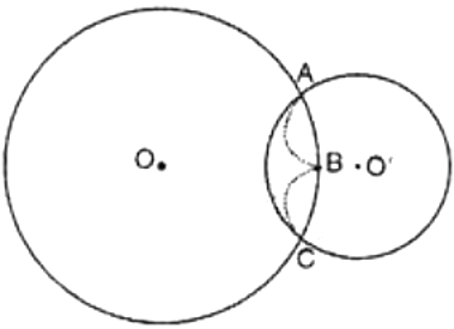
Suppose two circles C (O) and C (O’) intersect each other in three points, say A, B and C.
Then A, B and C are non-collinear points.
We know that:
There is one and only one circle passing through three non-collinear points.
Therefore, a unique circle passes through A, B and C.
{tex} \Rightarrow {/tex} O’ coincides with O and s = r where s and r are the radii of two circles C(O) and C(O')
A contradiction to the fact that C (O’,) {tex} \ne {/tex} C (O,)
{tex}\therefore {/tex} Our supposition is wrong.
Hence two different circles cannot intersect each other at more than two points.
Posted by Ashita Tamang 1 year, 10 months ago
- 2 answers
Posted by Yash Kaur 1 year, 9 months ago
- 1 answers
Preeti Dabral 1 year, 9 months ago
- Surface area of the sphere ={tex}4\pi r^2{/tex}
- For cylinder
Radius of the base = r
Height = 2r
{tex}\therefore{/tex} Curved surface area of the cylinder = {tex}2\pi rh=2\pi (r)(2r)=4\pi r^2{/tex} - Ratio of the areas obtained in (i) and (ii)
{tex}={{surface\ area\ of\ the\ sphere}\over{curved\ surface\ area\ of\ the\ cylinder}}{/tex}
{tex}={{4\pi r^2}\over{4\pi r^2}}={1\over1}=1:1{/tex}
Posted by Shivam Giri 1 year, 10 months ago
- 1 answers
Posted by Mukesh Singh 1 year, 10 months ago
- 3 answers
Vansh Katiyar 1 year, 10 months ago
Sambit Kumar Das 1 year, 10 months ago
myCBSEguide
Trusted by 1 Crore+ Students
Test Generator
Create papers online. It's FREE.
CUET Mock Tests
75,000+ questions to practice only on myCBSEguide app
Pavan Pavan 1 year, 9 months ago
0Thank You