CBSE > Class 09 > Mathematics | CBSE Board | Homework Help

Let ABCD be a square When diagonals are constructed we get two triangle that is ∆ ADC and ∆ BCD We have to prove that these triangles are congruent (same) to justify that diagonals are equal. In ∆ADC and ∆ BCD AD =BC (sides) DC =DC (common) Angle ADC = angle BCD (90) So ∆ADC is congruent to ∆BCD AC =BD (CPCT :- coresponding part of congruent triangle ) AC and BD are diagonals of square ABCD In same square let us take the ∆AOD and ∆BOC Note:- here O is the intersection point of diagonals of square In ∆AOD and ∆BOC AD = BC Angle ADO = Angle OBC Angle DAO =Angle OCB (Alternative interior angle) ∆AOD and ∆BOC are congruent AO = OC (CPCT) DO = OB (CPCT) Hence diagonals bisect each other Same way when we prove ∆AOD is congruent to ∆DOC using SSS congruence rule we will get Angle AOD = Angle DOC (CPCT)---------- note 1 let angle AOD = angle 1 let angle DOC = angle 2 When we add angle 1 and 2 we will get 180° Angle 1 + Angle 2 = 180° From note 1 Angle 1= angle 2 So, angle 1+ angle 1 = 180° 2(angle 1) = 180° Angle 1 = 90° Angle 1, angle 2 = 90° Hence diagonals of a square are equal and bisect each other at right angle Hope you got it!!! ☺️☺️

3Thank You

ANSWER

(i) What is the probability of selecting a male participant to inaugurate the meeting? (ii) If a team leader is to be selected from the participants. What is the probability of selecting a female team leader of age less than 30 years? OR (iii) If 4 more female participants of age less than 30 years and 5 more male participants of age more than 30 years joins the meeting, what is the probability of selecting a male participant of age less than 30 years to give vote of thanks? (iv) What is the probability of selecting a female participant of age more than 30 years to conclude the meeting?

Posted by Onefinemorning05@Gmail.Com Morning 2 years, 3 months ago

CBSE > Class 09 > Mathematics

1 answers

Onefinemorning05@Gmail.Com Morning 2 years, 3 months ago

Answer

1Thank You

ANSWER

0.345bar

Posted by Zainab Bushra 2 years, 3 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Prove that if 5=5a then what is the 4=1a ? If 4=1 is 0.25

Posted by Twing Gaming Gamerz 2 years, 3 months ago

CBSE > Class 09 > Mathematics

1 answers

Ravi Shankar Kumar 2 years, 3 months ago

Hlo

1Thank You

ANSWER

Prove 5is an irrational

Posted by Ujjwal Pandit 2 years, 3 months ago

CBSE > Class 09 > Mathematics

3 answers

Shamitha Shami 2 years, 3 months ago

Actually 5 is a rational number because it can be represented in the form of p/q Ex:- 5/1

1Thank You

Rashi Kaushik 2 years, 3 months ago

Sample paper solve set2

0Thank You

Mohit Yadav 2 years, 3 months ago

5 is not a irrational number

1Thank You

ANSWER

How we can proof that a given no. Is rational or irrational

Posted by Ali Talib 2 years, 3 months ago

CBSE > Class 09 > Mathematics

3 answers

Shamitha Shami 2 years, 3 months ago

* Hey 5/3 is rational number

0Thank You

Shamitha Shami 2 years, 3 months ago

If we can represent a number in the form of p/q then it is a rational number if cannot then it is a irrational number Ex:- 5/3 is irrational √2 is irrational

0Thank You

Ujjwal Pandit 2 years, 3 months ago

We let any no. In p/q form and squaring both side then q divide q2 then they divide q also

0Thank You

ANSWER

If p(x) =5x - 4x^2 + 3 then find p (-1)

Posted by Oas&Ojas Kedia 2 years, 3 months ago

CBSE > Class 09 > Mathematics

3 answers

Dena Joshi 2 years, 2 months ago

-6

0Thank You

Shamitha Shami 2 years, 3 months ago

Here substitute -1 in place of x in the equation 5x-4x^2+3 = 5(-1) -4(-1)^2 + 3 = -5 -4(1) + 3 = -5-4+3 = -9+3 =-6 hope you got it!!! ☺️☺️☺️

0Thank You

Vedshree Patil 2 years, 3 months ago

It 2

0Thank You

ANSWER

We observe that the cuboid is made of six rectanglar faces sum of area of all the six rectangles= _________ square units

Posted by Sameer John Santhi 2 years, 3 months ago

CBSE > Class 09 > Mathematics

2 answers

Sushamita Singh 2 years, 3 months ago

2(lb+bh+hl) , l = length h = height b = breadth

1Thank You

Harshit Agarwal 2 years, 3 months ago

2(lb+bh+hl)

1Thank You

ANSWER

t²+4t+3

Posted by Sumit Lohani 2 years, 3 months ago

CBSE > Class 09 > Mathematics

1 answers

Viswajeet Reddy Bheemireddy 2 years, 3 months ago

We know that factors of 3 are 1,3 Hence, 4t=1t+3t So, t²+4t+3 t²+1t+3t+3 t(t+1)+3(t+1) ((taking t and 3 common)) (t+3)(t+1) Hence this is your answer.

1Thank You

ANSWER

Two chord ab and cd are 6 cm & 12 cm respectively and distance between both is 3 cm find the radius of circle

Posted by Arnav Jain 2 years, 3 months ago

CBSE > Class 09 > Mathematics

1 answers

Preeti Dabral 2 years, 3 months ago

Let AB and CD be two parallel chords of a circle with centre O such that AB = 6 cm and CD = 12 cm. Let the radius of the circle be r cm. Draw OP $\perp$ AB and OQ $\perp$ CD. Since AB || CD and OP $\perp$ AB, OQ $\perp$ CD. Therefore, points O, Q, and P are collinear.

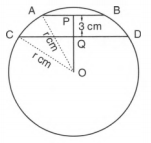
Clearly, PQ = 3 cm
Let OQ = x cm. Then, OP = (x + 3) cm
Applying Pythagoras theorem in right triangles OAP and OCQ, we obtain
OA² = OP² + AP² and OC² = OQ² + CQ²
$\Rightarrow$ r² = (x + 3)² + 3² and r² = x² + 6² [ $\because$ AP = $\frac{1}{2}$ AB = 3 cm and CQ = $\frac{1}{2}$ CD = 6 cm]
$\Rightarrow$ (x + 3)² + 3² = x² + 6² [On equating the values of r²]
$\Rightarrow$ x² + 6x + 9 + 9 = x² + 36 $\Rightarrow$ 6x = 18 $\Rightarrow$ x = 3
Putting the value of x in r² = x² + 6², we get
r² = 3² + 6² = 45 $\Rightarrow$ r = $\sqrt{45}$ cm = 6.7 cm
Hence, the radius of the circle is 6.7 cm.

0Thank You

ANSWER

In the given figure, ÐPQR = 120° where P, Q and R are points on the circle with centre O. Find ÐOPR

Posted by Khushee Bhogayta 2 years, 3 months ago

CBSE > Class 09 > Mathematics

1 answers

Preeti Dabral 2 years, 3 months ago

Here, ∠PQR=100^∘

Take a point S in the major arc. Join PS and RS.

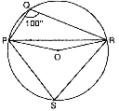
$\because$ PQRS is a cyclic quadrilateral.
$\therefore \angle \mathrm { PQR } + \angle \mathrm { PSQ } = 180 ^ { \circ }$
|The sum of either pair of opposite angles of a cyclic quadrilateral is 180^o
$\Rightarrow 100 ^ { \circ } + \angle P S R = 180 ^ { \circ }$
$\Rightarrow \angle P S R = 180 ^ { \circ } - 100 ^ { \circ }$
$\Rightarrow \angle P S R = 80 ^ { \circ }$ ......... (1)
Now, $\angle \mathrm { POR } = 2 \angle \mathrm { PSR }$
|The angle subtended by an arc at the centre is double the angle subtended by it at any point on the remaining part of the circle
$= 2 \times 80 ^ { \circ } = 160 ^ { \circ }$ ........ (2) |Using (1)
In $\triangle O P R$
$\because O P = O R$ |Radii of a circle
$\therefore \angle \mathrm { OPR } = \angle \mathrm { ORP }$ ....... (3)
|Angles opposite to equal sides of a triangle are equal
In $\triangle O P R$
$\angle O P R + \angle O R P + \angle P O R = 180 ^ { \circ }$ | Sum of all the angles of a triangle is 180^o
$\Rightarrow \angle \mathrm { OPR } + \angle \mathrm { OPR } + 160 ^ { \circ } = 180 ^ { \circ }$ |Using (2) and (1)
$\Rightarrow 2 \angle O P R + 160 ^ { \circ } = 180 ^ { \circ }$
$\Rightarrow 2 \angle O P R = 180 ^ { \circ } - 160 ^ { \circ } = 20 ^ { \circ }$
$\Rightarrow \angle \mathrm { OPR } = 10 ^ { \circ }$

0Thank You

ANSWER

If conical glass is 35cm in diameter and 12 cm deep then its capacity is in litre

Posted by Ansh Preet 2 years, 3 months ago

CBSE > Class 09 > Mathematics

1 answers

Barsha Deb 2 years, 3 months ago

0.42

2Thank You

ANSWER

15. Read the Source/Text given below and answer any four questions: 2x km ykm 2y km 600 km Ajay lives in Delhi. The city of Ajay's father in laws residence is at Jaipur is 600 km from Delhi. Ajay used to travel this 600 km partly by train and partly by car. He used to buy cheap items from Delhi and sale at Jaipur and also buying cheap items from Jaipur and sale at Delhi. Once From Delhi to Jaipur in forward journey he covered 2x km by train and the rest y km by taxi. But, while returning he did not get a reservation from Jaipur in the train. So first 2y km he had to travel by taxi and the rest x km by Train. From Delhi to Jaipur he took 8 hrs but in returning it took 10 hrs. i. What is the value of x? a. 400 km. b. 200 km c. 600 km d. 300 km ii. What is the value ofy ? a. 200 km b. 400 km c. 600 km d. 300 km iii. In Delhi to Jaipur journey how much distance did he travel by train? a. 200 km b. 300 km c. 600 km. d. 400 km iv. How much distance did he travel by train in both side journey? a. 200 km b. 300 km c. 400 km d. 600 km v. how much distance did he travel by taxi in both side journey? a. 200 km b. 600 km c. 400 km d. 300 km

Posted by Sourav Ranjan Das 2 years, 3 months ago

CBSE > Class 09 > Mathematics

2 answers

Lakshya Lakshya 2 years, 3 months ago

Hello

0Thank You

Pavan Pavan 2 years, 3 months ago

0Thank You

ANSWER

Ch 12 ka extra question

Posted by Ayushi Yadav 2 years, 3 months ago

CBSE > Class 09 > Mathematics

3 answers

Anshika Bansal 2 years, 3 months ago

Yes

0Thank You

Kiran Pawar 2 years, 3 months ago

Is 12.2 exercise cancelled from syllabus?

0Thank You

Samadhan Biswas 2 years, 3 months ago

Nahi hai

0Thank You

ANSWER

Scince

Posted by Veerendra Ravi 2 years, 3 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

X=√a+2b/√a+2b+√a-2b/-√a-2b then prove that bx^2-ax+b=0

Posted by Shivansh Pandey 2 years, 3 months ago

CBSE > Class 09 > Mathematics

1 answers

Pratik Bidhuri 2 years, 3 months ago

Answer

0Thank You

ANSWER

Which is greater among √2 , ³√4 and ⁴√3

Posted by Vaibhav Pandey 2 years, 3 months ago

CBSE > Class 09 > Mathematics

1 answers

Preeti Dabral 2 years, 3 months ago

Therefore, 3√4 is greater.

0Thank You

ANSWER

a hemispherical bowl made of brass has inner diameter 10.5 cm. Find the cost of tin plating it on inside at rate of rs.16 per 0.01m²

Posted by Saswat Singh 2 years, 3 months ago

CBSE > Class 09 > Mathematics

1 answers

Preeti Dabral 2 years, 3 months ago

Inner diameter of bowl = 10.5 cm
$\therefore$ Inner radius of bowl $\left( r \right)=\frac{10.5}{2}$ = 5.25 cm
Now, Inner surface area of bowl = $2\pi {{r}^{2}}$
$=2\times \frac{22}{7}\times 5.25\times 5.25$
$=2\times \frac{22}{7}\times \frac{21}{4}\times \frac{21}{4}$
= $\frac{693}{4}c{{m}^{2}}$
$\because$ Cost of tin-plating per $100\text{ }c{{m}^{2}}$ = ₹ 16
$\therefore$ Cost of tin-plating per $1\text{ }c{{m}^{2}}=\frac{16}{100}$
$\therefore$ Cost of tin-plating per $\frac{693}{4}c{{m}^{2}}=\frac{16}{100}\times \frac{693}{4}$ = ₹27.72