CBSE > Class 09 > Mathematics | CBSE Board | Homework Help

Here,
BC = CD … (Sides of square)
CQ = DR … (Given)
BC = BQ + CQ
$\therefore$ CQ = BC − BQ
$\therefore$ DR = BC – BQ .......(1)
Also,
CD = RC + DR
$\therefore$ DR = CD - RC = BC - RC ........(2)
From (1) and (2), we have,
BC - BQ = BC - RC
$\therefore$ BQ = RC
Now in $\triangle$ RCQ and $\triangle$ QBP
we have, PB = QC … (Given)
BQ = RC … [from (i)]
$\angle$ RCQ = $\angle$ QBP … 90^o each
Hence by SAS(Side-Angle-Side) congruence rule,
$\triangle$ RCQ $\cong$ $\triangle$ QBP
$\therefore$ QR = PQ … (by cpct)
$\triangle$ RCQ $\cong$ $\triangle$ QBP and QR = PQ … [from (2)]
$\therefore$ In $\triangle$ RPQ,
$\angle$ QPR = $\angle$ QRP = $\frac{1}{2}$ (180^o - 90^o) = $\frac{90}{2}$ = 45^o
$\therefore$ $\angle$ QPR = 45^o

1Thank You

Nilanjna Singh 2 years, 4 months ago

Thanks

0Thank You

ANSWER

Abcd is a cyclic quadrilateral in which ad=bc ab=cd and d=b, then cab is equal to

Posted by Hena Kausar 2 years, 4 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

In the given figure, OT bisects the chord AB such that AT = TB, OT = 12 cm, and the radius of the circle is 13 cm. Find the length of the chord AB.

Posted by Sai Varshitha.A 2 years, 4 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

In right triangle ABC, right angled at C, M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B (see the given figure). Show that: ( ) ( ) ( ) ( ) i AMC BMD ii DBC is a right angle. iii DBC ACB 1 iv CM AB

Posted by Jinali Zaveri 2 years, 4 months ago

CBSE > Class 09 > Mathematics

1 answers

Preeti Dabral 2 years, 4 months ago

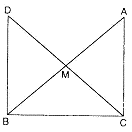
Given: In right triangle ABC, right angled at C. M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B.
To Prove:

$\triangle$ AMC $\cong$ $\triangle$ BMD
$\angle$ DBC is a right angle
$\triangle$ DBC $\cong$ $\triangle$ ACB
CM = $\frac {1} {2}$ AB

Proof:

In $\triangle$ AMC and $\triangle$ BMD
AM = BM ...[As M is the mid-point]
CM = DM ...[Given]
$\angle$ AMC = $\angle$ BMD ...[Vertically opposite angles]
$\therefore$ $\triangle$ AMC $\cong$ $\triangle$ BMD proved ...[SAS property] ...(1)
$\triangle$ AMC $\cong$ $\triangle$ BMD ...[From (1)]
$\angle$ ACM = $\angle$ BDM ...[c.p.c.t.]
These are alternate interior angles and they are equal.
$\therefore$ AC $\|$ BD
As AC $\|$ BD and transversal BC intersects them
$\therefore$ $\angle$ DBC + $\angle$ ACB = 180° ...[Sum of the consecutive interior angles of the transversal]
$\angle$ DBC + 90° = 180°
$\angle$ DBC = 180° - 90° = 90°
$\angle$ DBC is a right angle proved.
$\triangle$ AMC $\cong$ $\triangle$ BMD ...[From (1)]
$\therefore$ AC = BD ...[c.p.c.t.] ...(2)
In DDBC and DACB
BC = CB ...[Common]
$\angle$ DBC = $\angle$ ACB ...[each = 90°as proved above]
BD = CA ...[From (2)]
$\therefore$ $\triangle$ DBC $\cong$ $\triangle$ ACB ...[SAS property]
DDBC $\cong$ DACB ...[As proved in (iii)]
$\therefore$ DC = AB ...[c.p.c.t.]
$\therefore$ 2CM = AB ...[DM = CM = $\frac {1} {2}$ DC]
$\therefore$ CM = $\frac {1} {2}$ AB

0Thank You

ANSWER

Show that the quadrilateral formed by joining the mid points of the sides of a square is also a square

Posted by Sonali Verma 2 years, 4 months ago

CBSE > Class 09 > Mathematics

1 answers

Preeti Dabral 2 years, 4 months ago

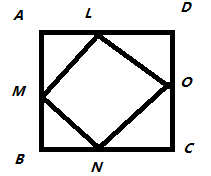
Let a square ABCD in which L, M, N & O are the midpoints.
In $\triangle$ AML and $\triangle$ CNO
AM = CO (AB = DC and M and O are the midpoints)
AL = CN (AD = BC and L and N are the midpoints)
$\angle$ MAL = $\angle$ NCO (all angles of a square = 90°)
by AAS criteria
$\triangle$ AML $\cong$ $\triangle$ CNO
$\therefore$ ML = ON (CPCT)
similarly $\triangle$ MBN $\cong$ $\triangle$ LDO
now,
in $\triangle$ AML,
$\angle$ AML = $\angle$ ALM (AM = AL)
= 45°
similarly in $\triangle$ LDO
$\angle$ DLO = 45°
$\therefore$ $\angle$ MLO = 90°
by the properties of SQUARE
All sides are equal and angles are 90°

0Thank You

ANSWER

x= 3y linear equations in the form ax+by+c=0

Posted by Harshdeep Raj 2 years, 4 months ago

CBSE > Class 09 > Mathematics

1 answers

Shreya Pandey 2 years, 4 months ago

1-3y+0=0

0Thank You

ANSWER

Can someone explain me the factor and remainder theorem please?

Posted by Allpesha Shambharkar 2 years, 4 months ago

CBSE > Class 09 > Mathematics

2 answers

Preeti Dabral 2 years, 4 months ago

Factor Theorem is generally applied to factoring and finding the roots of polynomial equations. It is the reverse form of the remainder theorem. Problems are solved based on the application of synthetic division and then to check for a zero remainder.

1Thank You

Allpesha Shambharkar 2 years ago

Thank you so much for answering 😊

0Thank You

ANSWER

What is phosphorus atomic mass

Posted by Nandini.M ......... 2 years, 4 months ago

CBSE > Class 09 > Mathematics

2 answers

Nandini.M ......... 2 years, 4 months ago

Yes ur right tanishk Verma

1Thank You

Tanishk Verma 2 years, 4 months ago

31 amu

2Thank You

ANSWER

Triangle ABC and triangle BDC is isosceles triangle on the same base BC and vertices A and D are on the same side of BC. If AD is extended to intersect BC at E. Show that (1) triangle ABD congrent triangle ACD (2) triangle ABE congrent triangle ACE (3)AE is bisects angle A as well as angle D (4)AE is the perpendicular bisector of BC

Posted by Alisha Parveen 2 years, 4 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Triangle ABS and triangle BDC is isosceles triangle on the same base BC and vertices A and D are on the same side of BC. If AD is extended to intersect BC at E. Show that (1) triangle ABD congrent triangle ACD (2) triangle ABE congrent triangle ACE (3)AE is bisects angle A as well as angle D (4)AE is the perpendicular bisector of BC

Posted by Alisha Parveen 2 years, 4 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Simplify - (√8-√5) (√8+√5)

Posted by Harsimran Kaur 2 years, 4 months ago

CBSE > Class 09 > Mathematics

3 answers

Tahalka .. 2 years, 4 months ago

3..

0Thank You

Tanishk Verma 2 years, 4 months ago

By using (a+b) (a-b) = a²-b² (√8)²-(√5)² = 8-5 = 3

2Thank You

Mohd Rihaan 2 years, 4 months ago

0Thank You

ANSWER

5_-9-

Posted by Krish Yadav 2 years, 4 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Ab parelllel cd

Posted by Shravan Namdeowar 2 years, 4 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Three angle of a quadrilateral are equal and the fourth angle is equal to 144°.find each of the equal angles of the quadrilateral

Posted by Jeel Patel 2 years, 5 months ago

CBSE > Class 09 > Mathematics

4 answers

Tahalka .. 2 years, 4 months ago

72°each

0Thank You

Aditya Yadav 2 years, 4 months ago

Sum of all angles in a quadrilateral is 360° 3x+144°=360 3x=360°-144° x=216°/3 x=72° Ans

0Thank You

Rani Sahu 2 years, 4 months ago

3 equal side = 72°

0Thank You

Laksh Rathi 2 years, 5 months ago

Hehe boi

0Thank You

ANSWER

Theroem 8.3

Posted by Durga Morya 2 years, 5 months ago

CBSE > Class 09 > Mathematics

1 answers

Suprabha Barik 2 years, 4 months ago

If each pair of opposite side of a quadrilateral is equal then it is a parallelogram.

2Thank You

ANSWER

O 5

Posted by Sandeep Singh 2 years, 5 months ago

CBSE > Class 09 > Mathematics

1 answers

Anchal Dualgch Dulgach 2 years, 5 months ago

0Thank You

ANSWER

If one rotation of 720 degree in one second then how many turns will it take in 1minute

Posted by Neha Srinitya Banoth 2 years, 5 months ago

CBSE > Class 09 > Mathematics

1 answers

Aditya Yadav 2 years, 4 months ago

720° rotation in one second Then 720°×60second =43200° One turns means 360° then 43200/360=120 turns

2Thank You

ANSWER

If a+ b+c =5,, ab+bc+ca=10,, Prove that a3 +b3+c3- 3abc=-25

Posted by Nupur Saraswat 2 years, 5 months ago

CBSE > Class 09 > Mathematics

1 answers

Aditya Yadav 2 years, 4 months ago

Wait bro......

1Thank You

ANSWER

The radius of a hemisphere balloon increase from 6cm to 12cm as air is being pumped into it. The ratio of the surface areas of the balloon in the two cases is

Posted by Kavin M 2 years, 5 months ago

CBSE > Class 09 > Mathematics

2 answers

Sheetal Gupta 2 years, 4 months ago

Curved surface area of small hemisphere/Curved surface area of big hemisphere 2πrh/2πrh (2sa2andπsaπandhsah cut jayga) Small hemisphere=6 Big hemisphere=12 6/12 Ratio=1:2

0Thank You

Lalit Chand Prajapati 2 years, 5 months ago

Hjfy

0Thank You

ANSWER

Is anyone here who participated mental maths quiz

Posted by Ishta Mahour 2 years, 5 months ago

CBSE > Class 09 > Mathematics

1 answers

Priyanshi Tripathi 2 years, 5 months ago

Yes ..

0Thank You

ANSWER

Circles eg 6

Posted by Dhan Kgv 2 years, 5 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Solve the following identities 1. (5x-4y)2

Posted by Minal Sinha 2 years, 5 months ago

CBSE > Class 09 > Mathematics

3 answers

Aditya Yadav 2 years, 4 months ago

Hello..... 25x2+16y2-2×-20xy 25x2+16y2+40xy

0Thank You

Priyanshi Tripathi 2 years, 5 months ago

10x-8y ...I hope this answer help uh

0Thank You

Vineet Yadav 2 years, 5 months ago

10x-8y

0Thank You

ANSWER

ABCD is a parallelogram of area 102 cm².If E and F are the mid-point of sides AB and CD respectively,then find the area of AEF

Posted by Yashika Lalwani 2 years, 5 months ago

CBSE > Class 09 > Mathematics

1 answers

Aditya Yadav 2 years, 4 months ago

Hello girl... By using theorem 8.1 A diagonal of a parallelogram divides it into two congrute triangles means area also will be same And AEFD is a parallelogram and AEF is a triangle and a trangle is half of parallelogram then 102/2=51 and 51 divide in to two triangle 51/2=25.5

0Thank You

ANSWER

ABCD is a parallelogram of area 102 cm².If E and F are the mid-point of sides AB and CD respectively,then find the area of AEFD

Posted by Yashika Lalwani 2 years, 5 months ago

CBSE > Class 09 > Mathematics

2 answers

Nikil Rawat 2 years, 5 months ago

First draw a figure in your notebook so that you can get a clear idea of the question. If ABCD is a parallelogram and we join the mid points of opposite sides then we can say that now the parallelogram is divided into two new quadrilateral which are equal. So, AEFD = BEFC Now we are given with the area of parallelogram which is 102cm square. We can say that AEFD + BECF = Area of parallelogram ABCD. Since AEFD = BECF the we can put AEFD in the place of BECF. AEFD + AEFD = 102cm 2AEFD = 102CM AEFD = 102/2 AEFD = 51cm square. HOPE THIS WAS HELPFUL

0Thank You

Pranjal Gupta 2 years, 5 months ago

Month day

0Thank You

ANSWER

3 - 2

Posted by Abhijeet Shukla 2 years, 5 months ago

CBSE > Class 09 > Mathematics

4 answers

Suprabha Barik 2 years, 4 months ago

0Thank You

Priyanshi Tripathi 2 years, 5 months ago

0Thank You

Ishta Mahour 2 years, 5 months ago

0Thank You

Anushka Rajput 2 years, 5 months ago

0Thank You

ANSWER

P(t)=2+t+2t

Posted by Jeevika Jeevika 2 years, 5 months ago

CBSE > Class 09 > Mathematics

2 answers

Priyanshi Tripathi 2 years, 5 months ago

I thing the correct answer is 5 because when we put the value of t is 1 so the answer is 5 ...u can put different values of t and u can fine different answers

0Thank You

Vineet Yadav 2 years, 5 months ago

2/3

0Thank You

ANSWER