In a circular table cover of …

Area of the design (shaded region)
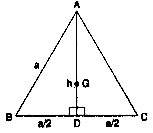
= Area of the circular table cover - Area of the equilateral triangle ABC
$= \pi ( 32 ) ^ { 2 } - \frac { \sqrt { 3 } } { 4 } a ^ { 2 } - ( 1 )$
Where a cm is the side of the equilateral triangle ABC.
Let h cm be the height of $\Delta A B C$
Since the centre of the circle coincides with the combined of the equilateral triangle.
Therefore, Radius of the 
circumscribed circle  = $\frac { 2 } { 3 } h \mathrm { cm }$

According to the question,
$\frac { 2 } { 3 } h = 32 \Rightarrow h = 48$
Again, $a ^ { 2 } = h ^ { 2 } + \left( \frac { a } { 2 } \right) ^ { 2 }$ ........ By Pythagoras theorem
$\Rightarrow a ^ { 2 } = h ^ { 2 } + \frac { a ^ { 2 } } { 4 }$
$\Rightarrow \mathrm { a } ^ { 2 } - \frac { \mathrm { a } ^ { 2 } } { 4 } = \mathrm { h } ^ { 2 } = \frac { 3 \mathrm { a } ^ { 2 } } { 4 } = \mathrm { h } ^ { 2 }$
$\Rightarrow a ^ { 2 } = \frac { 4 h ^ { 2 } } { 3 } \Rightarrow a ^ { 2 } = \frac { 4 ( 48 ) ^ { 2 } } { 3 }$
$\Rightarrow \mathrm { a } ^ { 2 } = 3072 \Rightarrow \mathrm { a } = \sqrt { 3072 }$
$\therefore$ Form (1)
Required area $= \pi ( 32 ) ^ { 2 } - \frac { \sqrt { 3 } } { 4 } ( 3072 )$
$= \frac { 22 } { 7 } ( 1024 ) - 768 \sqrt { 3 } = \left( \frac { 22528 } { 7 } - 768 \sqrt { 3 } \right) \mathrm { cm } ^ { 2 }$