Prove that opposite sides of quadrilateral …

Given: ABCD is a quadrilateral circumscribing a circle whose centre is O.
To prove:

1. $\angle$AOB + $\angle$COD = 180^o
2. $\angle$BOC + $\angle$AOD = 180^o

Construction: Join OP, OQ, OR and OS.
 
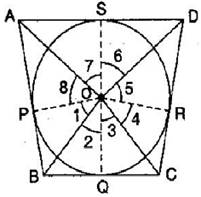
Proof: Since tangents from an external point to a circle are equal.
$\therefore$ AP = AS,
BP = BQ ........ (i)
CQ = CR
DR = DS
In $\triangle$OBP and $\triangle$OBQ,
OP = OQ [Radii of the same circle]
OB = OB [Common]
BP = BQ [From eq. (i)]
$\therefore$ $\triangle$OPB $\cong$ $\triangle$OBQ [By SSS congruence criterion]
$\therefore$ $\angle$1 = $\angle$2 [By C.P.C.T.]
Similarly, $\angle$3 = $\angle$4, $\angle$5 = $\angle$6, $\angle$7 = $\angle$8
Since, the sum of all the angles round a point is equal to 360^o.
$\therefore$ $\angle$1 + $\angle$2 + $\angle$3 + $\angle$4+ $\angle$5 + $\angle$6 + $\angle$7 + $\angle$8 = 360^o
$\Rightarrow$ $\angle$1 + $\angle$1 + $\angle$4 + $\angle$4+ $\angle$5 + $\angle$5 + $\angle$8 + $\angle$8 = 360^o
$\Rightarrow$  2 ($\angle$1 + $\angle$4 + $\angle$5 + $\angle$8)  = 360^o
$\Rightarrow$ $\angle$1 + $\angle$4 + $\angle$5 + $\angle$8 = 180^o
$\Rightarrow$ ($\angle$1 + $\angle$5)  + ($\angle$4 + $\angle$8) = 180^o
$\Rightarrow$ $\angle$AOB + $\angle$COD = 180^o
Similarly we can prove that
$\angle$BOC + $\angle$AOD = 180^o