Two poles of height a meter …

Let AB and CD be two poles of heights a metres and b metres respectively such that the poles are p metres apart i.e. AC= p metres. Suppose the lines AD and BC meet at O such that OL = h metres. Let CL= x and LA = y. Then, x + y = p.
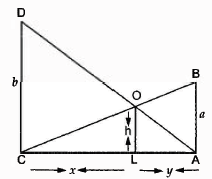
In {tex}\Delta{/tex} ABC and {tex}\Delta{/tex} LOC, we have

{tex}\angle{/tex} CAB = {tex}\angle{/tex}CLO [Each equal to 90°]
{tex}\angle{/tex} C = {tex}\angle{/tex} C [Common]
{tex}\therefore \quad \Delta C A B \sim \Delta C L O{/tex} [By AA-criterion of similarity]
{tex}\Rightarrow \quad \frac { C A } { C L } = \frac { A B } { L O }{/tex}
{tex}\Rightarrow \quad \frac { p } { x } = \frac { a } { h }{/tex} 
{tex}\Rightarrow \quad x = \frac { p h } { a }{/tex} ...(i)
In {tex}\Delta{/tex} ALO and {tex}\Delta{/tex} ACD, we have
{tex}\angle{/tex} ALO = {tex}\angle{/tex}ACD [Each equal to 90°]
{tex}\angle{/tex} A= {tex}\angle{/tex} A [Common]
{tex}\therefore{/tex} {tex}\Delta{/tex}ALO {tex}\sim{/tex} {tex}\Delta{/tex}ACD [By AA-criterion of similarity]
{tex}\Rightarrow \quad \frac { A L } { A C } = \frac { O L } { D C }{/tex}
{tex}\Rightarrow \quad \frac { y } { p } = \frac { h } { b }{/tex}
{tex}\Rightarrow \quad y = \frac { p h } { b }{/tex} [{tex}\because{/tex} AC = x+y+=p]...(ii)
From (i) and (ii), we have
{tex}x + y = \frac { p h } { a } + \frac { p h } { b }{/tex}
{tex}\Rightarrow \quad p = p h \left( \frac { 1 } { a } + \frac { 1 } { b } \right){/tex} [ {tex}\because{/tex} x+y=p]
{tex}\Rightarrow \quad 1 = h \left( \frac { a + b } { a b } \right) \Rightarrow h = \frac { a b } { a + b }{/tex} metres
Hence, the height of the intersection of the lines joining the top of each pole to the foot of the opposite pole is {tex}\frac { a b } { a + b }{/tex} metres.