NCERT Solutions class-11 Maths Exercise 7.3

Exercise 7.3

1. How many 3-digit numbers can be formed by using the digits 1 to 9 if no digits is repeated?

Ans. Total number of digits = 9 and Number of digits used without repetition = 3

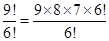Number of permutations = = = 504

---

2. How many 4-digit numbers are there with no digit repeated?

Ans. Total number of digits = 10 and Number of digits used without repetition = 4

0 cannot be filled in the fourth place therefore number of permutations for fourth place = 9

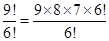Number of permutations = = = 504

Hence total number of permutations = = 4536

---

3. How many 3-digit even numbers can be made using the digits 1, 2, 3, 4, 6, 7 if no digit is repeated?

Ans. Total number of digits = 6 and unit place can be filled with any one of the digits 2, 4, 6.

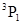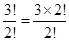Number of permutations = = = 3

Now, the tens and hundreds places can be filled by remaining 5 digits.

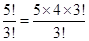Number of permutations = = = 20

Therefore, total number of permutations = = 60

---

4. Find the number of 4-digit numbers that can be formed using the digits 1, 2, 3, 4, 5 if no digit is repeated. How many of these will be even?

Ans. Total number of digits = 5 and Number of digits used without repetition = 4

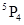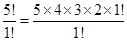Number of permutations = = = 120

Now, unit place can be filled with any one of the digits 2, 4 for even number.

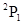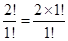Number of permutations = = = 2

Now, the remaining three places can be filled with the remaining 4 digits.

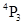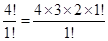Number of permutations = = = 24

Therefore, total number of permutations of even numbers = = 48

---

5. From a committee of 8 persons, in how many ways can we choose a chairman and a vice chairman assuming one person cannot hold more than one position?

Ans. Total number of persons = 8 and Number of persons used without repetition = 2

Number of permutations = = = 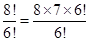56

---

6. Find 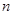if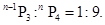

Ans. Given: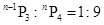

---

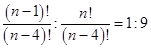
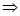
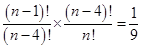
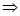
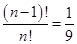
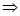
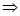
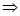
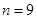
7. Find 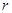if:

(i)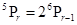

(ii)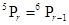

Ans. (i) Given: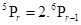

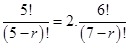
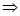
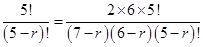
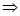
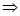
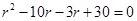
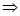
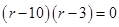
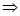
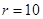or

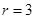
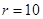is not possible because 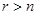, therefore,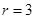

(ii) Given: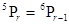

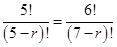
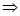
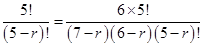
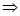
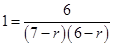
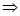
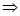
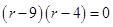
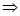
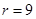or

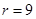is not possible because 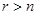, therefore,

---

8. How many words, with or without meaning can be formed using all the letters of the word EQUATION, using each letter exactly once?

Ans. Total number of letters in word EQUATION = 8

Number of letters used (all different) = 8

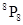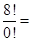
Number of permutations = = = 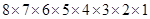40320

---

9. How many words, with or without meaning can be formed using all the letters of the word MONDAY, assuming that no letter is repeated if:

(i) 4 letters are used at a time

(ii) all letters are used at a time

(iii) all letters are used by first letter is a vowel?

Ans. Total number of letters in word MONDAY = 6 and number of vowels = 2

(i) Number of letters used = 4

Number of permutations = 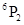= = 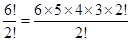360

(ii) Number of letters used = 6

Number of permutations = 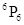= = 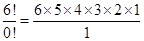720

(iii) First letter is vowel.

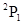Number of permutations of vowels = = = 2

Now, remaining five places can be filled with remaining five letters.

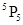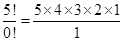Number of permutations = = = 120

Therefore, total number of permutations = 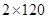= 240

---

10. In how many of the distinct permutations of the letters in MISSISSIPPI do the four I’s not come together?

Ans. Total letters of the word MISSISSIPPI = 11

Here M = 1, I = 4, S = 4 and P =2

Number of permutations = = 34650

When the four I’s come together then it becomes one letter so total number of letters in the word when all I’s come together = 8

Number of permutations = 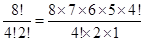= 840

Number of permutations when four I’s do not come together = 34650 – 840 = 33810

---

11. In how many ways can the letters of the word PERMUTATIONS be arranged if the:

(i) words start with P and end with S

(ii) vowels are all together

(iii) There are always 4 letters between P and S?

Ans. Total numbers in the word PERMUTATIONS = 12 and here T = 2

(i) First letter is P and last letter is S which are fixed.

Therefore, remaining 10 letters are to be arranged between P and S.

Number of permutations = = 1814400

(ii) There are 5 vowels in the word PERMUTATIONS. All vowels can be put together.

Number of permutations of all vowels together = 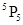= = 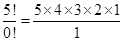120

Now consider the 5 vowels together as one letter. SO the number of letters in the word when all vowels are together = 8

Number of permutations = 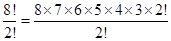= 20160

(iii) P and S are on 1^st and 6^th places P and S are on 2^nd and 7^th places

P and S are on 3^rd and 8^th places P and S are on 4^th and 9^th places

P and S are on 5^th and 10^th places P and S are on 6^th and 11^th places

P and S are on 7^th and 12^th places

Now, P and S can be put in 7 ways and also P and S can interchange their positions.

Number of permutations = 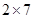= 14

Now the remaining 10 places can be filled with remaining 10 letters.

Number of permutations = = 1814400

Therefore, total number of permutations = 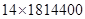= 25401600