Class 10 Basic Maths Sample Paper 2025

Class 10 Basic Maths Sample Paper 2025

Prepare for your exams with the Class 10 Basic Maths Sample Paper 2025 available on myCBSEguide. By solving the Class 10 Basic Maths Sample Paper 2025, you can get familiar with key topics such as arithmetic, algebra, and geometry. Regular practice will boost your problem-solving skills and improve your confidence. Access the latest Model papers and study. Access Mock Papers through the myCBSEguide app, which provides detailed solutions and expert guidance. The Class 10 Basic Maths Sample Paper 2025 is designed to mirror the actual exam format, making it an ideal resource for mock test practice. Stay one step ahead in your Class 10 Basic Maths preparation by using the Class 10 Basic Maths Sample Paper 2025. The Examin8 app allows educators to design high-quality practice tests and exams that cater to their specific teaching requirements, ensuring a streamlined and efficient exam preparation process for students.

Basic Maths has been newly introduced as subject for Class 10 students under the CBSE curriculum. This subject is designed for students who prefer a less advanced mathematical approach, focusing on practical topics. Basic Maths covers essential concepts such as arithmetic, algebra, geometry, and statistics, without the complexity of Standard Maths. Download the myCBSEguide app for Basic Maths sample papers and study resources. And for more Model Papers visit myCBSEGuide Website.

Maths Standard Sample Paper 2025 with Solution Download as PDF

Maths Basic Sample Paper 2025 with Solution Download as PDF

Class 11 Sample Papers Download as PDF

Class 10 – Basic Mathematics
Sample Paper – 01 (2024-25)

---

Maximum Marks: 80
Time Allowed: : 3 hours

---

General Instructions:

Read the following instructions carefully and follow them:

1. This question paper contains 38 questions.
2. This Question Paper is divided into 5 Sections A, B, C, D and E.
3. In Section A, Questions no. 1-18 are multiple choice questions (MCQs) and questions no. 19 and 20 are Assertion- Reason based questions of 1 mark each.
4. In Section B, Questions no. 21-25 are very short answer (VSA) type questions, carrying 02 marks each.
5. In Section C, Questions no. 26-31 are short answer (SA) type questions, carrying 03 marks each.
6. In Section D, Questions no. 32-35 are long answer (LA) type questions, carrying 05 marks each.
7. In Section E, Questions no. 36-38 are case study-based questions carrying 4 marks each with sub-parts of the values of 1,1 and 2 marks each respectively.
8. All Questions are compulsory. However, an internal choice in 2 Questions of Section B, 2 Questions of Section C and 2 Questions of Section D has been provided. An internal choice has been provided in all the 2 marks questions of Section E.
9. Draw neat and clean figures wherever required.
10. Take {tex}\pi=22 / 7{/tex} wherever required if not stated.
11. Use of calculators is not allowed.

---

To prepare effectively for the upcoming exams, practicing with the Class 10 Basic Maths Sample Paper 2025 is essential. These sample papers are designed to help you understand the format of the exam and get a feel for the types of questions that might appear. By solving the 2025 Class 10 Basic Maths Sample Paper, you can identify areas that need improvement and focus on mastering them before the exams.

Section A

1. The product of a rational number and an irrational number is

   a)both rational and irrational number

   b)an integer

   c)an irrational number only

   d)a rational number only

2. The LCM of the smallest prime number and the smallest odd composite number is:

   a)10

   b)9

   c)6

   d)18

3. 4x² – 20x + 25 = 0 have

   a)Real roots

   b)No Real roots

   c)Real and Equal roots

   d)Real and Distinct roots

4. The value of p if (-2, p) lies on the line represented by the equation 2x – 3y + 7 = 0, is

   a)-1

   b){tex}\frac{13}{2}{/tex}

   c){tex}-\frac{13}{2}{/tex}

   d)1

5. The roots of the quadratic equation 4x² – 5x + 4 = 0 are

   a)irrational

   b)rational and equal

   c)rational and distinct

   d)not real

6. If A = (-1, 2), B = (2, -1) and C = (3, 1) are any three vertices of a parallelogram, then find D (a, b)

   a)a = -2, b = 0

   b)a = 2, b = 0

   c)a = -2, b = 6

   d)a = 0, b = 4

7. ABCD is a trapezium such that BC || AD and AB = 4 cm. If the diagonals AC and BD intersect at O such that {tex} \frac{AO}{OC}{/tex} ={tex}\frac{DO}{OB}{/tex} = {tex}\frac{1}{2}{/tex} then, Find DC.

   a)8 cm

   b)6 cm

   c)9 cm

   d)7 cm

8. In a △ABC, it is given that AD is the internal bisector of ∠A. If AB = 10 cm, AC = 14 cm and BC = 6 cm, the CD = ?

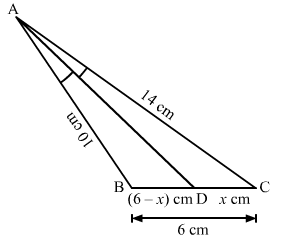
   a)3.5 cm

   b)7 cm

   c)4.8 cm

   d)10.5 cm

9. In the given figure, AB is a tangent to the circle centered at O. If OA = 6 cm and {tex}\angle{/tex}OAB = 30^o, then the radius of the circle is:
   

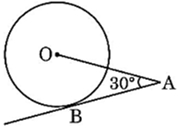
   a){tex}3\sqrt 3{/tex} cm

   b)2 cm

   c){tex}\sqrt 3{/tex} cm

   d)3 cm

10. If 5 tan A = 3, then the value of cot A is:

    a){tex} \frac{3}{4}{/tex}

    b){tex} \frac{5}{3}{/tex}

    c){tex} \frac{3}{5}{/tex}

    d){tex} \frac{4}{5}{/tex}

11. A vertical pole 10 m long casts a shadow of length 5 m on the ground. At the same time, a tower casts a shadow of length 12.5 m on the ground. The height of the tower is:

    a)22 m

    b)25 m

    c)24 m

    d)20 m

12. If {tex}\sin A = \frac{{12}}{{13}}{/tex}, then tan A =

    a){tex}\frac{{13}}{{12}}{/tex}

    b){tex}\frac{{12}}{5}{/tex}

    c){tex}\frac{{13}}{5}{/tex}

    d){tex}\frac{5}{{12}}{/tex}

13. The angle described by the hour hand in 2 hours is

    a)360^o

    b)60^o

    c)90^o

    d)30^o

14. Area of a sector of angle p (in degrees) of a circle with radius R is

    a){tex}\frac { p } { 360 } \times 2 \pi R{/tex}

    b){tex}\frac { \mathrm { p } } { 180 } \times \pi \mathrm { R } ^ { 2 }{/tex}

    c){tex}\frac { p } { 180 } \times 2 \pi \mathrm { R }{/tex}

    d){tex}\frac { p } { 720 } \times 2 \pi R ^ { 2 }{/tex}

15. If a digit is chosen at random from the digits 1, 2, 3, 4, 5, 6, 7, 8, 9, then the probability that it is odd and is a multiple of 3 is

    a){tex}\frac{1}{9}{/tex}

    b){tex}\frac{2}{9}{/tex}

    c){tex}\frac{2}{3}{/tex}

    d){tex}\frac{1}{3}{/tex}

16. The median and mode of distribution are 20 and 18, then the mean is

    a)21

    b)22

    c)20

    d)18

17. A sphere is placed inside a right circular cylinder so as to touch the top, base and lateral surface of the cylinder. If the radius of the sphere is r, then the volume of the cylinder is

    a)2{tex}\pi{/tex}r³

    b)8{tex}\pi{/tex}r³

    c){tex}\frac{8}{3} \pi r^{3}{/tex}

    d)4{tex}\pi{/tex}r³

18. The wickets taken by a bowler in 10 cricket matches are 2, 6, 4, 5, 0, 3, 1, 3, 2, 3. The mode of the data is

    a)1

    b)2

    c)4

    d)3

19. Assertion (A): The points (k, 2 – 2k), (-k +1, 2k) and (-4 – k, 6 – 2k) are collinear if k = {tex}\frac{1}{2}{/tex}
    Reason (R): Three points A, B and C are collinear in the same straight line if AB + BC = AC

    a)Both A and R are true and R is the correct explanation of A.

    b)Both A and R are true but R is not the correct explanation of A.

    c)A is true but R is false.

    d)A is false but R is true.

20. Assertion (A): H.C.F. of smallest prime and smallest composite is 2.
    Reason (R): Smallest prime is 2 and smallest composite is 4 so their H.C.F. is 2.

    a)Both A and R are true and R is the correct explanation of A.

    b)Both A and R are true but R is not the correct explanation of A.

    c)A is true but R is false.

    d)A is false but R is true.

    Maximize your exam preparation by downloading the myCBSEguide app. This all-in-one learning platform provides comprehensive study material for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams. With access to practice questions, sample papers, previous year papers, and detailed solutions, the app helps you study smarter and achieve better results. Get ahead of the competition and prepare efficiently with myCBSEguide.

    Examin8 App: Empower Teachers to Create Custom Exam Papers
    For teachers looking to create personalized assessments, the Examin8 app offers a simple and effective solution. Teachers can easily generate customized question papers with their own name, logo, and branding. The Examin8 app allows educators to design high-quality practice tests and exams that cater to their specific teaching requirements, ensuring a streamlined and efficient exam preparation process for students.

    Familiarity with the exam format is key to performing well, and the Class 10 Basic Maths Sample Paper 2025 is the perfect tool for this. It provides an accurate representation of the structure, question types, and marking scheme of the Basic Maths CBSE Class 10 exam 2025. By practicing with the Sample Paper Class 10 Basic Maths 2025, you will feel more confident and prepared when sitting for your final exams.

21. Section B
22. Solve the following pair of equations by substitution method:
    7x – 15y = 2 …(1)
    x + 2y = 3 …(2)
23. In the given figure,{tex}\frac{{AO}}{{OC}} = \frac{{BO}}{{OD}} = \frac{1}{2}{/tex} and AB = 5cm, Find the value of DC.
    

    OR

    Prove that, In a right triangle, the square on the hypotenuse is equal to the sum of the squares of the other two sides.

24. The length of a tangent from a point A at distance 5 cm from the centre of the circle is 4 cm. Find the radius of the circle.
25. If tan (A + B) = {tex}\sqrt3{/tex} and tan (A – B) = {tex}\frac{1}{\sqrt3}{/tex}; 0° < A + B {tex}\leq{/tex} 90°; A > B, then find A and B.
26. Find the area of a sector of a circle with radius 6 cm, if the angle of the sector is 60^o.

    OR

    An arc of length 15 cm subtends an angle of 45° at the centre of a circle. Find in terms of {tex}\pi{/tex} , the radius of the circle.

27. Section C
28. Show that {tex}5-\sqrt{3}{/tex} is irrational.
29. Find the zeroes of the given quadratic polynomial and verify the relationship between the zeroes and their coefficients for {tex}x^2+x-12{/tex}
30. The ratio of incomes of two persons is 9 : 7 and the ratio of their expenditures is 4 : 3. If each of them manages to save ₹ 2000 per month, then find their monthly incomes.

    OR

    Solve the system of the equation:
    99x + 101y = 499
    101x + 99y = 501

31. The radii of two concentric circles are 13 cm and 8 cm.AB is a diameter of the bigger circle.BD is a tangent to the smaller circle touching it at D. Find the length of AD.
32. In {tex}\triangle A B C{/tex}, right angled at B, if {tex}\tan A = \frac { 1 } { \sqrt { 3 } }{/tex}. Find the value of cos A cos C – sin A sin C

    OR

    If cosec{tex}\theta{/tex} = 2, show that {tex}\left( \cot \theta + \frac { \sin \theta } { 1 + \cos \theta } \right){/tex} = 2.

33. A die is thrown once. What is the probability that it shows
    1. a ‘3’,
    2. a ‘5’,
    3. an odd number
    4. a number greater than 4?
34. Section D
35. The difference of squares of two numbers is 204. The square of the smaller number is 4 less than 10 times the larger number. Find the two numbers.

    OR

    A dealer sells a toy for ₹ 24 and gains as much per cent as the cost price of the toy. Then find the cost price of the toy.38319

36. If a perpendicular is drawn from the vertex containing the right angle to the right angle triangle to the hypotenuse, then prove that the triangle on each side of the perpendicular is similar to each other and to the original triangle. Also, prove that the square of the perpendicular is equal to the product of the lengths of the two parts of the hypotenuse.
37. The interior of a building is in the form of cylinder of diameter 4.3 m and height 3.8 m, surmounted by a cone whose vertical angle is a right angle. Find the area of the surface and the volume of the building. (Use {tex} \pi {/tex} = 3.14).

    OR

    The boilers are used in thermal power plants to store water and then used to produce steam. One such boiler consists of a cylindrical part in middle and two hemispherical parts at its both ends. Length of the cylindrical part is 7m and radius of cylindrical part is {tex}\frac {7} {2}{/tex}m.
    Find the total surface area and the volume of the boiler. Also, find the ratio of the volume of cylindrical part to the volume of one hemispherical part.
    

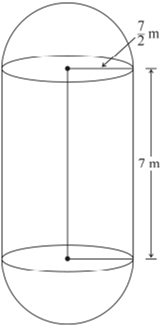
38. The following data gives the distribution of total monthly household expenditure of 200 families of a village. Find the modal monthly expenditure of the families. Also, find the mean monthly expenditure:
    

    Expenditure (in ₹)	Frequency
    1000-1500	24
    1500-2000	40
    2000-2500	33
    2500-3000	28
    3000-3500	30
    3500-4000	22
    4000-4500	16
    4500-5000	7

39. Section E
40. Read the following text carefully and answer the questions that follow:
    Elpis Technology is a TV manufacturer company. It produces smart TV sets not only for the Indian market but also exports them to many foreign countries. Their TV sets have been in demand every time but due to the Covid-19 pandemic, they are not getting sufficient spare parts, especially chips to accelerate the production. They have to work in a limited capacity due to the lack of raw materials.
    
    1. They produced 600 sets in the third year and 700 sets in the seventh year. Assuming that the production increases uniformly by a fixed number every year, find an increase in the production of TV every year. (1)
    2. They produced 600 sets in the third year and 700 sets in the seventh year. Assuming that the production increases uniformly by a fixed number every year, find in which year production of TV is 1000. (1)
    3. They produced 600 sets in the third year and 700 sets in the seventh year. Assuming that the production increases uniformly by a fixed number every year, find the production in the 10th year. (2)
       OR
       They produced 600 sets in the third year and 700 sets in the seventh year. Assuming that the production increases uniformly by a fixed number every year, find the total production in first 7 years. (2)
41. Read the following text carefully and answer the questions that follow:
    There are two routes to travel from source A to destination B by bus. First bus reaches at B via point C and second bus reaches from A to B directly. The position of A, B and C are represented in the following graph:
    Based on the above information, answer the following questions.
    
    Scale: x-axis : 1 unit = 1 km
    y-axis: 1 unit = 1 km
    
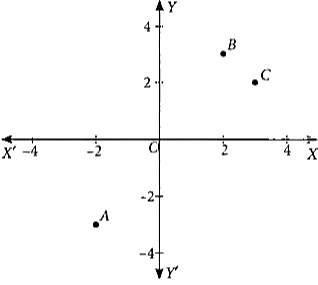
    1. If the fare for the second bus is ₹15/km, then what will be the fare to reach to the destination by this bus? (1)
    2. What is the distance between A and B? (1)
    3. What is the distance between A and C? (2)
       OR
       If it is assumed that both buses have same speed, then by which bus do you want to travel from A to B? (2)
42. Read the following text carefully and answer the questions that follow:
    In a society, there are many multistory buildings. The RWA of the society wants to install a tower and a water tank so that all the households can get water without using water pumps.
    For this they have measured the height of the tallest building in the society and now they want to install a tower that will be taller than that so that the level of water must be higher than the tallest building in their society. Here is one solution they have found and now they want to check if it will work or not.
    From a point on the ground 40 m away from the foot of a tower, the angle of elevation of the top of the tower is 300. the angle of elevation of the top of the water tank is 45^o.
    
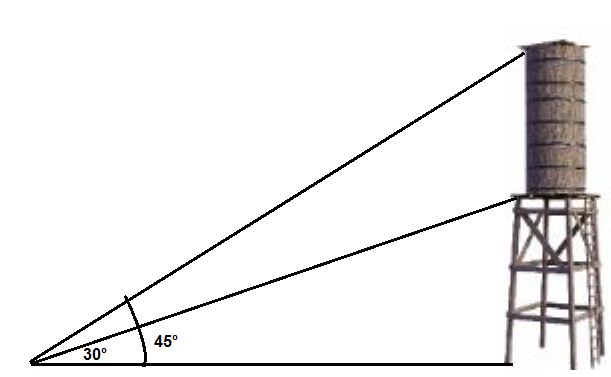
    1. What is the height of the tower? (1)
    2. What is the height of the water tank? (1)
    3. At what distance from the bottom of the tower the angle of elevation of the top of the tower is 45^o. (2)
       OR
       What will be the angle of elevation of the top of the water tank from the place at {tex}\frac{40}{\sqrt{3}}{/tex}m from the bottom of the tower. (2)
       Download the myCBSEguide App for Comprehensive Exam Preparation
       Enhance your exam preparation by downloading the myCBSEguide app. It offers complete study resources for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams, including practice questions, sample papers, and detailed solutions. Stay ahead in your studies with curated materials for every subject. Visit our website myCBSEGuideExamin8 App for Teachers: Create Custom Question Papers
       For educators, the Examin8 app is the perfect tool to design personalized test papers with custom branding, including your own name and logo. Easily create tailored assessments for students, ensuring that each paper meets specific learning objectives. Get started with our website Examin8 today to streamline your teaching process.Use the Class 10 Basic Maths Sample Paper 2025 as a tool to assess your readiness for the final exam. By practicing with these sample papers, you can evaluate your strengths and weaknesses and get a clear idea of how well you are prepared for the actual test. The 2025 Class 10 Basic Maths Sample Paper  is designed to mirror the actual exam format, making it an ideal resource for mock test practice.

Class 10 – Mathematics
Basic Sample Paper – 01 (2024-25)

---

Solutions

1. Section A
2. (a)

   both rational and irrational number

   Explanation:

   The product of a rational number and an irrational number can be either a rational number or an irrational number.
   e.g {tex} \sqrt5{/tex} {tex}\times{/tex} {tex}\sqrt2{/tex} = {tex} \sqrt10{/tex} which is  irrational
   but  {tex} \sqrt8{/tex} {tex}\times{/tex} {tex} \sqrt2{/tex} = {tex} \sqrt16{/tex}  = 4 which is a rational number
   Thus, the product of two irrational numbers can be either rational or irrational
   similarly, the product of rational and irrational numbers can be either rational or irrational
   5 {tex}\times{/tex} {tex} \sqrt2{/tex} = 5{tex} \sqrt2{/tex}  which is irrational.
   but 0 {tex}\times{/tex} {tex} \sqrt3{/tex}  = 0 which is rational.

3. (d)

   18

   Explanation: Smallest prime no. = 2
   Smallest composite no. = 9
   LCM [2, 9] = 2 {tex}\times{/tex} 9 = 18

4. (c)

   Real and Equal roots

   Explanation: D = b² – 4ac
   D = (-20)² – 4 {tex}\times{/tex} 4 {tex}\times{/tex} 25
   D = 400 – 400
   D = 0. Hence Real and equal roots.

5. (d)

   1

   Explanation: 2x – 3y + 7 = 0
   2(-2) -3p + 7 = 0
   3p = 3
   {tex}\Rightarrow{/tex} p = 1

6. (d)

   not real

   Explanation: not real

7. (d)

   a = 0, b = 4

   Explanation: In parallelogram ABCD, diagonals AC and AD bisect each other at O.
   O is mid-point of AC.
   
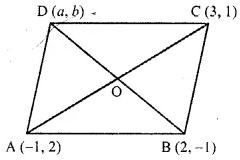
   {tex}\therefore{/tex} Co-ordinates of O will be
   {tex}\left(\frac{-1+3}{2}, \frac{2+1}{2}\right) \text { or }\left(\frac{2}{2}, \frac{3}{2}\right) \text { or }\left(1, \frac{3}{2}\right){/tex}
   {tex}\because{/tex} O is mid-point of BD
   {tex}\therefore \frac{2+a}{2}=1 \text { and } \frac{-1+b}{2}=\frac{3}{2} \Rightarrow 2+a=2{/tex}
   and -1 + b = 3 {tex}\Rightarrow{/tex} b = 3 + 1 = 4
   {tex}\therefore{/tex} a = 0, b = 4

8. (a)

   8 cm

   Explanation:

   

   In ΔAOB and ΔCOD,
   ∠ AOB = ∠COD     (Vertically opposite angles)
   {tex}\frac {AO} {OC}{/tex}= {tex}\frac {DO} {OB}{/tex}   (Given)
   Therefore according to SAS similarity criterion, we have
   ΔAOB {tex}\sim{/tex} ΔCOD
   {tex}\frac {AO}{OC}{/tex}= {tex}\frac {BO}{OD}{/tex} = {tex}\frac {AB}{DC}{/tex}[corresponding sides of similar triangles are proportional] ⇒ {tex}\frac 12{/tex}= {tex}\frac 4{DC}{/tex}
   ⇒ DC = 8 cm

9. (a)

   3.5 cm

   Explanation: By using angle bisector theore in △ABC, we have
   {tex}\frac{\mathrm{AB}}{\mathrm{AC}}=\frac{\mathrm{BD}}{\mathrm{DC}}{/tex}
   {tex}\Rightarrow \frac{10}{14}=\frac{6-x}{x}{/tex}
   {tex}\Rightarrow 10 x=84-14 x{/tex}
   {tex}\Rightarrow 24 x=84 {/tex}
   {tex}\Rightarrow x=3.5{/tex}
   Hence, the correct answer is 3.5.

10. (d)

    3 cm

    Explanation: sin 30^o = {tex}\frac{OB}{OA}{/tex}
    {tex}\frac12{/tex} = {tex}\frac r 6{/tex}
    r = 3 cm

11. (b)

    {tex} \frac{5}{3}{/tex}

    Explanation: 5 tan A = 3
    tan A = {tex}\frac{3}{5}{/tex}
    {tex}\cot A=\frac{1}{\operatorname{tan} A}=\frac{5}{3}{/tex}

12. (b)

    25 m

    Explanation: 25 m

13. (b)

    {tex}\frac{{12}}{5}{/tex}

    Explanation: Let BC = 12cm and AB = 13cm
    
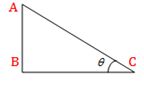
    {tex}\therefore {/tex} AC = {tex}\sqrt {{{\left( {13k} \right)}^2} + {{\left( {12k} \right)}^2}} {/tex} = {tex}\sqrt {169{k^2} – 144{k^2}} {/tex}
    {tex} \Rightarrow {/tex} AC = {tex}\sqrt {25{k^2}} {/tex} = {tex}5k{/tex} 5k
    {tex}\therefore {/tex}{tex}\tan {\text{A}} = \frac{{12k}}{{5k}} = \frac{{12}}{5}{/tex}

14. (b)

    60^o

    Explanation:

    {tex}\because {/tex} Angle described by the hour hand in 12 hours = 360^o
    {tex}\therefore {/tex} Angle described by the hour hand in 2 hours = {tex}\frac{{{{360}^ \circ }}}{{12}}{/tex} {tex}\times{/tex} 2 = 60^o

15. (d)

    {tex}\frac { p } { 720 } \times 2 \pi R ^ { 2 }{/tex}

    Explanation:

    Area of the sector of angle p of a circle with radius R

    {tex}= {\theta \over 360} \times \pi r ^2 = {p \over 360} \times \pi R ^2{/tex}

    {tex}= {p \over 2(360)} \times 2 \pi R ^2 = {p \over 720} \times 2\pi R ^2{/tex}

16. (b)

    {tex}\frac{2}{9}{/tex}

    Explanation:

    Total numbers of digits for 1 to 9(n) = 9
    Number divisible by 3(m) = 3, 6, 9
    Odd numbers out of 3, 6, 9 = 3, 9
    {tex}\therefore{/tex} Probability {tex}=\frac{m}{n}=\frac{2}{9}{/tex}

17. (a)

    21

    Explanation: 3 Median = 2{tex}\times{/tex} Mean + Mode
    {tex} \Rightarrow {/tex} 3 {tex}\times{/tex} 20 = 2{tex}\times{/tex} Mean + 18
    {tex} \Rightarrow {/tex} 2 {tex}\times{/tex}Mean = 60 – 18 = 42
    {tex} \Rightarrow {/tex} Mean = 21

18. (a)

    2{tex}\pi{/tex}r³

    Explanation: Volume of a sphere = (4/3){tex}\pi{/tex}r³
    Volume of a cylinder = {tex}\pi{/tex}r²h
    Given, sphere is placed inside a right circular cylinder so as to touch the top, base and lateral surface of the cylinder and the radius of the sphere is r.
    Thus, height of the cylinder = diameter = 2r and base radius = r
    Volume of the cylinder = {tex}\pi{/tex} {tex}\times{/tex} r² {tex}\times{/tex} 2r = 2{tex}\pi{/tex}r³

19. (d)

    3

    Explanation: In the given data, the frequency of 3 is more than those other wickets taken by a bowler.
    Therefore, Mode of given data is 3.

20. (a)

    Both A and R are true and R is the correct explanation of A.

    Explanation:

    Both A and R are true and R is the correct explanation of A.

21. (a)

    Both A and R are true and R is the correct explanation of A.

    Explanation: Smallest prime is 2 and the smallest composite is 4 so H.C.F. of 2 and 4 is 4.

    Download the myCBSEguide App for Comprehensive Exam Preparation
    For effective exam preparation, download the myCBSEguide app, which offers complete study material for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams. With a vast collection of practice questions, sample papers, and chapter-wise solutions, this app is your one-stop resource to study smarter and achieve better results.

    Examin8 App: Empower Teachers to Create Custom Papers
    Teachers can enhance their teaching and assessment process using the Examin8 app. This powerful tool allows educators to design customized exam papers with their name, logo, and branding. Whether for practice tests or final assessments, Examin8 Website provides an easy solution for creating professional, personalized question papers.

    An effective exam strategy can be developed by practicing with the Class 10 Basic Maths Sample Paper 2025. Solving sample papers not only helps you learn the material but also improves your time-management skills. The Class 10 Basic Maths Sample Paper 2025 will help you understand how to allocate time for each section, ensuring that you can complete your exam within the given time frame.

22. Section B
23. Step 1: By substitution method, we pick either of the equations and write one variable in terms of the other.
    7x – 15y = 2 …(1)
    and x + 2y = 3 …(2)
    Let us consider the Equation (2):
    x + 2y = 3
    and write it as x = 3 – 2y …(3)
    Step 2: Now substitute the value of x in Equation (1)
    We get 7(3 – 2y) – 15y = 2
    i.e., 21 – 14y – 15y = 2
    i.e., – 29y = –19
    Therefore {tex}\mathrm{y}=\frac{19}{29}{/tex}
    Step 3: Substituting this value of y in Equation (3), we get
    {tex}\mathrm{x}=3-2\left(\frac{19}{29}\right)=\frac{49}{29}{/tex}
    Therefore, the solution is x = {tex}\frac{49}{29}, \mathrm{y}=\frac{19}{29}{/tex}
24. In ​{tex}\triangle {/tex}AOB and ​{tex}\triangle {/tex}​COD
    ​{tex}\angle{/tex}​ AOB = ​{tex}\angle{/tex}​ COD [Vertically opposite angles] {tex}\frac{{AO}}{{OC}} = \frac{{BO}}{{OD}} \Rightarrow \frac{{AO}}{{OB}} = \frac{{OC}}{{OD}}{/tex}[Given] ​{tex}\therefore {/tex}​ ​{tex}\triangle {/tex}​AOB ​{tex} \sim {/tex}​ {tex}\triangle {/tex}COD [By SAS similarity] ​{tex}\therefore {/tex}​{tex}\frac{{AO}}{{OC}} = \frac{{BO}}{{OD}} = \frac{{AB}}{{CD}}{/tex}
    {tex}\frac{1}{2} = \frac{{AB}}{{DC}}\left[ {\frac{{AO}}{{OC}} = \frac{{BO}}{{OD}} = \frac{1}{2}} \right]{/tex}is given
    ​{tex}\Rightarrow {/tex}​ {tex}\frac{1}{2} = \frac{5}{{DC}}{/tex}
    {tex}\Rightarrow {/tex} DC = 10 cm

    OR

    Given : {tex}\triangle \mathrm{ABC}{/tex} is right angle at B
    To prove:  {tex}A C^{2}=A B^{2}+B C^{2}{/tex}
    

    Construction: Draw {tex}B D \perp A C{/tex}

    Proof:In {tex}\triangle{/tex}ABC and {tex}\triangle{/tex}ABD

    {tex}\angle{/tex}ABC={tex}\angle{/tex}ADB=90

    {tex}\angle{/tex}A is common

    {tex}\angle{/tex}ABC{tex}\sim{/tex}{tex}\angle{/tex}ADB
    {tex}\Rightarrow \frac{A D}{A B}=\frac{A B}{A C}{/tex}
    {tex}\Rightarrow{/tex} AD. AC = AB² …(i)
    Similarly {tex}\Delta \mathrm{BDC} \sim \Delta \mathrm{ABC}{/tex}
    {tex}\Rightarrow \frac{C D}{B C}=\frac{B C}{A C}{/tex}
    {tex}\Rightarrow{/tex} CD.AC = BC² …(ii)
    Adding (i) and (ii)
    AD.AC + CD. AC= AB² + BC²
    AC(AD + CD) = AB² + BC²
    AC {tex}\times{/tex} AC = AB² + BC²
    AC² = AB² + BC²
    Hence Proved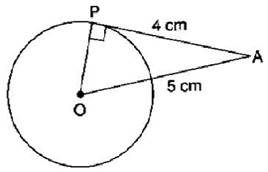

25. 
    We know that the tangent at any point of a circle is {tex}\perp{/tex} to the radius through the point of contact.
    {tex}\therefore{/tex} {tex}\angle{/tex}OPA = 90^o
    {tex}\therefore{/tex} OA² = OP² + AP² [By Pythagoras theorem] {tex}\Rightarrow{/tex}  (5)² = (OP)² + (4)²
    {tex}\Rightarrow{/tex}  25 = (OP)² + 16
    {tex}\Rightarrow{/tex}  OP² = 9
    {tex}\Rightarrow{/tex}  OP = 3 cm
26. We have,tan (A + B) = {tex}\sqrt3{/tex}{tex}\Rightarrow{/tex}tan(A+B) = tan 60°
    A + B = 60°……….(i)
    Again, tan (A – B ) ={tex}\frac{1}{\sqrt3}{/tex}{tex}\Rightarrow{/tex}tan(A-B) = tan 30°
    A – B = 30°……….(ii)
    Adding, (i) and (ii)
    2A = 90°
    {tex}\therefore{/tex} A = {tex}\frac{90^\circ}{2}{/tex} =45°
    Putting A=45{tex}^o{/tex} in equation (i),
    B = 60° – A = 60° – 45° = 15°
    Therefore,A = 45° and B = 15°.
27.  
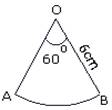
    We know that Area of sector ={tex}\frac { \theta } { 360 } \pi r ^ { 2 }{/tex}
    Here, {tex} \theta = 60 , r = 6{/tex}
    {tex}\therefore{/tex} Required area {tex}= \frac { 60 } { 360 } \times \frac { 22 } { 7 } \times ( 6 ) ^ { 2 }{/tex}
    {tex}= \frac { 1 } { 6 } \times \frac { 22 } { 7 } \times 36{/tex}
    {tex}= \frac { 22 \times 6 } { 7 }{/tex}
    {tex}= \frac { 132 } { 7 } = 18 \frac { 6 } { 7 } c m ^ { 2 }{/tex}

    OR

    Length of arc = 15cm
    {tex}\theta = 45^\circ {/tex}
    {tex}\therefore {/tex} length of arc = {tex}\frac{\theta }{{360^\circ }} \times 2\pi r{/tex}
    {tex} \Rightarrow 15 = \frac{{45^\circ }}{{360^\circ }} \times 2 \times \pi \times r{/tex}
    {tex}\Rightarrow r = \frac{{15 \times 360^\circ }}{{45^\circ \times 2 \times \pi }}{/tex}
    {tex} \Rightarrow r = \frac{{60}}{\pi }cm{/tex}

28. Section C
29. Let us assume, to the contrary, that {tex}5-\sqrt{3}{/tex} is rational.
    That is, we can find coprime numbers a and b (b ≠ 0) such that {tex}5-\sqrt{3}=\frac{a}{b}{/tex}
    Therefore, {tex}5-\frac{a}{b}=\sqrt{3}{/tex}
    Rearranging this equation, we get {tex}\sqrt{3}=5-\frac{a}{b}=\frac{5 b-a}{b}{/tex}
    Since a and b are integers, we get {tex}5-\frac{a}{b}{/tex} is rational, and so {tex}\sqrt{3}{/tex} is rational.
    But this contradicts the fact that {tex}\sqrt{3}{/tex} is irrational
    This contradiction has arisen because of our incorrect assumption that {tex}5-\sqrt{3}{/tex} is rational.
    So, we conclude that {tex}5-\sqrt{3}{/tex} is irrational.
30. x² + x – 12
    Let p(x) = x² + x – 12
    For zeroes of p(x), p(x) = 0
    {tex}\Rightarrow x ^ { 2 } + x – 12 = 0{/tex}
    {tex}\Rightarrow x ^ { 2 } + 4 x – 3 x – 12 = 0{/tex}
    By the method of splitting the middle term
    {tex}\Rightarrow x ( x + 4 ) – 3 ( x + 4 ) = 0{/tex}
    {tex}\Rightarrow ( x + 4 ) ( x – 3 ) = 0{/tex}
    {tex}\Rightarrow x + 4 = 0 \text { or } x – 3 = 0{/tex}
    {tex}\Rightarrow x = – 4 \text { or } x = 3{/tex}
    x = -4, 3
    So, the zeroes of x² + x – 12 are -4 and 3.
    Sum of zeroes
    {tex}= ( – 4 ) + 3 = – 1 = \frac { – 1 } { 1 } = \frac { – \text { Coefficient of } \mathrm { x } } { \text { Coefficient of } \mathrm { x } ^ { 2 } }{/tex}
    Product of its zeroes
    {tex}= ( – 4 ) \times ( 3 ) = – 12 = \frac { – 12 } { 1 } = \frac { \text { Constant term } } { \text { Coefficient of } \mathrm { x } ^ { 2 } }{/tex}Hence the relation between zeroes and coefficient is verified.
31. Let us denote the incomes of the two-person by ₹ 9x and ₹ 7x and their expenditures by ₹ 4y and ₹ 3y respectively.
    Then the equations formed in the situation is given by :
    9x – 4y = 2000 …(i)
    and 7x – 3y = 2000 …(2)
    Step 1: Multiply Equation (1) by 3 and Equation (2) by 4 to make the coefficients of y equal. Then, we get the equations:
    27x – 12y = 6000 …(3)
    28x – 12y = 8000 …(4)
    Step 2: Subtract Equation (3) from Equation (4) to eliminate y, because the coefficients of y are the same. So, we get
    (28x – 27x) – (12y – 12y) = 8000 – 6000
    i.e., x = 2000
    Step 3: Substituting this value of x in (1), we get
    9(2000) – 4y = 2000
    i.e., y = 4000
    So, the solution of the equations is x = 2000, y = 4000. Therefore, the monthly incomes of the persons are ₹18,000 and ₹14,000 respectively.

    OR

    Given, 99x + 101y = 499 ….(i)
    101x + 99y = 501… (ii)
    Adding eqn. (i) and (ii),
    ( 99x + 101y ) + (101x + 99y ) = 499 + 501
    99x + 101y + 101x + 99y = 1000
    200x + 200y = 1000
    x + y = 5 …(iii)
    Subtracting eqn. (ii) from eqn. (i), we get
    ( 99x + 101y ) – (101x + 99y ) = 499 – 501
    99x + 101y – 101x – 99y = -2
    -2x + 2y = – 2
    or, x – y= 1 …….. (iv)
    Adding equations (iii) and (iv)
    x + y + x – y = 5 + 1
    2x = 6
    {tex}\therefore {/tex} x = 3
    Substituting the value of x in eqn. (iii), we get
    3 + y = 5
    y = 2
    Hence the value of x and y of given equation are 3 and 2 respectively.

32. Produce BD to meet the bigger circle at E. Join AE.
    
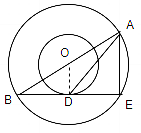
    Then, {tex}\angle AEB=90^o{/tex}[ angle in semi-circle] Clearly, {tex}OD\bot BE{/tex}
    Now, in {tex}\triangle AEB{/tex}, O and D are the mid-points of AB and BE, respectively.
    Therefore,by mid-point theorem, we have
    {tex}OD=\frac{1}{2}AE{/tex}
    {tex}\Rightarrow AE=2\times 8=16\;cm{/tex} [OD = radius of smaller circle = 8 cm ] In right-angled {tex}\triangle ODB{/tex},
    {tex}OB^2=OD^2+BD^2{/tex} [By pythagoras theorem] {tex}\Rightarrow BD^2=169-64=105{/tex}
    {tex}\Rightarrow BC=\sqrt{105}\;cm=DE{/tex} [BD = DE] Now, in right-angled {tex}\triangle AED,{/tex}
    {tex}AD^2=AE^2+ED^2{/tex} [by pythagoras theorem] {tex}\Rightarrow AD=\sqrt{(16)^2+(\sqrt{105})^2}=19\;cm{/tex}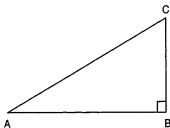
33. 
    we have,
    {tex}\tan A = \frac { 1 } { \sqrt { 3 } }{/tex}{tex}= tan30^\circ{/tex}
    {tex}\therefore A = 30^\circ{/tex}
    In {tex}\triangle{/tex}ABC, we have
    {tex}\angle A+\angle B+\angle C=180^\circ{/tex}
    {tex}\Rightarrow30^\circ+90^\circ+\angle C=180^\circ{/tex}
    {tex}\Rightarrow120^\circ+\angle C=180^\circ{/tex}
    {tex}\Rightarrow\angle C=180^\circ-120^\circ=60^\circ{/tex}
    So,
    cos A.cos C – sin A.sin C
    {tex}=\cos30^\circ.\cos60^\circ-\sin30^\circ.\sin60^\circ{/tex}
    {tex}=\frac{\sqrt3}2\cdot\frac12-\frac12\cdot\frac{\sqrt3}2=0{/tex}

    OR

    Let us draw a triangle ABC in which {tex}\angle{/tex}B = 90°.
    
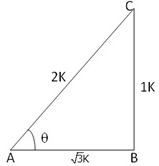
    Let {tex}\angle{/tex}A = {tex}\theta{/tex}°.
    Then, {tex}\text{cosec} \theta = \frac { A C } { B C } = \frac { 2 } { 1 }{/tex}
    Let BC = 1k and AC = 2k, where k is positive.
    By Pythagoras’ theorem, we have
    AC² = AB² + BC²
    {tex}\Rightarrow{/tex}AB² = AC² – BC²
    = (2k)² – (1k)² = 4k² – 1k² = 3k²
    {tex}\Rightarrow \quad B C = \sqrt 3k{/tex}
    {tex}\sin \theta = \frac { B C } { A C } = \frac { 1 k } { 2 k } = \frac { 1 } { 2 }{/tex}
    {tex}\cos \theta = \frac { A B } { A C } = \frac { \sqrt { 3 k } } { 2 k } = \frac { \sqrt { 3 } } { 2 }{/tex}
    {tex}\cot \theta = \frac { \cos \theta } { \sin \theta } = \left( \frac { \sqrt { 3 } } { 2 } \times \frac { 2 } { 1 } \right) = \sqrt { 3 }{/tex}
    {tex}\Rightarrow \left[ \cot \theta + \frac { \sin \theta } { 1 + \cos \theta } \right] = \left[ \sqrt { 3 } + \frac { \frac { 1 } { 2 } } { 1 + \frac { \sqrt { 3 } } { 2 } } \right]{/tex}
    {tex}= \left( \sqrt { 3 } + \frac { 1 } { 2 + \sqrt { 3 } } \right) = \left( \frac { 2 \sqrt { 3 } + 3 + 1 } { 2 + \sqrt { 3 } } \right){/tex}
    {tex}= \left( \frac { 2 \sqrt { 3 } + 4 } { 2 + \sqrt { 3 } } \right) = 2 \left( \frac { \sqrt { 3 } + 2 } { 2 + \sqrt { 3 } } \right) = 2{/tex}
    Hence, {tex}\left[ \cot \theta + \frac { \sin \theta } { 1 + \cos \theta } \right] ={/tex}2

34. When we throw a die, possible outcomes are 1,2,3,4,5 and 6.
    1. Let E₁ be the event of getting a 3.
       Then, the number of favourable outcomes = 1.
       The favourable outcome is 3.
       {tex}\therefore{/tex} P(getting a 3) = P(E₁) {tex}= \frac { 1 } { 6 }{/tex}.
    2. Let E₂ be the event of getting a 5
       Then, the number of favourable outcomes = 1.
       The favourable outcome is 5.
       {tex}\therefore{/tex}  P(getting a 5) = P(E₂) {tex}= \frac { 1 } { 6 }{/tex}.
    3. Let E₃ be the event of getting an odd number.
       Number of favourable outcomes = 3.
       The favourable outcomes are 1,3,5.
       {tex}\therefore{/tex} P(getting an odd number)={tex}P \left( E _ { 3 } \right) = \frac { 3 } { 6 } = \frac { 1 } { 2 }{/tex}.
    4. Let E₄ be the event of getting a number greater than 4.
       Number of favourable outcomes = 2.
       Then, the favourable outcomes are 5,6.
       {tex}\therefore{/tex} P(getting a number greater than 4) = P(E₄){tex}= \frac { 2 } { 6 } = \frac { 1 } { 3 }{/tex}.
35. Section D
36. Let the numbers are x and (x > y)
    x² – y² = 204 …(i)
    y² = 10x – 4 …(ii)
    By (i) and (ii)
    x² – 10x + 4 – 204 = 0
    x² – 10x + 200 = 0
    (x – 20) (x + 10) = 0
    x = 20, x = -10 (rejected)
    y = 14

    OR

    According to question a dealer sells a toy for Rs. 24 and gains as much per cent as the cost price of the toy.
    Let the cost price of the toy be Rs. x.
    Then, Gain = x%
    {tex} \Rightarrow{/tex} Gain = Rs. {tex} \left( x \times \frac { x } { 100 } \right){/tex} = Rs. {tex} \frac { x ^ { 2 } } { 100 }{/tex}.
    Therefore, S.P. = C.P. + Gain = {tex} x + \frac { x ^ { 2 } } { 100 }{/tex}.
    But, S.P. =Rs. 24.
    {tex} \therefore \quad x + \frac { x ^ { 2 } } { 100 } = 24{/tex} [Given] {tex} \Rightarrow{/tex} 100x + x² = 2400
    {tex} \Rightarrow{/tex} x² + 100x – 2400 = 0
    {tex} \Rightarrow{/tex} x² + 120x -20x -2400 = 0
    {tex} \Rightarrow \quad x ( x + 120 ) – 20 ( x + 120 ) = 0{/tex}
    {tex} \Rightarrow{/tex} (x + 120) (x – 20) = 0
    {tex} \Rightarrow{/tex} x = 20, -120
    {tex} \Rightarrow{/tex} x = 20 [because x > 0 ] Hence, the cost price of the toy is Rs. 20.

37. GIVEN A right triangle ABC right angled at B i.e. {tex}B D \perp A C.{/tex}
    PROOF We have,
    
    {tex}\angle{/tex}ABD + {tex}\angle{/tex}DBC = 90°
    Also, {tex}\angle{/tex}C + {tex}\angle{/tex}DBC + {tex}\angle{/tex}BDC = 180°
    {tex}\Rightarrow{/tex} {tex}\angle{/tex}C + {tex}\angle{/tex}DBC + 90° = 180°
    {tex}\Rightarrow{/tex} {tex}\angle{/tex}C + {tex}\angle{/tex}DBC = 90°
    But, {tex}\angle{/tex}ABD + {tex}\angle{/tex}DBC = 90°
    {tex}\therefore \quad \angle A B D + \angle D B C = \angle C + \angle D B C{/tex}
    {tex}\Rightarrow \quad \angle A B D = \angle C{/tex} …(i)
    Thus, in {tex}\Delta{/tex}ADB and {tex}\Delta{/tex}BDC, we have
    {tex}\angle{/tex}ABD = {tex}\angle{/tex}C [From (i)] and, {tex}\angle{/tex}ADB = {tex}\angle{/tex}BDC [Each equal to 90°] So, by AA-similarity criterion, we obtain {tex}\Delta A D B \sim \Delta B D C.{/tex}  ….(ii)
    In {tex}\Delta{/tex}ADB and {tex}\Delta{/tex}ABC, we have
    {tex}\angle{/tex}ADB = {tex}\angle{/tex}ABC [Each equal to 90°] and, {tex}\angle{/tex}A =  {tex}\angle{/tex}A [Common] So, by AA-similarity criterion, we obtain {tex}\Delta{/tex}ADB ~ {tex}\Delta{/tex}ABC …..(iii)
    In {tex}\Delta{/tex}BDC and {tex}\Delta{/tex}ABC, we have
    {tex}\angle{/tex}BDC = {tex}\angle{/tex}ABC [Each equal to 90°] {tex}\angle{/tex}C = {tex}\angle{/tex}C [Common] So, by AA – similarity criterion, we obtain {tex}\Delta{/tex}BDC ~ {tex}\Delta{/tex}ABC ……(iv)
    From (ii), we have
    {tex}\Delta{/tex}ADB ~ {tex}\Delta{/tex}BDC
    {tex}\Rightarrow \quad \frac { A D } { B D } = \frac { B D } { D C }{/tex}
    {tex}\Rightarrow \quad B D ^ { 2 } = A D \times D C{/tex}
38. r₁ = Radius of the base of the cylinder = {tex}\frac{4.3}{2}{/tex} m = 2.15 m
    {tex}\therefore{/tex} r₂ = Radius of the base of the cone = 2.15 m, h₁ = Height of the cylinder = 3.8 m
    
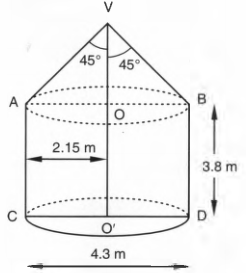
    In {tex}\triangle{/tex}VOA, we have
    {tex}\sin 45^{\circ}=\frac{O A}{V A} \Rightarrow \frac{1}{\sqrt{2}}=\frac{2.15}{V A} \Rightarrow V A=(\sqrt{2} \times 2.15) \mathrm{m}=(1.414 \times 2.15) \mathrm{m}=3.04 \mathrm{m}{/tex}
    Clearly, {tex}\triangle{/tex}VOA is an isosceles triangle. Therefore, VO = OA = 2.15 m
    Thus, we have
    h₂ = Height of the cone = VO = 2.15 m, l₂ = Slant height of the cone = VA = 3.04 m
    Let S be the Surface area of the building. Then,
    {tex}\Rightarrow{/tex} S = Surface area of the cylinder + Surface area of cone
    {tex}\Rightarrow{/tex} S = (2{tex}\pi{/tex}r₁h₁ + {tex}\pi{/tex}r₂l₂) m²
    {tex}\Rightarrow{/tex} S = (2{tex}\pi{/tex}r₁h₁ + {tex}\pi{/tex}r₁l₂) m² [{tex}\because{/tex} r₁ = r₂ – 2.15 m] {tex}\Rightarrow{/tex} S = {tex}\pi{/tex}r₁(2h₁ + l₂) m²
    {tex}\Rightarrow{/tex} S = 3.14 {tex}\times{/tex} 2.15 {tex}\times{/tex} (2 {tex}\times{/tex} 3.8 + 3.04) m² = 3.14 {tex}\times{/tex} 2.15 {tex}\times{/tex} 10.64 m² = 71.83 m²
    Let U be the volume of the building. Then,
    V = Volume of the cylinder + Volume of the cone
    {tex}\Rightarrow \quad V=\left(\pi r_{1}^{2} h_{1}+\frac{1}{3} \pi r_{2}^{2} h_{2}\right) \mathrm{m}^{3}{/tex}
    {tex}\Rightarrow \quad V=\left(\pi r_{1}^{2} h_{1}+\frac{1}{3} \pi r_{1}^{2} h_{2}\right) \mathrm{m}^{3} \quad\left[\because r_{2}=r_{1}\right]{/tex}
    {tex}\Rightarrow \quad V=\pi r_{1}^{2}\left(h_{1}+\frac{1}{3} h_{2}\right) \mathrm{m}^{3}{/tex}
    {tex}\Rightarrow \quad V=3.14 \times 2.15 \times 2.15 \times\left(3.8+\frac{2.15}{3}\right) \mathrm{m}^{3}{/tex}
    {tex}\Rightarrow \quad V=[3.14 \times 2.15 \times 2.15 \times(3.8+0.7166)] \mathrm{m}^{3}{/tex}
    {tex}\Rightarrow \quad V=(3.14 \times 2.15 \times 2.15 \times 4.5166) \mathrm{m}^{3}=65.55 \mathrm{m}^{3}{/tex}

    OR

    Given that,
    
    Length of cylindrical part = 7 m
    Radius of cylindrical part = {tex}\frac{7}{2}{/tex} m
    Total surface area of figure = 2{tex}\pi{/tex}rh + 2(2{tex}\pi{/tex}r²)
    = {tex}2 \pi\left[\frac{7}{2} \times 7+2 \times\left(\frac{7}{2}\right)^2\right]{/tex}
    = 308 m²
    Volume of boiler = Volume of cylindrical part + Volume of two hemispherical parts
    = {tex}\pi{/tex}r²h {tex}+\left(\frac{4}{3}\right) \pi r^3{/tex}
    = {tex}\pi\left(\frac{7}{2}\right)^2 \times(7)+\left(\frac{4}{3}\right) \pi\left(\frac{7}{2}\right)^3{/tex}
    = 269.5 + 179.66
    = 449.167 m³
    Required ratio = {tex}\frac{\text { Volume of cylindrical part }}{\text { Volume of one hemispherical part }}{/tex}
    = {tex}\frac{269.5}{89.83}{/tex}
    = 3

39. We may observe from the given data that maximum class frequency is 40 belonging to 1500 – 2000 interval.
    Class size (h) = 500
    Mode = l + {tex} \frac { f – f _ { 1 } } { 2 f – f _ { 1 } – f _ { 2 } } \times{/tex} h
    Lower limit {tex}(l) {/tex}of modal class = 1500
    Frequency (f) of modal class = 40
    Frequency (f₁) of class preceding modal class = 24
    Frequency (f₂) of class succeeding modal class = 33
    mode = 1500 + {tex}\frac { 40 – 24 } { 2 \times 40 – 24 – 33 } \times{/tex} 500
    = 1500 + {tex} \frac { 16 } { 80 – 57 } \times{/tex} 500
    = 1500 + 347.826
    = 1847.826 ≈ 1847.83

    Expenditure (in ₹.)	Number of families fi	xi	di = xi – 2750	ui	uifi
    1000-1500	24	1250	-1500	-3	-72
    1500-2000	40	1750	-1000	-2	-80
    2000-2500	33	2250	-500	-1	-33
    2500-3000	28	2750=a	0	0	0
    3000-3500	30	3250	500	1	30
    3500-4000	22	3750	1000	2	44
    4000-4500	16	4250	1500	3	48
    4500-5000	7	4750	2000	4	28
    	{tex}\Sigma f _ { i }{/tex}  = 200				{tex}\Sigma f _ { i } d _ { i }{/tex}  = – 35

    Mean {tex}\overline { x } = a + \frac { \Sigma f _ { i } d _ { i } } { \Sigma f _ { i } } \times{/tex} h
    {tex}\overline { x }{/tex} = 2750 + {tex}\frac { – 35 } { 200 } \times{/tex} 500
    {tex}\overline { x }{/tex}  = 2750 – 87.5
    {tex}\overline { x } {/tex} = 2662.5

40. Section E
    1. Since the production increases uniformly by a fixed number every year. Therefore, the sequence formed by the production in different years is an A.P. Let a be the first term and d be the common difference of the A.P. formed i.e., ‘a’ denotes the production in the first year and d denotes the number of units by which the production increases every year.
       We have, a₃ = 600 and
       a₃ = 600
       {tex}\Rightarrow{/tex} 600 = a + 2d
       {tex}\Rightarrow{/tex} a = 600 – 2d …(i)
       {tex}\Rightarrow{/tex} a₇ = 700
       {tex}\Rightarrow{/tex} a₇ = 700
       {tex}\Rightarrow{/tex} 700 = a + 6d
       {tex}\Rightarrow{/tex} a = 700 – 6d …(ii)
       From (i) and (ii)
       600 – 2d = 700 – 6d
       {tex}\Rightarrow{/tex} 4d = 100
       {tex}\Rightarrow{/tex} d = 25
    2. Since the production increases uniformly by a fixed number every year. Therefore, the sequence formed by the production in different years is an A.P. Let a be the first term and d be the common difference of the A.P. formed i.e., ‘a’ denotes the production in the first year and d denotes the number of units by which the production increases every year.
       We know that first term = a = 550 and common difference = d = 25
       a_n = 1000
       {tex}\Rightarrow{/tex} 1000 = a + (n – 1)d
       {tex}\Rightarrow{/tex} 1000 = 550 + 25n – 25
       {tex}\Rightarrow{/tex} 1000 – 550 + 25 = 25n
       {tex}\Rightarrow{/tex} 475 = 25n
       {tex}\Rightarrow{/tex} n = {tex}\frac{475}{25}{/tex} = 19
    3. Since the production increases uniformly by a fixed number every year. Therefore, the sequence formed by the production in different years is an A.P. Let a be the first term and d be the common difference of the A.P. formed i.e., ‘a’ denotes the production in the first year and d denotes the number of units by which the production increases every year.
       The production in the 10th term is given by a_10. Therefore, production in the 10th year = a₁₀ = a + 9d = 550 + 9 {tex}\times{/tex} 25 = 775. So, production in 10th year is of 775 TV sets.
       OR
       Since the production increases uniformly by a fixed number every year. Therefore, the sequence formed by the production in different years is an A.P. Let a be the first term and d be the common difference of the A.P. formed i.e., ‘a’ denotes the production in the first year and d denotes the number of units by which the production increases every year.
       Total production in 7 years = Sum of 7 terms of the A.P. with first term a (= 550) and d (= 25).
       {tex}S_n=\frac{n}{2}[2 a+(n-1) d]{/tex}
       {tex}\Rightarrow S_7=\frac{7}{2}[2 \times 550+(7-1) 25]{/tex}
       {tex}\Rightarrow S_7=\frac{7}{2}[2 \times 550+(6) \times 25]{/tex}
       {tex}\Rightarrow S_7=\frac{7}{2}[1100+150]{/tex}
       {tex}\Rightarrow{/tex} S₇ = 4375
    1. Distance travelled by second bus = 7.2 km
       {tex}\therefore{/tex} Total fare = 7.2 {tex}\times{/tex} 15 = ₹08
    2. Required distance = {tex}\sqrt{(2+2)^{2}+(3+3)^{2}}{/tex}
       {tex}= \sqrt{4^{2}+6^{2}}=\sqrt{16+36}{/tex} {tex}= 2 \sqrt{13}{/tex} km {tex}\approx{/tex} 7.2 km
    3. Required distance = {tex}\sqrt{(3+2)^{2}+(2+3)^{2}}{/tex}
       = {tex}\sqrt{5^{2}+5^{2}}=5 \sqrt{2}{/tex} km
       OR
       Distance between B and C
       = {tex}\sqrt{(3-2)^{2}+(2-3)^{2}}{/tex} = {tex}\sqrt{1+1}=\sqrt{2}{/tex} km
       Thus, distance travelled by first bus to reach to B
       = AC + CB = {tex}5 \sqrt{2}+\sqrt{2}=6 \sqrt{2}{/tex} km {tex}\approx{/tex} 8.48 km
       and distance travelled by second bus to reach to B
       = AB = {tex}2 \sqrt{13}{/tex} km = 7.2 km
       {tex}\therefore{/tex} Distance of first bus is greater than distance of the cond bus, therefore second bus should be chosen.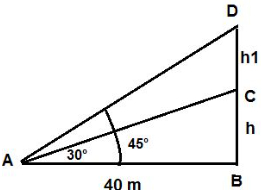
    4. 
       Let BC be the tower of height h  and CD be the water tank of height h₁
       In {tex}\Delta{/tex}ABD, we have
       {tex}\tan 45 ^ { \circ } = \frac { B D } { A B }{/tex}
       {tex}\Rightarrow 1 = \frac { h + h _ { 1 } } { 40 }{/tex}
       {tex}\Rightarrow h + h _ { 1 } = 40{/tex} …(1)
       In {tex}\Delta A B C,{/tex} we have
       {tex}\tan 30 ^ { \circ } = \frac { B C } { A B }{/tex}
       {tex}\Rightarrow \quad \frac { 1 } { \sqrt { 3 } } = \frac { h } { 40 }{/tex}
       {tex}\Rightarrow h = \frac { 40 } { \sqrt { 3 } } = \frac { 40 \sqrt { 3 } } { 3 } {/tex} = 23.1 m
       Thus height of the tower is 23.1 m.
    5. 
       Let BC be the tower of height h  and CD be the water tank of height h₁
       In {tex}\Delta{/tex}ABD, we have
       {tex}\tan 45 ^ { \circ } = \frac { B D } { A B }{/tex}
       {tex}\Rightarrow 1 = \frac { h + h _ { 1 } } { 40 }{/tex}
       {tex}\Rightarrow h + h _ { 1 } = 40{/tex} …(1)
       In {tex}\Delta A B C,{/tex} we have
       {tex}\tan 30 ^ { \circ } = \frac { B C } { A B }{/tex}
       {tex}\Rightarrow \quad \frac { 1 } { \sqrt { 3 } } = \frac { h } { 40 }{/tex}
       {tex}\Rightarrow h = \frac { 40 } { \sqrt { 3 } } = \frac { 40 \sqrt { 3 } } { 3 } {/tex} = 23.1 m
       Thus height of the tower is 23.1 m.
       Substituting the value of h in (1), we have
       {tex}23.1 + h_1 = 40{/tex}
       {tex}\Rightarrow{/tex} h₁ = 40 – 23.1
       = 6.9 m
       Thus height of the tank is 6.9 m.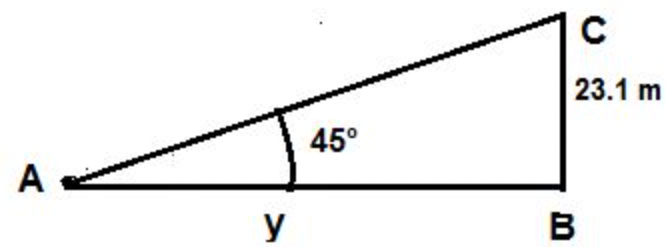
    6. 
       In the {tex}\triangle ABC {/tex} if {tex}\angle CAB =45^\circ{/tex} then
       {tex}\cot 45^\circ =\frac y{23.1}=1{/tex}
       y = 23.1 m
       Thus the angle of elevation will be 45^o at 23.1 m.
       OR
       
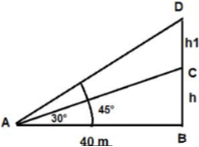
       Given that {tex}AB=\dfrac{40}{\sqrt3}{/tex}
       
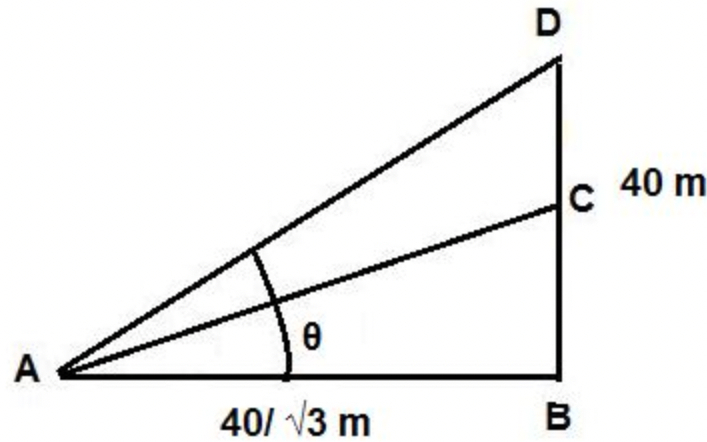
       In the {tex}\triangle ABD{/tex}
       {tex}\cot \theta=\frac{AB}{BD} =\frac{ \frac{40 }{\sqrt3} }{40}{/tex}
       {tex}\cot \theta=\frac1{\sqrt3}{/tex}
       {tex}\Rightarrow \theta=60^\circ{/tex}
       Hence the angle of elevation would be 60^o.
       Download the myCBSEguide App for Comprehensive Exam Preparation
       Prepare effectively for your exams with the myCBSEguide app, offering complete study material for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams. This app provides access to a wide range of practice questions, sample papers, and detailed solutions, helping you study smarter and achieve your academic goals. Download the myCBSEguide app today and get everything you need to excel in your exams.Examin8 App for Teachers: Create Custom Exam Papers Easily
       Teachers can enhance their teaching experience with the Examin8 app. This powerful tool allows educators to create customized question papers featuring their name and logo. Visit our Examin8 Website. The Class 10 Basic Maths Sample Paper 2025 is an excellent resource to help you solidify your understanding of key mathematical concepts. These sample papers cover all major topics in the syllabus, ensuring that you get ample practice for topics like algebra, geometry, and statistics. Regular practice with the Class 10 Basic Maths Sample Paper 2025 can enhance your problem-solving skills and build confidence for the exam.

myCBSEguide App for Board Students

Download myCBSEguide app

To excel in the Class 10 Basic Maths exam 2025, practicing with high-quality sample papers is essential. Our sample paper for Class 10 Basic Maths 2025 offers a comprehensive set of questions that follow the CBSE exam pattern.

Why Choose myCBSEguide for Your Exam Preparation?

1. Comprehensive Study Resources
myCBSEguide offers a complete range of study materials for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams. From textbooks to sample papers and previous years’ questions, all resources are curated to match the latest CBSE syllabus and exam patterns, ensuring that you are fully prepared for your exams.

2. Expert-Curated Content
Our study materials are developed by experienced educators who understand the nuances of the CBSE exam. Whether you are looking for concepts explanations, practice questions, or detailed solutions, myCBSEguide ensures the content is accurate, reliable, and aligned with the latest marking schemes.

3. Exam-Focused Practice Papers
myCBSEguide provides a wide variety of sample papers, mock tests, and practice questions for CBSE, JEE, NEET, and NDA exams. These exam-oriented resources are designed to help you familiarize yourself with the question format and improve time management, giving you the edge to score high.

4. Instant Access Anytime, Anywhere
With myCBSEguide, access all your study materials at your fingertips through our user-friendly app. Whether you’re at home, on the go, or revising at the last minute, you can easily download, read, and practice anytime, anywhere.

5. Trusted by Thousands of Students
myCBSEguide is trusted by thousands of students across India for its quality content and reliable exam preparation resources. Our platform has helped students consistently achieve top ranks in CBSE and competitive exams like JEE, NEET, and NDA.

6. Regularly Updated Content
Stay ahead with the latest content updates. myCBSEguide regularly updates its study material, ensuring it is always in line with the most recent changes in the CBSE syllabus and exam patterns. You’ll always have access to the most relevant and current study resources.