CBSE Class 12 Chapter 11 Physics Important Questions

myCBSEguide App

Download the app to get CBSE Sample Papers 2023-24, NCERT Solutions (Revised), Most Important Questions, Previous Year Question Bank, Mock Tests, and Detailed Notes.

Install Now

CBSE Class 12 Chapter 11 Physics Important Questions. We know Physics is tough subject within the consortium of science subjects physics is an important subject. But if you want to make career in these fields like IT Consultant, Lab Technician, Laser Engineer, Optical Engineer etc. You need to have strong fundamentals in physics to crack the exam. myCBSEguide has just released Chapter Wise Question Answers for class 12 Physics. There chapter wise Extra Questions with complete solutions are available for download in myCBSEguide website and mobile app. These Questions with solution are prepared by our team of expert teachers who are teaching grade in CBSE schools for years. There are around 4-5 set of solved Physics Extra Questions from each and every chapter. The students will not miss any concept in these Chapter wise question that are specially designed to tackle Board Exam. We have taken care of every single concept given in CBSE Class 12 Physics syllabus and questions are framed as per the latest marking scheme and blue print issued by CBSE for class 12.

Dual Nature of Radiation and Matter Class 12 Physics Extra Questions

Class – 12 Physics (Dual Nature of Radiation and Matter)

1. Stopping potential in the experimental set up shown in figure is
   

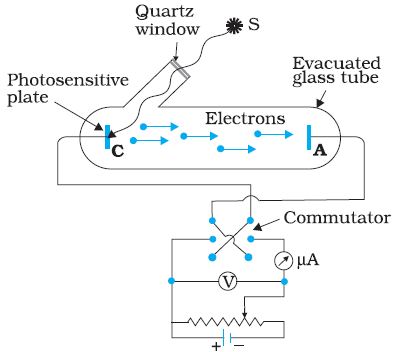
   1. positive potential V₀ given to the plate A for which the photocurrent stops or becomes zero
   2. positive potential V₀ given to the plate A for which the photocurrent stops increasing
   3. negative potential V₀ given to the plate A for which the photocurrent stops or becomes zero
   4. positive potential V₀ given to the plate A for which the photocurrent saturates
2. Photons can be

   1. deflected by electric fields
   2. scattered
   3. deflected by magnetic fields
   4. deflected by magnetic fields

3. If an electron moving with a speed of 2.5{tex} \times {\rm{1}}{0^{\rm{7}}}{\rm{m }}{{\rm{s}}^{ – {\rm{1}}}}{/tex} is deflected by an electric field of 1.6 k V {tex}{{\rm{m}}^{ – {\rm{1}}}}{/tex}perpendicular to its circular path, then e/m for the electron will be (given radius of circlar path = 2.3 m)

   1. 1.7{tex}\times {\rm{1}}{0^{{\rm{11}}}}{\rm{C k}}{{\rm{g}}^{ – {\rm{1}}}}{/tex}
   2. 1.8{tex} \times {\rm{1}}{0^{{\rm{11}}}}{\rm{C k}}{{\rm{g}}^{ – {\rm{1}}}}{/tex}
   3. 1.9{tex} \times {\rm{1}}{0^{{\rm{11}}}}{\rm{C k}}{{\rm{g}}^{ – {\rm{1}}}}{/tex}
   4. 1.85{tex} \times {\rm{1}}{0^{{\rm{11}}}}{\rm{C k}}{{\rm{g}}^{ – {\rm{1}}}}{/tex}
4. If the threshold wavelength of radiations required to eject a photoelectron from a metal surface is 6 {tex} \times {\rm{1}}{0^{ – {\rm{7}}}}{/tex} m, then work function of the metal is

   1. 3.4 {tex} \times {\rm{ 1}}{0^{ – {\rm{19}}}}{/tex} J
   2. 3.5 {tex} \times {\rm{ 1}}{0^{ – {\rm{19}}}}{/tex} J
   3. 3.3 {tex} \times {\rm{ 1}}{0^{ – {\rm{19}}}}{/tex} J
   4. 3.6 {tex} \times {\rm{ 1}}{0^{ – {\rm{19}}}}{/tex} J

5. According to the Einstein’s model stopping potential for a metal having work function {tex}{\phi _0}{/tex} is given by

   1. {tex}{V_0} = \left( {{h \over e}} \right)\nu + {{{\phi _0}} \over e}{/tex}
   2. {tex}{V_0} = \left( {{h \over e}} \right)\nu + 2{{{\phi _0}} \over e}{/tex}
   3. {tex}{V_0} = \left( {{h \over e}} \right)\nu – 2{{{\phi _0}} \over e}{/tex}
   4. {tex}{V_0} = \left( {{h \over e}} \right)\nu – {{{\phi _0}} \over e}{/tex}

6. Why is photoelectric emission not possible at all frequencies?

7. Ultraviolet light is incident on two photosensitive materials having work functions W₁ and W₂(W₁ > W₂). In which case will the kinetic energy of the emitted electrons be greater? Why?

8. The stopping potential in an experiment on photoelectric effect is 1.5 V. What is the maximum kinetic energy of the photoelectrons emitted?

9. An electron is revolving around the nucleus with a constant speed of 2. 2 {tex} \times {/tex} 10⁸ m/s. Find the de-Broglie wavelength associated with it.

10. A 100 W sodium lamp radius energy uniformly in all directions. The lamp is located at the centre of a large sphere that absorbs all the sodium light which is incident on it. The wavelength of the sodium light is 589 nm.

    1. What is the energy per photon associated with the sodium light?
    2. At what rate are the photons delivered to the sphere?

11. The maximum kinetic energy of a photoelectron is 3eV. What is its stopping potential?

12. Explain. Given {tex}{m_n} = 1.675 \times {10^{ – 27}}kg{/tex}(b) Obtain the de-Broglie wavelength associated with thermal neutrons at room temperature (27°C). Hence explain why a fast neutron beam needs to be thermalised with the environment before it can be used for neutron diffraction experiments.

13. Monochromatic light of wavelength 632.8 nm is produced by a helium neon laser. The power emitted is 9.42 mW.

    1. Find the energy and momentum of each photon in the light beam.
    2. How many photons per second, on the average, arrive at a target irradiated by this beam? (assume the beam to have uniform cross-section which is less than the target area), and
    3. How fast does a hydrogen atom have to travel in order to have the same momentum as that of the photon?

14. The work function for the following metals is given: Na : 2.75 eV K : 2.30 eV M₀ 4.17 eV Ni : 5.15 eV, Which of these metals will not give photoelectric emission for a radiation of wavelength {tex}3300\mathop A\limits^o {/tex} from a He-Cd laser placed 1m away from the photocell? What happens if the laser is brought nearer and placed 50 cm away?

15. When a surface is irradiated with light of {tex}\lambda = 4950\mathop {\text{A}}\limits^{\text{o}} {/tex}, a photocurrent appears which vanishes if a retarding potential greater than 0.6 V is applied across the photo tube. When a different source of light is used, it is found that the critical retarding potential is changed to 1.1 V. What is the work function of the surface and the wavelength of the second source? If the photoelectrons (after emission from the source ) are subjected to a magnetic field of 10 tesla what changes will be observed in the above two retarding potentials?

Class – 12 Physics (Dual Nature of Radiation and Matter)
Answers

1. 3. negative potential V₀ given to the plate A for which the photocurrent stops or becomes zero
      Explanation: The negative potential of the plate at which the photo electric current becomes zero is called stopping potential or cut-off potential. Its value is negative because some electron reach to the plate with their kinetic energy at zero potential.
   2. scattered
      Explanation: If photon strike with loosely bound electron then photoelectric effect takes place. Collision of photon with completely free electron give rise to Compton effect or Compton scattering.
   1. {tex}1.7 \times 10 ^ { 11 } \mathrm { Ckg } ^ { – 1 }{/tex}
      Explanation: Electric field provide required centripetal force for circular motion
      {tex}eE = \frac{{m{v^2}}}{r}{/tex}
      {tex}\frac{e}{m} = \frac{{{v^2}}}{{rE}} = \frac{{{{\left( {2.5 \times {{10}^7}} \right)}^2}}}{{2.3 \times 1.6 \times {{10}^3}}} = 1.7 \times {10^{11}}C\;K{g^{ – 1}}{/tex}
   3. 3.3 {tex} \times {\rm{ 1}}{0^{ – {\rm{19}}}}{/tex} J
      Explanation: {tex}{\phi _0} = {{hc} \over {{\lambda _0}}} = {{6.6 \times {{10}^{ – 34}} \times 3 \times {{10}^8}} \over {6 \times {{10}^{ – 7}}}} = 3.3 \times {10^{ – 19}}J{/tex}
   4. ​​​​​​{tex}V _ { 0 } = \left( \frac { h } { e } \right) \nu – \frac { \phi _ { 0 } } { e }{/tex}
      Explanation: {tex}e{V_0} = {K_{\max }}{/tex}
      {tex}{K_{\max }} = h\nu – {\phi _0}{/tex}
      {tex}e{V_0} = h\nu – {\phi _0}{/tex}
      {tex}{V_0} = \left( {\frac{h}{e}} \right)\nu – \frac{{{\phi _0}}}{e}{/tex}
2. Photoelectric emission is not possible at all frequencies because below the threshold frequency for photosensitive surface of different atoms emission is not possible.
3. K.E. of photoelectron = hv – W
   As given, W₁ > W₂
   Since, W₂ is lesser than W₁ thus the kinetic energy of the emitted electrons for the photoelectric material having work function W₂ will be greater.
4. Given, stopping potential, V = 1.5V
   KE_max = eV_o where, V₀ = cut-off potential
   KE_max = 1.5 eV
5. Given, v = 2.2 {tex} \times {/tex} 10⁸ m/s
   Here, m = 9.1 {tex} \times {/tex} 10^-31 kg
   h = 6.63 {tex} \times {/tex} 10^-34 kg-m²-s
   de-Broglie wavelength is given by
   {tex}\lambda{/tex}= h/mv…………………………(i)
   Substituting all values in Eq. (i), we get
   {tex}\lambda = \frac { 6.63 \times 10 ^ { – 34 } } { 9.1 \times 10 ^ { – 31 } \times 2.2 \times 10 ^ { 8 } }{/tex}
   {tex}\lambda = 3.31 \times 10 ^ { – 12 } \mathrm { m }{/tex}
6. Given,
   P (power) = 100 W
   {tex}\lambda = 589 \times {10^{ – 9}}m{/tex}
   1. Energy of each photon
      {tex}E = h\nu = \frac{{hc}}{\lambda } = \frac{{6.63 \times {{10}^{ – 34}} \times 3 \times {{10}^8}}}{{589 \times {{10}^{ – 9}}}}J{/tex}
      {tex} \Rightarrow E = 3.38 \times {10^{ – 19}}J{/tex}
   2. Number of photons delivered to sphere per second
      {tex}n = \frac{{Energy\;radiated\;per\;\sec ond}}{{Energy\;of\;each\;photon}}{/tex}
      P = nE
      or {tex}n = \frac{{100}}{{3.38 \times {{10}^{ – 19}}}} = 3 \times {10^{20}}{/tex}photons/s
7. Given, maximum kinetic energy of photoelectron = 3eV
   Therefore, Maximum KE = eV₀
   V₀ = stopping potential
   3eV= eV₀
   Hence, stopping potential
   V₀ = 3V
8. (b) Here, T = 27 + 273 = 300 K
   Boltzmann’s constant, k {tex} = 1.38 \times {10^{ – 23}}J\;mo{l^{ – 1}}{K^{ – 1}}{/tex}
   We know, average K.E. of neutron at absolute temperature T is given by {tex}E = \frac{3}{2}kT{/tex}. Where k is the Boltzmann’s constant.
   Now, {tex}\lambda = \frac{h}{{\sqrt {2mE} }} = \frac{h}{{\sqrt {3mkT} }}{/tex}
   {tex}\therefore \lambda = \frac{{6.63 \times {{10}^{ – 34}}}}{{\sqrt {3 \times 1.675 \times {{10}^{ – 27}} \times 1.38 \times {{10}^{ – 23}} \times 300} }} = 1.45 \times {10^{ – 10}}m{/tex}
   Since this wavelength is comparable to interatomic spacing {tex}( \sim 1\mathop A\limits^o ){/tex} in a crystal, therefore, thermal neutrons are suitable probe for diffraction experiments: so a high energy neutron beam should be first thermalised before using it for diffraction.
9. Given,
   Wavelength, {tex}\lambda = 632.8nm = 632.8 \times {10^{ – 9}}m{/tex}
   Frequency, {tex}\nu = \frac{c}{\lambda } = \frac{{3 \times {{10}^8}}}{{632.8 \times {{10}^{ – 9}}}}Hz{/tex}
   {tex} = 4.74 \times {10^{14}}Hz{/tex}
   1. E = hv
      {tex} = 6.63 \times {10^{ – 34}} \times 4.74 \times {10^{14}}J{/tex}
      {tex} = 3.14 \times {10^{ – 19}}J{/tex}
      p (momentum) {tex} = \frac{h}{\lambda } = \frac{{6.63 \times {{10}^{ – 34}}}}{{632.8 \times {{10}^{ – 9}}}} = 1.05 \times {10^{ – 27}}kg\;m{s^{ – 1}}{/tex}
   2. Power emitted, P = 9.42 mW {tex} = 9.42 \times {10^{ – 3}}W{/tex}
      P = nE
      {tex}n = \frac{P}{E} = \frac{{9.42 \times {{10}^{ – 3}}W}}{{3.14 \times {{10}^{ – 19}}J}} = 3 \times {10^{16}}photons/\sec {/tex}
   3. Velocity of hydrogen atom
      {tex} = \frac{{Momentum\;’p’\;of\;{H_2}\;atom\;(mv)}}{{Mass\;of\;{H_2}\;atom\;(m)}}{/tex}
      {tex} \Rightarrow v = \frac{{1.05 \times {{10}^{ – 27}}}}{{1.673 \times {{10}^{ – 27}}}}m{s^{ – 1}}{/tex} = 0.63ms^-1
10. (i) Work function of Na is
    {tex}{\phi _{Na}} = 1.92eV = 1.92 \times 1.6 \times {10^{ – 19}}J{/tex}
    {tex}\lambda = 3300\mathop A\limits^o = 3300 \times {10^{ – 10}}m{/tex}
    {tex}E = \frac{{hc}}{\lambda } = \frac{{6.6 \times {{10}^{ – 34}} \times 3 \times {{10}^8}}}{{3300 \times {{10}^{ – 10}}}}{/tex}
    {tex}E = \frac{{6.6 \times 3 \times {{10}^{ – 34 + 8 + 10 – 2}}}}{{33 \times 10}}J{/tex}
    {tex} = \frac{{6 \times {{10}^{ – 18 – 1}}}}{{1.6 \times {{10}^{ – 19}}}}J{/tex}
    {tex} = \frac{{60}}{{16}}eV{/tex}
    E = 3.75 eV
    It is observed that energy of incident radiation is less than Ni and Mo but larger than Na and K. So photoemission current take place from Na and K but not from Mo and Ni. Therefore, Mo and Ni will not give photoelectric emission. If the laser is brought closer the intensity of radiation increases without any change in frequency. This therefore, will not affect the result. However, photoelectric current from Na and K will increase.
11. According to Einstein’s equation of photo electricity
    {tex}\frac{1}{2}mv_{\max }^2 = e{V_0} = hv – {v_0}{/tex}
    or {tex}e{V_0} = \frac{{hc}}{\lambda } – {\phi _0}{/tex}
    where {tex}{\phi _0}{/tex} is the work function, {tex}\lambda{/tex} wavelength of incident light and V₀ is the stopping potential.
    For the first source,
    {tex}{\lambda _1} = 4950\mathop {\text{A}}\limits^{\text{o}} = 4950 \times {10^{ – 10}}m{/tex}
    V₀ = 0.6 V
    {tex}\therefore 1.6 \times {10^{ – 19}} \times 0.6{/tex} {tex} = \frac{{6.6 \times {{10}^{ – 34}} \times 3 \times {{10}^8}}}{{495 \times {{10}^{ – 9}}}} – {\phi _0}{/tex}
    or {tex}0.96 \times {10^{ – 19}} = 4 \times {10^{ – 19}} – {\phi _0}{/tex}
    {tex}\therefore {\phi _0} = 3.04 \times {10^{ – 19}}J{/tex} …..(i)
    {tex} = \frac{{3.04 \times {{10}^{ – 19}}}}{{1.6 \times {{10}^{ – 19}}}}eV{/tex} = 1.9 eV
    Let {tex}{\lambda _2}{/tex} be the wavelength of the second source.
    Given, {tex}V_0′ = 1.1V{/tex}
    Therefore,
    {tex}1.6 \times {10^{ – 19}} \times 1.1{/tex} {tex} = \frac{{6.6 \times {{10}^{ – 34}} \times 3 \times {{10}^8}}}{{{\lambda _2}}} – 3.04 \times {10^{ – 19}}J{/tex}(from I)
    or {tex}1.76 \times {10^{ – 19}}{/tex} {tex} = \frac{{19.8 \times {{10}^{ – 26}}}}{{{\lambda _2}}} – 3.04 \times {10^{ – 19}}{/tex}
    or {tex}\frac{{19.8 \times {{10}^{ – 26}}}}{{{\lambda _2}}} = 4.8 \times {10^{ – 19}}{/tex}
    {tex}\therefore {\lambda _2} = \frac{{19.8 \times {{10}^{ – 26}}}}{{4.8 \times {{10}^{ – 19}}}}m = 4.125 \times {10^{ – 7}}m{/tex} {tex}= 4125\mathop {\text{A}}\limits^{\text{o}}{/tex}
    When the ejected photoelectrons are subjected to the action of a magnetic field no change in retarding potential will be observed. This is because a magnetic field does not alter the kinetic energy of the photoelectrons. The magnetic field only changes the direction of motion.

Test Generator

Create question paper PDF and online tests with your own name & logo in minutes.

Create Now

myCBSEguide

Question Bank, Mock Tests, Exam Papers, NCERT Solutions, Sample Papers, Notes

Install Now