
myCBSEguide App
Download the app to get CBSE Sample Papers 2024-25, NCERT Solutions (Revised), Most Important Questions, Previous Year Question Bank, Mock Tests, and Detailed Notes.
Install NowNCERT Solutions for Class 10 Maths Exercise 6.4 Class 10 Maths book solutions are available in PDF format for free download. These ncert book chapter wise questions and answers are very helpful for CBSE board exam. CBSE recommends NCERT books and most of the questions in CBSE exam are asked from NCERT text books. Class 10 Maths chapter wise NCERT solution for Maths Book for all the chapters can be downloaded from our website and myCBSEguide mobile app for free.
NCERT solutions for Maths Triangles Download as PDF

NCERT Solutions for Class 10 Maths Triangles
1. Let  ABC
ABC 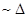 DEF and their areas be, respectively, 64 cm2 and 121 cm2. If EF = 15.4 cm, find BC.
DEF and their areas be, respectively, 64 cm2 and 121 cm2. If EF = 15.4 cm, find BC.
Ans. We have, 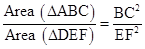

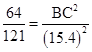

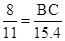
 BC =
BC =  cm = 11.2 cm
cm = 11.2 cm
2. Diagonals of a trapezium ABCD with AB  DC intersect each other at the point O. If AB = 2CD, find the ratio of the areas of triangles AOB and COD.
DC intersect each other at the point O. If AB = 2CD, find the ratio of the areas of triangles AOB and COD.
Ans. In  s AOB and COD, we have,
s AOB and COD, we have,
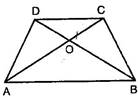
 AOB =
AOB =  COD[Vertically opposite angles]
COD[Vertically opposite angles]
 OAB =
OAB =  OCD[Alternate angles]
OCD[Alternate angles]
By AA-criterion of similarity,
 AOB
AOB
 COD
COD
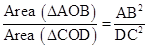

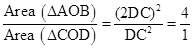
Hence, Area ( AOB) : Area (
AOB) : Area ( COD) = 4 : 1
COD) = 4 : 1
NCERT Solutions for Class 10 Maths Exercise 6.4
3. In figure, ABC and DBC are two triangles on the same base BC. If AD intersects BC at O, show that 
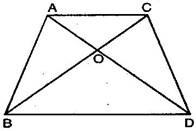
Ans. Given: Two  s ABC and DBC which stand on the same base but on the opposite sides of BC.
s ABC and DBC which stand on the same base but on the opposite sides of BC.
To Prove: 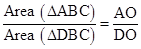
Construction: Draw AE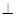 BC and DF
BC and DF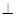 BC.
BC.
Proof: In  s AOE and DOF, we have,
s AOE and DOF, we have, 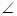 AEO =
AEO = 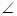 DFO =
DFO = 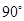
and 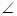 AOE =
AOE = 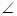 DOF[Vertically opposite)
DOF[Vertically opposite)
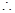
 AOE
AOE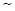
 DOF[By AA-criterion]
DOF[By AA-criterion]
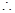
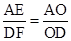 ……….(i)
……….(i)
Now, 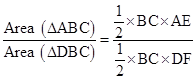

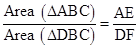

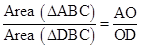 [using eq. (i)]
[using eq. (i)]
NCERT Solutions for Class 10 Maths Exercise 6.4
4. If the areas of two similar triangles are equal, prove that they are congruent.
Ans. Given: Two  s ABC and DEF such that
s ABC and DEF such that  ABC
ABC
 DEF
DEF
And Area( ABC) = Area (
ABC) = Area ( DEF)
DEF)
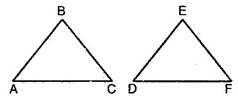
To Prove:  ABC
ABC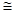
 DEF
DEF
Proof:  ABC
ABC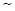
 DEF
DEF
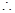
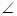 A =
A = 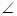 D,
D, 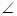 B =
B = 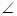 E,
E, 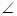 C =
C = 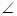 F
F
And 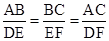
To establish  ABC
ABC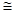
 DEF, it is sufficient to prove that, AB = DE, BC = EF and AC = DF
DEF, it is sufficient to prove that, AB = DE, BC = EF and AC = DF
Now, Area( ABC) = Area (
ABC) = Area ( DEF)
DEF)
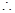
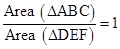

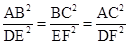 = 1
= 1

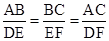 = 1
= 1
 AB = DE, BC = EF, AC = DF
AB = DE, BC = EF, AC = DF
Hence, ABC
ABC
 DEF
DEF
NCERT Solutions for Class 10 Maths Exercise 6.4
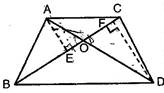
5. D, E and F are respectively the mid-points of sides AB, BC and CA of  ABC. Find the ratio of the areas of
ABC. Find the ratio of the areas of  DEF and
DEF and  ABC.
ABC.
Ans. Since, D and E are the mid-points of the sides BC and CA of  ABC respectively.
ABC respectively.
 DE
DE BA
BA  DE
DE FA ……….(i)
FA ……….(i)
Since, D and F are the mid-points of the sides BC and AB of  ABC respectively.
ABC respectively.
 DF
DF CA
CA  DE
DE AE ……….(ii)
AE ……….(ii)
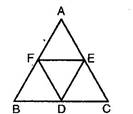
From (i) and (ii), we can say that AFDE is a parallelogram.
Similarly, BDEF is a parallelogram.
Now, in  s DEF and ABC, we have
s DEF and ABC, we have
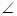 FDE =
FDE = 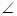 A[opposite angles of
A[opposite angles of  gm AFDE]
gm AFDE]
And 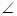 DEF =
DEF = 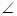 B[opposite angles of
B[opposite angles of  gm BDEF]
gm BDEF]
 By AA-criterion of similarity, we have
By AA-criterion of similarity, we have  DEF
DEF 
 ABC
ABC

[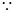 DE =
DE = 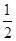 AB]
AB]
Hence, Area ( DEF): Area (
DEF): Area ( ABC) = 1 : 4
ABC) = 1 : 4
NCERT Solutions for Class 10 Maths Exercise 6.4
6. Prove that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
Ans. Given:  ABC
ABC
 PQR, AD and PM are the medians of
PQR, AD and PM are the medians of  s ABC and PQR respectively.
s ABC and PQR respectively.
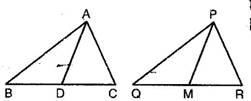
To Prove: 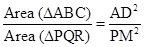
Proof: Since  ABC
ABC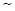
 PQR
PQR
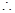
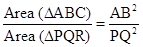 ……….(1)
……….(1)
But, 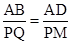 ……….(2)
……….(2)
 From eq. (1) and (2), we have,
From eq. (1) and (2), we have,
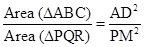
NCERT Solutions for Class 10 Maths Exercise 6.4
7. Prove that the area of an equilateral triangle described on one side of a square is equal to half the area of the equilateral triangle described on one of the diagonals.
Tick the correct answer and justify:
Ans. Given: A square ABCD,
Equilateral  s BCE and ACF have been drawn on side BC and the diagonal AC respectively.
s BCE and ACF have been drawn on side BC and the diagonal AC respectively.
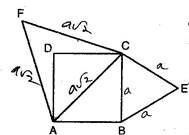
To Prove: Area ( BCE) =
BCE) = 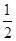 Area (
Area ( ACF)
ACF)
Proof:  BCE
BCE
 ACF
ACF
[Being equilateral so similar by AAA criterion of
similarity]

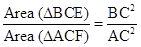

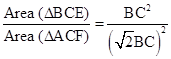
[ Diagonal =
Diagonal =  side
side  AC =
AC =  BC]
BC]

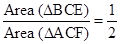
NCERT Solutions for Class 10 Maths Exercise 6.4
8. ABC and BDE are two equilateral triangles such that D is the mid-point of BC. Ratio of the areas of triangles ABC and BDE is:
(A) 2: 1
(B) 1: 2
(C) 4: 1
(D) 1: 4
Ans. (C) Since  ABC and
ABC and  BDE are equilateral, they are equiangular and hence,
BDE are equilateral, they are equiangular and hence,
 ABC
ABC
 BDE
BDE
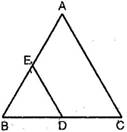

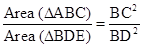

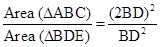
[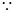 D is the mid-point of BC]
D is the mid-point of BC]

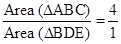
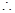 (C) is the correct answer.
(C) is the correct answer.
NCERT Solutions for Class 10 Maths Exercise 6.4
9. Sides of two similar triangles are in the ratio 4 : 9. Areas of these triangles are in the ratio:
(A) 2: 3
(B) 4: 9
(C) 81: 16
(D) 16: 81
Ans. (D) Since the ratio of the areas of two similar triangles is equal to the ratio of the squares of any two corresponding sides. Therefore,
Ratio of areas = 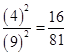
 (D) is the correct answer.
(D) is the correct answer.
NCERT Solutions for Class 10 Maths Exercise 6.4
NCERT Solutions Class 10 Maths PDF (Download) Free from myCBSEguide app and myCBSEguide website. Ncert solution class 10 Maths includes text book solutions from Mathematics Book. NCERT Solutions for CBSE Class 10 Maths have total 15 chapters. 10 Maths NCERT Solutions in PDF for free Download on our website. Ncert Maths class 10 solutions PDF and Maths ncert class 10 PDF solutions with latest modifications and as per the latest CBSE syllabus are only available in myCBSEguide.
CBSE app for Class 10
To download NCERT Solutions for Class 10 Maths, Computer Science, Home Science,Hindi ,English, Social Science do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT solutions, NCERT Exemplar solutions, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE

Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
myCBSEguide
Question Bank, Mock Tests, Exam Papers, NCERT Solutions, Sample Papers, Notes
Install Now
Very nice
Very useful……..
Hey, the fig. of Q 5 is wrong.Have a look at that.
Diagram of question 5 is wrong. Point F goes with AC not AB. Have a check guys. Do the correction or kids may suffer.
thaks team for ice crispy solutios
nyc
I LIKE IT SO MUCH
Good ?
fig.of ques.5 is wrong but allover nic
Good solve and very nice
Class 10 Science MCQ