
myCBSEguide App
Download the app to get CBSE Sample Papers 2025-26, NCERT Solutions (Revised), Most Important Questions, Previous Year Question Bank, Mock Tests, and Detailed Notes.
Install NowExercise 11.3
In each of the Exercises 1 to 9, find the coordinates of the foci, the vertices, the length of major axis, the minor axis, the eccentricity and the length of the latus rectum of the ellipse.
1. 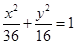
Ans. Given: Equation of ellipse: 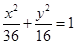
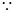 36 > 16
36 > 16

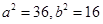

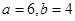
Now 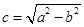 =
= 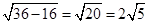
 Coordinates of foci are
Coordinates of foci are 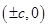

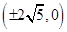
Coordinates of vertices are 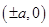

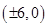
Length of major axis = 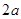 =
= 
Length of minor axis =  =
= 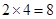
Eccentricity 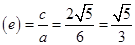
Length of latus rectum = 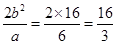
2. 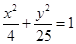
Ans. Given: Equation of ellipse: 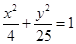
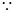 25 > 4
25 > 4 
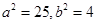

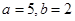
Now 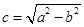 =
= 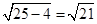
 Coordinates of foci are
Coordinates of foci are 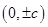

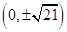
Coordinates of vertices are 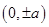

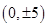
Length of major axis = 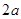 =
= 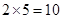
Length of minor axis =  =
= 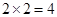
Eccentricity 
Length of latus rectum = 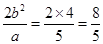
3. 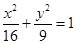
Ans. Given: Equation of ellipse: 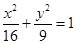
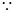 16 > 9
16 > 9 
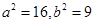


Now 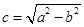 =
= 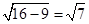
 Coordinates of foci are
Coordinates of foci are 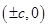

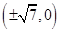
Coordinates of vertices are 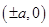


Length of major axis = 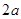 =
= 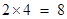
Length of minor axis =  =
= 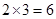
Eccentricity 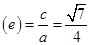
Length of latus rectum = 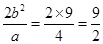
4. 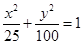
Ans. Given: Equation of ellipse: 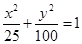
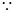 100 > 25
100 > 25 
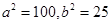

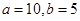
Now 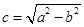 =
= 
 Coordinates of foci are
Coordinates of foci are 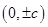


Coordinates of vertices are 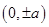

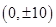
Length of major axis = 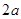 =
= 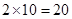
Length of minor axis =  =
= 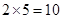
Eccentricity 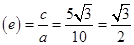
Length of latus rectum = 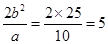
5. 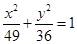
Ans. Given: Equation of ellipse: 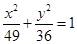
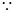 49 > 36
49 > 36 


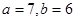
Now 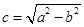 =
= 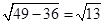
 Coordinates of foci are
Coordinates of foci are 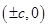

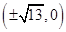
Coordinates of vertices are 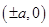

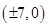
Length of major axis = 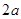 =
= 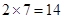
Length of minor axis =  =
= 
Eccentricity 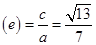
Length of latus rectum = 
6. 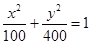
Ans. Given: Equation of ellipse: 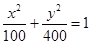
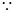 400 > 100
400 > 100 
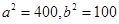

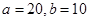
Now 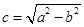 =
= 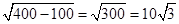
 Coordinates of foci are
Coordinates of foci are 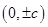

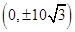
Coordinates of vertices are 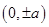

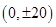
Length of major axis = 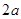 =
=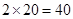
Length of minor axis =  =
= 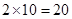
Eccentricity 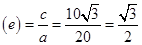
Length of latus rectum = 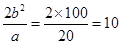
7. 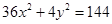
Ans. Given: Equation of ellipse: 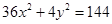

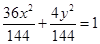

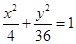
 36 > 4
36 > 4 



Now 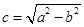 =
= 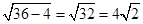
 Coordinates of foci are
Coordinates of foci are 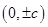

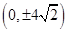
Coordinates of vertices are 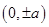

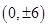
Length of major axis = 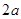 =
= 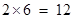
Length of minor axis =  =
= 
Eccentricity 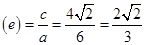
Length of latus rectum = 
8. 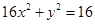
Ans. Given: Equation of ellipse: 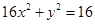

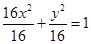

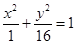
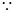 16 > 1
16 > 1 
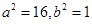

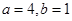
Now 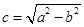 =
= 
 Coordinates of foci are
Coordinates of foci are 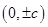

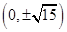
Coordinates of vertices are 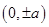

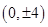
Length of major axis = 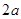 =
= 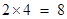
Length of minor axis =  =
= 
Eccentricity 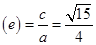
Length of latus rectum = 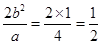
9. 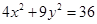
Ans. Given: Equation of ellipse: 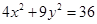



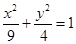
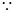 9 > 4
9 > 4 
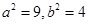

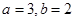
Now 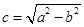 =
= 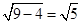
 Coordinates of foci are
Coordinates of foci are 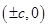

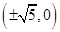
Coordinates of vertices are 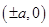

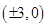
Length of major axis = 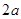 =
= 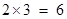
Length of minor axis =  =
= 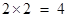
Eccentricity 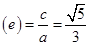
Length of latus rectum = 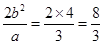
In each of the Exercises 10 to 20, find the equation of the ellipse that satisfies the given conditions:
10. Vertices  foci
foci 
Ans. Since foci  lie on
lie on 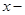 axis, therefore equation of ellipse is
axis, therefore equation of ellipse is 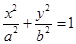
Now Vertices 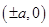

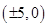

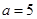
And Foci 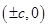



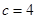
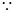
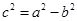

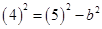

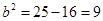
Therefore, the required equation of ellipse is 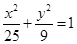 .
.
11. Vertices 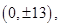 foci
foci 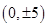
Ans. Since foci 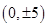 lie on
lie on 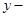 axis, therefore equation of ellipse is
axis, therefore equation of ellipse is 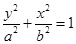
Now Vertices 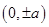

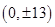

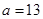
And Foci 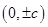

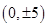

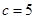
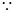
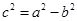

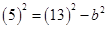


Therefore, the required equation of ellipse is 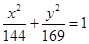 .
.
12. Vertices 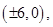 foci
foci 
Ans. Since foci  lie on
lie on 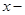 axis, therefore equation of ellipse is
axis, therefore equation of ellipse is 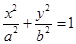
Now Vertices 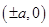

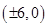

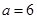
And Foci 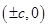



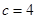
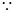
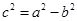

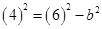


Therefore, the required equation of ellipse is  .
.
13. Ends of major axis 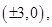 ends of minor axis
ends of minor axis 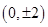
Ans. Ends of major axis  lie on
lie on 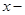 axis, therefore equation of ellipse is
axis, therefore equation of ellipse is 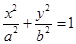
Now Ends of major axis 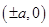

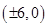

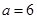
And Ends of minor of axis 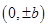

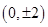

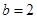
Therefore, the required equation of ellipse is 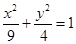 .
.
14. Ends of major axis  ends of minor axis
ends of minor axis 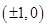
Ans. Ends of major axis  lie on
lie on 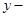 axis, therefore equation of ellipse is
axis, therefore equation of ellipse is 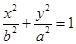
Now Ends of major axis 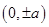



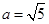
And Ends of minor of axis 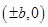

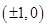

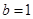
Therefore, the required equation of ellipse is 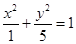 .
.
15. Length of major axis 26, foci 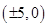
Ans. Since foci 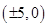 lie on
lie on 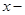 axis, therefore equation of ellipse is
axis, therefore equation of ellipse is 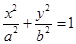
 Length of major axis =
Length of major axis = 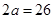

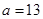
Foci 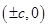 =
= 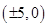

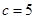
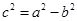

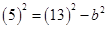


Therefore, the required equation of ellipse is  .
.
16. Length of minor axis 16, foci 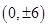
Ans. Since foci 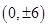 lie on
lie on 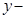 axis, therefore equation of ellipse is
axis, therefore equation of ellipse is 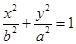
 Length of major axis =
Length of major axis = 

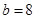
Foci 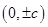 =
= 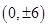

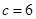
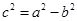



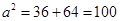
Therefore, the required equation of ellipse is 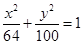 .
.
17. Foci 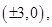
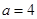
Ans. Since foci 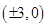 lie on
lie on 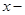 axis, therefore equation of ellipse is
axis, therefore equation of ellipse is 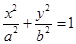
 Foci
Foci 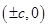 =
= 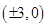


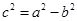



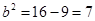
Therefore, the required equation of ellipse is 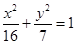 .
.
18. 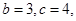 centre at origin; foci on
centre at origin; foci on 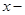 axis
axis
Ans. Since foci lie on 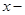 axis, therefore equation of ellipse is
axis, therefore equation of ellipse is 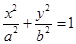

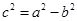

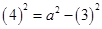

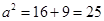
Therefore, the required equation of ellipse is 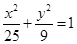 .
.
19. Centre at (0, 0), major axis on the 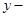 axis and passes through the points (3, 2) and (1, 6).
axis and passes through the points (3, 2) and (1, 6).
Ans. Since the major axis is along 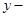 axis, therefore equation of ellipse is
axis, therefore equation of ellipse is 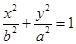
And the ellipse passes through the point (3, 2) therefore 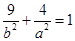 …..(i)
…..(i)
And the ellipse passes through the point (1, 6) therefore 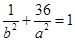 …..(ii)
…..(ii)
Solving eq. (i) and (ii), we have 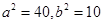
Therefore, the required equation of ellipse is 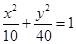 .
.
20. Major axis on the 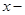 axis and passes through the points (4, 3) and (6, 2).
axis and passes through the points (4, 3) and (6, 2).
Ans. Since the major axis is along 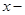 axis, therefore equation of ellipse is
axis, therefore equation of ellipse is 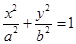
And the ellipse passes through the point (4, 3) therefore 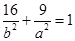 …..(i)
…..(i)
And the ellipse passes through the point (6, 2) therefore  …..(ii)
…..(ii)
Solving eq. (i) and (ii), we have 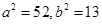
Therefore, the required equation of ellipse is 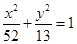 .
.

Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
Learn8 App
Practice unlimited questions for Entrance tests & government job exams at ₹99 only
Install Now