Class 9 Maths Sample Paper 2024-25

Class 9 Maths Sample Paper 2025

The CBSE Class 9 Maths Sample Paper 2024-25 includes both subjective and objective type questions, with a special focus on case study-based questions in the subjective section. Unlike Class 10, where students have the option to choose from multiple courses, all Class 9 Mathematics students study the same course content. he Class 9 Maths Sample Paper 2024-25 is an indispensable tool for students looking to excel in their exams. It provides a comprehensive overview of the exam pattern, with well-structured questions designed according to the latest CBSE guidelines. Practicing with this Class 9 Maths Sample Paper 2024-25 will help students understand the types of questions they can expect and assess their preparation level. Available on the myCBSEguide App and myCBSEGuide Website, these sample papers come with detailed solutions to help students grasp important concepts more effectively. By solving the sample paper, students can revise key topics and identify areas that need further attention, making it an ideal revision resource. Download the Class 9 Maths Sample Paper 2024-25 today to ensure thorough and structured exam preparation. To help you prepare effectively, you can download the model question paper for Class 9 Maths for free from the myCBSEguide App or the student dashboard. These sample papers are designed to align with the updated exam pattern and provide a comprehensive understanding of the subject. Download the sample paper today and get ready to ace your Class 9 Maths exam with the best resources available.Teachers can also take advantage of the Examin8 App and Examin8 Website to create custom exam papers with their name and logo.

Sample Paper of class 9 Math – in PDF

CBSE Sample Question Papers (Solved) Class 9 Mathematics for the session 2023-24 are now available, following the updated marking scheme and blueprint issued by CBSE. These Class 9 Mathematics sample papers are designed to align with the latest exam pattern and will help students familiarize themselves with the types of questions asked in the exams. We are also offering guess papers for Class 9 Mathematics for the 2025 annual exams. These sample question papers, available for free download in PDF format on the myCBSEguide App and myCBSEGuide Website, come with solutions to guide students through the exam preparation process. Practicing with these CBSE sample papers will not only boost confidence but also improve students’ chances of scoring high marks in their Class 9 Mathematics exam. Teachers can also take advantage of the Examin8 App and Examin8 Website to create custom exam papers with their name and logo.

The Best Model Papers for Class 9 Maths 2024-25

For Class 9 students preparing for the CBSE exams, model papers are crucial for understanding the exam pattern and practicing effectively. While CBSE provides official model papers for board exams (Classes 10 and 12), Class 9 model papers are usually prepared by schools. This means the format and difficulty level can vary, making it important to have access to standardized, well-structured papers.

myCBSEguide offers model papers for Class 9 that strictly follow the latest CBSE guidelines. These papers are designed to provide a moderate difficulty level, matching the actual exam format. By practicing with these model papers, students can familiarize themselves with multiple-choice, short-answer, and long-answer questions.

Key features of myCBSEguide model papers include:

• Adherence to CBSE syllabus: Updated to reflect the latest curriculum.
• Comprehensive coverage: Ensures all important topics are included.
• Real exam experience: Simulates actual exam conditions.
• Detailed solutions: Helps students understand concepts better.

These model papers offer a reliable and consistent resource for students to practice and prepare confidently for their Class 9 exams. Download the myCBSEguide app or visit our website for free access to high-quality model papers and start your preparation today!

Sample Papers of Class 9 Maths 2025 with solution

Download PDF 

CBSE Sample Papers Class 9 Mathematics

Unlike Class 10 and 12, CBSE does not conduct board exams for Class 9 students. Instead, these exams are home exams, where the responsibility of conducting the exam lies with the respective schools. However, schools must follow the guidelines issued by CBSE while preparing the question paper to ensure uniformity in terms of difficulty level and exam format.

Key Points about CBSE Class 9 Home Exams:

• No Board Exams for Class 9: The exams for Class 9 students are not board exams but are still an important part of the academic year.
• Following CBSE Guidelines: While the exams are home-based, schools must strictly adhere to the guidelines provided by CBSE for the structure and content of the question paper. This ensures consistency and fairness across all schools. You can find detailed solutions to the Class 9 Maths Sample Paper 2024-25 on the myCBSEguide website for better understanding.
• Uniform Difficulty Level and Format: To maintain uniformity, CBSE provides model question papers and examination materials through its official portal. Schools are required to download the question papers directly from the CBSE website, ensuring that the format and difficulty level align with CBSE standards.

Class 09 – Mathematics
Sample Paper – 01 (2024-25)

---

Maximum Marks: 80
Time Allowed: : 3 hours

---

General Instructions:

Read the following instructions carefully and follow them:

1. This question paper contains 38 questions.
2. This Question Paper is divided into 5 Sections A, B, C, D and E.
3. In Section A, Questions no. 1-18 are multiple choice questions (MCQs) and questions no. 19 and 20 are Assertion- Reason based questions of 1 mark each.
4. In Section B, Questions no. 21-25 are very short answer (VSA) type questions, carrying 02 marks each.
5. In Section C, Questions no. 26-31 are short answer (SA) type questions, carrying 03 marks each.
6. In Section D, Questions no. 32-35 are long answer (LA) type questions, carrying 05 marks each.
7. In Section E, Questions no. 36-38 are case study-based questions carrying 4 marks each with sub-parts of the values of 1,1 and 2 marks each respectively.
8. All Questions are compulsory. However, an internal choice in 2 Questions of Section B, 2 Questions of Section C and 2 Questions of Section D has been provided. An internal choice has been provided in all the 2 marks questions of Section E.
9. Draw neat and clean figures wherever required.
10. Take {tex}\pi=22 / 7{/tex} wherever required if not stated.
11. Use of calculators is not allowed.

---

1. Section A
2. The equation of y-axis is:

   a)x = 0

   b)y = x

   c)y = 0

   d)y {tex}\ne{/tex} x

3. The side of a triangle is 12 cm, 16 cm, and 20 cm. Its area is

   a)100sq cm

   b)90sq cm

   c)120sq cm

   d)96 sq cm

4. In the figure, O is the centre of eh circle and {tex}\angle AOB = {80^o}{/tex}. The value of x is :
   

   a){tex}{60^o}{/tex}

   b){tex}{30^o}{/tex}

   c){tex}{160^o}{/tex}

   d){tex}{40^o}{/tex}

5. In the given figure, AD is a median of {tex}\triangle{/tex}ABC and E is the midpoint of AD. If BE is joined and produced to meet AC in F then AF = ?
   

   a){tex}\frac{1}{3} A C{/tex}

   b){tex}\frac{3}{4} AC{/tex}

   c){tex}\frac{2}{3} AC{/tex}

   d){tex}\frac{1}{2} A C{/tex}

6. The value of {tex}\left\{8^{\frac {-4} 3} \div 2^{-2}\right\}^{\frac 1 2}{/tex}, is

   a)4

   b)2

   c){tex}\frac{1}{2}{/tex}

   d){tex}\frac{1}{4}{/tex}

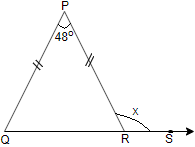
7. In the adjoining fig, PQ = PR. If {tex}\angle QPR{/tex} = 48{tex}^\circ {/tex}, then value of x is:
   

   a)132^o

   b)114{tex}^\circ {/tex}

   c)104^o

   d)96^o

8. x = 2, y = 3 is a solution of the linear equation

   a)x + 2y = 8

   b)x + y = 8

   c)-x + y = 8

   d)2x + y = 8

9. If {tex}{x^2} + kx – 3 = (x – 3)(x + 1){/tex}, then the value of ‘k’ is

   a)-3

   b)2

   c)-2

   d)3

10. The value of {tex}\left(\frac{81}{16}\right)^{\frac{-3}{4}} \times\left\{\left(\frac{25}{9}\right)^{\frac{-3}{2}} \div\left(\frac{5}{2}\right)^{-3}\right\}{/tex} is

    a)4

    b)2

    c)3

    d)1

11. In Triangle ABC which is right angled at B. Given that AB = 9cm, AC = 15cm and D, E are the mid-points of the sides AB and AC respectively. Find the length of BC?

    a)13cm

    b)13.5cm

    c)12cm

    d)15cm

12. The decimal representation of a rational number is

    a)always terminating

    b)always non-terminating

    c)either terminating or repeating

    d)either terminating or non-repeating

13. Which of the following points lie on the line y = 3x – 4?

    a)(2, 2)

    b)(4, 12)

    c)(5, 15)

    d)(3, 9)

14. In a figure, if OP||RS, {tex}\angle{/tex}OPQ = 110° and {tex}\angle{/tex}QRS = 130°, then {tex}\angle{/tex}PQR is equal to
    

    a)40°

    b)50°

    c)70°

    d)60°

15. The product of any two irrational numbers is

    a)sometimes rational, sometimes irrational

    b)always a rational number

    c)always an integer

    d)always an irrational number.

16. In the given figure, O is the centre of a circle in which {tex}\angle{/tex}OAB = 20° and {tex}\angle{/tex}OCB = 50°. Then, {tex}\angle{/tex}AOC = ?
    

    a)20°

    b)70°

    c)60°

    d)50°

17. The perpendicular distance of the point P (4, 3) from x-axis is

    a)3

    b)6

    c)4

    d)5

18. The linear equation 3x – 5y = 15 has

    a)no solution

    b)infinitely many solutions

    c)a unique solution

    d)two solutions

19. x³ + y³ + z³ – 3xyz is

    a)(x + y + z)³

    b)(x + y + z)(x² + y² + z² – xy – yz – zx)

    c)(x – y + z)³

    d)(x + y + z)³ – 3xyz

20. Assertion (A): In {tex}\Delta{/tex}ABC, E and F are the midpoints of AC and AB respectively. The altitude AP at BC intersects FE at Q. Then, AQ = QP.
    Reason (R): Q is the midpoint of AP.

    a)Both A and R are true and R is the correct explanation of A.

    b)Both A and R are true but R is not the correct explanation of A.

    c)A is true but R is false.

    d)A is false but R is true.

21. Assertion (A): {tex}\sqrt{3}{/tex} is an irrational number.
    Reason (R): Square root of a positive integer which is not a perfect square is an irrational number.

    a)Both A and R are true and R is the correct explanation of A.

    b)Both A and R are true but R is not the correct explanation of A.

    c)A is true but R is false.

    d)A is false but R is true.

    To prepare effectively for your exams, download the myCBSEguide App and myCBSEGuide Website. This app offers a comprehensive range of study materials, including sample papers, solutions, and practice questions for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams. It’s designed to help students build a solid foundation and enhance their exam readiness.Teachers can also take advantage of the Examin8 App and Examin8 Website to create custom exam papers with their name and logo. This powerful tool allows educators to generate personalized assessments, making it easier to track student progress and tailor their teaching approach.Whether you’re a student or a teacher, myCBSEguide and Examin8 are the perfect apps to streamline learning and teaching. Download them now to start preparing for success! Practicing the Class 9 Maths Sample Paper 2024-25 is a great way to boost your confidence before the board exams.

    Section B

22. Why is Axiom 5, in the list of Euclid’s axioms, considered a universal truth?
23. In fig. AC = XD, C is the mid-point of AB and D is the mid-point of XY. Using a Euclid’s axiom, show that AB = XY.
    
24. Name the quadrant in which the following points lie:  (i) A(2, 9)   (ii) B(–3, 5)    (iii) C(–4, –7)   (iv) D(3, –2)
25. Examine, whether {tex}\sqrt{5}-2{/tex} is rational or irrational.

    OR

    Rationalise the denominator of: {tex}\frac{\sqrt{5}}{2 \sqrt{3}}{/tex}.

26. A Joker’s cap is in the form of a right circular cone of base radius 7 cm and height 24 cm. Find the area of the sheet required to make 10 such caps.

    OR

    Find the ratio of the curved surface areas of two cones if their diameters of the bases are equal and slant heights are in the ratio 4 : 3.

27. Section C
28. Give three rational numbers between {tex}\frac {1} {3} {/tex} and {tex}\frac {1} {2}{/tex}.
29. The monthly profits (in Rs) of 100 shops are distributed as follows:
    

    Profits per shop:	0-50	50-100	100-50	150-200	200-250	250-300
    No. of shops:	12	18	27	20	17	6

    Draw a histogram for the data and show the frequency polygon for it.

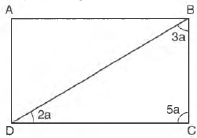
30. In Figure find the four angles A, B, C and D in the parallelogram ABCD.
    
31. Find at least 3 solutions for the following linear equation in two variables: x + y – 4 = 0
32. The population of Delhi State in different census years is as given below:
    

    Census year	1961	1971	1981	1991	2001
    Population in Lakhs	30	55	70	110	150

    Represent the above information with the help of a bar graph.

    OR

    A random survey of the number of children of various age groups playing football match in a park was found as follows

    Age (in years)	1-2	2-3	3-5	5-7	7-10	10-15	15-17
    Number of children	5	4	10	12	9	10	8

    Draw a histogram to represent the above data.

33. Factorize: {tex}{x^3} + 13{x^2} + 32x + 20{/tex}
34. Section D
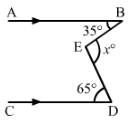
35. In each of the figures given below, AB {tex}\parallel{/tex} CD. Find the value of x{tex}^\circ{/tex} in each case.
    

    OR

    Two lines AB and CD intersect at a point O such that {tex}\angle B O C+\angle A O D=280^{\circ}{/tex}, as shown in the figure. Find all the four angles.
    

36. What length of tarpaulin 3 m wide will be required to make conical tent of height 8 m and base radius 6 m? Assume that the extra length of material that will be required for stitching margins and wastage in cutting is approximately 20 cm. {tex}\left( \text{Use }\pi \text{ = 3}\text{.14} \right){/tex}
37. Two sides of a triangular field are 85 m and 154 m in length and its perimeter is 324 m. Find the area of the field.

    OR

    Find the area of a triangular field whose sides are 91 m, 98 m and 105 m in length. Find the height corresponding to the longest side.

38. If ax³ + bx² + x – 6 has x + 2 as a factor and leaves a remainder 4 when divided by (x – 2), find the values of a and b.
39. Section E
40. Read the following text carefully and answer the questions that follow:
    Ajay is writing a test which consists of ‘True’ or ‘False’ questions. One mark is awarded for every correct answer while ¼ mark is deducted for every wrong answer. Ajay knew answers to some of the questions. Rest of the questions he attempted by guessing.
    
    1. If he answered 110 questions and got 80 marks and answer to all questions, he attempted by guessing were wrong, then how many questions did he answer correctly? (1)
    2. If he answered 110 questions and got 80 marks and answer to all questions, he attempted by guessing were wrong, then how many questions did he guess? (1)
    3. If answer to all questions he attempted by guessing were wrong and answered 80 correctly, then how many marks he got? (2)
       OR
       If answer to all questions he attempted by guessing were wrong, then how many questions answered correctly to score 95 marks? (2)
41. Read the following text carefully and answer the questions that follow:
    As shown In the village of Surya there was a big pole PC. This pole was tied with a strong wire of 10 m length. Once there was a big spark on this pole, thus wires got damaged very badly. Any small fault was usually repaired with the help of a rope which normal board electricians were carrying on bicycles.
    This time electricians need a staircase of 10 m so that it can reach at point P on the pole and this should make 60^o with line AC.
    
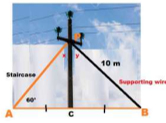
    1. Show that {tex}\triangle{/tex}APC and {tex}\triangle{/tex}BPC are congruent. (1)
    2. Find the value of {tex}\angle{/tex}x. (1)
    3. Find the value of {tex}\angle{/tex}y. (2)
       OR
       What is the value of {tex}\angle{/tex}PBC? (2)
42. Read the following text carefully and answer the questions that follow:
    Rohan draws a circle of radius 10 cm with the help of a compass and scale. He also draws two chords, AB and CD in such a way that the perpendicular distance from the center to AB and CD are 6 cm and 8 cm respectively. Now, he has some doubts that are given below.
    
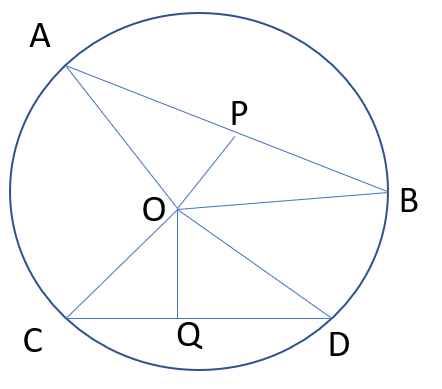
    1. Show that the perpendicular drawn from the Centre of a circle to a chord bisects the chord. (1)
    2. What is the length of CD? (1)
    3. What is the length of AB? (2)
       ORHow many circles can be drawn from given three noncollinear points? (2)

       To boost your exam preparation and practice more questions, download the myCBSEguide App and myCBSEGuide Website. The app offers comprehensive study material for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams, providing everything you need to excel. Whether you’re a student looking for practice papers, NCERT solutions, or detailed exam notes, myCBSEguide is the ultimate resource for effective study.

       Key Features of the myCBSEguide App:

       • Complete Study Material: Access detailed notes, sample papers, model papers, and solutions for CBSE, JEE, NEET, and NDA.
       • Practice Papers: Get an extensive collection of practice questions and mock tests to prepare for exams.
       • NCERT Solutions: Find chapter-wise solutions for all subjects aligned with the CBSE curriculum.
       • User-Friendly Interface: Easily navigate through the app to find study resources tailored to your exam needs.

       For teachers, the Examin8 App and Examin8 Website offers the ability to create custom question papers with your own name and logo. This is a fantastic tool for schools and educators to generate personalized exam papers that adhere to the official guidelines and maintain exam integrity. The Class 9 Maths Sample Paper 2024-25 includes all important topics and is designed according to the latest CBSE guidelines. Start your revision early with the Class 9 Maths Sample Paper 2024-25 to avoid last-minute stress.

       Download myCBSEguide for students and Examin8 for teachers to make exam preparation more effective and personalized.

       Class 09 – Mathematics
       Sample Paper – 01 (2024-25)

---

Solution

1. Section A
2. (a)

   x = 0

   Explanation: The value of abscissa or x-corrdinate is always zero at any point on y-axis.

   So, x = 0 is the equation of y-axis.

3. (d)

   96 sq cm

   Explanation: {tex}s = {{12 + 16 + 20} \over 2} = {{48} \over 2}{/tex} = 24 cm
   Area of triangle  = {tex}\sqrt {s\left( {s – a} \right)\left( {s – b} \right)\left( {s – c} \right)} {/tex}
   = {tex}\sqrt {24\left( {24 – 16} \right)\left( {24 – 12} \right)\left( {24 – 20} \right)} {/tex}
   = {tex}\sqrt {24 \times 8 \times 12 \times 4} {/tex} = 12 {tex}\times{/tex} 8 = 96 sq cm

4. (d)

   {tex}{40^o}{/tex}

   Explanation: Angle made by a chord at the centre is twice the angle made by it on any point on the circumference.
   {tex}x={\angle AOB\over2}={80^0\over2}=40^0{/tex}

5. (a)

   {tex}\frac{1}{3} A C{/tex}

   Explanation: Let G be the mid-point of FC and join DG
   
   ​In ∆BCF,
   G is the mid-point of FC and D is the mid-point of BC
   Thus, DG|| BF
   DG || EF
   Now, In ∆ ADG,
   E is the mid-point of AD and EF is parallel to DG.
   Thus, F is the mid-point of AG.
   AF = FG = GC [G is the mid-point of FC] Hence, AF ={tex} \frac13{/tex} AC

6. (c)

   {tex}\frac{1}{2}{/tex}

   Explanation: {tex}\left\{8^{\frac {-4} 3} \div 2^{-2}\right\}^{\frac 1 2}{/tex}
   = {tex}\left[\left(2^{3}\right)^{\frac{-4}{3}} \div 2^{-2}\right]^{\frac{1}{2}}\\{/tex}
   = {tex}\left[2^{3 \times \frac{-4}{3}} \div 2^{-2}\right]^{\frac{1}{2}}\\ {/tex}
   = {tex}\left[2^{-4} \div 2^{-2}\right]^{\frac{1}{2}}\\ {/tex}
   ={tex}\left[2^{-4-(-2)} \right]^{\frac{1}{2}}\\ {/tex}
   ={tex}\left[2^{-4+2} \right]^{\frac{1}{2}}\\ {/tex}
   = {tex}\left[2^{-2} \right]^{\frac{1}{2}}\\ {/tex}
   = {tex} \left(\frac{1}{2^{2}}\right)^{\frac{1}{2}}\\{/tex}
   ={tex} \left(\frac{1}{2}\right)^{2\times\frac{1}{2}}\\{/tex}
   = {tex}\frac{1}{2}{/tex}

7. (b)

   114{tex}^\circ {/tex}

   Explanation: It is an iscosceles triangle and hence angles opposite to equal sides are equal
   Angle PQR and PRQ will be equal. Let suppose Angle PQR be Y
   I.e Y+Y+48=180
   = Y = 66
   X = 180 – 66 = 114

8. (a)

   x + 2y = 8

   Explanation: x + 2y = 8
   When x=2 and y=3 then 2+ 2×3=8

9. (c)

   -2

   Explanation: {tex}{x^2} + kx – 3 = (x – 3)(x + 1){/tex}

   =>     {tex}{x^2} + kx – 3 = {x^2} + \left( { – 3 + 1} \right)x + \left( { – 3} \right) \times 1{/tex}

   =>     {tex}{x^2} + kx – 3 = {x^2} – 2x – 3{/tex}

   On comparing the term, we get {tex}k=-2{/tex}

10. (d)

    1

    Explanation: {tex}\left(\frac{81}{16}\right)^{\frac{-3}{4}} \times\left\{\left(\frac{25}{9}\right)^{\frac{-3}{2}} \div\left(\frac{5}{2}\right)^{-3}\right\}{/tex}
    {tex}\Rightarrow\left(\frac{3}{2}\right)^{4 \times \frac{-3}{4}} \times\left\{\left(\frac{5}{3}\right)^{2 \times \frac{-3}{2}} \div\left(\frac{5}{2}\right)^{-3}\right\}{/tex}
    {tex}\Rightarrow\left(\frac{3}{2}\right)^{-3} \times\left\{\left(\frac{5}{3}\right)^{-3} \div\left(\frac{5}{2}\right)^{-3}\right\}{/tex}
    {tex}\Rightarrow\left(\frac{3}{2}\right)^{-3} \times\left(\frac{5}{3} \times \frac{2}{5}\right)^{-3}{/tex}
    {tex}\Rightarrow\left(\frac{3}{2}\right)^{-3} \times\left(\frac{2}{3}\right)^{-3}{/tex}
    {tex}\Rightarrow\left(\frac{3}{2} \times \frac{2}{3}\right)^{-3}{/tex}
    {tex}\Rightarrow{/tex} (1)^-3 = 1

11. (c)

    12cm

    Explanation:

    
    Applying pythagoras theorem in {tex}\triangle \mathrm { ABC }{/tex}
    {tex}\mathrm { AC } ^ { 2 } = \mathrm { AB } ^ { 2 } + \mathrm { BC } ^ { 2 }{/tex}
    {tex}15 ^ { 2 } = 9 ^ { 2 } + \mathrm { BC } ^ { 2 }{/tex}
    {tex}225 = 81 + \mathrm { BC } ^ { 2 }{/tex}
    {tex}225 – 81 = \mathrm { BC } ^ { 2 }{/tex}
    {tex}\mathrm { BC } ^ { 2 } = 144{/tex}
    BC  = 12 cm

12. (c)

    either terminating or repeating

    Explanation: Rational numbers can be represented in decimal forms rather than representing in fractions. They can easily be represented as decimals by just dividing numerator ‘p’ by denominator ‘q’ (as rational numbers is in the form of p/q).

    A rational number can be expressed as a terminating or nonterminating, recurring decimal.

    For example:

    (i) {tex}5\over 2{/tex} = 2.5,

    {tex}2\over 8{/tex}= 0.25,

    7 = 7.0, etc., are rational numbers which are terminating decimals.

    (ii) {tex}5 \over 9{/tex}= 0.555555555……. = 0.5 ̇,

    {tex}1 \over 6{/tex} = 0.166666 ….. = 0.16 ̇

    {tex}9 \over 11{/tex}= 0.818181…… = 0.81  ̇etc., are rational numbers which are nonterminating, recurring decimals.

13. (a)

    (2, 2)

    Explanation:

    When we put x=2 in the given equation,
    Then, y = (3×2) – 4
    y =6–4 = 2, so point is (2, 2)satisfied the given equation,
    Hence point (2, 2) will lie on the line y = 3x – 4

14. (d)

    60°

    Explanation: Produce OP to intersect RQ at point N.
    Now, OP || RS and transversal RN intersects them at N and R respectively.
    {tex}\therefore{/tex} {tex}\angle R N P=\angle S R N{/tex} (Alternate interior angles)
    {tex}\Rightarrow{/tex} {tex}\angle{/tex}RNP = 130^o
    {tex}\therefore \angle P N Q=180^{\circ}-130^{\circ}=50^{\circ}{/tex}  (Linear pair)
    {tex}\angle O P Q=\angle P N Q+\angle P Q N{/tex} (Exterior angle property)
    {tex}\Rightarrow{/tex} 110^o = 50^o + {tex}\angle P Q N{/tex}
    {tex}\Rightarrow{/tex} {tex}\angle P Q N{/tex} = 110^o – 50^o = 60^o = {tex}\angle P Q R{/tex}

15. (a)

    sometimes rational, sometimes irrational

    Explanation: The product of any two irrational numbers is sometimes rational, sometimes irrational. It depends upon the numbers you choose.
    e,g, {tex}\sqrt 2 \times \sqrt 2 = 2{/tex} is rational whereas {tex}\sqrt 2 \times \sqrt3 = \sqrt 6{/tex} is irrational

16. (c)

    60°

    Explanation: OA = OB {tex}\Rightarrow{/tex} {tex}\angle{/tex}OBA = {tex}\angle{/tex}OAB = 20°.
    In {tex}\triangle{/tex}OAB,
    {tex}\angle{/tex}OAB + {tex}\angle{/tex}OBA + {tex}\angle{/tex}AOB = 180°
    {tex}\Rightarrow{/tex} 20° + 20° + {tex}\angle{/tex}AOB = 180°
    {tex}\Rightarrow{/tex} {tex}\angle{/tex}AOB = 140°.
    OB = OC {tex}\Rightarrow{/tex} {tex}\angle{/tex}OBC = {tex}\angle{/tex}OCB = 50°.
    In {tex}\triangle{/tex}OCB,
    {tex}\angle{/tex}OCB + {tex}\angle{/tex}OBC + {tex}\angle{/tex}COB = 180°
    {tex}\Rightarrow{/tex} 50° + 50° + {tex}\angle{/tex}COB = 180°
    {tex}\Rightarrow{/tex} {tex}\angle{/tex}COB = 80°.
    {tex}\angle{/tex}AOB = 140° {tex}\Rightarrow{/tex} {tex}\angle{/tex}AOC + {tex}\angle{/tex}COB = 140°
    {tex}\Rightarrow{/tex} {tex}\angle{/tex}AOC + 80° = 140°
    {tex}\Rightarrow{/tex} {tex}\angle{/tex}AOC = 140° – 80°
    {tex}\Rightarrow{/tex} {tex}\angle{/tex}AOC = 60°.

17. (a)

    3

    Explanation: The perpendicular distance of any point from x-axis is always equal to the value of ordinate.

18. (b)

    infinitely many solutions

    Explanation: Given linear equation: 3x – 5y = 15 Or, x = {tex}\frac{5 y+15}{3}{/tex}
    When y = 0, x = {tex}\frac{15}{3}{/tex} = 5
    When y = 3, x = {tex}\frac{30}{3}{/tex} = 10
    When y = -3, x = {tex}\frac{0}{3}{/tex} = 0

    xx	5	10	0
    yy	0	3	-3

    Plot the points A(5,0) , B(10,3) and C(0,−3). Join the points and extend them in both the directions.
    We get infinite points that satisfy the given equation.
    Hence, the linear equation has infinitely many solutions.
    

19. (b)

    (x + y + z)(x² + y² + z² – xy – yz – zx)

    Explanation:

    x³ + y³ + z³ – 3xyz = x³ + y³ + 3x²y + 3xy² + z³ – 3xyz – 3x²y – 3xy²
    = (x + y)³ +z³ – 3xy(x + y + z)
    = (x + y + z) ((x + y)² + z² – (x + y)z) – 3xy (x + y + z)
    = (x + y + z) (x² + 2x²y + y² + z2 – xy – xz – 3xy)
    = (x + y + z) (x² + y² + z² – xy – yz – zx)

20. (b)

    Both A and R are true but R is not the correct explanation of A.

    Explanation: In {tex}\Delta{/tex}ABC, E and F are midpoint of the sides AC and AB respectively.
    FE || BC [By mid-point theorem] Now, in {tex}\Delta{/tex}ABP, F is mid-point of AB and FQ || BP. Q is mid-point of AP
    AQ = QP
    

21. (a)

    Both A and R are true and R is the correct explanation of A.

    Explanation: Both A and R are true and R is the correct explanation of A.

    To enhance your exam preparation and practice more questions, download the myCBSEguide app and myCBSEguide Website. This app offers comprehensive study material for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams, making it a one-stop resource for students aiming to excel. With sample papers, mock tests, chapter-wise solutions, and more, myCBSEguide ensures you have all the tools needed to succeed in your exams. Ensure comprehensive exam preparation with the Class 9 Maths Sample Paper 2024-25, available on myCBSEguide.  The Class 9 Maths Sample Paper 2024-25 offers a variety of question types, ensuring a well-rounded revision.

    For teachers, the Examin8 Website and Examin8 app is the ideal solution to create customized question papers with your school’s name and logo. This app helps educators design unique and professional exam papers that adhere to official guidelines.

    Download the myCBSEguide app for expert study material and exam prep resources, and for teachers, the Examin8 app to easily generate personalized question papers.

    Section B

22. Euclid’s Axiom 5 states that “The whole is greater than the part. Since this is true for anything in any part of the world. So, this is a universal truth.
23.  In the above figure, we haveAB = AC + BC = AC + AC = 2AC  (Since, C is the mid-point of AB) ..(1)
    XY = XD + DY = XD + XD = 2XD (Since, D is the mid-point of XY) ..(2)
    Also, AC = XD (Given) ..(3)
    From (1),(2)and(3), we get
    AB = XY, According to Euclid, things which are double of the same things are equal to one another.
24. (i) I quadrant
    (ii) II quadrant
    (iii) III quadrant
    (iv) IV quadrant
25. Let x = {tex}\sqrt{5}-2{/tex} be the rational number
    Squaring on both sides, we get
    x² = {tex}(\sqrt{5}-2)^{2}{/tex}
    x² = 5 + 4 – {tex}4 \sqrt{5}{/tex}
    x² = 9 – {tex}4 \sqrt{5}{/tex}
    {tex}\sqrt{5}{/tex} = {tex}\frac{x^{2}-9}{-4}{/tex} = a rational number.
    {tex}\Rightarrow \sqrt{5}{/tex} is a rational number
    So, we arrive at a contradiction that {tex} \sqrt{5}{/tex} is an irrational number.
    So {tex}(\sqrt{5}-2){/tex} is an irrational number.

    OR

    {tex}\frac{\sqrt{5}}{2 \sqrt{3}}{/tex}
    Multiply both numerator and denominator by {tex}\sqrt{3}{/tex}
    = {tex}\frac{\sqrt{5}}{2 \sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}}{/tex}
    = {tex}\frac{\sqrt{15}}{2 \times 3}{/tex}
    = {tex}\frac{\sqrt{15}}{6}{/tex}

26. Radius of cap (r) = 7 cm, Height of cap (h) = 24 cm
    Slant height of the cone (l) = {tex}\sqrt{{{r}^{2}}+{{h}^{2}}}{/tex} = {tex}\sqrt{{{\left( 7 \right)}^{2}}+{{\left( 24 \right)}^{2}}}{/tex}
    = {tex}\sqrt{49+576}{/tex}
    = {tex}\sqrt{625}{/tex}
    = 25 cm
    Area of sheet required to make a cap = CSA of cone = {tex}\pi rl{/tex}
    = {tex}\frac{22}{7}\times 7\times 25{/tex}
    {tex}=\text{ }550\text{ }c{{m}^{2}}{/tex}=550 cm²
    {tex}\therefore{/tex}  Area of sheet required to make 10 caps =10 {tex}\times{/tex} 550 = 5500 cm²

    OR

    Since diameter of two cones are equal
    {tex}\therefore{/tex} Their radius are equal
    {tex}\therefore{/tex} r₁ = r₂ = r (say)
    Let ratio be x,
    {tex}\therefore{/tex} Slant height ‘l₁‘ of 1^st cone = 4x
    Similarly, slant height ‘l₂‘ of 2^nd cone = 3x
    {tex}\therefore{/tex} {tex}\frac { C . S . A _ { 1 } } { C . S . A _ { 2 } } = \frac { \pi r _ { 1 } l _ { 1 } } { \pi r _ { 2 } l _ { 2 } } = \frac { \pi \times r \times 4 x } { \pi \times r \times 3 x } = \frac { 4 } { 3 }{/tex}

27. Section C
28. Here    a = {tex}\frac {1} {3}{/tex}, b = {tex}\frac {1} {2}{/tex}, n = 3
    {tex}\therefore{/tex} {tex}\frac{b-a}{n+1}=\frac{\frac{1}{2}-\frac{1}{3}}{3+1}{/tex}{tex}=\frac{\frac{3-2}{6}}{4}{/tex}{tex}=\frac{\frac{1}{6}}{4}=\frac{1}{24}{/tex}
    {tex}\therefore{/tex} Three rational numbers between {tex}\frac {1} {3}{/tex} and {tex}\frac {1} {2}{/tex} are
    {tex}\frac{1}{3}+\frac{1}{24}, \frac{1}{3}+2\left(\frac{1}{24}\right), \frac{1}{3}+3\left(\frac{1}{24}\right){/tex}
    {tex}\frac{1}{3}+\frac{1}{24}{/tex}, {tex}\frac{1}{3}+\frac{1}{12}{/tex}, {tex}\frac{1}{3}+\frac{1}{8}{/tex}
    {tex}\frac{3}{8}, \frac{5}{12}, \frac{11}{24}{/tex}
29.  Monthly profits (in Rs) of 100 shops
30. In {tex}\triangle{/tex}BCD, we have
    {tex}\angle{/tex}BDC + {tex}\angle{/tex}DCB + {tex}\angle{/tex}CBD = 180^o
    {tex}\Rightarrow{/tex} 2a + 5a + 3a = 180^o
    {tex}\Rightarrow{/tex} 10a = 180^o
    {tex}\Rightarrow{/tex} a = 18^o
    {tex}\therefore{/tex} {tex}\angle{/tex}C = 5a = 5 {tex}\times{/tex} 18^o = 90^o
    Since opposite angles are equal in a parallelogram. Therefore,
    {tex}\angle{/tex}A = {tex}\angle{/tex}C {tex}\Rightarrow{/tex} {tex}\angle{/tex}A = 90^o
    {tex}\Rightarrow{/tex} 2 ({tex}\angle{/tex}A + {tex}\angle{/tex}B) = 360^o [{tex}\because{/tex} {tex}\angle{/tex}A = {tex}\angle{/tex}C and {tex}\angle{/tex}B = {tex}\angle{/tex}D] {tex}\Rightarrow{/tex} {tex}\angle{/tex}A + {tex}\angle{/tex}B = 180^o
    {tex}\Rightarrow{/tex} 90^o + {tex}\angle{/tex}B = 180^o [{tex}\because{/tex} {tex}\angle{/tex}A = 90^o] {tex}\Rightarrow{/tex} {tex}\angle{/tex}B = 90^o
    Hence, {tex}\angle{/tex}A = 90^o,  {tex}\angle{/tex}B = 90^o {tex}\angle{/tex}C = 90^o and {tex}\angle{/tex}D = 90^o
31. x + y – 4 = 0
    ⇒ y = 4 – x
    Put x = 0, then y = 4 – 0 = 4
    Put x = 1, then y = 4 – 1 = 3
    Put x = 2, then y = 4 – 2 = 2
    Put x = 3, then y = 4 – 3 = 1
    ∴ (0, 4), (1, 3), (2, 2) and (3, 1) are the solutions of the equation x + y – 4 = 0
32. the population of Delhi State in different census years

    OR

    Here, minimum class size = 2 – 1 = 1
    Adjusted frequency of a class = {tex}\frac{Minimum \ class \ size}{Class \ size \ of \ the \ class}{/tex} {tex}\times {/tex} Frequency of the class
    Frequency distribution after adjusting frequency

    Age (in years)	Number of children (Frequency)	Width of the class	Adjusted frequency
    1-2	5	1	{tex}\frac{1}{1}{/tex} {tex}\times{/tex} 5 = 5
    2-3	4	1	{tex}\frac{1}{1}{/tex} {tex}\times{/tex} 4 = 4
    3-5	10	2	{tex}\frac{1}{2}{/tex}{tex}\times{/tex}10 = 5
    5-7	12	2	{tex}\frac{1}{2}{/tex}{tex}\times{/tex}12 = 6
    7-10	9	3	{tex}\frac{1}{3}{/tex} {tex}\times{/tex} 9 = 3
    10-15	10	5	{tex}\frac{1}{5}{/tex} {tex}\times{/tex} 10 = 2
    15-17	8	2	{tex}\frac{1}{2}{/tex} {tex}\times{/tex} 8 = 4

    The required histogram is as follows
    

33. {tex}{x^3} + 13{x^2} + 32x + 20{/tex}
    We need to consider the factors of 20, which are {tex} \pm 5, \pm 4, \pm 2, \pm 1{/tex}
    Let us substitute -1 in the polynomial {tex}{x^3} + 13{x^2} + 32x + 20{/tex}, to get
    {tex}{\left( { – 1} \right)^3} + 13{\left( { – 1} \right)^2} + 32\left( { – 1} \right) + 20 = – 1 + 13 – 32 + 20\,\, = – 20 + 20\,\, = 0{/tex}
    Thus, according to factor theorem, we can conclude that {tex}\left( {x + 1} \right){/tex} is a factor of the polynomial {tex}{x^3} + 13{x^2} + 32x + 20{/tex}
    Let us divide the polynomial {tex}{x^3} + 13{x^2} + 32x + 20 by \left( {x + 1} \right){/tex},to get
    
    {tex}{x^3} + 13{x^2} + 32x + 20{/tex}
    {tex}= \left( {x + 1} \right)\left( {{x^2} + 12x + 20} \right){/tex}
    {tex}= \left( {x + 1} \right)\left( {{x^2} + 2x + 10x + 20} \right){/tex}
    {tex} = \left( {x + 1} \right)\left[ {x\left( {x + 2} \right) + 10\left( {x + 2} \right)} \right]{/tex}
    Therefore, we can conclude that on factorizing the polynomial {tex}{x^3} + 13{x^2} + 32x + 20{/tex}, we get
    {tex}\left( {x + 1} \right)\left( {x – 10} \right)\left( {x + 2} \right){/tex}
34. Section D
35. 
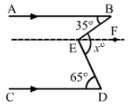
    Draw EF {tex}\parallel{/tex} AB {tex}\parallel{/tex} CD
    Now, AB {tex}\parallel{/tex} EF and BE is the transversal.
    Then,
    {tex}\angle A B E=\angle B E F{/tex} [Alternate Interior Angles] {tex}\Rightarrow \angle B E F=35^{\circ}{/tex}
    Again, EF {tex}\parallel{/tex} CD and DE is the transversal
    Then,
    {tex}\angle D E F=\angle F E D{/tex}
    {tex}\Rightarrow \angle F E D=65^{\circ}{/tex}
    {tex}\therefore x^{\circ}=\angle B E F+\angle F E D{/tex}
    x{tex}^\circ{/tex}= 35{tex}^\circ{/tex} + 65°
    x{tex}^\circ{/tex}= 100°

    OR

    We know that if two lines intersect, then the vertically-opposite angles are equal.
    Let {tex}\angle B O C=\angle A O D=x^{\circ}{/tex}
    {tex}\angle B O C+\angle A O D{/tex} = 280{tex}^{\circ}{/tex}
    x + x = {tex}280^{\circ}{/tex}
    {tex}\Rightarrow{/tex} 2x = {tex}280^{\circ}{/tex}
    {tex}\Rightarrow{/tex} x =  {tex}140^{\circ}{/tex}
    {tex}\therefore \angle B O C=\angle A O D=140^{\circ}{/tex}
    Also, let {tex}\angle A O C=\angle B O D=y^{\circ}{/tex}
    We know that the sum of all angles around a point is 360°
    {tex}\therefore \angle A O C+\angle B O C{/tex}{tex}+\angle B O D+\angle A O D=360^{\circ}{/tex}
    {tex}\Rightarrow{/tex} y + 140 + y + 140 = 360°
    {tex}\Rightarrow{/tex} 2y = {tex}80^{\circ}{/tex}
    {tex}\Rightarrow{/tex} y = 40°
    Hence, {tex}\angle A O C=\angle B O D=40^{\circ}{/tex}
    {tex}\therefore \angle B O C=\angle A O D=140^{\circ}{/tex} and {tex}\angle A O C=\angle B O D=40^{\circ}{/tex}

36. Height of the conical tent {tex}\left( h \right){/tex} = 8 m and Radius of the conical tent {tex}\left( r \right){/tex} = 6 m
    Slant height of the tent {tex}\left( l \right)=\sqrt{{{r}^{2}}+{{h}^{2}}}{/tex}
    ={tex}\sqrt{{{\left( 6 \right)}^{2}}+{{\left( 8 \right)}^{2}}}{/tex}
    ={tex}\sqrt{36+64}{/tex}
    ={tex}\sqrt{100}{/tex}
    = 10 m
    Area of tarpaulin = Curved surface area of tent = {tex}\pi rl{/tex} {tex}=3.14\times 6\times 10=188.4\text{ }{{m}^{2}}{/tex}
    Width of tarpaulin = 3 m
    Let Length of tarpaulin = L
    {tex}\therefore {/tex} Area of tarpaulin = {tex}Length\times Breadth\text{ }=\text{ }L\times 3{/tex} = 3L
    Now According to question, 3L = 188.4
    {tex}\Rightarrow {/tex} L = 188.4/3 = 62.8 m
    The extra length of the material required for stitching margins and cutting is 20 cm = 0.2 m.
    So the total length of tarpaulin bought is (62.8 + 0.2) m = 63 m
37. Let:
    a = 85 m and b = 154 m
    Given that perimeter = 324 m
    Perimeter= 2s = 324 m
    {tex}\Rightarrow{/tex} s ={tex}\frac{324}{2}{/tex}m
    or, a + b + c = 324
    {tex}\Rightarrow{/tex} c = 324 – 85 – 154 = 85 m
    By Herons’s formula, we have:
    Area of triangle {tex}=\sqrt{s(s-a)(s-b)(s-c)}{/tex}
    {tex}=\sqrt{162(162-85)(162-154)(162-85)}{/tex}
    {tex}=\sqrt{162 \times 77 \times 8 \times 77}{/tex}
    {tex}=\sqrt{1296 \times 77 \times 77}{/tex}
    {tex}=\sqrt{36 \times 77 \times 77 \times 36}{/tex}
    {tex}=36 \times 77{/tex}
    = 2772 m²

    OR

    Let:
    a = 91 m, b = 98 m, and c = 105 m
    {tex}\therefore s=\frac{a+b+c}{2}{/tex}{tex}=\frac{91+98+105}{2}{/tex} = 147 m
    {tex}\Rightarrow{/tex} s = 147 m
    By Heron’s formula, we have:
    Area of triangle {tex}=\sqrt{s(s-a)(s-b)(s-c)}{/tex}
    {tex}=\sqrt{147(147-91)(147-98)(147-105)}{/tex}
    {tex}=\sqrt{147 \times 56 \times 49 \times 42}{/tex}
    {tex}=\sqrt{7 \times 3 \times 7 \times 2 \times 2 \times 2 \times 7 \times 7 \times 7 \times 7 \times 3 \times 2}{/tex}
    {tex}=7 \times 7 \times 7 \times 2 \times 3 \times 2{/tex}
    = 1446 m²
    We know that the longest side is 105 m.
    Thus, we can find out the height of the triangle corresponding to 42 cm.
    Area of triangle = 4116 m²
    {tex}\Rightarrow \frac{1}{2} \times{/tex} Base {tex}\times{/tex} Height = 4116  {tex}\Rightarrow \frac{1}{2} \times{/tex}(105)(Height) = 4116
    {tex}\Rightarrow{/tex} Height {tex}=\frac{4116 \times 2}{105}={/tex} 78.4 m

38. Let p(x) = ax³ + bx² + x – 6 be the given polynomial.
    Now,
    (x + 2) is a factor of p(x)
    {tex}\Rightarrow{/tex} p (-2) = 0 [{tex}\because{/tex} x + 2 = 0 {tex}\Rightarrow{/tex} x = -2] {tex}\Rightarrow{/tex} a(-2)³ + b(-2)² + (-2) – 6 = 0
    {tex}\Rightarrow{/tex} -8a + 4b – 2 – 6 = 0 {tex}\Rightarrow{/tex} -8a + 4b = 8 {tex}\Rightarrow{/tex} -2a + b = 2 …(i)
    It is given that p(x) leaves the remainder 4 when it is divided by (x – 2).
    {tex}\therefore{/tex} p(2) = 4 [{tex}\because{/tex} x – 2 = 0 {tex}\Rightarrow{/tex} x = 2] {tex}\Rightarrow{/tex} a(2)³ + b(2)² + 2 – 6 = 4
    {tex}\Rightarrow{/tex} 8a + 4b – 4 = 4 {tex}\Rightarrow{/tex} 8a + 4b = 0 {tex}\Rightarrow{/tex} 2a + b = 2 …(ii)
    Adding (i) and (ii), we get
    2b = 4 {tex}\Rightarrow{/tex} b = 2
    Putting b = 2 in (i), we get
    – 2a + 2 = 2 {tex}\Rightarrow{/tex} -2a = 0 {tex}\Rightarrow{/tex} a = 0.
    Hence, a = 0 and b = 2
39. Section E
    1. Let the no of questions whose answer is known to Ajay be x and number questions attempted by guessing be y.
       x + y = 110
       x + 14y = 80 {tex}\Rightarrow{/tex} 4x + y = 320x + y = 110 …(1)
       4x + y = 320 …(2)
       Solving (1) and (2)
       x + y – 4x – y = 110 – 320 = -210
       {tex}\Rightarrow{/tex} -3x = -210
       {tex}\Rightarrow{/tex} x = 70
    2. x + y = 110
       x + 14y = 80 {tex}\Rightarrow{/tex} 4x + y = 320
       x + y = 110 …(1)
       4x + y = 320 …(2)
       Solving (1) and (2)
       x + y – 4x – y = 110 – 320 = – 210
       {tex}\Rightarrow{/tex} – 3x = – 210
       {tex}\Rightarrow{/tex} x = 70
       Put x = 70 in (1)
       70 + y = 110
       {tex}\Rightarrow{/tex} y = 40
       40 question he answered by guessing.
    3. {tex}70 – 40 \times \frac{1}{4} = 70 – 10{/tex} = 60 marks
       He scored 60 marks.x – {tex}\frac{1}{4}{/tex}(110 – x) = 95
       OR
       {tex}\Rightarrow{/tex} 4x – 110 + x = 380
       {tex}\Rightarrow{/tex} 5x = 380 + 110 = 490
       {tex}\Rightarrow{/tex} x = {tex}\frac{490}{5}{/tex} = 98
       So he answered 98 correct answers 12 by guessing.
    1. In {tex}\triangle{/tex}APC and {tex}\triangle{/tex}BPC
       AP = BP (Given)
       CP = CP (common side)
       {tex}\angle{/tex}ACP = {tex}\angle{/tex}BCP = 90^o
       By RHS criteria {tex}\triangle{/tex}APC {tex}\cong{/tex} {tex}\triangle{/tex}BPC
    2. In {tex}\triangle{/tex}ACP
       {tex}\angle{/tex}APC + {tex}\angle{/tex}PAC + {tex}\angle{/tex}ACP = 180^o
       {tex}\Rightarrow{/tex} x + 60^o + 90^o = 180^o (angle sum property of {tex}\triangle{/tex})
       {tex}\Rightarrow{/tex} {tex}\angle{/tex}x = 180^o – 150^o = 30^o
       {tex}\angle{/tex}x = 30^o
    3. In {tex}\triangle{/tex}APC and {tex}\triangle{/tex}BPC
       Corresponding part of congruent triangle
       {tex}\angle{/tex}X = {tex}\angle{/tex}Y
       {tex}\Rightarrow{/tex} {tex}\angle{/tex}Y = 30^o (given {tex}\angle{/tex}X = 30^o)
       OR
       In {tex}\triangle{/tex}APC and {tex}\triangle{/tex}BPC
       Corresponding part of congruent triangle
       {tex}\angle{/tex}PAC = {tex}\angle{/tex}PBC
       {tex}\Rightarrow{/tex} {tex}\angle{/tex}PBC = 60^o (given {tex}\angle{/tex}PAC = 60^o)
    1. In {tex}\Delta{/tex}AOP and {tex}\Delta{/tex}BOP
       {tex}\angle{/tex}APO = {tex}\angle{/tex}BPO (Given)
       OP = OP (Common)
       AO = OB (radius of circle)
       {tex}\Delta{/tex}AOP {tex}\cong{/tex} {tex}\Delta{/tex}BOP
       AP = BP (CPCT)
    2. In right {tex}\Delta{/tex}COQ
       CO² = OQ² + CQ²
       {tex}\Rightarrow{/tex} 10² = 8² + CQ²
       {tex}\Rightarrow{/tex} CQ² = 100 – 64 = 36
       {tex}\Rightarrow{/tex} CQ = 6
       CD = 2CQ
       {tex}\Rightarrow{/tex} CD = 12 cm
    3. In right {tex}\Delta{/tex}AOB
       AO² = OP² + AP²
       {tex}\Rightarrow{/tex} 10² = 6² + AP²
       {tex}\Rightarrow{/tex} AP² = 100 – 36 = 64
       {tex}\Rightarrow{/tex} AP = 8
       AB = 2AP
       {tex}\Rightarrow{/tex} AB = 16 cm
       OR
       There is one and only one circle passing through three given non-collinear points.
       To improve your exam performance, download the myCBSEguide app and myCBSEguide Website for access to complete study materials for CBSE, NCERT, JEE (Main), NEET-UG, and NDA exams. The app offers practice questions, sample papers, mock tests, and NCERT solutions, making it an essential resource for students aiming for top scores. Whether you need to revise concepts or test your knowledge, myCBSEguide provides everything to help you prepare effectively.For teachers, the Examin8 Website and Examin8 app is the perfect tool to create personalized question papers. Teachers can design custom exams with their own name and logo, streamlining the paper creation process while maintaining exam integrity. Solving the Class 9 Maths Sample Paper 2024-25 will help students familiarize themselves with the exam pattern.Download myCBSEguide now for comprehensive study material and exam resources, and Examin8 for teachers to create customized question papers easily.

Sample Papers for Class 9 all subjects 2024-25

• Mathematics
• Science
• Social Science
• English Language and Literature
• Hindi  A
• Hindi B

To excel in your Class 9 exams, download sample papers for Science, Social Science, Mathematics, English Communicative, English Language and Literature, Hindi Course A, and Hindi Course B from the myCBSEguide app and myCBSEguide Website. These sample papers are designed to help students practice effectively and boost their exam preparation. The Class 9 Maths Sample Paper 2024-25 is an essential resource for students preparing for their final exams. Download the Class 9 Maths Sample Paper 2024-25 from myCBSEguide for accurate exam preparation.

myCBSEguide offers a wide range of resources, including:

• Sample Papers with Solutions: Practice with real exam-like questions and detailed solutions.
• Chapter-wise Test Papers: Ideal for focused practice on each subject.
• NCERT Solutions & NCERT Exemplar Solutions: Complete solutions for all NCERT exercises.
• Quick Revision Notes: Ready-to-refer notes for last-minute revision.
• CBSE Guess Papers & Important Question Papers: Focus on high-weightage topics.

Download the myCBSEguide app today for easy access to all these resources and get the best exam preparation materials in one place!