16. Find a G.P. for which sum of the first two terms is 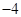 and the fifth term is 4 times the third term.
and the fifth term is 4 times the third term.
Ans. Let 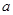 be the first term and
be the first term and 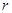 be the common ratio of given G.P.
be the common ratio of given G.P.
Given: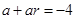

 ……..(i)
……..(i)
And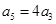

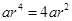

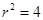

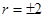
Putting 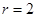 in eq. (i), we get
in eq. (i), we get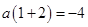

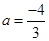
Therefore, required G.P. is 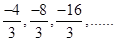
Putting 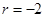 in eq. (i), we get
in eq. (i), we get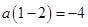

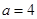
Therefore, required G.P. is 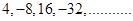
17. If the 4th, 10th and 16th terms of a G.P. are 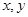 and
and 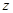 respectively. Prove that
respectively. Prove that 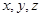 are in G.P.
are in G.P.
Ans. Let 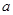 be the first term and
be the first term and 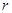 be the common ratio of given G.P.
be the common ratio of given G.P.
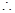


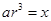 ……….(i)
……….(i)
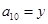

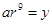 ……….(i)
……….(i)
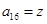

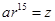 ……….(i)
……….(i)
From eq. (ii), 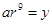

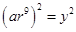

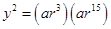

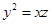 [From eq. (i) and (iii)]
[From eq. (i) and (iii)]
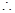
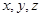 are in G.P.
are in G.P.
18. Find the sum to 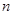 terms of the sequences 8, 88, 888, 8888, ……
terms of the sequences 8, 88, 888, 8888, ……
Ans. Here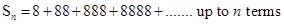

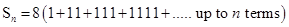

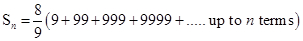

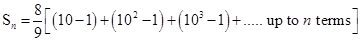

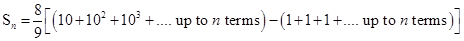

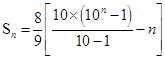
= 
= 
19. Find the sum of the product of the corresponding terms of the sequences 2, 4, 16, 32 and 128, 32, 8, 2, 
Ans. Multiplying the corresponding terms of the given sequences 2, 4, 16, 32 and 128, 32, 8, 2, 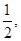


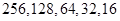 are in G.P.
are in G.P.
Here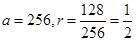 and
and 
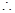
 when
when 

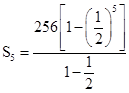 =
= 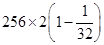

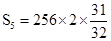 = 496
= 496
20. Show that the products of the corresponding terms of the sequences 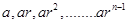 and
and 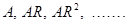
 form a G.P. and find the common ratio.
form a G.P. and find the common ratio.
Ans. Multiplying the corresponding terms of the given sequences, we have
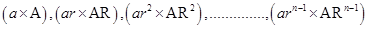

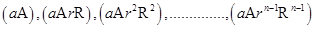 are in G.P.
are in G.P.
Here First term =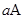 and common ratio =
and common ratio = 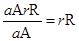
21. Find four numbers forming a geometric progression in which the third term is greater than the first term by 9 and the second term is greater than by 4th by 18.
Ans. Let the four numbers in G.P. be 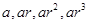
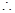
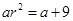 and
and 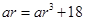
Now,

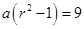 ……….(i)
……….(i)
And



 ……….(ii)
……….(ii)
Dividing eq. (ii) by eq. (i), we have
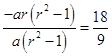

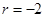
Putting value of 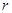 in eq. (i), we get
in eq. (i), we get


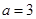
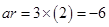
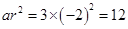
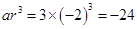
Therefore, the required numbers are 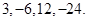
22. If the 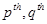 and
and 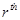 terms of a G.P. are
terms of a G.P. are  and
and 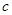 respectively. Prove that
respectively. Prove that 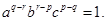
Ans. Let A be the first term and R be the common ratio of given G.P.
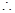
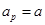

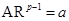 ……….(i)
……….(i)
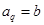

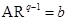 ……….(ii)
……….(ii)
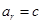

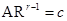 ……….(iii)
……….(iii)
Now, L.H.S. = 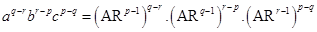
= 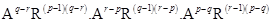
= 
= 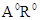 =
=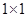 = 1 = R.H.S.
= 1 = R.H.S.
23. If the first and the 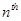 term of a G.P. are
term of a G.P. are 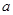 and
and 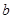 respectively and if P is the product of
respectively and if P is the product of 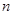 terms, prove that
terms, prove that 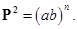
Ans. Here, first term of G.P. is 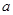
And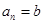

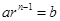

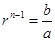 ……….(i)
……….(i)
Given: P = 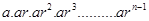
 P =
P = 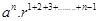

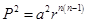 [Squaring both sides]
[Squaring both sides]

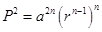

 [From eq. (i)]
[From eq. (i)]



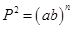
24. Show that the ratio of the sum of first 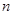 terms of a G.P. to the sum of terms from
terms of a G.P. to the sum of terms from 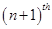 to
to 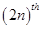 term is
term is 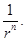
Ans. Let 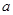 be the first term and
be the first term and 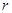 be the common ratio of given G.P.
be the common ratio of given G.P.
Then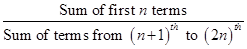
= 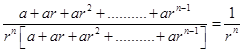
25. If 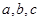 and
and  are in G.P., show that
are in G.P., show that 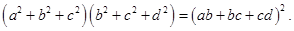
Ans. Let 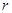 be the common ratio of given G.P.
be the common ratio of given G.P.
Then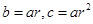 and
and 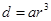
Now, L.H.S. = 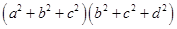
= 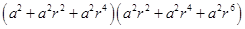
= 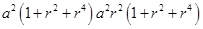 =
= 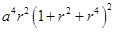
R.H.S. = 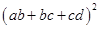
= 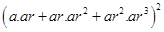
= 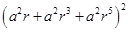
= 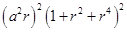 =
= 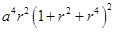
Therefore, L.H.S. = R.H.S.
26. Insert two numbers between 3 and 81 so that the resulting sequence us G.P.
Ans. Let  be two numbers between 3 and 81 such that
be two numbers between 3 and 81 such that 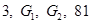 are in G.P.
are in G.P.
Here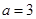 and
and 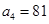

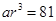

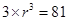

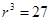


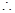
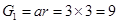
And 
Therefore, the required numbers are 9 and 27.
27. Find the value of 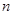 so that
so that 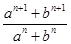 may be the geometric mean between
may be the geometric mean between 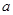 and
and 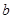 .
.
Ans. Since, G.M. between two numbers 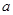 and
and 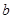 is
is 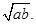
According to question,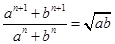



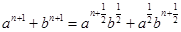

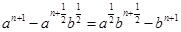

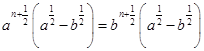

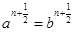

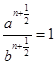

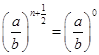

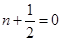

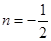
28. The sum of two numbers is 6 times their geometric mean, show that numbers are in the ratio 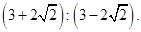
Ans. Given: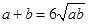

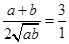
Applying component and dividend, we get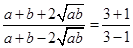

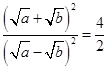


Again, Applying component and dividend, we get
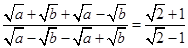

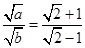
Squaring both sides,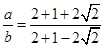

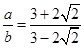
Therefore, the numbers are in the ratio 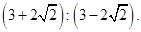
29. If A and G be A.M. and G.M. respectively between two positive numbers, prove that the numbers are 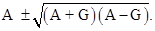
Ans. Given: A = 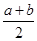 and G =
and G = 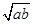
Now,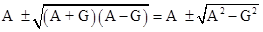
= 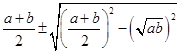
= 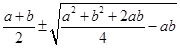
= 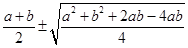
= 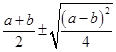 =
= 
= 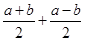 and
and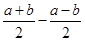
=  and
and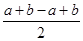
= 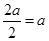 and
and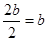
30. The number of bacteria in a certain culture doubles every hour. If there were 30 bacteria present in the culture originally, how many bacteria will be present at the end of 2nd hour, 4th hour and 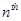 hour?
hour?
Ans. Bacteria present in the culture originally = 30
Since the bacteria doubles itself after each hour, then the sequence of bacteria after each hour is a G.P.
Here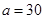 and
and 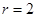
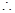 Bacteria at the end of 2nd hour =
Bacteria at the end of 2nd hour = 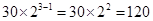
And Bacteria at the end of 4th hour = 
And Bacteria at the end of 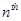 hour =
hour = 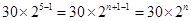
31. What will Rs. 500 amount to 10 years after its deposit in a bank which pays annual interest rate of 10% compounded annually?
Ans. Original amount = Rs. 500, Rate of interest = 10% compounded annually
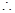 Interest of one year =
Interest of one year = 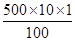 = Rs. 50
= Rs. 50
And Amount after one year = 500 + 50 = Rs. 550
Here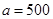 and
and 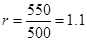
Therefore, amount after 10 years = 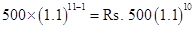
32. If A.M. and G.M. of roots of a quadratic equation are 8 and 5 respectively then obtain the quadratic equation.
Ans. Let 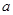 and
and  be the roots of required quadratic equation.
be the roots of required quadratic equation.
Then A.M. = 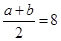

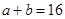
And G.M. = 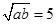

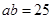
Now, Quadratic equation 

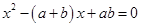

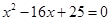
Therefore, required equation is 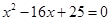 .
.

Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
Learn8 App
Practice unlimited questions for Entrance tests & government job exams at ₹99 only
Install Now