CBSE > Class 11 > Physics | CBSE Board | Homework Help

Ask questions which are clear, concise and easy to understand.

Ask Question

Inertia??

Posted by Aadya Singh 4 years, 11 months ago

CBSE > Class 11 > Physics

0 answers

ANSWER

Moment of inertia of solid sphere... ✍✍✍✍

Posted by ?????? ?? 4 years, 11 months ago

CBSE > Class 11 > Physics

2 answers

Pk ⚔️ 4 years, 11 months ago

Hello bhai....kaise ho?? Pahchana?...padhai kaise chal rahi??.....ghar me sab badhiya?

2Thank You

Yogita Ingle 4 years, 11 months ago

Moment of inertia of sphere is normally expressed as;

I = ⅖ MR²

Here, r and m are the radius and mass of the sphere respectively. Students have to keep in mind that we are talking about the moment of inertia of a solid sphere about its central axis above. Additionally, if we talk about the moment of inertia of the sphere about its axis on the surface it is expressed as;

I = 7/5 MR²

1Thank You

ANSWER

Three forces 3 Newton 5 Newton and 4 newton act simultaneously on a particle along east west and South respectively calculate the magnitude and direction of the resultant force

Posted by Devanshi Jain 4 years, 11 months ago

CBSE > Class 11 > Physics

2 answers

Dεερακ Ȿιηɠꜧ 4 years, 11 months ago

Resulting force : 2N along west, 4N along south
Total force = √(2²+4²) = √20 = 2√5N along south west

1Thank You

Yogita Ingle 4 years, 11 months ago

The magnitude of three forces 3N,4N and 5N will be zero, if these vectors from a close polygon will all the sides in the same order as shown in figure. Hence,the acceleration of body may be zero

1Thank You

ANSWER

Can a single nucleus emit alpha,beta and gamma ray together

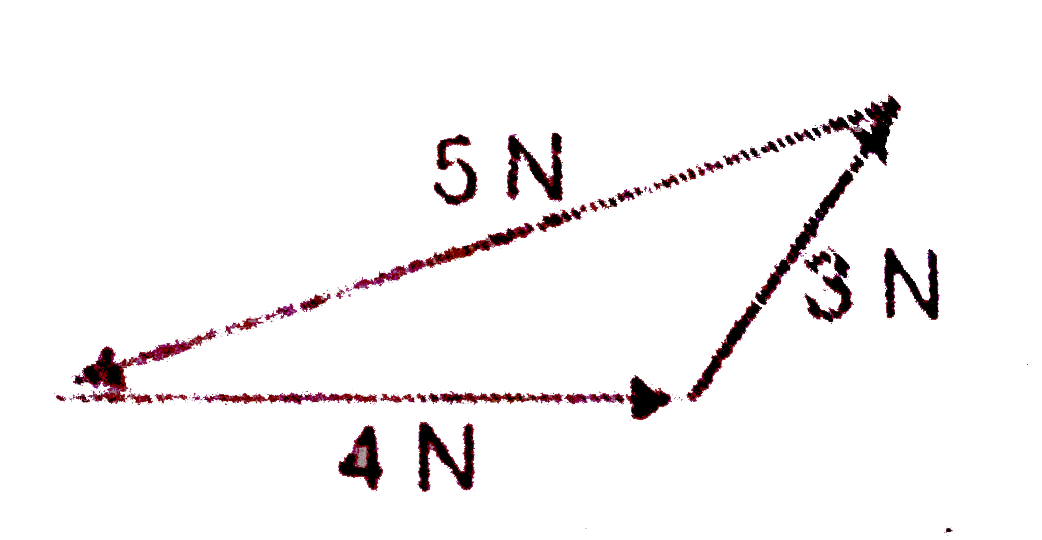
Posted by Priya Negi 4 years, 11 months ago

CBSE > Class 11 > Physics

1 answers

Yogita Ingle 4 years, 11 months ago

No, the radioactive nucleus does not emit alpha and beta radiations simultaneously. Some nucleus emit alpha particles, others beta particles and changes into a new nucleus and to balance the energy, gamma radiations are emitted.

0Thank You

ANSWER

Tomorrow is my physics test of Chptr 1 & 2 of Unit 5... Plz tell some important articles what I have to do.. +1 Non-medical PHYSICS PART-2... PLZ TELL

Posted by Sadul Sharma 4 years, 11 months ago

CBSE > Class 11 > Physics

1 answers

Sadul Sharma 4 years, 11 months ago

Motion of system of particles and rigid body (unit name -5) Chptr names_centre of mass(ch-1)......... Rotational motion (ch-2)

1Thank You

ANSWER

Prove g m1m2/r2

Posted by Palak Sharma 4 years, 11 months ago

CBSE > Class 11 > Physics

0 answers

ANSWER

the density of mercury is 13.6 g/cm3. convert this to kg/m3

Posted by Himanshu Sharma 4 years, 11 months ago

CBSE > Class 11 > Physics

1 answers

Yogita Ingle 4 years, 11 months ago

SI unit of density is Kg/m³
1 m = 100 cm => 1 cm = 10-² m
1 Kg = 1000g => 1g = 10-³ Kg

now,
13.6 g/cm³ = 13.6 × ( 10-³)/(10-²)³ Kg/m³

= 13.6 × 10-³/10^-6 Kg/m³

= 13.6 × 10^(-3 + 6) Kg/m³

= 13.6 × 10³ Kg/m³

0Thank You

ANSWER

Expression for energy stored in string

Posted by Pooja Kirola 4 years, 11 months ago

CBSE > Class 11 > Physics

1 answers

Gaurav Seth 4 years, 11 months ago

Hooke’s Law, F = -kx, where F is the force and x is the elongation.
The work done = energy stored in stretched string = F.dx
The energy stored can be found from integrating by substituting for force,
and we find,
The energy stored = kx²/2, where x is the final elongation.
The energy density = energy/volume
= (kx²/2)/(AL)
=1/2(kx/A)(x/L)
= 1/2(F/A)(x/L)
= 1/2(stress)(strain)

0Thank You

ANSWER

Convert into 100 Dyne Newton

Posted by Himanshu Sharma 4 years, 11 months ago

CBSE > Class 11 > Physics

2 answers

Abirami Kamalbabu 4 years, 10 months ago

1N = 10^5 dyne 100 dyne = 10^2 dyne 10^2 / 10^5 = 10^-3 N So 100 dyne = 10^-3 N

0Thank You

Aman Dahiya 4 years, 11 months ago

100 dyne = 100 gcms-² 1000g=1kg 100cm=1m 100 dyne = 100×1/1000×100 N = 0.001 N

1Thank You

ANSWER

Convert 100 into Newton

Posted by Himanshu Sharma 4 years, 11 months ago

CBSE > Class 11 > Physics

1 answers

Aman Dahiya 4 years, 11 months ago

1 N = 1 kg ms-² = 1000 g ×100 m ×1 s-²= 100000 gcms-² =100000 dyne 100 N = 100 ×100000 dyne = 10000000 dyne

0Thank You

ANSWER

Calculate the angle 1min in radian

Posted by Shivraj Bhartiya 4 years, 11 months ago

CBSE > Class 11 > Physics

2 answers

Dεερακ Ȿιηɠꜧ 4 years, 11 months ago

π/60×180 = π/ 10800

1Thank You

Yogita Ingle 4 years, 11 months ago

We know that,

360° = 2π radians

Hence, 1° = 2 π/ 360 → (1)

10° = [2 π/ 360° ] x 10 = π/ 18 radians

60 minutes = 1°

Substituting (1),

60 minutes = 2 π/ 360

One minute = 2 π/ (360 x 60)

One minute = π/ 10800 radians → (2)

0Thank You

ANSWER

Any bes time table for studying

Posted by Chaithra. H Chaithra. H 4 years, 11 months ago

CBSE > Class 11 > Physics

1 answers

Class 12 4 years, 11 months ago

Every timetable is best timetable, Its up to you that ,are u gonna follow it up in a best way... Thats all what i can say

2Thank You

ANSWER

How to study physics yrr?

Posted by ⠀ 4 years, 11 months ago

CBSE > Class 11 > Physics

3 answers

Har Har Mahadev 🙏 4 years, 11 months ago

Practice numericals, learn concept .

0Thank You

Pk ⚔️ 4 years, 11 months ago

Ham sab yek hi kasti me sawar hai???

2Thank You

⠀ 4 years, 11 months ago

Physicsafter 10 has become so dimag ghumau. In theory its ok , but in numericals it creates a problem

2Thank You

ANSWER

Convert into 10 joule to into erg

Posted by Himanshu Sharma 4 years, 11 months ago

CBSE > Class 11 > Physics

2 answers

Dεερακ Ȿιηɠꜧ 4 years, 11 months ago

1J= 10⁷erg
10J = 10×10⁷ erg = 10⁸erg

0Thank You

Yogita Ingle 4 years, 11 months ago

we know, 1 Joule = 1 Kg m²/s²
1 erg = 1 g cm²/s²
so, 1 Joule = 1Kg m²/s²
= {1000 g} {100cm}²/s². [ 1kg = 1000g and 1m = 100cm]
= 1000 × 10000 g cm²/s²
= 10000000 erg
hence, 1 Joule = 10⁷ erg
so, 10 Joule = 10 × 10⁷ erg = 10⁸ erg

2Thank You

ANSWER

Posted by Pranshi Dwivedi 4 years, 11 months ago

CBSE > Class 11 > Physics

1 answers

Yash Rawat 4 years, 11 months ago

True, But not always, because magnitude of any vector or vectors taken separately is always greater than that of which are taken together, But this idea becomes wrong when vectors which are taken together have same sign because in this case there is effective addition of magnitude of both vectors and then there result is equal to that which are taken seperately.

0Thank You

ANSWER

What is principal of superposition??

Posted by Pk ⚔️ 4 years, 11 months ago

CBSE > Class 11 > Physics

1 answers

Yogita Ingle 4 years, 11 months ago

Considering two waves, travelling simultaneously along the same stretched string in opposite directions as shown in the figure above. We can see images of <a href="https://byjus.com/physics/wave-theory-of-light/">waveforms</a> in the string at each instant of time. It is observed that the net displacement of any element of the string at a given time is the algebraic sum of the displacements due to each wave.

Let us say two waves are travelling alone and the displacements of any element of these two waves can be represented by y₁(x, t) and y₂(x, t). When these two waves overlap, the resultant displacement can be given as y(x,t).

Mathematically, y (x, t) = y₁(x, t) + y₂(x, t)

As per the principle of superposition, we can add the overlapped waves algebraically to produce a resultant wave. Let us say the wave functions of the moving waves are

y₁ = f1(x–vt),

y₂ = f2(x–vt)

y_n = f_n (x–vt)

then the wave function describing the disturbance in the medium can be described as

y = f₁(x – vt)+ f₂(x – vt)+ …+ f_n(x – vt)

or, y=∑ i=1 to n = fi (x−vt)

1Thank You

ANSWER

For MCQ class-11 which book is right.

Posted by Aakash Kumar 4 years, 11 months ago

CBSE > Class 11 > Physics

4 answers

Divyanshu Barnwal 4 years, 11 months ago

NEET Champion for med. JEE Champion for non med.

0Thank You

Divyanshu Barnwal 4 years, 11 months ago

JEE Champion

0Thank You

Kya Kroge Naam Jaankar 4 years, 11 months ago

Dc pandey

0Thank You

Kalki Kalki 4 years, 11 months ago

Arihant

1Thank You

ANSWER

Mai need bataya tha na ki school opan ho gay

Posted by Annu Yadav 4 years, 11 months ago

CBSE > Class 11 > Physics

1 answers

Aakash Kumar 4 years, 11 months ago

School will be open after 1 month.

0Thank You

ANSWER

What are significant figures

Posted by Adarsh Kaurav 4 years, 11 months ago

CBSE > Class 11 > Physics

1 answers

Sachi Chauhan 4 years, 11 months ago

Significant figures of a number written in positional notation are the digits that carry meaningful contribution in measurnments.it is used to get an accurate and precise answer

0Thank You

ANSWER

Energy of projectile

Posted by Adarsh Kaurav 4 years, 11 months ago

CBSE > Class 11 > Physics

0 answers

ANSWER

.The ceiling of a long hall is 25 m high. What is the maximum horizontal distance that a ball thrown with a speed of 40 m s -1 can go without hitting the ceiling of the hall? 14. What is collision? Derive expressions for one dimensional collision. 15. Derive the expressions for the energy stored in spring? 16. Derive the expression for banking road? 17. Derive the expression for work energy theoram for constant force. 18. What is triangular law of vectors. 19. What is projectile motion? Derive the expression for maximum height? 20. Derive the expression for centrifugal force. 21.Derive the expressions for first second and third law of motion.

Posted by Tanushree Namdeo 4 years, 11 months ago

CBSE > Class 11 > Physics

0 answers

ANSWER

Check the correctness of physical equation s=ut+1/2at2 where u is the initial velocity v is the final velocity a is the acceleration s is the displacement and t is that time in which the the charge occurs

Posted by Chandrakala.S Chandu.S 4 years, 11 months ago

CBSE > Class 11 > Physics

1 answers

Parush Kumar 4 years, 11 months ago

s is displacement hear so the dimensions formula of s is [L] We know that we can add and subtract only those quantity which have same unit so dimensions formula of ut and 1/2at² is same Now u is initnial velocity it's dimensions formula is =[LT^-1] And t is time so =[T] ut dimensions formula is [L] The formula s=ut+1/2at² is dimensionally correct

0Thank You

ANSWER

Itis given that A=Bsquare . If A=100+-plus Minus 0.20,then B equal to?

Posted by Aman Bahuguna 4 years, 11 months ago

CBSE > Class 11 > Physics