Ask questions which are clear, concise and easy to understand.
Ask QuestionPosted by Ritikesh Kumar 5 years, 7 months ago
- 2 answers
Posted by Pragya Thakur 5 years, 7 months ago
- 2 answers
Reshma Ramesh 5 years, 7 months ago
Posted by Mohammed Rasith 5 years, 7 months ago
- 1 answers
Reshma Ramesh 5 years, 7 months ago
Posted by Deepak Kumar 5 years, 7 months ago
- 4 answers
Raj Kumar 5 years, 7 months ago
Posted by Ankit Gupta 5 years, 7 months ago
- 6 answers
Posted by Ayush Raj 5 years, 7 months ago
- 1 answers
Desheeba Nehal Odakkal 5 years, 7 months ago
Posted by Adarsh Agarwal 5 years, 7 months ago
- 1 answers
Posted by Aman Deep 5 years, 7 months ago
- 1 answers
Gaurav Seth 5 years, 7 months ago

or
Given,
Side a=18cm
Side b=10cm
Perimeter=42cm=a+b+c
:. Putting value
42=18+10+c
42=28+c
42-28=c
14=c
Now,
S=(a+b+c)/2
:. Putting value
S=42/2
S=21
Now according to Heron's formula-
Area of a triangle
=√{s(s-a)(s-b)(s-c)}
:. Putting value
=√{21(21-18)(21-10)(21-14)}
=√{21(3)(11)(7)}
=√4851
=21√11cm²
Posted by Aman Deep 5 years, 7 months ago
- 0 answers
Posted by Diya Lalwani 5 years, 7 months ago
- 1 answers
Sia ? 5 years, 7 months ago
Given: PQ = PR

To prove: PS > PQ
Proof: In △PRQ, we have
PR = PQ [Given]
⇒∠1=∠R
[∴ Angles opposite to the equal side of the triangle are equal]
But, ∠1>∠S [∴ Exterior angle of a triangle is greater than each of the remote interior angles]
⇒∠R>∠S [∵∠1=∠R]
⇒ PS < PR [∵ In a triangle, side opposite to the large is longer]
Hence, proved.
Posted by Anuj Kumar 5 years, 7 months ago
- 1 answers
Posted by Abigail Miruse 5 years, 7 months ago
- 0 answers
Posted by Bushra Nazer 5 years, 7 months ago
- 0 answers
Posted by Sakthi Nayagam 5 years, 7 months ago
- 0 answers
Posted by Harsimran Singh 5 years, 7 months ago
- 2 answers
Posted by Tejas Lamba 5 years, 7 months ago
- 4 answers
Shreya Raj 5 years, 7 months ago
Posted by Akhil Rana 5 years, 7 months ago
- 5 answers
Posted by Hitaishi Lilhare 5 years, 7 months ago
- 1 answers
Muskan Chauhan 5 years, 7 months ago
Posted by Samarth Gupta 5 years, 7 months ago
- 0 answers
Posted by Arjun Singh 5 years, 7 months ago
- 3 answers
Posted by Furqan Azam 5 years, 7 months ago
- 1 answers
Gaurav Seth 5 years, 7 months ago
Question: If f(x) = x4 - 2x3 + 3x2 - ax + b is divided by(x - 1) and (x + 1), it leaves the remainder 5 and 19 respectively. Find 'a' and 'b'.
Answer:
Given f(x) = x4 - 2x3 + 3x2 - ax + b
When f(x) is divided by (x-1), it leaves a remainder 5
 f(1) = 5
f(1) = 5
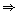 1 - 2(1)3 + 3(1)2 - a(1) + b = 5
1 - 2(1)3 + 3(1)2 - a(1) + b = 5
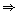 1 - 2 + 3 - a +b = 5
1 - 2 + 3 - a +b = 5
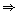 -a + b = 3 … (i)
-a + b = 3 … (i)
When f(x) is divided by (x+1), it leaves a remainder 19
 f(-1) = 19
f(-1) = 19
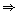 (-1)4 - 2(-1)3 + 3(-1)2 - a(-1) + b = 19
(-1)4 - 2(-1)3 + 3(-1)2 - a(-1) + b = 19
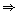 1 + 2 + 3 + a + b = 19
1 + 2 + 3 + a + b = 19
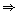 a +b = 13 … (ii)
a +b = 13 … (ii)
Adding (i) and (ii),
2b = 16 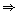 b = 8
b = 8
(i) 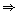 a = b - 3 = 8 - 3 = 5
a = b - 3 = 8 - 3 = 5
Posted by Anushka Mahanty 5 years, 7 months ago
- 5 answers
S.Bhavitha S.Bhavitha 5 years, 7 months ago
Badal Sahu 5 years, 7 months ago
Shini Byju 5 years, 7 months ago
Posted by Suman Kumar 5 years, 7 months ago
- 2 answers
Posted by Anshu Kumar 5 years, 7 months ago
- 1 answers
Posted by Fathima Shaniya 5 years, 7 months ago
- 2 answers
Ravinder Singh 5 years, 7 months ago
Posted by Jaismeen Kaur 5 years, 7 months ago
- 3 answers

myCBSEguide
Trusted by 1 Crore+ Students

Test Generator
Create papers online. It's FREE.

CUET Mock Tests
75,000+ questions to practice only on myCBSEguide app
 myCBSEguide
myCBSEguide
Mr Funny India Creation 5 years, 7 months ago
0Thank You