CBSE > Class 09 > Mathematics | CBSE Board | Homework Help

We have, $x^{2}+\frac{1}{x^{2}}=34$
Also,
$\Rightarrow\left(x+\frac{1}{x}\right)^{2}=x^{2}+\frac{1}{x^{2}}+2 x^{2} \times \frac{1}{x^{2}}$
$\Rightarrow \left(x+\frac{1}{x}\right)^{2}=34+2$
$\Rightarrow \ n+\frac{1}{n}=\sqrt{36}=6$
Now,
$\Rightarrow \left(x+\frac{1}{x}\right)^{3}=x^{3}+\frac{1}{x^{3}}+3 x_{\times} \frac{1}{x}\left(x+\frac{1}{x}\right)$
$\Rightarrow 6^{3}=x^{3}+\frac{1}{x^{3}}+3 \times 6$
$\Rightarrow \quad x^{3}+\frac{1}{x^{3}}$ = 216 - 18
$x^{3}+\frac{1}{x^{3}}-9$ = 216 - 18 - 9
= 216 - 27
=189

0Thank You

ANSWER

Ifx

Posted by Aman Kumar 5 years, 7 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

In the given figure , PQ parallel , angle PAB =70°and angle ACS =100°. Find the measure of angle BAC and angle CAQ

Posted by Sandy Devi 5 years, 7 months ago

CBSE > Class 09 > Mathematics

1 answers

Badal Sahu 5 years, 6 months ago

Where is fig.

0Thank You

ANSWER

ABCD is a quadrilateral in which AC & BD are two diagonals .Show that AB+BC+CD+AD>AC+BD

Posted by Shivraj Patil 5 years, 7 months ago

CBSE > Class 09 > Mathematics

1 answers

Sia ? 5 years, 7 months ago

Given: A quadrilateral ABCD.
To prove: AB + BC + CD + DA > AC + BD
Proof: In $\Delta ABC$ , we have

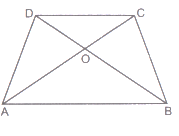
AB + BC > AC…(1) [ $\because$ Sum of the lengths of any two sides of a triangle must be greater than the third side]
In $\Delta BCD$ , we have
BC + CD > BD...(2) [Same reason]
In $\Delta CDA$ , we have
CD + DA > AC…(3) [Same reason]
In $\Delta DAB$ , we have
AD + AB > BD…(4) [Same reason]
Adding (1), (2), (3) and (4), we get
AB + BC + BC + CD + CD + DA + AD + AB > AC + BD + AC + BD
$\Rightarrow$ 2AB + 2BC + 2CD + 2DA > 2AC + 2BD
$\Rightarrow$ 2(AB + BC + CD + DA) > 2(AC + BD)
$\Rightarrow$ AB + BC + CD + DA > AC + BD
Hence, proved.

0Thank You

ANSWER

X minus root under 2 is a factor of 7 x square - 4 root under 2x-6

Posted by Chandeshwar Prasad 5 years, 7 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

2(x)2+2

Posted by Raghvendra Singh Singh 5 years, 7 months ago

CBSE > Class 09 > Mathematics

2 answers

Ayushi Maheshwari 5 years, 6 months ago

4x+2

0Thank You

Aditiya Super 30 5 years, 7 months ago

4x+2=0. 4x=-2. X=-1/2

0Thank You

ANSWER

(256)^0.16×(256)^0.09

Posted by Shubham Thite 5 years, 7 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

In triangle abc if angle A =60 and angle B=50 then which side of triangle the longest and the shortest

Posted by All Categories Video By Gaurav Grs 5 years, 7 months ago

CBSE > Class 09 > Mathematics

3 answers

Chirag Sharma 5 years, 7 months ago

Longest side - AB Shortest side - AC

1Thank You

Anjali Arya 5 years, 7 months ago

AB is longest &AC is shortest

0Thank You

Manav M? 5 years, 7 months ago

AB is the longest side and AC is the shortest

2Thank You

ANSWER

How many type of line

Posted by Sachin Gupta 5 years, 7 months ago

CBSE > Class 09 > Mathematics

1 answers

Manish Kumar 5 years, 7 months ago

Three types of line Line Line segment Ray

0Thank You

ANSWER

How many type if line

Posted by Sachin Gupta 5 years, 7 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Find the value of a and b ^7+^6/^7-^6 = a + b ^42

Posted by Ananya Paul 5 years, 7 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

The taxi fare in the City is as follows for the first kilometre the fare is rupees 8 and for the the subsequent distance it is rupees 5 per kilometre taking the distance covered as x kilometre and Total fare as rupees 5 light a linear equation for the information and draw its graph

Posted by Deepak Singh 5 years, 7 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Solve into fractions 4 x square minus 9 y square - 2 x minus 3 y

Posted by Ishika Roy 5 years, 7 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

The perimeter of triangular field is 240 dm if two of it sides are 78 dm and 50 dm ,find the length of perpendicular on side of length 50 dm from the opposite vertex

Posted by Bhargavi Vala 5 years, 7 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

IN a triangle ABC , AB=15 cm ,BC=13 cm,and Ac=14 cm. Find the area of triangle ABC and Hence its altitude on Ac.

Posted by Bhargavi Vala 5 years, 7 months ago

CBSE > Class 09 > Mathematics

3 answers

Gaurav Seth 5 years, 7 months ago

Question: In a ∆ABC, AB = 15 cm, BC = 13 cm and AC = 14 cm. Find the area of ∆ABC and hence its altitude on AC.

Answer

The triangle sides are

Let a = AB = 15 cm, BC = 13 cm = b, c = AC = 14 cm say

Now,

1Thank You

Ritu Devi 5 years, 7 months ago

1/2*13*15= 97.5

0Thank You

Ritu Devi 5 years, 7 months ago

15+13+14

1Thank You

ANSWER

The record of a weather station shows that out of the past 150 consecutive days its weather forecast were correct 90 times a) what is the probability that on a given day it was correct. b) what is the probability that it was not correct on a given day

Posted by Sushma Yadav 5 years, 7 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Write the solutions of equation of two line passing through (3,-5)

Posted by Pooja Khokhar 5 years, 7 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Express y in terms of x. 3y+5x=9

Posted by Pooja Khokhar 5 years, 7 months ago

CBSE > Class 09 > Mathematics

1 answers

Shubham Garg 5 years, 6 months ago

y = 9 - 5x / 3

0Thank You

ANSWER

Express x in the term of y for the equation 3x+4y=7

Posted by Pooja Khokhar 5 years, 7 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

0.123333...so on is equal to 122/990 how

Posted by Omaan 10119931 5 years, 7 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Find arational no. Which is not between √3 And √4

Posted by Davinder Kumar 5 years, 7 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

All the equations in liner equation with two variables

Posted by Sreeraman Mahima 5 years, 7 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

How locate √26 in number line

Posted by Ankit Kumar 5 years, 7 months ago

CBSE > Class 09 > Mathematics

2 answers

Brijesh Kumar 5 years, 7 months ago

a+b+c ka holiskkyar

0Thank You

Purvi Shah 5 years, 7 months ago

Draw a 26cm line on a page and and draw 1 cm more and then draw the perpendicular biscetor of it.After drawing the bisector u will get a mid pont name it B and keep the rounder at point B and draw a semi circle where the semi circle ends name it point C and from point C draw a line attaching to semi circle and from the the length of attachment dram the cuve line with the help of rounder

3Thank You

ANSWER

2x+9=0

Posted by Piyush Thakur 5 years, 7 months ago

CBSE > Class 09 > Mathematics