The angle of elevation of the …

CBSE, JEE, NEET, CUET

Question Bank, Mock Tests, Exam Papers

NCERT Solutions, Sample Papers, Notes, Videos

The angle of elevation of the top of a tower 30 m high from the foot of another tower in the same plane is 60°. The angle of elevation of the top of second tower from the foot of the first tower is 30°. Find the distance between two towers and the height of the other tower. Also, find the length of the wire attached to the tops of both the towers.

Posted by Utkarsh Kumar Yadav 2 years ago

CBSE > Class 10 > Mathematics

5 answers

Preeti Dabral 2 years ago

Let distance between the two towers = AB = x m
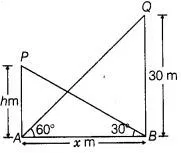
and height of the other tower = PA = h m
Given that, height of the tower = QB = 30 m and $\angle$ QAB = 60°, $\angle$ PBA = 30°.

Now, in $\Delta Q A B, \tan 60^{\circ}=\frac{Q B}{A B}=\frac{30}{x}$
$\Rightarrow \sqrt{3}=\frac{30}{x}$
$x=\frac{x^{2}}{3}-\frac{x}{3}-\frac{x-3}{3}-x+0.5 \pi$
and in $\Delta P B A$
$\tan 30^{\circ}=\frac{P A}{A B}=\frac{h}{x}$
$\Rightarrow \frac{1}{\sqrt{3}}=\frac{h}{10 \sqrt{3}}$ $[\because x=10 \sqrt{3} \mathrm{m}]$
$\Rightarrow h = 10 m$
Hence, the requied distance and height are $10\sqrt3$ and 10m, respectively.

0Thank You

Ankit Class 2 years ago

Ye figure draw kaise ki ho batao

0Thank You

Ankit Class 2 years ago

PQ is the length of the wire attached to the tops of both the towers

0Thank You

Ankit Class 2 years ago

PQ=√x^2 +(30-h)^2 . PQ=√(10√3)^2 + (30-10)^2 . PQ=√ 300+400. PQ=√700. PQ= 10√7 m

0Thank You

Ankit Class 2 years ago

Math standard mein ye question puchha tha n mere mein bhi puchha tha set 1 tha mera 21 feb ko paper tha n

0Thank You

ANSWER

Related Questions

Is it possible to design a rectangular mango Grove whose length is twice it's breadth, and the area is 800m²? If so, find it's length and breadth

Posted by Akshata Choukse 8 months, 2 weeks ago

CBSE > Class 10 > Mathematics

0 answers

(A + B )²

Posted by Abhishek Gupta 8 months, 2 weeks ago

CBSE > Class 10 > Mathematics

1 answers

X-y=5

Posted by Kishan Thakur 6 months, 1 week ago

CBSE > Class 10 > Mathematics

1 answers

Find the sum of the odd no. Between 0 and 50

Posted by Kanika . 6 months, 2 weeks ago

CBSE > Class 10 > Mathematics

1 answers

If 1/2 is a root of equation x^2+kx- 5/4 =0 , then what is the value of k

Posted by Parinith Gowda Ms 8 months, 2 weeks ago

CBSE > Class 10 > Mathematics

1 answers

Find the nature of quadratic equation x^2 +x -5 =0

Posted by Parinith Gowda Ms 8 months, 2 weeks ago

CBSE > Class 10 > Mathematics

0 answers

sin60° cos 30°+ cos60° sin 30°

Posted by Vanshika Bhatnagar 8 months, 2 weeks ago

CBSE > Class 10 > Mathematics

2 answers

Find cosec 30 degree and cause 60 degree geometrical

Posted by Hari Anand 5 months ago

CBSE > Class 10 > Mathematics

0 answers

Venu Gopal has twice

Posted by Lakshay Kumar 4 months, 3 weeks ago

CBSE > Class 10 > Mathematics

0 answers

This content has been hidden. One or more users have flagged this content as inappropriate. Once content is flagged, it is hidden from users and is reviewed by myCBSEguide team against our Community Guidelines. If content is found in violation, the user posting this content will be banned for 30 days from using Homework help section. Suspended users will receive error while adding question or answer. Question comments have also been disabled. Read community guidelines at https://mycbseguide.com/community-guidelines.html

Few rules to keep homework help section safe, clean and informative.

Don't post personal information, mobile numbers and other details.
Don't use this platform for chatting, social networking and making friends. This platform is meant only for asking subject specific and study related questions.
Be nice and polite and avoid rude and abusive language. Avoid inappropriate language and attention, vulgar terms and anything sexually suggestive. Avoid harassment and bullying.
Ask specific question which are clear and concise.

Remember the goal of this website is to share knowledge and learn from each other. Ask questions and help others by answering questions.

Posted by S Prajwal 2 months, 3 weeks ago

CBSE > Class 10 > Mathematics