Two chord ab and cd are …

Let AB and CD be two parallel chords of a circle with centre O such that AB = 6 cm and CD = 12 cm. Let the radius of the circle be r cm. Draw OP {tex}\perp{/tex} AB and OQ {tex}\perp{/tex} CD. Since AB || CD and OP {tex}\perp{/tex} AB, OQ {tex}\perp{/tex} CD. Therefore, points O, Q, and P are collinear.

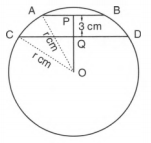
Clearly, PQ = 3 cm
Let OQ = x cm. Then, OP = (x + 3) cm
Applying Pythagoras theorem in right triangles OAP and OCQ, we obtain
OA² = OP² + AP² and OC² = OQ² + CQ² 
{tex}\Rightarrow{/tex} r² = (x + 3)² + 3² and r² = x² + 6² [{tex}\because{/tex} AP = {tex}\frac{1}{2}{/tex} AB = 3 cm and CQ = {tex}\frac{1}{2}{/tex}CD = 6 cm]
{tex}\Rightarrow{/tex} (x + 3)² + 3² = x² + 6² [On equating the values of r²]
{tex}\Rightarrow{/tex}x² + 6x + 9 + 9 = x² + 36 {tex}\Rightarrow{/tex} 6x = 18 {tex}\Rightarrow{/tex} x = 3
Putting the value of x in r² = x² + 6², we get
r² = 3² + 6² = 45 {tex}\Rightarrow{/tex} r = {tex}\sqrt{45}{/tex} cm = 6.7 cm
Hence, the radius of the circle is 6.7 cm.