The formula of moment inertia in terms of the radius of gyration is given as follows:
I = mk2 (1)
where I is the moment of inertia and m is the mass of the body
Accordingly, the radius of gyration is given as follows
k= √ l / m (2)
The unit of the radius of gyration is mm. By knowing the radius of gyration, one can find the moment of inertia of any complex body equation (1) without any hassle.
Consider a body having n number of particles each having a mass of m. Let the perpendicular distance from the axis of rotation be given by r1, r2, r3,…,rn. We know that the moment of inertia in terms of radius of gyration is given by the equation (1). Substituting the values in the equation, we get the <a href="https://byjus.com/jee/moment-of-inertia/">moment of inertia</a> of the body as follows
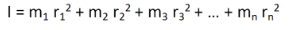
………… (3)
If all the particles have the same mass then equation (3) becomes
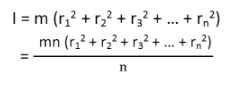
We can write mn as M which signifies the total mass of the body. Now the equation becomes
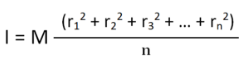
………… (4)
From equation (4), we get
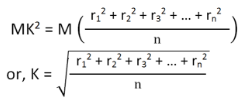
From the above equation, we can infer that the radius of gyration can also be defined as the root-mean-square distance of various particles of the body from the axis of rotation.




 myCBSEguide
myCBSEguide

Gaurav Seth 4 years, 9 months ago
The formula of moment inertia in terms of the radius of gyration is given as follows:
I = mk2 (1)
where I is the moment of inertia and m is the mass of the body
Accordingly, the radius of gyration is given as follows
k= √ l / m (2)
The unit of the radius of gyration is mm. By knowing the radius of gyration, one can find the moment of inertia of any complex body equation (1) without any hassle.
Consider a body having n number of particles each having a mass of m. Let the perpendicular distance from the axis of rotation be given by r1, r2, r3,…,rn. We know that the moment of inertia in terms of radius of gyration is given by the equation (1). Substituting the values in the equation, we get the <a href="https://byjus.com/jee/moment-of-inertia/">moment of inertia</a> of the body as follows
………… (3)
If all the particles have the same mass then equation (3) becomes
We can write mn as M which signifies the total mass of the body. Now the equation becomes
………… (4)
From equation (4), we get
From the above equation, we can infer that the radius of gyration can also be defined as the root-mean-square distance of various particles of the body from the axis of rotation.
2Thank You