ABCD is a quadrilateral in which …

CBSE, JEE, NEET, CUET

Question Bank, Mock Tests, Exam Papers

NCERT Solutions, Sample Papers, Notes, Videos

Install Now

ABCD is a quadrilateral in which AC & BD are two diagonals .Show that AB+BC+CD+AD>AC+BD

Posted by Shivraj Patil 5 years, 7 months ago

CBSE > Class 09 > Mathematics

1 answers

Sia ? 5 years, 7 months ago

Given: A quadrilateral ABCD.
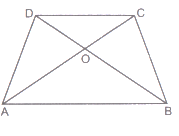
To prove: AB + BC + CD + DA > AC + BD
Proof: In $\Delta ABC$ , we have

AB + BC > AC…(1) [ $\because$ Sum of the lengths of any two sides of a triangle must be greater than the third side]
In $\Delta BCD$ , we have
BC + CD > BD...(2) [Same reason]
In $\Delta CDA$ , we have
CD + DA > AC…(3) [Same reason]
In $\Delta DAB$ , we have
AD + AB > BD…(4) [Same reason]
Adding (1), (2), (3) and (4), we get
AB + BC + BC + CD + CD + DA + AD + AB > AC + BD + AC + BD
$\Rightarrow$ 2AB + 2BC + 2CD + 2DA > 2AC + 2BD
$\Rightarrow$ 2(AB + BC + CD + DA) > 2(AC + BD)
$\Rightarrow$ AB + BC + CD + DA > AC + BD
Hence, proved.

0Thank You

ANSWER

Related Questions

3√2×4√2×12√32

Posted by Manas Khurana 8 months, 4 weeks ago

CBSE > Class 09 > Mathematics

0 answers

2x+5y

Posted by Yash Pandey 1 month, 2 weeks ago

CBSE > Class 09 > Mathematics

0 answers

Cost of a pen is two and half times the cost of a pencil. Express the situation as a linear equation in two variables

Posted by Kirshika Ganesh 2 months ago

CBSE > Class 09 > Mathematics

0 answers

Ch-1 introduction

Posted by Sheikh Alfaz 3 months, 1 week ago

CBSE > Class 09 > Mathematics

0 answers

X³-12x²+39x-28

Posted by Sukhi Devasi 8 months, 4 weeks ago

CBSE > Class 09 > Mathematics

0 answers

This content has been hidden. One or more users have flagged this content as inappropriate. Once content is flagged, it is hidden from users and is reviewed by myCBSEguide team against our Community Guidelines. If content is found in violation, the user posting this content will be banned for 30 days from using Homework help section. Suspended users will receive error while adding question or answer. Question comments have also been disabled. Read community guidelines at https://mycbseguide.com/community-guidelines.html

Few rules to keep homework help section safe, clean and informative.

Don't post personal information, mobile numbers and other details.
Don't use this platform for chatting, social networking and making friends. This platform is meant only for asking subject specific and study related questions.
Be nice and polite and avoid rude and abusive language. Avoid inappropriate language and attention, vulgar terms and anything sexually suggestive. Avoid harassment and bullying.
Ask specific question which are clear and concise.

Remember the goal of this website is to share knowledge and learn from each other. Ask questions and help others by answering questions.

Posted by T Prudhvi 6 months ago

CBSE > Class 09 > Mathematics