polynomial x4+7x3+7x2+px+q is exactly divisible by …

Factors of x² + 7x + 12 :
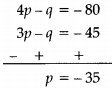
x² + 7x + 12 = 0
$\Rightarrow$ x² + 4x + 3x + 12 = 0
$​​\Rightarrow$ x(x + 4) + 3(x + 4) = 0
$​​\Rightarrow$(x + 4) (x + 3) = 0
$​​\Rightarrow$ x = - 4, -3 ...(i)
Since p(x) = x⁴ + 7x³ + 7x² + px + q
If p(x) is exactly divisible by x²+ 7x + 12, then x = - 4 and x = - 3 are its zeroes. So putting x = - 4 and x = - 3.
p(- 4) = (- 4)⁴ + 7(- 4)³ + 7(- 4)² + p(- 4) + q
but p(- 4) = 0
$\therefore$ 0 = 256 - 448 + 112 - 4p + q
0 = - 4p + q - 80
$\Rightarrow$4p - q = - 80 ...(i)
and p(-3) = (-3)⁴ + 7(-3)³ + 7(-3)² + p(-3) + q
but p(-3) = 0
$\Rightarrow$0 = 81-189 + 63 - 3p + q
$\Rightarrow$0 = -3p + q -45
$\Rightarrow$3p -q = -45 ..........(ii)

On putting the value of p in eq. (i),we get,
$4(-35) - q = - 80$
$\Rightarrow$$-140 - q = - 80$
$\Rightarrow\ $$-q = 140 - 80$
$\Rightarrow\ $ $-q = 60$
$\therefore$ $q = -60$
Hence, p = -35, q = -60