Relations and Functions Extra Questions for Class 12 Mathematics. myCBSEguide has just released Chapter Wise Question Answers for class 12 Maths. There chapter wise Practice Questions with complete solutions are available for download in myCBSEguide website and mobile app. These Questions with solution are prepared by our team of expert teachers who are teaching grade in CBSE schools for years. There are around 4-5 set of solved Chapter 1 Relations and Functions Mathematics Extra Questions from each and every chapter. The students will not miss any concept in these Chapter wise question that are specially designed to tackle Board Exam. We have taken care of every single concept given in CBSE Class 12 Mathematics syllabus and questions are framed as per the latest marking scheme and blue print issued by CBSE for class 12.
Class 12 Chapter 1 Maths Extra Questions
Relations and Functions Chapter 1 Important Questions
Chapter 1 Relations and Functions
-
In Z , the set of integers, inverse of – 7 , w.r.t. ‘ * ‘ defined by a * b = a + b + 7 for all {tex}a,b \in Z{/tex} ,is
- -7
- -14
- 14
- 7
-
If A = { 1, 2, 3}, then the relation R = {(1, 2), (2, 3), (1, 3)} in A is ___.
- transitive only
- reflexive only
- symmetric only
- symmetric and transitive only
-
A relation R on a set A is called an empty relation if
- no element of A is related to any element of A
- every element of A is related to one element of A
- one element of A is related to all the elements of A
- every element of A is related to any element of A
-
Let f and g be two functions from R to R defined as {tex}f(x) = \left\{ {\begin{array}{*{20}{c}} {0,x\;is\;rational} \\ {1,x\;is\;irrational} \end{array}} \right\}{/tex}, {tex}g(x) = \left\{ {\begin{array}{*{20}{c}} { – 1,x\;is\;rational} \\ {0,x\;is\;irrational} \end{array}} \right\}{/tex} then, (gof)(e) + (fog) {tex}(\pi ){/tex} =.
- 1
- 2
- -1
- 0
-
Let R be the relation on N defined as xRy if x + 2 y = 8. The domain of R is
- {2, 4, 6, 8}
- {2, 4, 8}
- {1, 2, 3, 4}
- {2, 4, 6}
- If n(A) = p and n(B) = q, then the number of relations from set A to set B = ________.
- A function is called an onto function, if its range is equal to ________.
- A binary operation * on a set X is said to be ________, if a * b = b * a, where a, b {tex}\in{/tex} X.
-
Find gof f(x) = |x|, g(x) = |5x + 1|.
-
Show that function f: N {tex}\rightarrow{/tex} N, given by f(x) = 2x, is one – one.
-
Let S = {1, 2, 3} Determine whether the function f: S {tex}\rightarrow{/tex} S defined as below have inverse.
f = {(1, 1), (2, 2), (3, 3)} -
Let f : {1, 3, 4} {tex}\rightarrow{/tex} {1, 2, 5} and g : {1, 2, 5} {tex}\rightarrow{/tex} {1, 3} be given by f = {(1, 2), (3, 5), (4, 1)} and g = {(1, 3), (2, 3), (5, 1)}. Write down gof.
-
Consider f: {1, 2, 3} {tex}\rightarrow{/tex} {a, b, c} given by f(1) = a, f(2) = b and f(3) = c find f-1 and show that (f-1)-1 = f.
-
Is g = {(1, 1), (2, 3), (3, 5), (4, 7)} a function? If g is described by g(x) = ax + b, then what value should be assigned to a and b.
-
Show that the relation R defined by (a, b) R (c, d) {tex}\Rightarrow{/tex} a + d = b + c on the set N{tex}\times{/tex}N is an equivalence relation.
-
Let the function f : R {tex}\rightarrow{/tex} R be defined by f(x) = cosx, {tex}\forall{/tex}x {tex}\in{/tex} R. Show that f is neither one-one nor onto.
-
Let L be the set of all lines in plane and R be the relation in L define if R = {(l1, L2 ): L1 is{tex} \bot {/tex} to L2 } .Show that R is symmetric but neither reflexive nor transitive.
-
If the function f : R {tex}\to{/tex} R is given by f (x) = x2 + 2 and g: R {tex}\to{/tex} R is given by {tex}g ( x ) = \frac { x } { x – 1 } ; x \neq 1{/tex} then find fog and gof and hence find fog(2) and gof(- 3).
Chapter 1 Relations and Functions
Solution
-
- -7
Explanation: If ‘ e ‘ is the identity ,then a*e = a {tex} \Rightarrow {/tex}a + e + 7 = a {tex} \Rightarrow {/tex} e = – 7 . Also,inverse of e is e itself. Hence , inverse of -7 is -7.
- transitive only
Explanation: A relation R on a non-empty set A is said to be transitive if xRy and y Rz {tex} \Rightarrow {/tex} xRz, for all x {tex} \in {/tex} R. Here, (1, 2) and (2, 3) belongs to R implies that (1, 3) belongs to R.
- no element of A is related to any element of A
Explanation: For any set A ,an empty relation may be defined on A as: there is no element exists in the relation set which satisfies the relation for a given set A i.e.
let A={1,2,3,4,5} and R={(a,b): a,b {tex} \in {/tex} A and a+b= 10},so we get R={ } which is an empty relation.
- -1
Explanation: (gof)(e) + (fog)({tex}\pi {/tex}) =g(f(e)) + f(g({tex}\pi {/tex}) = g(1) + f(0) = – 1 + 0 = -1.
- {2, 4, 6}
Explanation: As xRy if x + 2 y = 8 , therefore, domain of the relation R is given by x = 8 – 2y ∈ N. When y = 1, {tex} \Rightarrow {/tex} x = 6, when y = 2, {tex} \Rightarrow {/tex} x =4 , when y =3 , {tex} \Rightarrow {/tex} x = 2. Therefore, domain is { 2, 4, 6 }.
- -7
- 2pq
- codomain
- commutative
- gof (x) = g [f(x)] = g [|x|] =| 5 |x| + 1 |
- For, f(x1) = f(x2)
2x1 = 2x2
x1 = x2
So, The function f is one – one - Since different elements have different images. So, f is one – one. Also, every element of codomain has pre-image so, f is onto
Now f is one – one and onto, so that f is invertible with inverse f-1 = {(1, 1) (2, 2) (3, 3)} - f = {(1, 2), (3, 5), (4, 1)} and g = {(1, 3), (2, 3), (5, 1)}
Now, f(1) = 2, f(3) = 5, f(4) = 1 and g(1) = 3, g(2) = 3, g(5) =1
gof(x)=g(f(x))
gof(1)=g(f(1))=g(2)=3
g[f(3)] = g(5) = 1 and g[f(4)] = g(1) = 3
Hence, gof = {(1, 3), (3, 1), (4, 3)} - f = {(1, a) (2, b) (3, c)}
f-1 = { (a, 1) (b, 2) (c, 3)}
(f -1) -1 = {(1, a) (2, b) (3, c)}
Hence (f-1)-1 = f. - Yes, g is a function since every element in domain has a unique image in range.
Now, Let g(x) = ax + b Then Given,
g(1) = a + b = 1 &
g(2) = 2a +b = 3
Subtracting g(1) from g(2) Gives
(2a + b) – (a + b) = a = 2 & Substituting It into g(1)
We have b = -1 - we have,a + b = b + a for all (a, b) {tex}\in N×N{/tex}, which implies (a,b) R (a,b).Thus, R is reflexive.
Let (a,b),(c,d) {tex}\in N×N{/tex} be such that
(a, b) R (c, d)
{tex}\Rightarrow{/tex} a + d= b + c
{tex}\Rightarrow{/tex} d + a = c + b
{tex}\Rightarrow{/tex} c + b = d + a
{tex}\Rightarrow{/tex} (c, d) R (a, b) for all (a, b), (c, d) {tex}\in N×N{/tex}
Hence R is symmetric.
Let (a,b),(c,d),(e,f) {tex}\in N×N{/tex} such that (a,b) R (c,d) and
(c,d) R (e,f).Then,
(a, b) R (c, d) {tex}\Rightarrow{/tex} a + d = b + c……. (1)
(c, d) R (e, f) {tex}\Rightarrow{/tex} c + f = d + e ………(2)
Adding (1) and (2)
(a + d) + (c+f) = (b + c) + (d + e)
a + f = b+ e
(a, b) R (e, f)
Hence, R is transitive
So, R is an equivalence relation. - Given function, f(x) = cosx, {tex}\forall{/tex}x {tex}\in{/tex} R
Now, {tex}f\left( {\frac{\pi }{2}} \right) = \cos \frac{\pi }{2} = 0{/tex}
{tex} \Rightarrow f\left( {\frac{{ – \pi }}{2}} \right) = \cos \frac{\pi }{2} = 0{/tex}
{tex}\Rightarrow f\left( {\frac{\pi }{2}} \right) = f\left( {\frac{{ – \pi }}{2}} \right){/tex}
But {tex}\frac{\pi }{2} \ne \frac{{ – \pi }}{2} = 0{/tex}
So, f (x) is not one-one
Now, f(x) = cosx, {tex}\forall{/tex}x {tex}\in{/tex} R is not onto as there is no pre-image for any real number. Which does not belong to the intervals [-1, 1], the range of cos x. - R is not reflexive, as a line L1 cannot be {tex} \bot {/tex} to itself i.e (L1, L1 ){tex}\notin{/tex} R
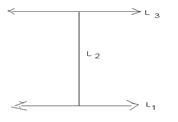
Now ( L1, L2){tex}\in{/tex}R
{tex}\Rightarrow{/tex}L1 {tex}\bot {/tex} L2
{tex}\Rightarrow{/tex}L2 {tex}\bot {/tex} L1
{tex}\Rightarrow{/tex}(L2, L1){tex} \in {/tex}R
R is symmetric
Now (L1, L2) and (L2, L3) {tex}\in{/tex} R
i.e L1 {tex}\bot{/tex} L2 and L2 {tex}\bot{/tex} L3
Then L1 can never be {tex}\bot {/tex} to L3 in fact L1 || L3
i.e (L1, L2) {tex}\in{/tex} R, (L2, L3) {tex} \in{/tex} R.
But (L1, L3) {tex}\notin{/tex} R
R is not transitive. - We are given that, f : R {tex}\to{/tex} R and g : R {tex}\to{/tex} R defined as f(x) = x2 + 2 and g(x) = {tex}\frac { x } { x – 1 } ; x \neq 1{/tex}.
First we see whether fog and gof exist for the given functions.
Since, range f {tex} \subseteq {/tex} domain g and range g {tex} \subseteq {/tex} domain f
Hence,fog and gof exist for the given functions.
Now, for any x {tex}\in{/tex} R – {1}, we have (fog)(x) = f[g(x)] = {tex}f \left[ \frac { x } { x – 1 } \right] = \left( \frac { x } { x – 1 } \right) ^ { 2 } + 2{/tex}
{tex}= \frac { x ^ { 2 } + 2 ( x – 1 ) ^ { 2 } } { ( x – 1 ) ^ { 2 } }{/tex}= {tex}\frac { x ^ { 2 } + 2 \left( x ^ { 2 } + 1 – 2 x \right) } { ( x – 1 ) ^ { 2 } }{/tex}
={tex}\frac { 3 x^2 + 2 – 4 x } { ( x – 1 ) ^ 2 }{/tex}
{tex}\therefore{/tex} fog: R {tex}\to{/tex} R is defined by
(fog)(x)= {tex}\frac { 3 x ^ { 2 } – 4 x + 2 } { ( x – 1 ) ^ { 2 } } , x \neq 1{/tex} ……(i)
For any x {tex}\in{/tex} R we have
gof(x) = g[f(x)] = g(x2 + 2) = {tex}\frac { x ^ { 2 } + 2 } { \left( x ^ { 2 } + 2 \right) – 1 } = \frac { x ^ { 2 } + 2 } { x ^ { 2 } + 1 }{/tex}
{tex}\therefore{/tex} gof: R {tex}\to{/tex} R is defined by
(gof)(x) = {tex}\frac { x ^ { 2 } + 2 } { x ^ { 2 } + 1 }{/tex} ……..(ii)
On putting x = 2 in Eq. (i), we get
fog(2) = {tex}\frac { 3 \times ( 2 ) ^ { 2 } – 4 ( 2 ) + 2 } { ( 2 – 1 ) ^ { 2 } }{/tex}= {tex}\frac { 3 \times 4 – 8 + 2 } { ( 1 ) ^ { 2 } }{/tex}
= 12 – 8 + 2 = 6
On putting x = – 3 in Eq. (ii), we get
gof(-3) = {tex}\frac { ( – 3 ) ^ { 2 } + 2 } { ( – 3 ) ^ { 2 } + 1 } = \frac { 9+2 } { 9+1 }=\frac{11}{10}{/tex}
Chapter Wise Important Questions Class 12 Maths Part I and Part II
- Relations and Functions
- Inverse Trigonometric Functions
- Matrices
- Determinants
- Continuity and Differentiability
- Application of Derivatives
- Integrals
- Application of Integrals
- Differential Equations
- Vector Algebra
- Three Dimensional Geometry
- Linear Programming
- Probability

Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
Learn8 App
Practice unlimited questions for Entrance tests & government job exams at ₹99 only
Install Now