
myCBSEguide App
Download the app to get CBSE Sample Papers 2024-25, NCERT Solutions (Revised), Most Important Questions, Previous Year Question Bank, Mock Tests, and Detailed Notes.
Install NowExercise 10.1
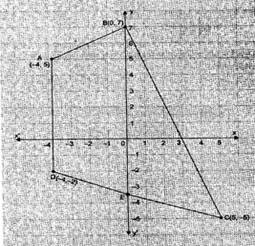
1. Draw a quadrilateral in the Cartesian plane, whose vertices are 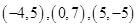 and
and 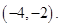 Also, find its area.
Also, find its area.
Ans. Area of quadrilateral ABCD = Area of 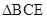 + Area of trapezium ABED
+ Area of trapezium ABED
= 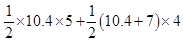
= 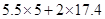
= 26 + 34.8 = 60.8 sq. units
2. The base of an equilateral triangle with side 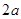 lies along the
lies along the  axis such that the mid-point of the base is at origin. Find the vertices of the triangle.
axis such that the mid-point of the base is at origin. Find the vertices of the triangle.
Ans. Given: Length of side of equilateral triangle = 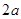 . The base of triangle lies along
. The base of triangle lies along  axis and the mid-point of base is at origin so that the coordinates of vertices are
axis and the mid-point of base is at origin so that the coordinates of vertices are 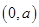 and
and 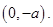
Let vertices of the third vertex be 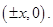

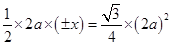

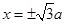
 The vertices of triangle are
The vertices of triangle are 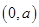 ,
, 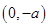 and
and 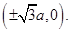
3. Find the distance between P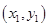 and Q
and Q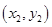 when (i) PQ is parallel to the
when (i) PQ is parallel to the  axis (ii) PQ is parallel to the
axis (ii) PQ is parallel to the  axis.
axis.
Ans. Given: P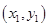 and Q
and Q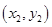 are two points.
are two points.
 PQ =
PQ = 
(i) PQ is parallel to  axis, then
axis, then 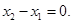
 PQ =
PQ = 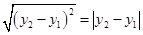
(ii) PQ is parallel to  axis, then
axis, then 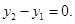
 PQ =
PQ = 
4. Find the point on the  axis, which is equidistant from the points (7, 6) and (3, 4).
axis, which is equidistant from the points (7, 6) and (3, 4).
Ans. Let P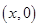 be any point on the
be any point on the  axis which is equidistant from Q (7, 6) and R (3, 4).
axis which is equidistant from Q (7, 6) and R (3, 4).
 PQ =
PQ = 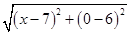
= 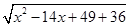
= 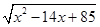
And PR = 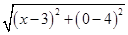
= 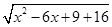
= 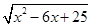
According to question, PQ = PR

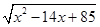
= 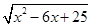
Squaring both sides, 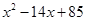 =
= 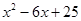

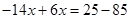

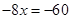


Therefore, the coordinates of the point are 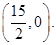
5. Find the slope of a line, which passes through the origin and the mid-point of the line segment of joining the points P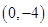 and B (8, 0).
and B (8, 0).
Ans. Here, mid-point of the line segment joining P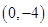 and Q (8, 0) is
and Q (8, 0) is
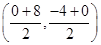

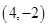
Since the line passes through points (0, 0) and 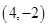 .
.
Therefore, Slope of the line = 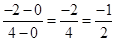
6. Without using the Pythagoras theorem, show that the points (4, 4), (3, 5) and 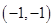 are the vertices of a right angled triangle.
are the vertices of a right angled triangle.
Ans. Let A (4, 4), B (3, 5) and C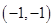 be three vertices of a
be three vertices of a 
 Slope of AB =
Slope of AB = 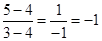
Slope of BC = 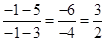
Slope of AC = 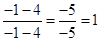
Now, Slope of AB x Slope of AC = 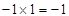
This shows that AB  AC. Thus
AC. Thus  is right angled at point A.
is right angled at point A.
7. Find the slope of the line, which makes an angle of 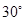 with the positive direction of
with the positive direction of  axis measured anticlockwise.
axis measured anticlockwise.
Ans. The line makes an angle of 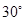 with the positive direction of
with the positive direction of  axis.
axis.
Now the line makes an angle of 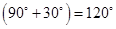 with the positive direction of
with the positive direction of  axis.
axis.
 Slope of the line =
Slope of the line = 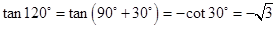
8. Find the value of 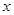 for which the points
for which the points 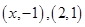 and
and 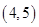 are collinear.
are collinear.
Ans. Let A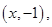 B (2, 1) and C (4, 5) be three collinear points.
B (2, 1) and C (4, 5) be three collinear points.
 Slope of AB =
Slope of AB = 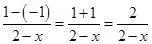
Slope of BC = 
According to question, Slope of AB = Slope of BC



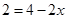

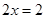

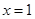
9. Without using distance formula, show that the points 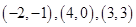 and
and 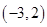 are the vertices of a parallelogram.
are the vertices of a parallelogram.
Ans. Let A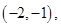 B (4, 0), C (3, 3) and D
B (4, 0), C (3, 3) and D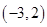 be vertices of a quadrilateral ABCD.
be vertices of a quadrilateral ABCD.
 Slope of AB =
Slope of AB = 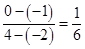 Slope of BC =
Slope of BC = 
Slope of DC = 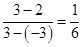 Slope of AD =
Slope of AD = 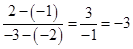
Here Slope of AB = Slope of DC
 AB
AB  DC
DC
And Slope of BC = Slope of AD
 BC
BC  AD
AD
Therefore, ABCD is a parallelogram.
10. Find the angle between the  axis and the line joining the points
axis and the line joining the points 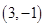 and
and 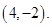
Ans. Let A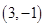 and B
and B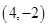 be two points. Let Q be the angle which AB makes with positive direction of
be two points. Let Q be the angle which AB makes with positive direction of  axis.
axis.
 Slope of AB =
Slope of AB = 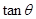
Also Slope of AB = 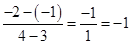

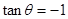

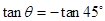

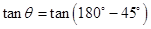

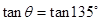

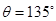
11. The slope of a line is double of the slope of the another line. If tangent of the angle between them is 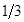 find the slopes of the lines.
find the slopes of the lines.
Ans. Given: 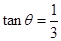 . Let the slopes of two lines be
. Let the slopes of two lines be  and
and 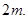

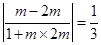

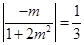

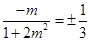
Taking 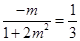

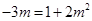

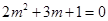

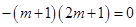

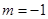 and
and 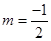
Taking 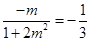

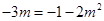

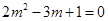

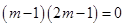

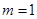 and
and 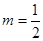
Therefore, the slopes of lines are 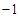 and
and 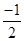 or 1 and
or 1 and 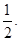
12. A line passes through 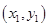 and
and  If slope of the line is
If slope of the line is 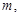 show that
show that 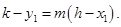
Ans. Let A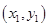 and B
and B be two points. It is given that Slope of AB =
be two points. It is given that Slope of AB = 
 Slope of AB =
Slope of AB = 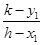 =
=  (given)
(given)


13. Is three points 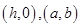 and
and 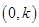 lies on a line, show that
lies on a line, show that 
Ans. Let A B
B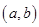 and C
and C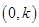 be three points lie on the line.
be three points lie on the line.
 Slope of AB =
Slope of AB = 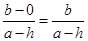 Slope of BC =
Slope of BC = 
Slope of AB = Slope of BC (given)





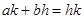

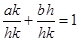

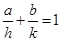
14. Consider the following population and year graph, find the slope of the line AB and using it, find what will be the population in the year 2010?
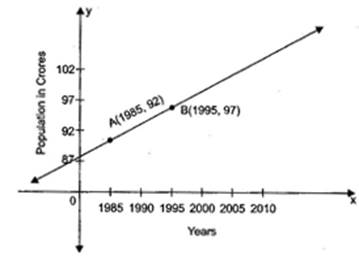
Ans. Given: The points on the line are A (1985, 92) and B (1995, 97).
 Slope of AB =
Slope of AB = 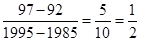
Let the population in year 2010 be 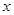 crores. Then C
crores. Then C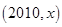 lies on the line.
lies on the line.
 Slope of BC =
Slope of BC = 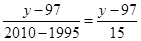
Since points A, B and C lie on the line.
 Slope of AB = Slope of BC
Slope of AB = Slope of BC



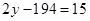

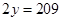


Therefore, population in 2010 will be 104.5 crores.

Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
myCBSEguide
Question Bank, Mock Tests, Exam Papers, NCERT Solutions, Sample Papers, Notes
Install Now
Nice Questions
Very clear answers .Each answers are in step by step
thank you so much for your help
Very nice questions Thank you so much for your help
thank you
Very nice solutions
Very good very nice
Very clear steps.
Very nice each solution of questions are step by step
thanks …it helps me a lot…?
Thanks one of the most helping
Thank you
Thank you so much very nice solution