NCERT Solutions class 12 Maths Exercise 5.1 Part-1 Class 12 Maths book solutions are available in PDF format for free download. These ncert book chapter wise questions and answers are very helpful for CBSE board exam. CBSE recommends NCERT books and most of the questions in CBSE exam are asked from NCERT text books. Class 12 Maths chapter wise NCERT solution for Maths part 1 and Maths part 2 for all the chapters can be downloaded from our website and myCBSEguide mobile app for free.
Download NCERT solutions for Continuity and Differentiability as PDF.

NCERT Solutions class 12 Continuity & Differentiability
1. Prove that the function 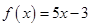 is continuous at
is continuous at  at
at 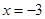 and at
and at 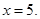
Ans. Given: 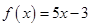
Continuity at 
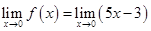 = 5 (0) – 3 = 0 – 3 = – 3
= 5 (0) – 3 = 0 – 3 = – 3
And  5 (0) – 3 = 0 – 3 = – 3
5 (0) – 3 = 0 – 3 = – 3
Since 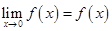 , therefore,
, therefore,  is continuous at
is continuous at 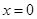 .
.
Continuity at 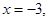
 = 5 (– 3) – 3 = – 15 – 3 = – 18
= 5 (– 3) – 3 = – 15 – 3 = – 18
And 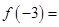 5 (– 3) – 3 = – 15 – 3 = – 18
5 (– 3) – 3 = – 15 – 3 = – 18
Since 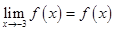 , therefore,
, therefore,  is continuous at
is continuous at 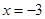
Continuity at 
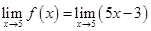 = 5 (5) – 3 = 25 – 3 = 2
= 5 (5) – 3 = 25 – 3 = 2
And 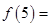 5 (5) – 3 = 25 – 3 = 22
5 (5) – 3 = 25 – 3 = 22
Since 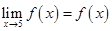 , therefore,
, therefore,  is continuous at
is continuous at 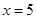 .
.
NCERT Solutions class 12 Maths Exercise 5.1 Part-1
2. Examine the continuity of the function 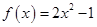 at
at 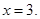
Ans. Given: 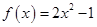
Continuity at 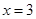 ,
, 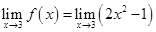 =
= 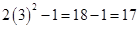
And 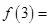
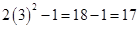
Since 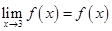 , therefore,
, therefore,  is continuous at
is continuous at 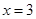 .
.
NCERT Solutions class 12 Maths Exercise 5.1 Part-1
3. Examine the following functions for continuity:
(a) 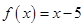
(b) 
(c) 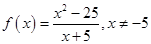
(d) 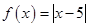
Ans. (a) Given: 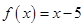
It is evident that  is defined at every real number
is defined at every real number 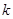 and its value at
and its value at 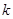 is
is 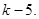
It is also observed that 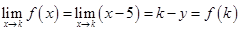
Since  , therefore,
, therefore,  is continuous at every real number and it is a continuous function.
is continuous at every real number and it is a continuous function.
(b) Given: 
For any real number 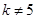 , we get
, we get 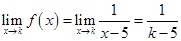
And 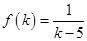
Since  , therefore,
, therefore,  is continuous at every point of domain of
is continuous at every point of domain of  and it is a continuous function.
and it is a continuous function.
(c) Given: 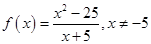
For any real number 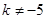 , we get
, we get
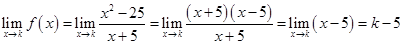
And 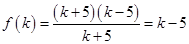
Since  , therefore,
, therefore,  is continuous at every point of domain of
is continuous at every point of domain of  and it is a continuous function.
and it is a continuous function.
(d) Given: 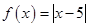
Domain of  is real and infinite for all real
is real and infinite for all real 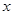
Here 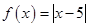 is a modulus function.
is a modulus function.
Since, every modulus function is continuous, therefore,  is continuous in its domain R.
is continuous in its domain R.
NCERT Solutions class 12 Maths Exercise 5.1 Part-1
4. Prove that the function 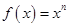 is continuous at
is continuous at 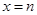 where
where 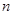 is a positive integer.
is a positive integer.
Ans. Given: 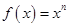 where
where 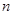 is a positive integer.
is a positive integer.
Continuity at 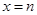 ,
, 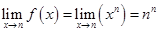
And 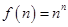
Since  , therefore,
, therefore,  is continuous at
is continuous at 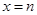 .
.
5. Is the function  defined by
defined by 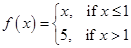 continuous at
continuous at  at
at 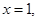 at
at 
Ans. Given: 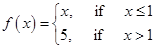
At 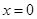 , It is evident that
, It is evident that  is defined at 0 and its value at 0 is 0.
is defined at 0 and its value at 0 is 0.
Then 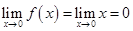 and
and 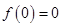
Therefore,  is continuous at
is continuous at 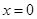 .
.
At 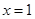 , Left Hand limit of
, Left Hand limit of 
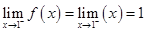
Right Hand limit of 
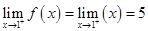
Here 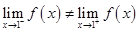
Therefore,  is not continuous at
is not continuous at 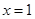 .
.
At 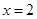 ,
,  is defined at 2 and its value at 2 is 5.
is defined at 2 and its value at 2 is 5.
 , therefore,
, therefore, 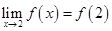
Therefore,  is not continuous at
is not continuous at 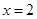 .
.
NCERT Solutions class 12 Maths Exercise 5.1 Part-1
Find all points of discontinuity of 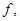 where
where  is defined by: (Exercise 6 to 12)
is defined by: (Exercise 6 to 12)
6. 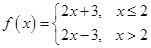
Ans. Given: 
Here  is defined for
is defined for 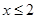 i.e., on
i.e., on  and also for
and also for 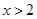 i.e., on
i.e., on 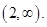
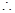 Domain of
Domain of  is
is 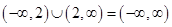 = R
= R
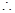 For all
For all 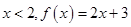 is a polynomial and hence continuous and for all
is a polynomial and hence continuous and for all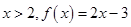 is a continuous and hence it is also continuous on R – {2}.
is a continuous and hence it is also continuous on R – {2}.
Now Left Hand limit =  = 2 x 2 + 3 = 4 + 3 = 7
= 2 x 2 + 3 = 4 + 3 = 7
Right Hand limit = 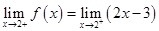 = 2 x 2 – 3 = 4 – 3 = 1
= 2 x 2 – 3 = 4 – 3 = 1
Since 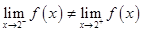
Therefore, 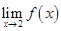 does not exist and hence
does not exist and hence  is discontinuous at
is discontinuous at 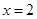 (only)
(only)
7. 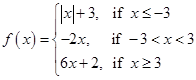
Ans. Given: 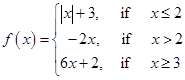
Here  is defined for
is defined for 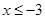 i.e., on
i.e., on 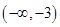 and for
and for 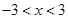 and also for
and also for 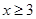 i.e., on
i.e., on 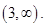
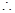 Domain of
Domain of  is
is  = R
= R
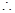 For all
For all  is a polynomial and hence continuous and for all
is a polynomial and hence continuous and for all 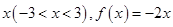 is a continuous and hence it is also continuous and also for all
is a continuous and hence it is also continuous and also for all 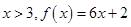 . Therefore,
. Therefore,  is continuous on R –
is continuous on R – 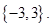
It is observed that 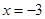 and
and 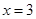 are partitioning points of domain R.
are partitioning points of domain R.
Now Left Hand limit = 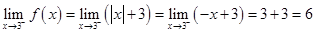
Right Hand limit = 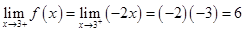
And 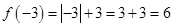
Therefore,  is continuous at
is continuous at 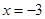 .
.
Again Left Hand limit = 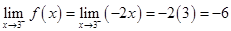
Right Hand limit = 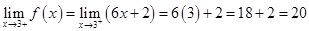
Since 
Therefore, 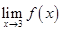 does not exist and hence
does not exist and hence  is discontinuous at
is discontinuous at 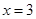 (only).
(only).
8. 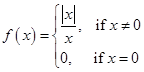
Ans. Given: 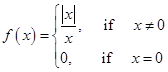 i.e.,
i.e., 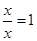 if
if 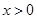 and
and 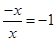 if
if 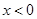
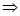
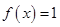 if
if 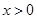 ,
, 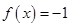 if
if 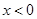 and
and 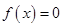 if
if 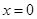
It is clear that domain of  is R as
is R as  is defined for
is defined for 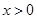 ,
, 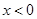 and
and 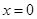 .
.
For all 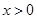 ,
, 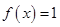 is a constant function and hence continuous.
is a constant function and hence continuous.
For all 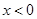 ,
, 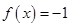 is a constant function and hence continuous.
is a constant function and hence continuous.
Therefore  is continuous on R – {0}.
is continuous on R – {0}.
Now Left Hand limit = 
Right Hand limit = 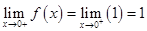
Since 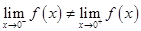
Therefore, 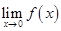 does not exist and hence
does not exist and hence  is discontinuous at
is discontinuous at 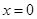 (only).
(only).
9. 
Ans. Given: 
At  L.H.L. =
L.H.L. = 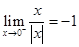 And
And 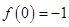
R.H.L. = 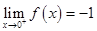
Since L.H.L. = R.H.L. = 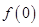
Therefore,  is a continuous function.
is a continuous function.
Now, for 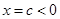

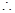
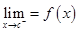
Therefore,  is a continuous at
is a continuous at 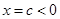
Now, for 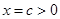
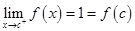
Therefore,  is a continuous at
is a continuous at 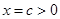
Hence, the function is continuous at all points of its domain.
10. 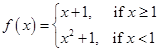
Ans. Given: 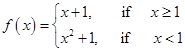
It is observed that  being polynomial is continuous for
being polynomial is continuous for  and
and 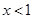 for all
for all 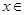 R.
R.
Continuity at 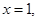 R.H.L. =
R.H.L. = 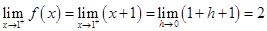
L.H.L. = 
And 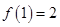
Since L.H.L. = R.H.L. = 
Therefore,  is a continuous at
is a continuous at 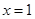 for all
for all 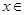 R.
R.
Hence,  has no point of discontinuity.
has no point of discontinuity.
11. 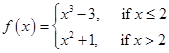
Ans. Given: 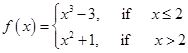
At 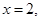 L.H.L. =
L.H.L. = 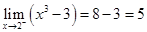
R.H.L. = 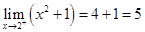
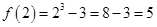
Since L.H.L. = R.H.L. = 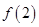
Therefore,  is a continuous at
is a continuous at 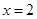
Now, for 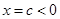
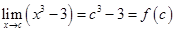 and
and 
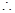
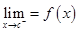
Therefore,  is a continuous for all
is a continuous for all 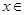 R.
R.
Hence the function has no point of discontinuity.
12. 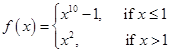
Ans. Given: 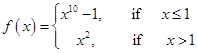
At 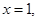 L.H.L. =
L.H.L. = 
R.H.L. = 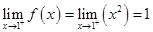
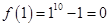
Since L.H.L. 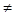 R.H.L.
R.H.L.
Therefore,  is discontinuous at
is discontinuous at 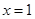
Now, for 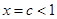
 and for
and for 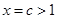
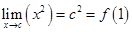
Therefore,  is a continuous for all
is a continuous for all 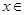 R – {1}
R – {1}
Hence for all given function 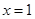 is a point of discontinuity.
is a point of discontinuity.
NCERT Solutions class 12 Maths Exercise 5.1 Part-1
13. Is the function defined by  a continuous function?
a continuous function?
Ans. Given: 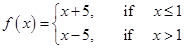
At 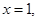 L.H.L. =
L.H.L. = 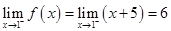
R.H.L. = 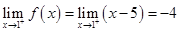
Since L.H.L. 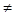 R.H.L.
R.H.L.
Therefore,  is discontinuous at
is discontinuous at 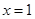
Now, for 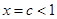
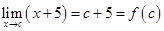 and for
and for 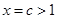
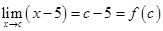
Therefore,  is a continuous for all
is a continuous for all 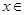 R – {1}
R – {1}
Hence  is not a continuous function.
is not a continuous function.
Discuss the continuity of the function 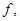 where
where  is defined by:
is defined by:
14. 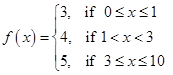
Ans. Given: 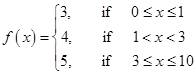
In the interval 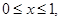
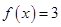
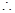
 is continuous in this interval.
is continuous in this interval.
At 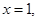 L.H.L. =
L.H.L. =  and R.H.L. =
and R.H.L. = 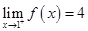
Since L.H.L. 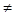 R.H.L.
R.H.L.
Therefore,  is discontinuous at
is discontinuous at 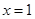
At 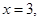 L.H.L. =
L.H.L. = 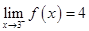 and R.H.L. =
and R.H.L. = 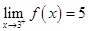
Since L.H.L. 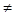 R.H.L.
R.H.L.
Therefore,  is discontinuous at
is discontinuous at 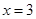
Hence,  is discontinuous at
is discontinuous at 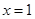 and
and 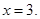
15. 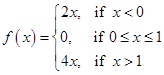
Ans. Given: 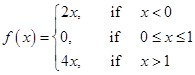
At  L.H.L. =
L.H.L. = 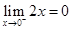 and R.H.L. =
and R.H.L. = 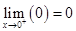
Since L.H.L. = R.H.L.
Therefore,  is continuous at
is continuous at 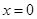
At 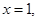 L.H.L. =
L.H.L. = 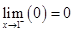 and R.H.L. =
and R.H.L. = 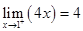
Since L.H.L. 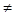 R.H.L.
R.H.L.
Therefore,  is discontinuous at
is discontinuous at 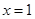
When 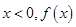 being a polynomial function is continuous for all
being a polynomial function is continuous for all 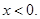
When 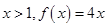 . It is being a polynomial function is continuous for all
. It is being a polynomial function is continuous for all 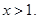
Hence 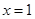 is a point of discontinuity.
is a point of discontinuity.
16. 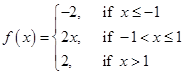
Ans. Given: 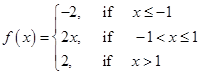
At 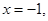 L.H.L. =
L.H.L. = 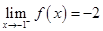 and R.H.L. =
and R.H.L. = 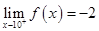
Since L.H.L. = R.H.L.
Therefore,  is continuous at
is continuous at 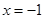
At 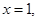 L.H.L. =
L.H.L. = 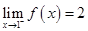 and R.H.L. =
and R.H.L. = 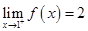
Since L.H.L. = R.H.L.
Therefore,  is continuous at
is continuous at 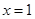
NCERT Solutions class 12 Maths Exercise 5.1 Part-1
NCERT Solutions Class 12 Maths PDF (Download) Free from myCBSEguide app and myCBSEguide website. Ncert solution class 12 Maths includes text book solutions from both part 1 and part 2. NCERT Solutions for CBSE Class 12 Maths have total 20 chapters. 12 Maths NCERT Solutions in PDF for free Download on our website. Ncert Maths class 12 solutions PDF and Maths ncert class 12 PDF solutions with latest modifications and as per the latest CBSE syllabus are only available in myCBSEguide
CBSE App for Class 12
To download NCERT Solutions for class 12 Physics, Chemistry, Biology, History, Political Science, Economics, Geography, Computer Science, Home Science, Accountancy, Business Studies and Home Science; do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT solutions, NCERT Exemplar solutions, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.

Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
Learn8 App
Practice unlimited questions for Entrance tests & government job exams at ₹99 only
Install Now