Miscellaneous Exercise
1. In a parabolic reflector is 20 cm in diameter and 5 cm deep, find the focus.
Ans. A parabolic reflector with diameter PR = 20 cm and OQ = 5 cm
Vertex of parabola is (0, 0)
Let focus of the parabola be 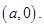
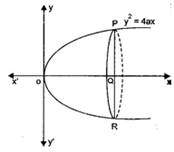
Now, PR = 20 cm
 PQ = 10 cm
PQ = 10 cm
 Coordinate of the point P are (5, 10)
Coordinate of the point P are (5, 10)
Since the point lies on the parabola 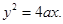

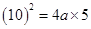

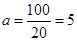
2. An arch is in the form of a parabola with its axis vertical. The arch is 10 m high and 5 m wide at the base. How wide is it 2 m from the vertex of the parabola?
Ans. Let AB be the parabolic arch having O as the vertex and OY as the axis.

The parabola is of the form 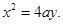
Now, CD = 5 m
 OD = 2.5 m
OD = 2.5 m
And BD = 10 m
Therefore, coordinates of point B are (2.5, 10).
Since the point B lies on the parabola 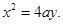

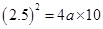

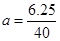

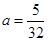
 Equation of the parabola is
Equation of the parabola is 

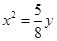
Let PQ = 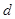
 NQ =
NQ = 
 Coordinate of the point Q are
Coordinate of the point Q are 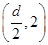
Since the point Q lies on the parabola 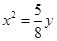





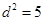

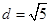
Therefore, width of arch is 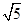 m = 2.24 m approx.
m = 2.24 m approx.
3. The cable of a uniformly loaded suspension bridge hange in the form of a parabola. The roadway which is horizontal and 100 m long is supported by vertical wires attached to the cable, the longest wire being 30 m and the shortest being 6 m. Find the length of a supporting wire attached to the roadway 18 m from the middle.
Ans. Let AOB be the cable of uniformly loaded suspension bridge. Let AL and BM be the longest wires of length 30 m each. Let OC be the shortest wire of length 6 m and LM be the roadway.
Now AL = BM = 30 m, OC = 6 m and LM = 100 m
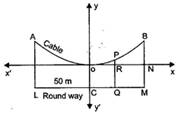
 LC = CM =
LC = CM = 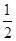 LM = 50 m
LM = 50 m
Let O be the vertex and axis of the parabola be 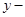 axis.
axis.
Therefore, the equation of the parabola in standard form is 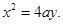
 Coordinates of the point B are (50, 24)
Coordinates of the point B are (50, 24)
Since point B lies on the parabola 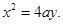

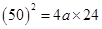

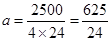
Therefore, equation of parabola is 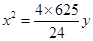

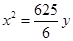
Let length of the supporting wire PQ at a distance of 18 m be 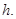
 OR = 18 m and PR = PQ – QR = PQ – OC =
OR = 18 m and PR = PQ – QR = PQ – OC = 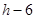
 Coordinates of point P are
Coordinates of point P are 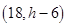
Now, since the point P lies on parabola 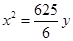

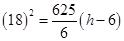





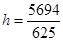 9.11 m approx.
9.11 m approx.
4. An arch is in the form of a semi-ellipse. It is 8 m wide and 2 m high at the centre. Find the height of the arch at a point 1.5 m from one end.
Ans. Given: Width of elliptical arch (AB)
= 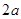 = 8
= 8 
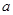 = 4 m
= 4 m
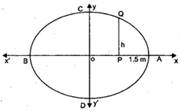
Height of the centre (OB) = 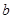 = 2 m
= 2 m
The axis of ellipse is 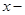 axis.
axis.
Therefore, the equation of ellipse in standard form is  .
.



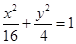
Now, AP = 1.5 m
 OP = OA – AP = 4 – 1.5 = 2.5 m
OP = OA – AP = 4 – 1.5 = 2.5 m
Let PQ = 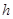 , then coordinates of Q are
, then coordinates of Q are 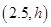
Since the point Q lies on the ellipse 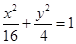

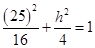

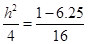

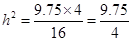

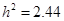

 = 1.56 m approx.
= 1.56 m approx.
5. A rod of length 12 cm moves with its ends always touching the coordinate axes. Determine the equation of the locus of a point P on the rod, which is 3 cm from the end in contact with the  axis.
axis.
Ans. Let AB be a rod of length 12 cm and P be any point on the rod such that PA = 3 cm and PB = 9 cm.
be any point on the rod such that PA = 3 cm and PB = 9 cm.
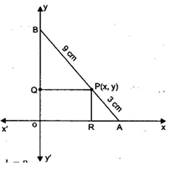
Let AR = 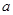 and BQ =
and BQ = 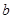
Then 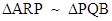

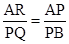



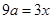

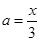
And 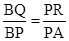

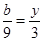

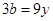

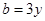
Now, OA = OR + AR = 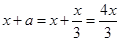
And OB = OQ + BQ = 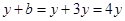
In right angled triangle AOB, 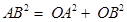

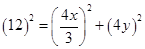

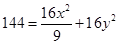

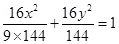

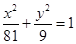
which is required locus of point P and which represents an ellipse.
6. Find the area of the triangle formed by the lines the vertex of the parabola 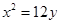 to the ends of its latus rectum.
to the ends of its latus rectum.
Ans. Given: Equation of parabola 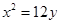 which is in the form of
which is in the form of 
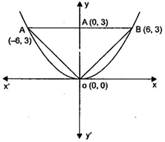

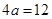

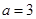
Focus of the parabola is (0, 3).
Let AB be the latus rectum of the parabola, then 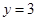

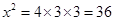

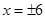
The coordinates of A are 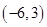
and coordinates of B are (6, 3).
 Area of
Area of 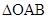
= 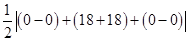
= 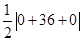
= 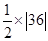 = 18 sq. units
= 18 sq. units
7. A man running a race leave no space course notes that the sum of the distances from the two flag posts from him is always 10 m and the distance between the flag posts is 8 m. Find the equation of the posts traced by the man.
Ans. Let 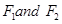 be two points where the flag parts are fixed on the ground. The origin O is the mid-point of
be two points where the flag parts are fixed on the ground. The origin O is the mid-point of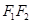 .
.
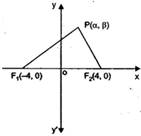

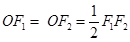 =
= 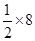 = 4 m
= 4 m
 Coordinates of F1 are
Coordinates of F1 are 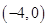 and F2 are (4, 0).
and F2 are (4, 0).
Let P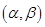 be any point on the track.
be any point on the track.

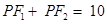

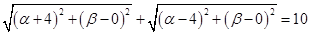


Squaring both sides, we have,

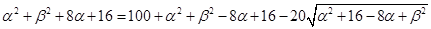


Squaring both sides again, we have,

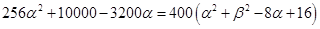

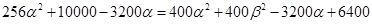

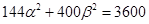

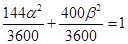

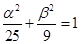
which is the required equation of locus of point P.
8. An equilateral triangle is inscribed n the parabola 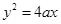 where one vertex is at the vertex of the parabola. Find the length of the side of the triangle.
where one vertex is at the vertex of the parabola. Find the length of the side of the triangle.
Ans. Given: Equation of the parabola 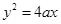 .
.
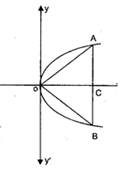
Let 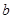 be the side of an equilateral
be the side of an equilateral 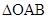 whose one vertex
whose one vertex
is the vertex of parabola and let OC = 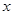
Now, AB = 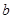
 AC = BC =
AC = BC = 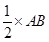 =
= 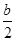
Coordinates of point A are 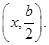 x’ x
x’ x
Since, point A lies on the parabola 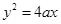

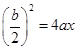

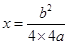


In right angled triangle 
OA2 = OC2 + AC2

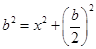

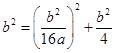

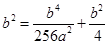

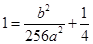

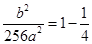

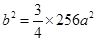

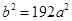

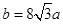
Therefore, the side of triangle is 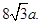

Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
Learn8 App
Practice unlimited questions for Entrance tests & government job exams at ₹99 only
Install Now