9.46*10**15

A light year is the distance light travels in one year (365 days).

It is generally accepted that if the coefficient of linear expansion is the same in all directions for a given material, then the coefficient of superficial expansion is approximately twice the coefficient of linear expansion and the coefficient of cubical expansion three times the coefficient of linear expansion.

{tex}\begin{aligned} & v=\frac{\text { Rate of flow }}{a} \\ & \text { Rate of flow }=0.2 \mathrm{~m}^3 \mathrm{~s}^{-1} a= \\ & v=\frac{0.2 \mathrm{~m}^s \mathrm{~s}^{-1}}{0.02 \mathrm{~m}^2}=10 \mathrm{~ms}^{-1} \end{aligned}{/tex}

Gravitational force is a conservative force because the work done against gravity is always conserved. For example: when we throw an object vertically above to the height of h metre then the work done against the gravity is W'=mgh where, mass of the object =m height =h acceleration due to gravity =-g and when the object falls back from the same height the work done is w" = mgh Here the total work done, W=W'+W"\ W=-mgh+mgh=0 Hence the total work done in travelling certain height and falling back to same place is zero, this is the property of conservative ford as the total work done depends only on the initial and final position of the object. Since the distance travelled is zero, the work done must be zero which is the same here. That's why gravitational force is a conservative force. Forces that do not store energy are called non-conservative of dissipative forces. Friction force is an example of a non-conservative force because it do not store energy rather than storing it. Frictional force oppose the motion of the object i.e. f   i ∝ −   Δ   r   i Thus the net work done by frictional force is negative as, Δ W = ∑ i f   i Δ r   i = − ∑ i | f   i | ri|<0 Negative sign indicates that the frictional force continually drains energy from the object as it moves over the surface . So it disipates energy in the form of heat.

the reflecting and transmitting power of a perfectly black body is 1,as it reflects the same amount of energy it absorbed.

as the body slow downs to zero at maximum point , the velocity at max point/height is 0.

30

Joule

Joule

Joule(J)

It is defined as the product of linear momentum of the partical and the perpendicular direction of the particle from axis of rotation . L=r×p L=rp sin Q SI unit =kg m²/sec Dimensional formula =[MLT¯¹][L] =[ML²T¯¹]

that energy can neither be created or not be destroyed

that energy can neither be created or not be destroyed..

The speed of light in vacuum (c) = 1 new unit of length s^-1
Time taken by light to reach the Earth,
t = 8 min + 20s = (8 {tex}\times{/tex} 60 + 20) s = 500 s
{tex}\therefore{/tex} Distance between the Sun and the Earth
= Speed of light {tex}\times{/tex} Time
x = c {tex}\times{/tex} t = 1 new unit of length s^-1 {tex}\times{/tex} 500 s
= 500 new units of length

Suppose, body is initially at rest and force 'F' is applied on the body to displace it through 'ds' along it's own direction. Then small workdene, dw = f.ds dw = fds Acc. to Newton's 2nd law of motion f = ma Therefore, dw = ma ds dw = m dv/dt ds dw = m ds/dt dv dw = mvdv Integrating both sides In order to increase it's velocity from 0-v workdone is given by -- w = m sign of integration upper limit v and lower limit 0 vdv w = m[v square/2] upper limit v and lower limit 0 w = m[v square/2-0] w = 1/2mv square workdone is stored in form of energy Therefore, K.E. = 1/2mv square

Dimensional variable are physical quantities that have numerical value and some specific dimensions. Examples include length, velocity, and acceleration, among other.

Similarities: Both opposes relative motion. ... Viscous force depends on the velocity gradient and area of contact and frictional force independent of area of contact and relative velocity. Viscosity of liquid decrease with increase in temperature, Where as friction independent of temperature.

benzene contracts in winter. So 5 litre of benzene will weigh more in winter than in summer.

20m height

Let us suppose that during travelling 40 m in last 2 sec its initial velocity was u. Then, ut+1/2 gt² =40 =2u-19.6=40 u=29.8 m/sec Now considering from top to this position, u=0and v=29.8m/s Then by, v²−u²=2gh we get, 29.8²-0²=2×9.8×h h=45.3m So, total height of tower =45.3+40=85.30 m

No

Sol or ans.

Angle= Length of arc/ Radius of arc ​ π/6=x/31 x =(31×π​)\6 ​ =31×3.14/6 =16.22cm ​

Velocity: Rate of change of displacement

Velocity is the rate of change of displacement. Acceleration is the rate of change of velocity.velocity Is a vector physical quantity because it consist of both magnitude and direction.accerleration is also a vector physical quantity as it is just the rate of change of velocity

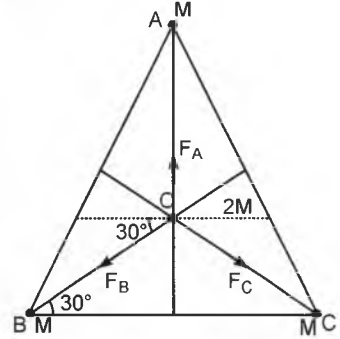
1. As AO = BO = CO = 1 m, hence we have
   {tex}\left| \overrightarrow { F _ { A } } \right| = \left| \overrightarrow { F _ { B } } \right| = \left| \overrightarrow { F _ { C } } \right| = \frac { G M \cdot 2 M } { ( 1 ) ^ { 2 } } = 2 G M ^ { 2 }{/tex}
   If we consider direction parallel to BC as x-axis and perpendicular direction as y-axis, then as shown in figure, we have
   {tex}\vec { F } _ { A } = 2 G M ^ { 2 } \hat { j }{/tex}
   {tex}\vec { F } _ { B } = \left( - 2 G M ^ { 2 } \cos 30 ^ { \circ } \hat { i } - 2 G M ^ { 2 } \sin 30 ^ { \circ } \hat { j } \right){/tex}
   and {tex}\overrightarrow { F _ { C } } = \left( 2 G M ^ { 2 } \cos 30 ^ { \circ } \hat { i } - 2 G M ^ { 2 } \sin 30 ^ { \circ } \hat { j } \right){/tex}
   Therefore, the net force on mass 2M placed at the centroid O is given by,
   {tex}\vec { F } = \vec { F } _ { A } + \vec { F } _ { B } + \vec { F } _ { C } {/tex} 
   {tex}= 2 G M ^ { 2 } \left[ j + \left( - \frac { \sqrt { 3 } } { 2 } \hat { i } - \frac { 1 } { 2 } \hat { i } \right) + \left( \frac { \sqrt { 3 } } { 2 } \hat { i } - \frac { 1 } { 2 } \hat { j } \right) \right]{/tex}
   {tex}=0{/tex}

The work obtained in bringing a body from infinity to a point in gravitational field is called gravitational potential energy. Force of attraction between the earth and the object when an object is at the distance a from the center of the earth. F=x2GmM.

Distance to market s=2.5km=2.5×10³ =2500m

Speed with which he goes to market =5km/h={tex}5 \frac{10^3}{3600}=\frac{25}{18} \mathrm{~m} / \mathrm{s}{/tex}

Speed with which he comes back = {tex}7.5 \mathrm{~km} / \mathrm{h}=7.5 \times \frac{10^3}{3600}=\frac{75}{36} \mathrm{~m} / \mathrm{s}{/tex}

(a)Average velocity is zero since his displacement is zero.

(b) (i)Since the initial speed is 5km/s and the market is 2.5 km away,time taken to reach market: {tex}\frac{2.5}{5}=1 / 2 \mathrm{~h}=30 \text { minutes. }{/tex}

Average speed over this interval =5km/h

(ii)After 30 minutes,the man is travelling wuth 7.5 km/h speed for 50-30=20 minutes.The distance he covers in 20 minutes :{tex}7.5 \times \frac{1}{3}=2.5 \mathrm{~km}{/tex}

His average speed in 0 to 50 minutes: V_avg ​ =distance traveled/time {tex}=\frac{2.5+2.5}{(50 / 60)}=6 \mathrm{~km} / \mathrm{h}{/tex}

(iii)In 40-30=10 minutes he travels a distance of : {tex}7.5 \times \frac{1}{6}=1.25 \mathrm{~km}{/tex}

{tex}\mathrm{V}_{\mathrm{avg}}=\frac{2.5+1.25}{(40 / 60)}=5.625 \mathrm{~km} / \mathrm{h}{/tex}

As the depth increased the mass of the earth decreases. At the surface of earth this value will be maximum because radius will be maximum. When radius becomes less this value also decreases. Hence acceleration due to gravity decreases with increase in the depth.

g is 0 at centre of earth

A solid cylinder rolling up an inclination is shown in the following figure.

Initial velocity of the solid cylinder, v = 5 m/s
Angle of inclination, {tex}\theta = 30 ^ { \circ }{/tex}
Height reached by the cylinder = h

1. Energy of the cylinder at point A will be purely kinetic due to the rotation and translational motion. Hence, total energy at A
   = KE_rot + KE_trans
   =   {tex}\frac { 1 } { 2 } I \omega ^ { 2 } + \frac { 1 } { 2 } m v ^ { 2 }{/tex}

   The energy of the cylinder at point B will be purely in the form of gravitational potential energy  = mgh
   Using the law of conservation of energy, we can write:
   {tex}\frac { 1 } { 2 } I \omega ^ { 2 } +\frac { 1 } { 2 } m v ^ { 2 } = m g h{/tex}
   Moment of inertia of the solid cylinder, {tex}I = \frac { 1 } { 2 } m r ^ { 2 }{/tex}
   {tex}\therefore \frac { 1 } { 2 } \left( \frac { 1 } { 2 } m r ^ { 2 } \right) \omega ^ { 2 } + \frac { 1 } { 2 } m v ^ { 2 } = m g h{/tex}
   {tex}\frac { 1 } { 4 } I \omega ^ { 2 } + \frac { 1 } { 2 } m v ^ { 2 } = m g h{/tex}
   But we have the relation, {tex}v = r \omega{/tex}
   {tex}\therefore \frac { 1 } { 4 } v ^ { 2 } + \frac { 1 } { 2 } v ^ { 2 } = g h{/tex}
   {tex}\frac { 3 } { 4 } v ^ { 2 } = g h{/tex}
   {tex}\therefore h = \frac { 3 } { 4 } \frac { v ^ { 2 } } { g }{/tex}
   {tex}= \frac { 3 } { 4 } \times \frac { 5 \times 5 } { 9.8 } = 1.91 \mathrm { m }{/tex}

   To find the distance covered along the inclined plane
   In {tex}\Delta A B C{/tex}:
   {tex}\sin \theta = \frac { B C } { A B }{/tex}
   {tex}\sin 30 ^ { \circ } = \frac { h } { A B }{/tex}
   {tex}A B = \frac { 1.91 } { 0.5 } = 3.82 \mathrm { m }{/tex}
   Hence, the cylinder will travel 3.82 m up the inclined plane.

2. {tex}v = \left( \frac { 2 g h } { 1 + \frac { K ^ { 2 } } { R ^ { 2 } } } \right) ^ { \frac { 1 } { 2 } }{/tex}
   {tex}\therefore v = \left( \frac { 2 g A B \sin \theta } { 1 + \frac { K ^ { 2 } } { R ^ { 2 } } } \right) ^ { \frac { 1 } { 2 } }{/tex}
   For the solid cylinder, {tex}K ^ { 2 } = \frac { R ^ { 2 } } { 2 }{/tex}
   {tex}\therefore v = \left( \frac { 2 g A B \sin \theta } { 1 + \frac { 1 } { 2 } } \right) ^ { \frac { 1 } { 2 } }{/tex}
   {tex}= \left( \frac { 4 } { 3 } g A B \sin \theta \right) ^ { \frac { 1 } { 2 } }{/tex}
   The time taken to return to the bottom is:
   {tex}t = \frac { A B } { v }{/tex}
   {tex}= \frac { A B } { \left( \frac { 4 } { 3 } g A B \sin \theta \right) ^ { \frac { 1 } { 2 } } } = \left( \frac { 3 A B } { 4 g \sin \theta } \right) ^ { \frac { 1 } { 2 } }{/tex}
   {tex}= \left( \frac { 11.46 } { 19.6 } \right) ^ { \frac { 1 } { 2 } } = 0.7645{/tex}
   So the total time taken by the cylinder to return to the bottom is (2 {tex}\times{/tex} 0.764)= 1.53 s.as time of ascend is equal to time of descend for the following problem.