Ask questions which are clear, concise and easy to understand.
Ask QuestionPosted by Mehak Preet Kaur 2 years, 3 months ago
- 2 answers
Posted by Tripti Rawat 2 years, 3 months ago
- 5 answers
Posted by Sona Cutie 2 years, 3 months ago
- 0 answers
Posted by Sabari Karthikeyan 2 years, 3 months ago
- 5 answers
Posted by Nikita Kumari 2 years, 3 months ago
- 3 answers
Posted by Test Account 2 years, 3 months ago
- 0 answers
Posted by Chandrima Choudhary 2 years, 3 months ago
- 0 answers
Posted by Shivam Yadav 2 years, 3 months ago
- 1 answers
Posted by Krish Kanyal 2 years, 3 months ago
- 2 answers
Aditya Kediya 2 years, 3 months ago
Posted by Ravi Teza 2 years, 3 months ago
- 2 answers
Posted by Tejas T. 2 years, 3 months ago
- 0 answers
Posted by Tejas T. 2 years, 3 months ago
- 0 answers
Posted by Palak Singh 2 years, 3 months ago
- 3 answers
Posted by Mahash Thennavan 2 years, 3 months ago
- 5 answers
Posted by Abhinav Dev 2 years, 3 months ago
- 3 answers
Mohammed Affiq 2 years, 3 months ago
Posted by Sameeksha Rawat 2 years, 3 months ago
- 5 answers
Posted by Kirti Rane 2 years, 3 months ago
- 1 answers
Preeti Dabral 2 years, 3 months ago
LHS =sinA−sinBcosA+cosB+cosA−cosBsinA+sinB=sin2A−sin2B+cos2A−cos2B(cosA+cosB)(sinA+sinB)=(sin2A+cos2A)−(sin2B+cos2B)(cosA+cosB)(sinA+sinB)=(cosA+cosB)(sinA+sinB)=0= RHS
Posted by Bunny . 2 years, 3 months ago
- 1 answers
Preeti Dabral 2 years, 3 months ago
Let (-4, 6) divide AB internally in the ratio k:1. Using the section formula, we get
(−4,6)=(3k−6k+1,−8k+10k+1)
So, −4=3k−6k+1
i.e., -4k - 4 = 3k - 6
i.e., 7k = 2
i.e., k:1 = 2:7
The same can be checked for the y-coordinate also.
Therefore, the ratio in which the point (-4,6) divides the line segment AB is 2: 7.
Posted by Aniket Topwal 2 years, 3 months ago
- 1 answers
Preeti Dabral 2 years, 3 months ago
Let A(a, a), B(-a, -a) C(−√3a,,√3a)
AB=√(−a−a)2+(−a−a)2=√8a2=2√2a
BC=√(−√3a+a)2+(√3a+a)2=2√2a
AC=√(−√3a−a)2+(√3a−a)2=2√2a
∴ AB = BC = AC = 2√2a
arΔABC=√34×(side)2
=√34×(2√2a)2
=2√3a2 sq. units
Posted by Ritu Kumari 2 years, 3 months ago
- 2 answers
Posted by Vis H 2 years, 3 months ago
- 1 answers
Preeti Dabral 2 years, 3 months ago
Given: A circle with centre O. A tangent CD at C.
Diameter AB is produced to D.
BC and AC chords are joined, ∠BAC = 30°
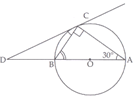
To prove: BC = BD
Proof: DC is tangent at C and, CB is chord at C.
Therefore, ∠DCB = ∠BAC [∠s in alternate segment of a circle]
⇒ ∠DCB = 30° …(i) [∵ ∠BAC = 30° (Given)]
AOB is diameter. [Given]
Therefore, ∠BCA = 90° [Angle in s semi circle]
Therefore, ∠ABC = 180° - 90° - 30° = 60°
In ΔBDC,
Exterior ∠B = ∠D + ∠BCD
⇒ 60° = ∠D + 30°
⇒ ∠D = 30° …(ii)
Therefore, ∠DCB = ∠D = 30° [From (i), (ii)]
⇒ BD = BC [∵ Sides opposite to equal angles are equal in a triangle]
Hence, proved.
Posted by Aishiki Jana 2 years, 3 months ago
- 1 answers
Pranjal Gupta 2 years, 3 months ago

myCBSEguide
Trusted by 1 Crore+ Students

Test Generator
Create papers online. It's FREE.

CUET Mock Tests
75,000+ questions to practice only on myCBSEguide app
 myCBSEguide
myCBSEguide
Ankit Kumar 2 years, 3 months ago
1Thank You