CBSE > Class 10 > Mathematics | CBSE Board | Homework Help

By working out difficult sums Refer sample papers View previous years question paper In the answer sheet make sure to always attend the question If ever you don't know the solution for any question then just attend it and draw neat diagram as every step has marks (write Given, to prove, construct- which contains 1 mark)

0Thank You

Aastha Vats 2 years, 2 months ago

0Thank You

ANSWER

Find LCM and HCF 26and91

Posted by Mohd Ali 2 years, 2 months ago

CBSE > Class 10 > Mathematics

2 answers

Aastha Vats 2 years, 2 months ago

HCF :- 13 and LCM :- 14×13 = 182

0Thank You

Aryan Tomar 2 years, 2 months ago

LCM :- 182 HCF :- 14

0Thank You

ANSWER

Solve for x and y: x/a + y/b=2 ; ax - by = a2 - b2.

Posted by Nirmitee Kushwaha 2 years ago

CBSE > Class 10 > Mathematics

1 answers

Preeti Dabral 2 years ago

$\frac { x } { a } + \frac { y } { b } = 2$
$\frac { b x + a y } { a b } = 2$
$bx + ay = 2ab$ ........(i)
$ax - by = (a^2 - b^2)$ ...(ii)
Multiplying (i) by b and (ii) by a
$b^2x + bay = 2ab^2$ .............(iii)
$a^2x - bay = a(a^2 - b^2)$ ........(iv)
Adding (iii) and (iv),we get
$b^2x + a^2x = 2ab^2 + a^3 - ab^2$
$x(b^2 + a^2) = 2ab^2 + a^3 - ab^2$
$x(b^2 + a^2) = ab^2 + a^3$
$x(b^2 + a^2) =a(b^2 + a^2)$
$x = \frac { a \left( b ^ { 2 } + a ^ { 2 } \right) } { \left( b ^ { 2 } + a ^ { 2 } \right) } = a$
Putting x = a in (i),we get
$b \times a + a y = 2 a b$
$a y = 2 a b - a b \Rightarrow a y = a b$ or y = b
$\therefore$ solution is x = a, y = b

0Thank You

ANSWER

6/2(1+2)

Posted by Saket Tiwari 2 years, 2 months ago

CBSE > Class 10 > Mathematics

2 answers

Omya Aryan 2 years, 2 months ago

6/2(1+2)= 6/2(3)=18/2=9

2Thank You

Saket Tiwari 2 years, 2 months ago

2Thank You

ANSWER

NCERT Solutions?

Posted by Tripti Rawat 2 years, 2 months ago

CBSE > Class 10 > Mathematics

2 answers

Mohd Ali 2 years, 2 months ago

Find LCM and HCF 26and91

0Thank You

Krishna Kanwar 2 years, 2 months ago

Yess

0Thank You

ANSWER

I not able to understand surface area and area related to circle. So, how I prepare these two chapters in 15 to 16 days any trick

Posted by Shruti Singh 2 years, 2 months ago

CBSE > Class 10 > Mathematics

1 answers

Shanu Yadav 2 years, 2 months ago

Learn all the formulas of both the chapters and practice ncert questions then refer rs agrawal or rd sharma book

3Thank You

ANSWER

How I prepare for exam

Posted by Shruti Singh 2 years, 2 months ago

CBSE > Class 10 > Mathematics

2 answers

Shruti Singh 2 years, 2 months ago

My pre boards are going on , so I'm very nervous

1Thank You

Shanu Yadav 2 years, 2 months ago

I'm also nervous regarding exams.

0Thank You

ANSWER

32+34

Posted by Yashvi Nain 2 years, 2 months ago

CBSE > Class 10 > Mathematics

5 answers

Tanya Chauhan 2 years, 2 months ago

0Thank You

Saad Chaudhary 2 years, 2 months ago

0Thank You

Shanu Yadav 2 years, 2 months ago

0Thank You

Vivek Sisodiya 2 years, 2 months ago

0Thank You

Vivek Sisodiya 2 years, 2 months ago

2Thank You

ANSWER

If fixi =625 and fi =25 then the value of x =

Posted by Umpi Hibu 2 years, 2 months ago

CBSE > Class 10 > Mathematics

3 answers

Shanu Yadav 2 years, 2 months ago

625/25=25

0Thank You

Dev Katiyar 2 years, 2 months ago

1Thank You

Vivek Sisodiya 2 years, 2 months ago

Answer is 25

0Thank You

ANSWER

2/6 - 4/9 × 5.9

Posted by Vijayasri Venkatesan 2 years, 2 months ago

CBSE > Class 10 > Mathematics

3 answers

Omya Aryan 2 years, 2 months ago

-2.2

0Thank You

Choudhary Kundan 2 years, 2 months ago

Use app Login Question Find x if 4x+6x=9x A ln2−ln3ln(3−1)+ln2 Correct Answer B ln2−ln3ln(5−1)+ln2 C ln2−ln3ln(5−1)−ln2 D ln3−ln2ln(5−1)+ln2 Medium Open in App Solution Verified by Toppr Correct option is A) 6x+4x=9x divide by 4x to form (23)x 4x6x+1=4x9x (23)x+1=(23)2x ((23)x)2−(23)x−1=0 So (23)x=21±1−4.1(−1)=21±,5 For positive solution (23)x=21+5 Applying logarithm xln(23)=ln(21+5) lnba=lna−lnb x=ln3−ln2ln(1+5)−ln2.

0Thank You

Uttam Soni 2 years, 2 months ago

-0. 98

0Thank You

ANSWER

For an A.P., if T25 - T20, then find 'd'.

Posted by Gayatri Pathade 2 years, 2 months ago

CBSE > Class 10 > Mathematics

2 answers

Aastha Vats 2 years, 2 months ago

a+24d - (a+19d) = 24d - 19 d = 5d d = 1/5

0Thank You

Muzaif Ur Rahman 2 years, 2 months ago

Write question properly

0Thank You

ANSWER

Prove that the perpendicular at the point of contact of the tangent to a circle passes through the centre.

Posted by Punam Kumari 2 years, 2 months ago

CBSE > Class 10 > Mathematics

1 answers

Muzaif Ur Rahman 2 years, 2 months ago

In circle C(O,r) AB is a tangent CONST. Join OA AND OB And OC PROOF IN TRIANGLE(OAC) & TRIANGLE(OBC) OC=OC -----common side ANGLE OAC = OBC ANGLE C=90° ------ BY RADIUS TANGENT THEORM THEREFORE BY RHS CRITERIA TRIANGLE OAC=OBC THEREFORE OC IS PERPENDICULAR TO AB hence PROVED

1Thank You

ANSWER

Amit's father is planning some construction work in his terrace area. He ordered 360 bricks and instructed the supplier to keep the bricks in such a way that the bottom row has 30 bricks and next is one less than that and so on. The supplier stacked these 360 bricks in the following manner: 30 bricks in the bottom row, 29 bricks in the next row, 28 bricks in the row next to it and so on. Using the above information, answer the following questions: (i) Which row has 26 bricks?

Posted by Aditya . 2 years, 2 months ago

CBSE > Class 10 > Mathematics

4 answers

Saad Chaudhary 4 months, 2 weeks ago

5th row

6Thank You

Harsh Harsh 2 years, 2 months ago

5 th row

3Thank You

Punam Kumari 2 years, 2 months ago

Sorry bro ye galat h 6

0Thank You

Punam Kumari 2 years, 2 months ago

6 rows

3Thank You

ANSWER

Which row has 26 bricks?

Posted by Aditya . 2 years, 2 months ago

CBSE > Class 10 > Mathematics

1 answers

Rithika S 2 years, 2 months ago

5th row

0Thank You

ANSWER

The digit of a positive number of three digit are in AP and their sum is 15.The number obtained by reversing the digit is 594 less than the original number.Find the number.

Posted by T G Full 2 years ago

CBSE > Class 10 > Mathematics

1 answers

Preeti Dabral 2 years ago

Let the digits of the required 3 - digit number at hundreds, tens and ones places be $a - d, ~ a , ~ a + d$ respectively
Then their sum = 15
i.e, $a - d + a + a + d$ = 15
$\Rightarrow$ $3a$ = 15
$\Rightarrow$ $a$ = 5
Required three digit number = $100(a - d) + 10a + a + d$
= $100a - 100d + 10a + a + d$
= $111a - 99d$
Number obtained by reversing the digits = $100(a + d) + 10a + a - d$
= $100a + 100d + 10a + a - d$
= $111a + 99d$
According to the question,
$111a + 99d = 111a - 99d - 594$
$\Rightarrow$ $594 = 111a - 99d - 111a - 99d$
$\Rightarrow$ $594 = -198d$
$\Rightarrow$ $\frac { - 594 } { 198 } = d$
$d = -3$
The number is 111a - 99d
$111 \times 5 - 99 \times - 3$ = 852
555 + 297 = 852
Therefore, Number is 852.

0Thank You

ANSWER

Can you tell me 8 chapter?

Posted by Mitali Negi 2 years, 2 months ago

CBSE > Class 10 > Mathematics

1 answers

Harsh Harsh 2 years, 2 months ago

Introduction to trigonometry

0Thank You

ANSWER

Which chapter was not come in syllabus

Posted by Sukhveer Singh 2 years, 2 months ago

CBSE > Class 10 > Mathematics

2 answers

Neelam Devi 2 years, 2 months ago

Construction Chapter no. 11

2Thank You

Adarsh Jha 2 years, 2 months ago

2Thank You

ANSWER

How would we solve identity problem of trigonometry

Posted by Akm Akm 2 years, 2 months ago

CBSE > Class 10 > Mathematics

1 answers

Parth Yadav 2 years, 2 months ago

It depends on question. Practice the ncert sum by reffering to answer. You will get an idea.

0Thank You

ANSWER

In given fig. O is the centre of a circle, If the area of the sector OAPB is 5/36 times the area of the circle then find the value of x.

Posted by Srusthi Sb 2 years ago

CBSE > Class 10 > Mathematics

1 answers

Preeti Dabral 2 years ago

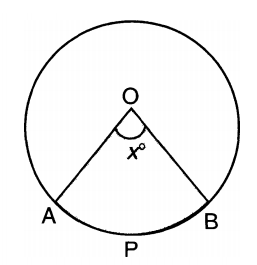
As per given condition
Area of sector OAPB = $\frac { 5 } { 36 }$ times the area of circle
$\therefore \quad \pi r ^ { 2 } \times \frac { \theta } { 360 } = \frac { 5 } { 36 } \pi r ^ { 2 }$
$\therefore \quad \pi r ^ { 2 } \times \frac { x } { 360 } = \frac { 5 } { 36 } \pi r ^ { 2 }$
$\frac { x } { 360 } = \frac { 5 } { 36 }$ or,
^{$\begin{array}{l}36\mathrm x=360\;\times5\end{array}$

$\\\mathrm x=\frac{360\;\times5}{36}$

$\\\mathrm x=\;10\;\times5$

$\\\mathrm x\;=\;50$}

0Thank You

ANSWER

Solve the following pair of linear equations by the substitution method x+y=14,x−y=4

Posted by Jainpriyanka Mehta 2 years, 2 months ago

CBSE > Class 10 > Mathematics

2 answers

Sparshika Singh 2 years, 2 months ago

x+y=14 x=14-y___(1) Substituting value in second equation x-y=4 14-y-y=4 14-2y=4 10=2y y=5 From (1) x=14-y =14-5 =9

0Thank You

Banty Kumari 2 years, 2 months ago

X+Y=14-------(i) X-Y=4--------(ii) From eq----(i) X+Y=14 Y=14-x---------(iii) Putting its value in eq-------(ii) X-Y=4 X-(14-x)=4 X-14+x=4 2x-14=4 2x=4+14 2x=18 X=18/2 X=9 Putting it's value in eq-------(iii) Y=14-x Y=14-9 Y=5 •:x=9,y=5

0Thank You

ANSWER

The pilot of an aircraft flying horizontally at a speed of 1200km / h * r observes that the angle of depression of a point on the ground changes from 30 deg to 45 deg in 15 seconds. Find the height at which the aircraft is flying.

Posted by Ramraj Kumar 2 years, 2 months ago

CBSE > Class 10 > Mathematics