CBSE > Class 10 > Mathematics | CBSE Board | Homework Help | myCBSEguide

No products in the cart.

Ask questions which are clear, concise and easy to understand.

Ask Question

Express the trigonometric ratios sin A, sec A and tan A in terms of cot A.

Posted by _Jass_ Mahey_ 2 years, 1 month ago

CBSE > Class 10 > Mathematics

0 answers

ANSWER

Half the perimeter of a rectangle garden. whose lenght is 4 m more than it's width, is 36 m. Find the dimension of the garden

Posted by _Jass_ Mahey_ 2 years, 1 month ago

CBSE > Class 10 > Mathematics

2 answers

Muhammad Amaan Rahman 𝔸𝕄𝔸𝔸ℕ 𝕄𝕌ℍ𝔸𝕄𝕄𝔸𝔻 2 years, 1 month ago

Given : Half the perimeter of a garden whose length is 4 m more than its width is 36 m. To do: We have to find the dimensions of the garden. Solution : Perimeter of a rectangle of length l and breadth b=2(l+b). Let the length of the rectangle be l and the width of the rectangle be b. Therefore, Half the perimeter of rectangle = 2 (l + b) 2 Half the perimeter of rectangle =l+b. Given that half the perimeter of the rectangular garden =36 m. l+b=36 m......(i) It is given that, length of the rectangular garden is 4 m more than its width. This implies, l=b+4.......(ii) Substituting (ii) in (i), we get, b+4+b=36 2b=36−4 2b=32 b= 32/2 b=16 m Substitute b=16 in (ii) l=16+4 l=20 m Therefore, the length of the rectangular garden is 20 m and the width of the rectangular garden is 16 m.

Ankit Class 2 years, 1 month ago

Let length be l and breadth be b of rectangular garden. l= b+4. (l+b)/2 = 36 . l+b= 72. b+4 + b = 72. 2b+4= 72. 2b= 68. b= 34 m. l=b+4 = 34+4= 38 m.

ANSWER

In each of following find the value of 'ne for which the points are collinear. (7, -2), (5, 1), (3, k)

Posted by _Jass_ Mahey_ 2 years, 1 month ago

CBSE > Class 10 > Mathematics

0 answers

ANSWER

Find the area of the triangle whose vertices are : (2, 3), (-1, 0), (2, -4,) (-5, -1) , (3-5), (5, 2)

Posted by _Jass_ Mahey_ 2 years, 1 month ago

CBSE > Class 10 > Mathematics

0 answers

ANSWER

Evaluate the following: (1) sin 60°cos 30°+sin 30° cos 60° (2) 2tan²45° + cos²30°- -sin² 60°

Posted by _Jass_ Mahey_ 2 years, 1 month ago

CBSE > Class 10 > Mathematics

0 answers

ANSWER

Which term of the AP:121, 117,113,...,is it's first negative term? [Hint:find n for a/n<0]

Posted by _Jass_ Mahey_ 2 years, 1 month ago

CBSE > Class 10 > Mathematics

1 answers

Ankit Class 2 years, 1 month ago

a=121 , d= -4 let l=o . a+ (n-1)d = l . 121+(n-1)x-4= 0. (n-1)=121/4. n= 30.25+1. n = 31.25 , Here we know that n always be natural number hence n should be 31. n= 31.

ANSWER

Exercise 1.1 q 2

Posted by Sharvan Verma 2 years, 1 month ago

CBSE > Class 10 > Mathematics

1 answers

_Jass_ Mahey_ 2 years, 1 month ago

Let 'a' be a postive odd integer also. Let 'q' be quotient and 'r' be the remainder after dividing by six. Then a=6q+r, where 0<r<6. Postive integer are 1,2,3,4,5 a=6q+0, 6q+1, 6q+2, 6q+3, 6q+4, 6q+5. But a=6q, 6q+2 and 6q+4 are even. When a is add it is the form 6q+1, 6q+3, 6q+5 for some integer q. .°. Hence proved...

ANSWER

Find the value of a and p for which the following pair of linear equation has infinite solutions 2x + 3y=7 ax +(a+p)y=28

Posted by Ansika Shetty 2 years, 1 month ago

CBSE > Class 10 > Mathematics

1 answers

Ankit Class 2 years, 1 month ago

(2/a )=(3/a+p)= (7/28). 2/a= 7/28 . 2/a= 1/4 . a= 8. (3/p+8)= 7/28. (3/p+8)= 1/4. P+8 = 12. P= 4 .

ANSWER

From geometry, you are already familiar with the construction of angles of __°, _°and__°and__°.

Posted by _Jass_ Mahey_ 2 years, 1 month ago

CBSE > Class 10 > Mathematics

2 answers

Ankit Class 2 years, 1 month ago

0° , 30° ,45° ,60° , 90° ,120° , 135° ,150° , 180° any many more

Ankit Class 2 years, 1 month ago

😂

ANSWER

If 3cot A=4 , right angled at B, if tan A=1/√3 find the value of : sin A cos C+ cos A sin C

Posted by _Jass_ Mahey_ 2 years, 1 month ago

CBSE > Class 10 > Mathematics

1 answers

Ashish Tiwari 2 years, 1 month ago

1

ANSWER

Using Euclid's algorithm, find the largest number that divided 1251, 9377 and 15628 leaving remainder 1,2 and 3 respectively

Posted by Minato Uzmaki 2 years, 1 month ago

CBSE > Class 10 > Mathematics

1 answers

Ankit Class 2 years, 1 month ago

Subtract 1,2,3 from these no we get 1250, 9375, 15625 now use Euclid algorithm first in any of two then you get HCF of those two number then use again Euclid algorithm in HCF that you got with the third number

ANSWER

If 2sinQ=1 find the value of Q

Posted by Ishu Bhagat Singh Agarwal 2 years, 1 month ago

CBSE > Class 10 > Mathematics

2 answers

Prince Sharma 2 years, 1 month ago

2sinQ=1 =>sinQ=1/2 =>sinQ=sin30° [On comparing] =>Q=30°

Ishu Bhagat Singh Agarwal 2 years, 1 month ago

Sin30°

ANSWER

If the distance between the points (4,p) and (1,0) is 5, then the Value of p is

Posted by Sukhmandeep Kaur 2 years, 1 month ago

CBSE > Class 10 > Mathematics

3 answers

Prince Sharma 2 years, 1 month ago

(4 -1)² +(p - 0)² = 5². => 9 + p² =25. => p² = 16. => p = 4

Ritesh Chourasia 2 years, 1 month ago

4

_Jass_ Mahey_ 2 years, 1 month ago

P=3

ANSWER

The distance between the point P(-11/3,5) and Q(-2/3,5)

Posted by Anshuman Behera 1 year, 9 months ago

CBSE > Class 10 > Mathematics

3 answers

Ankit Class 2 years, 1 month ago

D=√ (-11+2/3)^2 +(5-5)^2 . D= √ 9 . D= 3 unit

Ankit Class 2 years, 1 month ago

I don' t like much calculation because l am little weak in calculation 🤣

Ankit Class 2 years, 1 month ago

Use distance formula

ANSWER

What is trigonometry ratio

Posted by Vishwaraj Kumar 2 years, 1 month ago

CBSE > Class 10 > Mathematics

2 answers

Ankit Class 2 years, 1 month ago

Ratio of any two side in a right angle triangle whose specific name was kept by their specific ratio like p/ h , b/ h , p/ b etc

Sukhmandeep Kaur 2 years, 1 month ago

Sin - Sine Cos - Cosine Tan - Tangent Cosec - Cosecant Sec - Secant Cot - Cotangent

ANSWER

Factorisation of 5x²-4-8x=0

Posted by Srishti Kumari 2 years, 1 month ago

CBSE > Class 10 > Mathematics

2 answers

Maya Shukla 2 years, 1 month ago

x=2 and -2/5

Prince Sharma 2 years, 1 month ago

5x²-8x-4=0 => 5x²-10x+2x-4=0 => 5x(x-2) + 2(x-2)=0 => (x-2)(5x+2)=0 => x=2,-2/5

ANSWER

If A=2n+13 B=n+7 Where n is any natural no. Find HCF of A and B

Posted by Vinjul Gaming 2 years, 1 month ago

CBSE > Class 10 > Mathematics

2 answers

Ankit Class 2 years, 1 month ago

HCF (A,B) = 1 . Because you can check by taking any natural number in place of n .

Prem Latha 2 years, 1 month ago

Ans

ANSWER

Find HCF of 660 and 704 by prime factorization.

Posted by Dikshant Yaduvanshi 2 years, 1 month ago

CBSE > Class 10 > Mathematics

2 answers

Maya Shukla 2 years, 1 month ago

Question acha hai 🤟

Maya Shukla 2 years, 1 month ago

660- 2,2,3,5,11. 704-2,2,2,2,2,2,11. Answer hai 22

ANSWER

The angle of elevation of the top of a tower 30 m high from the foot of another tower in the same plane is 60°. The angle of elevation of the top of second tower from the foot of the first tower is 30°. Find the distance between two towers and the height of the other tower. Also, find the length of the wire attached to the tops of both the towers.

Posted by Utkarsh Kumar Yadav 2 years, 1 month ago

CBSE > Class 10 > Mathematics

5 answers

Preeti Dabral 2 years, 1 month ago

Let distance between the two towers = AB = x m
and height of the other tower = PA = h m
Given that, height of the tower = QB = 30 m and $\angle$ QAB = 60°, $\angle$ PBA = 30°.

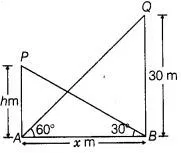
Now, in $\Delta Q A B, \tan 60^{\circ}=\frac{Q B}{A B}=\frac{30}{x}$
$\Rightarrow \sqrt{3}=\frac{30}{x}$
$x=\frac{x^{2}}{3}-\frac{x}{3}-\frac{x-3}{3}-x+0.5 \pi$
and in $\Delta P B A$
$\tan 30^{\circ}=\frac{P A}{A B}=\frac{h}{x}$
$\Rightarrow \frac{1}{\sqrt{3}}=\frac{h}{10 \sqrt{3}}$ $[\because x=10 \sqrt{3} \mathrm{m}]$
$\Rightarrow h = 10 m$
Hence, the requied distance and height are $10\sqrt3$ and 10m, respectively.

Ankit Class 2 years, 1 month ago

Ye figure draw kaise ki ho batao

Ankit Class 2 years, 1 month ago

PQ is the length of the wire attached to the tops of both the towers

Ankit Class 2 years, 1 month ago

PQ=√x^2 +(30-h)^2 . PQ=√(10√3)^2 + (30-10)^2 . PQ=√ 300+400. PQ=√700. PQ= 10√7 m

Ankit Class 2 years, 1 month ago

Math standard mein ye question puchha tha n mere mein bhi puchha tha set 1 tha mera 21 feb ko paper tha n

ANSWER

What would be the value of h = 20t-16t² at t =3/2 ? Interpret the result.

Posted by Vinayak Guleria. 2 years, 1 month ago

CBSE > Class 10 > Mathematics

1 answers

Preeti Dabral 2 years, 1 month ago

h = 20t - 16t²

= (20 x 3/2) - 16 x (3/2)²

= 30 - ( 16 x 9/4)

= 30 - 36 = -6

ANSWER

The distance between the points (0, 2√5) and (-2 √5, 0) is

Posted by Y Music 2 years, 1 month ago

CBSE > Class 10 > Mathematics

1 answers

Preeti Dabral 2 years, 1 month ago

The distance between the two points is 2√10 units .

ANSWER

In the given figure PQ is a tangent to a circle centred at O If aob =95 then measure of abq will be

Posted by Samerjot Sandhu 2 years, 1 month ago

CBSE > Class 10 > Mathematics

1 answers

Y Music 2 years, 1 month ago

The distance between the points (0, 2√5) and (-2 √5, 0) is

ANSWER

Find the distance between the following pairs (2, 3) , (4, 1

Posted by Vettri Vel 2 years, 1 month ago

CBSE > Class 10 > Mathematics

2 answers

_Jass_ Mahey_ 2 years, 1 month ago

√16

Sahil Singh 2 years, 1 month ago

This content has been hidden. One or more users have flagged this content as inappropriate. Once content is flagged, it is hidden from users and is reviewed by myCBSEguide team against our Community Guidelines. If content is found in violation, the user posting this content will be banned for 30 days from using Homework help section. Suspended users will receive error while adding question or answer. Question comments have also been disabled. Read community guidelines at https://mycbseguide.com/community-guidelines.html

Few rules to keep homework help section safe, clean and informative.

Don't post personal information, mobile numbers and other details.
Don't use this platform for chatting, social networking and making friends. This platform is meant only for asking subject specific and study related questions.
Be nice and polite and avoid rude and abusive language. Avoid inappropriate language and attention, vulgar terms and anything sexually suggestive. Avoid harassment and bullying.
Ask specific question which are clear and concise.

Remember the goal of this website is to share knowledge and learn from each other. Ask questions and help others by answering questions.

ANSWER

If one zero of the quadratic polynomial x²+3x+k is 2 then the value of k is-

Posted by Ankita Bera 2 years, 1 month ago

CBSE > Class 10 > Mathematics

5 answers

Maya Shukla 2 years, 1 month ago

Answer hai -10

_Jass_ Mahey_ 2 years, 1 month ago

By joining B and D. You will get two triangles AND and BCD. Now the area of ∆ABD = 1/2 (-5(-5-5)+(-4) (5-7) +4(7+5) = 1/2(50+8+48) =106/2=53square units Also, the area of ∆BCD=1/2(-4(-6-5) -1(5+5) +4(-5+6) =1/2(44-10+4) =19 square units So, the area of quadrilateral ABCD=53+19=72square units [Note:to find thr area of a polygon, we divide it into triangular regions, which have no common area of these regions. ]

Priyom Shah 2 years, 1 month ago

-10

Sahil Singh 2 years, 1 month ago

This content has been hidden. One or more users have flagged this content as inappropriate. Once content is flagged, it is hidden from users and is reviewed by myCBSEguide team against our Community Guidelines. If content is found in violation, the user posting this content will be banned for 30 days from using Homework help section. Suspended users will receive error while adding question or answer. Question comments have also been disabled. Read community guidelines at https://mycbseguide.com/community-guidelines.html

Few rules to keep homework help section safe, clean and informative.

Don't post personal information, mobile numbers and other details.
Don't use this platform for chatting, social networking and making friends. This platform is meant only for asking subject specific and study related questions.
Be nice and polite and avoid rude and abusive language. Avoid inappropriate language and attention, vulgar terms and anything sexually suggestive. Avoid harassment and bullying.
Ask specific question which are clear and concise.

Remember the goal of this website is to share knowledge and learn from each other. Ask questions and help others by answering questions.

Vettri Vel 2 years, 1 month ago

Don't know that is the answer

ANSWER

Prove that sin⁴a+cos⁴a/1-2sin²acos²a=1

Posted by Roshan Gupta 2 years, 1 month ago

CBSE > Class 10 > Mathematics

2 answers

Rishika Kabra 2 years, 1 month ago

Sin²a+ cos²a)²= (sin²a)²+ (cos²a)²+2 sin²a cos²a=1 Sin⁴a+cos⁴a= 1-2sin²a cos²a 1-2 sin²a cos²a/1-2sin²a cos²a=1 1=1 LHS= RHS hence proved

Rishika Kabra 2 years, 1 month ago

(Sin²a+ cos²a)²= (sin²a)²+ (cos²a)²+2 sin²a cos²a

ANSWER

C.I F 15 25 6 25 35 11 35 45 22 45 55 23 55 65 14 65 75 5 Find mode

Posted by Abhinav Singh 2 years, 1 month ago

CBSE > Class 10 > Mathematics

1 answers

Ankit Class 2 years, 1 month ago

This content has been hidden. One or more users have flagged this content as inappropriate. Once content is flagged, it is hidden from users and is reviewed by myCBSEguide team against our Community Guidelines. If content is found in violation, the user posting this content will be banned for 30 days from using Homework help section. Suspended users will receive error while adding question or answer. Question comments have also been disabled. Read community guidelines at https://mycbseguide.com/community-guidelines.html

Few rules to keep homework help section safe, clean and informative.

Don't post personal information, mobile numbers and other details.
Don't use this platform for chatting, social networking and making friends. This platform is meant only for asking subject specific and study related questions.
Be nice and polite and avoid rude and abusive language. Avoid inappropriate language and attention, vulgar terms and anything sexually suggestive. Avoid harassment and bullying.
Ask specific question which are clear and concise.

Remember the goal of this website is to share knowledge and learn from each other. Ask questions and help others by answering questions.

ANSWER

A man has certain notes of denominations Rs. 20 and Rs. 5 which amount to Rs. 380. If the number of notes of each kind is interchanged, they amount to Rs. 60 less as before. Find the number of notes of each denomination.

Posted by Parth Patel 2 years, 1 month ago

CBSE > Class 10 > Mathematics

1 answers

Preeti Dabral 2 years, 1 month ago

Let’s consider the number of 20 rupee notes to be x
And the number of 5 rupee notes be y

Then, according to the given conditions, we have

20x+5y=380 … (i) and

5x+20y=380−60

⇒5x+20y=320 … (ii)

Now, multiplying eq(i) by 4 and subtracting with eq(ii), we get

(80x+20y)−(5x+20y)=1520−320

⇒75x=1200

$\begin{aligned} & \Rightarrow \mathrm{x}=\frac{1200}{75} \\ & \Rightarrow \mathrm{x}=16 \end{aligned}$

On substituting the value of x in eq(i), we get

20(16)+5y=380

⇒320+5y=380

⇒5y=380−320=60

$\begin{aligned} & \Rightarrow y=\frac{60}{5} \\ & \Rightarrow y=12 \end{aligned}$

Therefore,

The number of 20 rupee notes =16 and

The number of 5 rupee notes =12

ANSWER

Two tangent TP and TQ are drawn to a circle with centre O from an external point T. Prove that <PTQ = 2 angle OPQ

Posted by Manisha Choudhary 2 years, 1 month ago

CBSE > Class 10 > Mathematics

1 answers

Preeti Dabral 2 years, 1 month ago

Given A circle with centre O and an external point T and two tangents TP and TQ to the circle, where P, Q are the points of contact.
To Prove: $\angle$ PTQ = 2 $\angle$ OPQ
Proof: Let $\angle$ PTQ = $\theta$
Since TP, TQ are tangents drawn from point T to the circle.
TP = TQ
$\therefore$ TPQ is an isoscles triangle
$\therefore$ $\angle$ TPQ = $\angle$ TQP = $\frac12$ (180^o - $\theta$ ) = 90^o - $\fracθ2$
Since, TP is a tangent to the circle at point of contact P
$\therefore$ $\angle$ OPT = 90^o
$\therefore$ $\angle$ OPQ = $\angle$ OPT - $\angle$ TPQ = 90^o - (90^o - $\frac12$ $\theta$ ) = $\fracθ2$ = $\frac12$ $\angle$ PTQ
Thus, $\angle$ PTQ = 2 $\angle$ OPQ

ANSWER

If alpha and beta are the zeroes of the quadratic polynomial f(x) = x²-4x+3 then find the value of (alpha⁴beta²+alpha²beta⁴)

Posted by Divyansh Hans 2 years, 1 month ago

CBSE > Class 10 > Mathematics

1 answers

Preeti Dabral 2 years, 1 month ago

Solution is given below

ANSWER

Simplest form of √(1-cos^2 ) (1+tan^2) is

Posted by Vikash Yadav 2 years, 1 month ago

CBSE > Class 10 > Mathematics

1 answers

Raghav Soni 2 years, 1 month ago

Sec^2

ANSWER

Page 57 of 1974

myCBSEguide App

myCBSEguide

Trusted by 1 Crore+ Students

Test Generator

Test Generator

Create papers online. It's FREE.

CUET Mock Tests

CUET Mock Tests

75,000+ questions to practice only on myCBSEguide app

Google Play

Download myCBSEguide App