Ask questions which are clear, concise and easy to understand.
Ask QuestionPosted by Rishith Bhardwaj 5 years, 11 months ago
- 2 answers
Posted by Kiran Devi 5 years, 11 months ago
- 1 answers
Posted by Kiran Devi 5 years, 11 months ago
- 0 answers
Posted by Priyanshi Agarwal 5 years, 11 months ago
- 2 answers
Posted by Sourabh Sharma 5 years, 11 months ago
- 1 answers
Yogita Ingle 5 years, 11 months ago
The mean (or average) of a number of observations is the sum of the values of all the observations divided by the total number of observations.
Median: Let’s arrange their score in ascending order in blue table. Now we will take the middle most score. Since we are talking about central tendency of data, middle most score should also reflect central tendency of data.
When the number of observations (n) is odd, the median is the value of the ((n+1)/2) Th observation.
When the number of observations (n) is even, the median is the mean of (n/2) & (n/2 + 1) Th observations.
Posted by Pankaj Tyagi 5 years, 11 months ago
- 1 answers
Gaurav Seth 5 years, 11 months ago
In point A(1, -1), x-coordinate is positive and y-coordinate is negative, so it lies in IV quadrant. In point B(4, 5), both coordinates are positive, so it lies in I quadrant. On plotting these point, we get the following graph.
(i) On joining the points A and B, we get the line segment AB. Now, to find the coordinates of a point on this line segment between A and B draw a perpendicular to X-axis from x = 2 and 3.
[since, x = 2 and 3 lies between A and B] say it intersect line segment AB at P and p’. Now, draw a perpendicular to Y-axis from P and p’, they intersect Y-axis at y = 1 and 3, respectively. Thus, we get points (2,1) and (3, 3) which lie between line segment AB.
(ii) Extent the line segment AB. Now, draw a perpendicular to X-axis from x = 5, say it intersects extended line segment at Q. Again, draw a perpendicular to Y-axis from Q and it intersects Y-axis at y = 7. Thus, we get the point Q(5,7) which lies outside the line segment AB.
Posted by Chinky Bhan 5 years, 11 months ago
- 1 answers
Sia ? 5 years, 11 months ago
4+√54−√5+4−√54+√5
=(4+√5)(4+√5)+(4−√5)(4−√5)(4−√5)(4+√5)
=(4+√5)2+(4−√5)2(4−√5)(4+√5)
={(4)2+2(4)(√5)+(√5)2}+{(4)2−2(4)(√5)+(√5)2}(4)2−(√5)2
=(16+8√5+5)+(16−8√5+5)16−5=4211
Posted by Harsh Gupta 5 years, 11 months ago
- 1 answers
Pramod Pant 5 years, 11 months ago
Posted by Shubhangi Mozarkar 5 years, 11 months ago
- 1 answers
Yogita Ingle 5 years, 11 months ago
5/7=0.7142 9/11=0.818181
first we have to change the the number into decimal or rational
decimal form of 5/7 is 0.71428571 and the decimal form of 9/11 is 81818181
hence the rational number are between 0.71428571 and 0.81818181
rational numbers between 5/7 and 9/11
1) 0.72428571
2) 0.73428571
3) 0.74428571
.
.
.
.
upto 0.80818181
you can take any number between them as irrational.
Posted by Naman Sen 5 years, 11 months ago
- 0 answers
Posted by Shivika Arora 5 years, 11 months ago
- 1 answers
Namrata Jindal 5 years, 11 months ago
Posted by Riya Suriyavanshi 5 years, 11 months ago
- 0 answers
Posted by Riya Suriyavanshi 5 years, 11 months ago
- 1 answers
Sia ? 5 years, 11 months ago
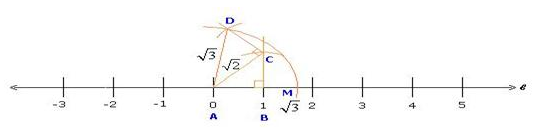
Take AB= BC = 1cm
∠B=900
In △ABC
AC2 = AB2 + BC2
AC2 = 1 + 1
AC2 = 2
AC = √2
In △OCD
∠C=900
OD2 = OC2 + DC2
OD2 = (√2)2 + 1
OD2 = 2 + 1
OD2 = 3
OD = √3
Posted by Dalveer Singh 5 years, 11 months ago
- 0 answers
Posted by Jashanjot K Jashan 5 years, 11 months ago
- 2 answers
Sia ? 5 years, 11 months ago
First rational number between 12 and 13
=12[12+13]⇒12[3+26]⇒512
= 12,512 and 13
Second rational number between12and13
= 12[12+512]⇒12[6+512]⇒1124
= 512and1124 are two rational numbers between 12and13
Sachin Ahlawat 5 years, 11 months ago
Posted by Shorya Sharma 5 years, 11 months ago
- 2 answers
Rupa Goel 5 years, 11 months ago
Posted by Pradeep Yadav 5 years, 11 months ago
- 0 answers
Posted by Dev Bhati 5 years, 11 months ago
- 0 answers
Posted by Keshav Deshmukh 5 years, 11 months ago
- 1 answers
Sia ? 5 years, 11 months ago
Given: Perimeter of rectangular wall = 2(l+b) = 250 m ……….(i)
Now Area of the four walls of the room
=Total cost to paint walls of the roomCost to paint 1 m2 of the walls
=1500010=1500m2 ……….(ii)
∵ Area of the four walls = Lateral surface area = 2(bh+hl)=2h(b+l) = 1500
⇒ 250×h=1500 [using eq. (i) and (ii)
⇒ h=1500250 = 6 m
Hence required height of the hall is 6 m.
Posted by Md Irshad 5 years, 11 months ago
- 3 answers
Sachin Ahlawat 5 years, 11 months ago
Posted by Shreya Maheshwari 5 years, 11 months ago
- 0 answers
Posted by Naresh Mittal 5 years, 11 months ago
- 0 answers
Posted by Sudha Nandakumar 5 years, 11 months ago
- 2 answers
Md Irshad 5 years, 11 months ago
Posted by Ayisha Deora 5 years, 11 months ago
- 0 answers
Posted by Krishna Aggarwal 5 years, 11 months ago
- 1 answers
Sameeksha V H 5 years, 11 months ago
Posted by Shruti Narasimhan 5 years, 11 months ago
- 0 answers
Posted by Nikshit Joshi 5 years, 11 months ago
- 2 answers
Sia ? 5 years, 11 months ago
A rational number lying between 36 and 25
36+252=15+12302=27302=2760
Sakshi Sahu 5 years, 11 months ago
Posted by Simrandeep Kaur 5 years, 11 months ago
- 3 answers
Posted by Sumit Thakur 5 years, 11 months ago
- 0 answers

myCBSEguide
Trusted by 1 Crore+ Students

Test Generator
Create papers online. It's FREE.

CUET Mock Tests
75,000+ questions to practice only on myCBSEguide app
 myCBSEguide
myCBSEguide
Annesha Mondal 5 years, 11 months ago
1Thank You